Homework Help is Here – Start Your Trial Now!
Math
Statistics
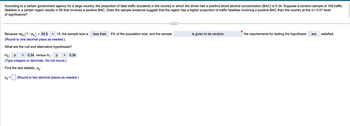
According to a certain government agency for a large country, the proportion of fatal traffic accidents in the country in which the driver had a positive blood alcohol concentration (BAC) is 0.34. Suppose a random sample of 109 traffic fatalities in a certain region results in 50 that involved a positive BAC. Does the sample evidence suggest that the region has a higher proportion of traffic fatalities involving a positive BAC than the country at the α = 0.01 level of significance? Because npo (1-Po) =10, the sample size is (Round to one decimal place as needed.) 5% of the population size, and the sample the requirements for testing the hypothesis ▼satisfied.

According to a certain government agency for a large country, the proportion of fatal traffic accidents in the country in which the driver had a positive blood alcohol concentration (BAC) is 0.34. Suppose a random sample of 109 traffic fatalities in a certain region results in 50 that involved a positive BAC. Does the sample evidence suggest that the region has a higher proportion of traffic fatalities involving a positive BAC than the country at the α = 0.01 level of significance? Because npo (1-Po) =10, the sample size is (Round to one decimal place as needed.) 5% of the population size, and the sample the requirements for testing the hypothesis ▼satisfied.

MATLAB: An Introduction with Applications
MATLAB: An Introduction with Applications
6th Edition
ISBN:9781119256830
Author:Amos Gilat
Publisher:Amos Gilat
Chapter1: Starting With Matlab
Section: Chapter Questions
Problem 1P
See similar textbooks
icon
Related questions
Question
According to a certain government agency for a large country, the proportion of fatal traffic accidents in the country in which the driver had a positive blood alcohol concentration (BAC) is 0.34. Suppose a random sample of 109 traffic fatalities in a certain region results in 50 that involved a positive BAC. Does the sample evidence suggest that the region has a higher proportion of traffic fatalities involving a positive BAC than the country at the α = 0.01 level of significance? = Because npo (1 - Po) 10, the sample size is (Round to one decimal place as needed.) 5% of the population size, and the sample the requirements for testing the hypothesis satisfied.
Transcribed Image Text:According to a certain government agency for a large country, the proportion of fatal traffic accidents in the country in which the driver had a positive blood alcohol concentration (BAC) is 0.34. Suppose a random sample of 109 traffic fatalities in a certain region results in 50 that involved a positive BAC. Does the sample evidence suggest that the region has a higher proportion of traffic fatalities involving a positive BAC than the country at the α = 0.01 level of significance? = Because npo (1 - Po) 10, the sample size is (Round to one decimal place as needed.) 5% of the population size, and the sample the requirements for testing the hypothesis satisfied.
Expert Solution
trending now

Trending now

This is a popular solution!

steps

Step by step

Solved in 7 steps with 10 images

SEE SOLUTIONCheck out a sample Q&A here
Blurred answer
Follow-up Questions
Read through expert solutions to related follow-up questions below.
Follow-up Question
According to a certain government agency for a large country, the proportion of fatal traffic accidents in the country in which the driver had a positive blood alcohol concentration (BAC) is 0.34. Suppose a random sample of 109 traffic fatalities in a certain region results in 50 that involved a positive BAC. Does the sample evidence suggest that the region has a higher proportion of traffic fatalities involving a positive BAC than the country at the x = 0.01 level of significance? Because npo (1-Po) = 24.5 > 10, the sample size is (Round to one decimal place as needed.) What are the null and alternative hypotheses? p = 0.34 versus H₁: p > 0.34 Ho: p (Type integers or decimals. Do not round.) Find the test statistic, Zo. Zo = 2.62 (Round to two decimal places as needed.) Find the P-value. P-value = (Round to three decimal places as needed.) less than 5% of the population size, and the sample is given to be random, the requirements for testing the hypothesis are satisfied.
Transcribed Image Text:According to a certain government agency for a large country, the proportion of fatal traffic accidents in the country in which the driver had a positive blood alcohol concentration (BAC) is 0.34. Suppose a random sample of 109 traffic fatalities in a certain region results in 50 that involved a positive BAC. Does the sample evidence suggest that the region has a higher proportion of traffic fatalities involving a positive BAC than the country at the x = 0.01 level of significance? Because npo (1-Po) = 24.5 > 10, the sample size is (Round to one decimal place as needed.) What are the null and alternative hypotheses? p = 0.34 versus H₁: p > 0.34 Ho: p (Type integers or decimals. Do not round.) Find the test statistic, Zo. Zo = 2.62 (Round to two decimal places as needed.) Find the P-value. P-value = (Round to three decimal places as needed.) less than 5% of the population size, and the sample is given to be random, the requirements for testing the hypothesis are satisfied.
Solution
Bartleby Expert
SEE SOLUTION
Follow-up Question
According to a certain government agency for a large country, the proportion of fatal traffic accidents in the country in which the driver had a positive blood alcohol concentration (BAC) is 0.34. Suppose a random sample of 109 traffic fatalities in a certain region results in 50 that involved a positive BAC. Does the sample evidence suggest that the region has a higher proportion of traffic fatalities involving a positive BAC than the country at the x = 0.01 level of significance? Because npo (1-Po = 24.5 > 10, the sample size is (Round to one decimal place as needed.) What are the null and alternative hypotheses? p = 0.34 versus H₁: p> 0.34 Ho: P (Type integers or decimals. Do not round.) Find the test statistic, Zo. Zo (Round to two decimal places as needed.) = less than 5% of the population size, and the sample is given to be random, the requirements for testing the hypothesis are satisfied.
Transcribed Image Text:According to a certain government agency for a large country, the proportion of fatal traffic accidents in the country in which the driver had a positive blood alcohol concentration (BAC) is 0.34. Suppose a random sample of 109 traffic fatalities in a certain region results in 50 that involved a positive BAC. Does the sample evidence suggest that the region has a higher proportion of traffic fatalities involving a positive BAC than the country at the x = 0.01 level of significance? Because npo (1-Po = 24.5 > 10, the sample size is (Round to one decimal place as needed.) What are the null and alternative hypotheses? p = 0.34 versus H₁: p> 0.34 Ho: P (Type integers or decimals. Do not round.) Find the test statistic, Zo. Zo (Round to two decimal places as needed.) = less than 5% of the population size, and the sample is given to be random, the requirements for testing the hypothesis are satisfied.
Solution
Bartleby Expert
SEE SOLUTION
Follow-up Question
According to a certain government agency for a large country, the proportion of fatal traffic accidents in the country in which the driver had a positive blood alcohol concentration (BAC) is 0.34. Suppose a random sample of 109 traffic fatalities in a certain region results in 50 that involved a positive BAC. Does the sample evidence suggest that the region has a higher proportion of traffic fatalities involving a positive BAC than the country at the α = 0.01 level of significance? Because npo (1-Po) = 24.5 > 10, the sample size is (Round to one decimal place as needed.) What are the null and alternative hypotheses? Ho: versus H₁: (Type integers or decimals. Do not round.) less than 5% of the population size, and the sample is given to be random, the requirements for testing the hypothesis are satisfied.
Transcribed Image Text:According to a certain government agency for a large country, the proportion of fatal traffic accidents in the country in which the driver had a positive blood alcohol concentration (BAC) is 0.34. Suppose a random sample of 109 traffic fatalities in a certain region results in 50 that involved a positive BAC. Does the sample evidence suggest that the region has a higher proportion of traffic fatalities involving a positive BAC than the country at the α = 0.01 level of significance? Because npo (1-Po) = 24.5 > 10, the sample size is (Round to one decimal place as needed.) What are the null and alternative hypotheses? Ho: versus H₁: (Type integers or decimals. Do not round.) less than 5% of the population size, and the sample is given to be random, the requirements for testing the hypothesis are satisfied.
Solution
Bartleby Expert
SEE SOLUTION
Similar questions
  • SEE MORE QUESTIONS
Recommended textbooks for you
MATLAB: An Introduction with Applications
MATLAB: An Introduction with Applications
Statistics
ISBN:
9781119256830
Author:
Amos Gilat
Publisher:
John Wiley & Sons Inc
Probability and Statistics for Engineering and th…
Probability and Statistics for Engineering and th…
Statistics
ISBN:
9781305251809
Author:
Jay L. Devore
Publisher:
Cengage Learning
Statistics for The Behavioral Sciences (MindTap C…
Statistics for The Behavioral Sciences (MindTap C…
Statistics
ISBN:
9781305504912
Author:
Frederick J Gravetter, Larry B. Wallnau
Publisher:
Cengage Learning
Elementary Statistics: Picturing the World (7th E…
Elementary Statistics: Picturing the World (7th E…
Statistics
ISBN:
9780134683416
Author:
Ron Larson, Betsy Farber
Publisher:
PEARSON
The Basic Practice of Statistics
The Basic Practice of Statistics
Statistics
ISBN:
9781319042578
Author:
David S. Moore, William I. Notz, Michael A. Fligner
Publisher:
W. H. Freeman
Introduction to the Practice of Statistics
Introduction to the Practice of Statistics
Statistics
ISBN:
9781319013387
Author:
David S. Moore, George P. McCabe, Bruce A. Craig
Publisher:
W. H. Freeman
SEE MORE TEXTBOOKS