Hello,Can someone please solve this problem? ## Snowball Inelastic Collision Problem**Context:** Snowballs make perfectly inelastic collisions; when two snowballs slam into each other, they continue as one bigger snowball. In this problem, you and your friend each throw a snowball of the same mass \(m\) and speed, with horizontal velocities \(v_1\) and \(v_2\). They collide mid-air. 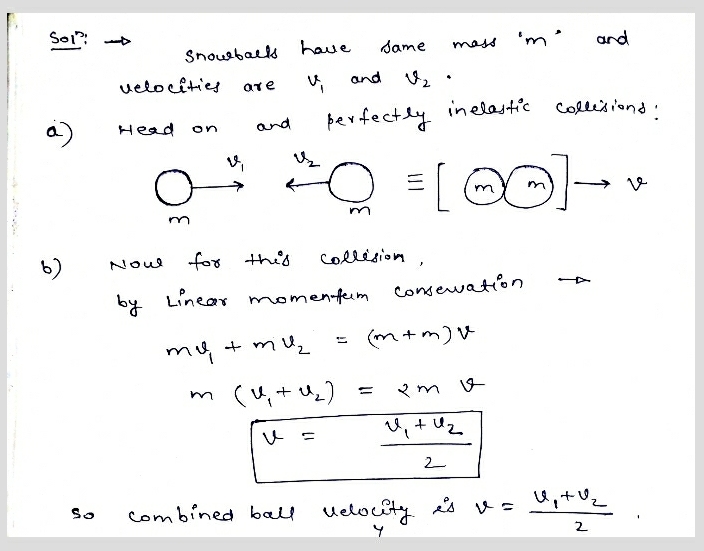We'll skip considering vertical motion due to gravitational acceleration.### Tasks:**a. Head-On Collision:** - You and your friend are on opposing teams; the snowballs meet head-on.- **Task:** Make a drawing of the situation as viewed from above.**b. Velocity After Head-On Collision:** - **Question:** With which velocity is the combined snowball moving?**c. Joining the Same Team:** - Now, your friend joins your team, and the snowballs move in the same direction, with a small angle \(\alpha\) between them.- **Task:** Make a drawing of this situation as seen from above.**d. Direction of Combined Snowball:** - **Question:** Which direction is the combined snowball moving now?**e. Speed of Combined Snowball:** - **Question:** With which speed is the combined snowball moving?### Considerations:For both scenarios (a and c), illustrations will help visualize the collisions:- **Head-on collision (a):** Expect two snowballs moving directly towards each other.- **Same direction with angle (c):** Position vectors at a small angle \(\alpha\), converging post-collision.**Concepts to Explore:**- Conservation of momentum for perfectly inelastic collisions.- Vector addition for finding resultant directions and velocities in part (c).Understanding these physical principles will aid in solving problems involving inelastic collisions and vector motion analysis.

Answered: Image /qna-images/answer/7b2b58be-13c6-4af2-bce3-acbd4bfab1eb.jpg

5) Snowballs make perfectly inelastic collisions; when two snowballs slam into each other, they continue on as one bigger snowball. You and your friend each throw a snowball of the same mass m and speed with horizontal velocities V₁ and v2; they collide mid-air. Let's skip considering the vertical motion due to gravitational acceleration. a. First, you and your friend are on opposing teams; and so, the snowballs meet head-on. Make a drawing of the situation (such as looking on from above). b. With which velocity is the combined snowball moving? c. Now, your friend is joining your team, and so the two snowballs move generally in the same direction, but with a small angle a between them. Make a drawing of the situation (looking on from above). d. Which direction is the combined snowball moving now? e. With which speed is the combined snowball moving?

5) Snowballs make perfectly inelastic collisions; when two snowballs slam into each other, they continue on as one bigger snowball. You and your friend each throw a snowball of the same mass m and speed with horizontal velocities V₁ and v2; they collide mid-air. Let's skip considering the vertical motion due to gravitational acceleration. a. First, you and your friend are on opposing teams; and so, the snowballs meet head-on. Make a drawing of the situation (such as looking on from above). b. With which velocity is the combined snowball moving? c. Now, your friend is joining your team, and so the two snowballs move generally in the same direction, but with a small angle a between them. Make a drawing of the situation (looking on from above). d. Which direction is the combined snowball moving now? e. With which speed is the combined snowball moving?