2. For the 1-D wavefunction: $(x) = Nxe (=²7), the particle? (X¹) O(x)= Nxe , where -∞0 ≤x≤00, what is/are the most probable location(s) of -∞0≤x≤
2. For the 1-D wavefunction: $(x) = Nxe (=²7), the particle? (X¹) O(x)= Nxe , where -∞0 ≤x≤00, what is/are the most probable location(s) of -∞0≤x≤
Related questions
Question

Transcribed Image Text:3:49 PM Thu Sep 22
<
2. For the 1-D wavefunction: p(x) = Nxe\
the particle?
5
(x) = Nxe
<x> =
-
<x>= 4*^d ²
Pd
-∞
<x> = N²
Nxe
(X)
(2x²))
то
xe
X
, where -∞0 ≤ x ≤00, what is/are the most probable location(s) of
Nxe
( 201² ) ) x dx
2
(x²)
xdx
-∞0 ≤ x ≤ 0
1
x= x
38%
+:
2
6
Expert Solution
Step 1
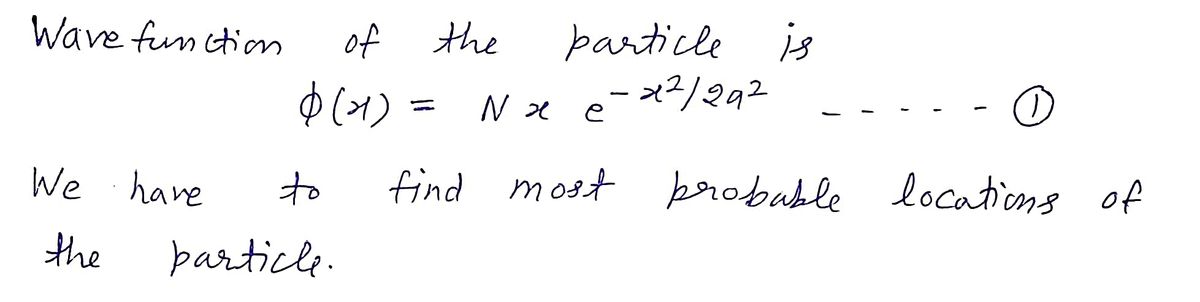
Step by step
Solved in 4 steps with 4 images
