Chapter 9.3, Problem 67AYU

Mandelbrot Sets

(a) Consider the expression $a_n={\left(a_{n-1}\right)}^2+z$, where $z$ is some complex number (called the seed) and $a_0=z$. Compute $a_1\left(=a_0^2+z\right)$, $a_2\left(=a_1^2+z\right)$, $a_3\left(=a_2^2+z\right)$, $a_4,a_5$ and $a_6$ for the following seeds: $z_1=0.1-0.4i$, $z_2=0.5+0.8i$, $z_3=-0.9+0.7i$, $z_4=-1.1+0.1i$, $z_5=0-1.3i$, and $z_6=1+1i$.

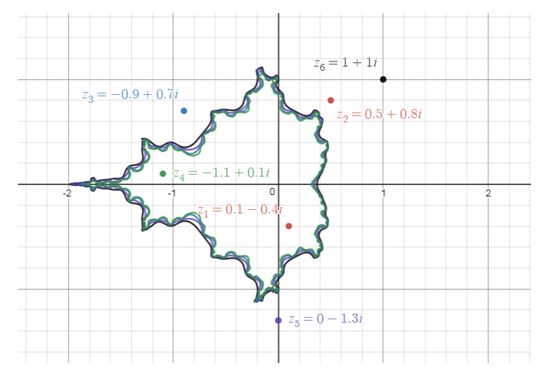
(b) The dark portion of the graph represents the set of all values $z=x+yi$ that are in the Mandelbrot set.

Determine which complex numbers in part (a) are in this set by plotting them on the graph. Do the complex numbers that are not in the Mandelbrot set have any common characteristics regarding the values of found in part (a)?

(c) Compute $\left|z\right|=\sqrt{x^2+y^2}$ for each of the complex numbers in part

(a). Now compute $\left|a_6\right|$ for each of the complex numbers in part (a).

For which complex numbers is $\left|a_6\right|\le \left|z\right|$ and $\left|z\right|\le 2$? Conclude that the criterion for a complex number to be in the Mandelbrot set is that $\left|a_n\right|\le \left|z\right|$ and $\left|z\right|\le 2$.

To determine

Which complex numbers are in this set by plotting them on the graph.

(ii) Do the complex numbers that are not in the Mandelbrot set have any common characteristics regarding the values of $a_6$.

(iii) compute $\left|z\right|$ for each of the complex numbers and $\left|a_6\right|$ for each complex numbers.

Answer to Problem 67AYU

Solution:

$\left|z_1\right|=\sqrt{{\left(0.1\right)}^2+{\left(-0.4\right)}^2}=\sqrt{0.17}$

$\left|z_2\right|=\sqrt{{\left(0.5\right)}^2+{\left(0.8\right)}^2}=\sqrt{0.89}$

$\left|z_3\right|=\sqrt{{\left(-0.9\right)}^2+{\left(0.7\right)}^2}=\sqrt{1.3}$

$\left|z_4\right|=\sqrt{{\left(-1.1\right)}^2+{\left(0.1\right)}^2}=\sqrt{1.22}$

$\left|z_5\right|=\sqrt{{\left(0\right)}^2+{\left(-1.3\right)}^2}=\sqrt{1.69}$

$\left|z_6\right|=\sqrt{{\left(1\right)}^2+{\left(1\right)}^2}=\sqrt{2}$

Explanation of Solution

$a_0=z$

$a_1=a_0^2+z=z^2+z$

$a_2=a_1^2+z=z^2+z+z=z^2+2z$

$⋮$

$a_6=a_5^2+z=z^2+6z$

From the above values each of the $a$ values increase by $z$.

$\left|z_1\right|=\sqrt{{\left(0.1\right)}^2+{\left(-0.4\right)}^2}=\sqrt{0.17}$

$\left|z_2\right|=\sqrt{{\left(0.5\right)}^2+{\left(0.8\right)}^2}=\sqrt{0.89}$

$\left|z_3\right|=\sqrt{{\left(-0.9\right)}^2+{\left(0.7\right)}^2}=\sqrt{1.3}$

$\left|z_4\right|=\sqrt{{\left(-1.1\right)}^2+{\left(0.1\right)}^2}=\sqrt{1.22}$

$\left|z_5\right|=\sqrt{{\left(0\right)}^2+{\left(-1.3\right)}^2}=\sqrt{1.69}$

$\left|z_6\right|=\sqrt{{\left(1\right)}^2+{\left(1\right)}^2}=\sqrt{2}$