
Air Quality
As a researcher for the EPA, you have been asked to determine if the air quality in the United States has changed over the past 2 years. You select a random sample of 10 metropolitan areas and find the number of days each year that the areas failed to meet acceptable air quality standards. The data are shown.
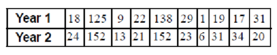
Source: The World Almanac and Book of Facts.
Based on the data, answer the following questions.
1. What is the purpose of the study?
2. Are the samples independent or dependent?
3. What hypotheses would you use?
4. What is (are) the critical value(s) that you would use?
5. What statistical test would you use?
6. How many degrees of freedom are there?
7. What is your conclusion?
8. Could an independent means test have been used?
9. Do you think this was a good way to answer the original question?
1.
To find: The purpose of the study.
Explanation of Solution
The purpose of the givens study is “to determine if the air quality in the United States has changed over the past 2 years”.
2.
To classify: The samples as independent or dependent.
Answer to Problem 1AC
The samples are dependent.
Explanation of Solution
Independent samples:
If the sample values from one population do not associate with the sample values from other population, then the two samples are said to be independent samples.
Dependent samples:
If the sample values from one population associated or matched with the sample values from other population, then the two samples are said to be dependent samples.
Matched pair design occurred at two situations, which are listed below:
- Subjects are matched with pairs and each treatment is given to one subject in each pair.
- Before and after observations on the same subjects.
Here, the samples are dependent because same metropolitan areas are taken and the samples that are related. Thus, it can be concluded that the samples are dependent.
3.
To find: The hypotheses of the study.
Answer to Problem 1AC
Hypotheses:
Null hypothesis:
Alternative hypothesis:
Explanation of Solution
Null hypothesis:
Null hypothesis is a statement about population parameter, its value is equal to the claim value, which is denoted by
Alternative hypothesis:
It is complementary to the null hypothesis. That is, it differs from the null hypothesis. The possible symbols used in the alternative hypothesis would be <,>, or ≠. It is denoted by
State the null and alternative hypotheses:
Null hypothesis:
Alternative hypothesis:
4.
To find: The critical value.
Answer to Problem 1AC
The critical value is ±2.262.
Explanation of Solution
Calculation:
Degrees of freedom:
Software Procedure:
Step-by-step procedure to obtain the critical value using the MINITAB software:
- Choose Graph > Probability Distribution Plot choose View Probability> OK.
- From Distribution, choose ‘t’ distribution.
- In Degrees of freedom, enter 9.
- Click the Shaded Area tab.
- Choose Probability value and Both Tail for the region of the curve to shade.
- Enter the Probability value as 0.05.
- Click OK.
Output using the MINITAB software is given below:
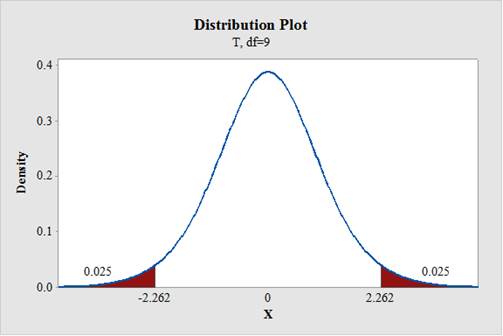
From the output, the critical value is ±2.262.
5.
To find: The statistical test.
Answer to Problem 1AC
The t test for dependent samples can be used.
Explanation of Solution
Here, the samples are dependent because same metropolitan areas are taken and the samples that are related.
Thus, the t test for dependent samples can be used.
6.
To find: The degrees of freedom.
Answer to Problem 1AC
The degrees of freedom is 9.
Explanation of Solution
Calculation:
Degrees of freedom:
Thus, the degrees of freedom is 9.
7.
To describe: The conclusion.
Answer to Problem 1AC
The conclusion is that there is no enough evidence to support the claim that the air quality in the United States has changed over the past 2 years.
Explanation of Solution
Calculation:
Software Procedure:
Step-by-step procedure to obtain the test value using the MINITAB software:
- Choose Stat > Basic Statistics > 1-Sample t.
- In Samples in Column, enter the column of Difference.
- In Perform hypothesis test, enter the test mean as 0.
- Check Options; enter Confidence level as 95%.
- Choose not equal in alternative.
- Click OK.
Output using the MINITAB software is given below:
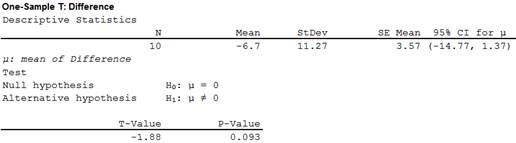
From the output, the test value is –1.88.
Decision:
Decision rule:
If
If
Here, the value of test statistic is greater than the critical value.
That is,
Therefore, the null hypothesis is not rejected,
Thus, the decision is “fail to reject the null hypothesis”.
Hence, there is no enough evidence to support the claim that the air quality in the United States has changed over the past 2 years.
8.
To check: Whether the independent test can be used.
Answer to Problem 1AC
The independent means test cannot be used.
Explanation of Solution
Here, each metropolitan area had two readings. That is, the samples are related. Thus, the independent means test cannot be used.
9.
To describe: The result.
Explanation of Solution
Answer will vary. One of the possible answers is given below:
The answer is that there are other measures of air quality in the U.S that could have examined to answer the original question.
Want to see more full solutions like this?
Chapter 9 Solutions
Loose Leaf for Elementary Statistics: A Step By Step Approach
- Suppose we wish to test the hypothesis that women with a sister’s history of breast cancer are at higher risk of developing breast cancer themselves. Suppose we assume that the prevalence rate of breast cancer is 3% among 60- to 64-year-old U.S. women, whereas it is 5% among women with a sister history. We propose to interview 400 women 40 to 64 years of age with a sister history of the disease. What is the power of such a study assuming that the level of significance is 10%? I only need help writing the null and alternative hypotheses.arrow_forward4.96 The breaking strengths for 1-foot-square samples of a particular synthetic fabric are approximately normally distributed with a mean of 2,250 pounds per square inch (psi) and a standard deviation of 10.2 psi. Find the probability of selecting a 1-foot-square sample of material at random that on testing would have a breaking strength in excess of 2,265 psi.4.97 Refer to Exercise 4.96. Suppose that a new synthetic fabric has been developed that may have a different mean breaking strength. A random sample of 15 1-foot sections is obtained, and each section is tested for breaking strength. If we assume that the population standard deviation for the new fabric is identical to that for the old fabric, describe the sampling distribution forybased on random samples of 15 1-foot sections of new fabricarrow_forwardUne Entreprise œuvrant dans le domaine du multividéo donne l'opportunité à ses programmeurs-analystes d'évaluer la performance des cadres supérieurs. Voici les résultats obtenues (sur une échelle de 10 à 50) où 50 représentent une excellente performance. 10 programmeurs furent sélectionnés au hazard pour évaluer deux cadres. Un rapport Excel est également fourni. Programmeurs Cadre A Cadre B 1 34 36 2 32 34 3 18 19 33 38 19 21 21 23 7 35 34 8 20 20 9 34 34 10 36 34 Test d'égalité des espérances: observations pairéesarrow_forward
- A television news channel samples 25 gas stations from its local area and uses the results to estimate the average gas price for the state. What’s wrong with its margin of error?arrow_forwardYou’re fed up with keeping Fido locked inside, so you conduct a mail survey to find out people’s opinions on the new dog barking ordinance in a certain city. Of the 10,000 people who receive surveys, 1,000 respond, and only 80 are in favor of it. You calculate the margin of error to be 1.2 percent. Explain why this reported margin of error is misleading.arrow_forwardYou find out that the dietary scale you use each day is off by a factor of 2 ounces (over — at least that’s what you say!). The margin of error for your scale was plus or minus 0.5 ounces before you found this out. What’s the margin of error now?arrow_forward
- Suppose that Sue and Bill each make a confidence interval out of the same data set, but Sue wants a confidence level of 80 percent compared to Bill’s 90 percent. How do their margins of error compare?arrow_forwardSuppose that you conduct a study twice, and the second time you use four times as many people as you did the first time. How does the change affect your margin of error? (Assume the other components remain constant.)arrow_forwardOut of a sample of 200 babysitters, 70 percent are girls, and 30 percent are guys. What’s the margin of error for the percentage of female babysitters? Assume 95 percent confidence.What’s the margin of error for the percentage of male babysitters? Assume 95 percent confidence.arrow_forward
- You sample 100 fish in Pond A at the fish hatchery and find that they average 5.5 inches with a standard deviation of 1 inch. Your sample of 100 fish from Pond B has the same mean, but the standard deviation is 2 inches. How do the margins of error compare? (Assume the confidence levels are the same.)arrow_forwardA survey of 1,000 dental patients produces 450 people who floss their teeth adequately. What’s the margin of error for this result? Assume 90 percent confidence.arrow_forwardThe annual aggregate claim amount of an insurer follows a compound Poisson distribution with parameter 1,000. Individual claim amounts follow a Gamma distribution with shape parameter a = 750 and rate parameter λ = 0.25. 1. Generate 20,000 simulated aggregate claim values for the insurer, using a random number generator seed of 955.Display the first five simulated claim values in your answer script using the R function head(). 2. Plot the empirical density function of the simulated aggregate claim values from Question 1, setting the x-axis range from 2,600,000 to 3,300,000 and the y-axis range from 0 to 0.0000045. 3. Suggest a suitable distribution, including its parameters, that approximates the simulated aggregate claim values from Question 1. 4. Generate 20,000 values from your suggested distribution in Question 3 using a random number generator seed of 955. Use the R function head() to display the first five generated values in your answer script. 5. Plot the empirical density…arrow_forward
 Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill Big Ideas Math A Bridge To Success Algebra 1: Stu...AlgebraISBN:9781680331141Author:HOUGHTON MIFFLIN HARCOURTPublisher:Houghton Mifflin Harcourt
Big Ideas Math A Bridge To Success Algebra 1: Stu...AlgebraISBN:9781680331141Author:HOUGHTON MIFFLIN HARCOURTPublisher:Houghton Mifflin Harcourt


