
(a)
Interpretation:
The effect of condensation temperature on the coefficient of performance is to be determined for different condensation temperatures assuming isentropic compression of vapor.
Concept introduction:
Below shown diagram represents vapor-compression refrigeration cycle on a TS diagram which include four steps of the cycle. Line 1→2 shows a liquid which is evaporating at constant pressure and providing a means for heat absorption at constant low temperature. The vapor thus produced is then compressed to a higher pressure. It is then cooled and condensed at higher temperature by heat rejection. By an expansion process, the liquid from the condenser is returned to its original pressure. This process is carried out by throttling through a partly open valve. Due to the fluid friction in the valve there is pressure drop in this irreversible process.
The line 4→1 represents throttling process which occurs at constant enthalpy. The path of isentropic compression is shown by line 2→3' and the actual compression is shown by the line 2→3 where the direction of slope is in increasing enthalpy which reflects inherent irreversibility.
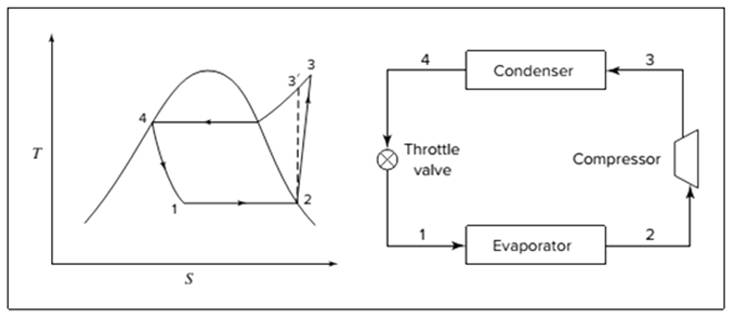
The equations used to calculate the heat absorbed in evaporator and the heat rejected in condenser are:
|QC|=H2−H1 ...... (1)
|QH|=H3−H4 ...... (2)
The work of compression is:
W=H3−H2 ...... (3)
The coefficient of performance is:
ω=H2−H1H3−H2 ...... (4)
The rate of circulation of refrigerant, ˙m is determined from the rate of heat absorption in the evaporator given by the equation:
˙m=|˙QC|H2−H1 ...... (5)
For Carnot refrigeration cycle, highest possible value of ω is attained at the given values of TC and TH . Due to irreversible expansion in a throttle valve and irreversible compression in the vapor-compression cycle, lower values of ω is obtained.
(a)
Answer to Problem 9.13P
The coefficient of performance for condensation temperature of 16∘C is 9.049 .
The coefficient of performance for condensation temperature of 28∘C is 5.729 .
The coefficient of performance for condensation temperature of 40∘C is 3.737 .
As the condensation temperature is increased, the coefficient of performance decreases.
Explanation of Solution
Given information:
In a refrigerator, tetrafluoroethene acts as a refrigerant and operates with an evaporation temperature of −12∘C . Different condensation temperature for the isentropic compression of vapor are 16∘C, 28∘C, and 40∘C .
From table 9.1, the values of H2, and S2 and the pressure for the saturated tetrafluoroethene at −12∘C are:
H2=391.46 kJ/kgS2=1.735 kJ/(kg⋅K)P2=1.852 bar
Assume that the compressor efficiency is η=1.00 , so,
H3=H′3
For condensation temperature of 16∘C .
The saturation pressure at point 4 is the pressure at which the vapor condenses and H4(16∘C) at this point is:
Psat(16∘C)=5.043 barH4(16∘C)=221.87 kJ/kg
Also, H1(16∘C)=H4(16∘C)=221.87 kJ/kg
For isentropic compression,
S′3=S2=1.735 kJ/(kg⋅K)
At point 3′ , the thermodynamic state properties are defines using the saturation pressure. From figure F.2 of appendix F, follow the constant enthalpy curve of 1.735 kJ/(kg⋅K) to get the enthalpy H3(16∘C) at pressure 5.043 bar as:
H3(16∘C)=410.2 kJ/kg
Using equation (4), the coefficient of performance for condensation temperature of 16∘C is calculated as:
ω(16∘C)=H2−H1(16∘C)H3(16∘C)−H2=391.46−221.87410.2−391.46=9.049
For condensation temperature of 28∘C .
The saturation pressure at point 4 is the pressure at which the vapor condenses and H4(28∘C) at this point is:
Psat(28∘C)=7.269 barH4(28∘C)=238.84 kJ/kg
Also, H1(28∘C)=H4(28∘C)=238.84 kJ/kg
For isentropic compression,
S′3=S2=1.735 kJ/(kg⋅K)
At point 3′ , the thermodynamic state properties are defines using the saturation pressure. From figure F.2 of appendix F, follow the constant enthalpy curve of 1.735 kJ/(kg⋅K) to get the enthalpy H3(28∘C) at pressure 7.269 bar as:
H3(28∘C)=418.1 kJ/kg
Using equation (4), the coefficient of performance for condensation temperature of 28∘C is calculated as:
ω(28∘C)=H2−H1(28∘C)H3(28∘C)−H2=391.46−238.84418.1−391.46=5.729
For condensation temperature of 40∘C .
The saturation pressure at point 4 is the pressure at which the vapor condenses and H4(40∘C) at this point is:
Psat(40∘C)=10.166 barH4(40∘C)=256.41 kJ/kg
Also, H1(40∘C)=H4(40∘C)=256.41 kJ/kg
For isentropic compression,
S′3=S2=1.735 kJ/(kg⋅K)
At point 3′ , the thermodynamic state properties are defines using the saturation pressure. From figure F.2 of appendix F, follow the constant enthalpy curve of 1.735 kJ/(kg⋅K) to get the enthalpy H3(40∘C) at pressure 10.166 bar as:
H3(40∘C)=427.6 kJ/kg
Using equation (4), the coefficient of performance for condensation temperature of 40∘C is calculated as:
ω(40∘C)=H2−H1(40∘C)H3(40∘C)−H2=391.46−256.41427.6−391.46=3.737
As the condensation temperature is increased, the coefficient of performance decreases.
(b)
Interpretation:
The effect of condensation temperature on the coefficient of performance is to be determined for different condensation temperatures assuming compressor efficiency of 75% .
Concept introduction:
Below shown diagram represents vapor-compression refrigeration cycle on a TS diagram which include four steps of the cycle. Line 1→2 shows a liquid which is evaporating at constant pressure and providing a means for heat absorption at constant low temperature. The vapor thus produced is then compressed to a higher pressure. It is then cooled and condensed at higher temperature by heat rejection. By an expansion process, the liquid from the condenser is returned to its original pressure. This process is carried out by throttling through a partly open valve. Due to the fluid friction in the valve there is pressure drop in this irreversible process.
The line 4→1 represents throttling process which occurs at constant enthalpy. The path of isentropic compression is shown by line 2→3' and the actual compression is shown by the line 2→3 where the direction of slope is in increasing enthalpy which reflects inherent irreversibility.
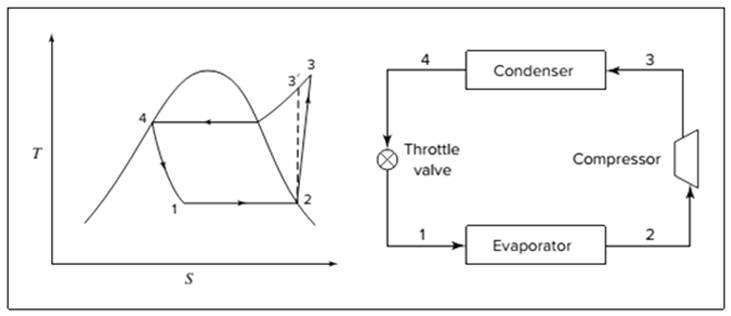
The equations used to calculate the heat absorbed in evaporator and the heat rejected in condenser are:
|QC|=H2−H1 ...... (1)
|QH|=H3−H4 ...... (2)
The work of compression is:
W=H3−H2 ...... (3)
The coefficient of performance is:
ω=H2−H1H3−H2 ...... (4)
The rate of circulation of refrigerant, ˙m is determined from the rate of heat absorption in the evaporator given by the equation:
˙m=|˙QC|H2−H1 ...... (5)
For Carnot refrigeration cycle, highest possible value of ω is attained at the given values of TC and TH . Due to irreversible expansion in a throttle valve and irreversible compression in the vapor-compression cycle, lower values of ω is obtained.
(b)
Answer to Problem 9.13P
The coefficient of performance for condensation temperature of 16∘C is 6.786 .
The coefficient of performance for condensation temperature of 28∘C is 4.297 .
The coefficient of performance for condensation temperature of 40∘C is 2.802 .
As the condensation temperature is increased, the coefficient of performance decreases.
Explanation of Solution
Given information:
In a refrigerator, tetrafluoroethene acts as a refrigerant and operates with an evaporation temperature of −12∘C . Different condensation temperature for the compressor efficiency of 75% are 16∘C, 28∘C, and 40∘C .
From table 9.1, the values of H2, and S2 and the pressure for the saturated tetrafluoroethene at −12∘C are:
H2=391.46 kJ/kgS2=1.735 kJ/(kg⋅K)P2=1.852 bar
The compressor efficiency is given as, η=0.75 .
For condensation temperature of 16∘C .
The saturation pressure at point 4 is the pressure at which the vapor condenses and H4(16∘C) at this point is:
Psat(16∘C)=5.043 barH4(16∘C)=221.87 kJ/kg
Also, H1(16∘C)=H4(16∘C)=221.87 kJ/kg
For isentropic compression,
S′3=S2=1.735 kJ/(kg⋅K)
At point 3′ , the thermodynamic state properties are defines using the saturation pressure. From figure F.2 of appendix F, follow the constant enthalpy curve of 1.735 kJ/(kg⋅K) to get the enthalpy H′3(16∘C) at pressure 5.043 bar as:
H′3(16∘C)=410.2 kJ/kg
Calculate ΔH23(16∘C) as:
ΔH23(16∘C)=H′3 (16∘C)−H2η=410.2−391.460.75=24.99 kJ/kg
Now, calculate H3(16∘C) as:
H3(16∘C)=H2+ΔH23(16∘C)=391.46+24.99=416.45 kJ/kg
Using equation (4), the coefficient of performance for condensation temperature of 16∘C is calculated as:
ω(16∘C)=H2−H1(16∘C)H3(16∘C)−H2=391.46−221.87416.45−391.46=6.786
For condensation temperature of 28∘C .
The saturation pressure at point 4 is the pressure at which the vapor condenses and H4(28∘C) at this point is:
Psat(28∘C)=7.269 barH4(28∘C)=238.84 kJ/kg
Also, H1(28∘C)=H4(28∘C)=238.84 kJ/kg
For isentropic compression,
S′3=S2=1.735 kJ/(kg⋅K)
At point 3′ , the thermodynamic state properties are defines using the saturation pressure. From figure F.2 of appendix F, follow the constant enthalpy curve of 1.735 kJ/(kg⋅K) to get the enthalpy H′3(28∘C) at pressure 7.269 bar as:
H′3(28∘C)=418.1 kJ/kg
Calculate ΔH23(28∘C) as:
ΔH23(28∘C)=H′3 (28∘C)−H2η=418.1−391.460.75=35.52 kJ/kg
Now, calculate H3(28∘C) as:
H3(28∘C)=H2+ΔH23(28∘C)=391.46+35.52=426.98 kJ/kg
Using equation (4), the coefficient of performance for condensation temperature of 28∘C is calculated as:
ω(28∘C)=H2−H1(28∘C)H3(28∘C)−H2=391.46−238.84426.98−391.46=4.297
For condensation temperature of 40∘C .
The saturation pressure at point 4 is the pressure at which the vapor condenses and H4(40∘C) at this point is:
Psat(40∘C)=10.166 barH4(40∘C)=256.41 kJ/kg
Also, H1(40∘C)=H4(40∘C)=256.41 kJ/kg
For isentropic compression,
S′3=S2=1.735 kJ/(kg⋅K)
At point 3′ , the thermodynamic state properties are defines using the saturation pressure. From figure F.2 of appendix F, follow the constant enthalpy curve of 1.735 kJ/(kg⋅K) to get the enthalpy H′3(40∘C) at pressure 10.166 bar as:
H′3(40∘C)=427.6 kJ/kg
Calculate ΔH23(40∘C) as:
ΔH23(40∘C)=H′3 (40∘C)−H2η=427.6−391.460.75=48.19 kJ/kg
Now, calculate H3(40∘C) as:
H3(40∘C)=H2+ΔH23(40∘C)=391.46+48.19=439.65 kJ/kg
Using equation (4), the coefficient of performance for condensation temperature of 40∘C is calculated as:
ω(40∘C)=H2−H1(40∘C)H3(40∘C)−H2=391.46−256.41439.65−391.46=2.802
As the condensation temperature is increased, the coefficient of performance decreases.
Want to see more full solutions like this?
Chapter 9 Solutions
Loose Leaf For Introduction To Chemical Engineering Thermodynamics
- For spherical sand particles with Dp = 0.03 and ρparticles = 150 lbm / ft3 estimate the minimum fluidizing velocity for air and for water. Assume ε = 0.3. In the case of the water we must rederive Eq. 11.42, taking into account the buoyant force on the particles. Below are the provide answers. Please show all work to get to the correct answers.arrow_forwardPlease show all workarrow_forward2. A moving bed adsorption column needs to be designed to separate hydrophobic proteins from a fermentation broth. The following adsorption equilibrium data was observed in preliminary isotherm studies. The resin used was activated carbon with a porosity of 0.2. The overall mass transfer coefficient was determined to be 10 h¹, and the ratio of volumetric flow rate of broth to resin is 10. Determine the diameter of the column if the column height is limited to 2.5 m (indoor operation) with a flow rate of 20 m³/h, influent concentration of 7 g/L, and effluent concentration of 0.1 g/L. qi (mg/kg) Ci (g/L) 0.1 4.7 7.5 0.25 10.6 0.5 15.0 1.0 23.7 2.5 33.5 5.0 41.1 7.5arrow_forward
- 3. You are given a mixture of four proteins, whose properties are listed in the table below. Propose a process to purify each protein so that you end up with four solutions of pure protein. What resin would you use to bind the protein(s)? What changes to the buffer would you make to desorb the protein(s)? Contains an N-terminal His6-tag. Two 50 kDa subunits contain a non-heme Fe2+ in the active site. Protein Size (kDa) pl Specific Properties A 100 6.0 B 40 7.7 C 240 8.3 Ꭰ 225 5.5 Contains FAD redox center and an NADH binding domain. Composed of six 40-kDa subunits, each of which contains a [2Fe-2S] cluster. Composed of three subunits: 100 kDa structural subunit, 75 kDa subunit with a molybdopterin center, and 50 kDa subunit with FAD and an NADH binding domain.arrow_forwardb) Explain the key features of the Langmuir adsorption model - Drawing a diagram with empty and occupied sites. Show how new molecules would adsorb. drawing the diagram, showing free and empty sites, and their number (to use for next section) - Define the capacity and binding affinity parameters in terms of things shown on the diagram Defining the capacity and binding affinity parameters in terms of bound, free sites, and free molecules - Plot what would be a typical breakthrough curve and give an explanation approximately when breakthrough would occur plotting a typical sigmoidal breakthrough curve and saying it would certainly occur by the time capacity is used, but also could be much earlier if the affinity is lowarrow_forwardWater at 20°C flows at a steady average velocity of 5.25 m/s through a smooth pipe of diameter 5.08 cm. The flow is fully developed through the entire section of pipe. The total pipe length is 10.56 m, and there are two 90' elbows. Determine the friction coefficient and the head loss due to friction per meter length of the pipe. Control volume Prepared by Engr. Kirsten Gaarrow_forward
 Introduction to Chemical Engineering Thermodynami...Chemical EngineeringISBN:9781259696527Author:J.M. Smith Termodinamica en ingenieria quimica, Hendrick C Van Ness, Michael Abbott, Mark SwihartPublisher:McGraw-Hill Education
Introduction to Chemical Engineering Thermodynami...Chemical EngineeringISBN:9781259696527Author:J.M. Smith Termodinamica en ingenieria quimica, Hendrick C Van Ness, Michael Abbott, Mark SwihartPublisher:McGraw-Hill Education Elementary Principles of Chemical Processes, Bind...Chemical EngineeringISBN:9781118431221Author:Richard M. Felder, Ronald W. Rousseau, Lisa G. BullardPublisher:WILEY
Elementary Principles of Chemical Processes, Bind...Chemical EngineeringISBN:9781118431221Author:Richard M. Felder, Ronald W. Rousseau, Lisa G. BullardPublisher:WILEY Elements of Chemical Reaction Engineering (5th Ed...Chemical EngineeringISBN:9780133887518Author:H. Scott FoglerPublisher:Prentice Hall
Elements of Chemical Reaction Engineering (5th Ed...Chemical EngineeringISBN:9780133887518Author:H. Scott FoglerPublisher:Prentice Hall
 Industrial Plastics: Theory and ApplicationsChemical EngineeringISBN:9781285061238Author:Lokensgard, ErikPublisher:Delmar Cengage Learning
Industrial Plastics: Theory and ApplicationsChemical EngineeringISBN:9781285061238Author:Lokensgard, ErikPublisher:Delmar Cengage Learning Unit Operations of Chemical EngineeringChemical EngineeringISBN:9780072848236Author:Warren McCabe, Julian C. Smith, Peter HarriottPublisher:McGraw-Hill Companies, The
Unit Operations of Chemical EngineeringChemical EngineeringISBN:9780072848236Author:Warren McCabe, Julian C. Smith, Peter HarriottPublisher:McGraw-Hill Companies, The





