EBK ALGEBRA 2
11th Edition
ISBN: 9780544714304
Author: Larson
Publisher: HMH PUB
expand_more
expand_more
format_list_bulleted
Expert Solution & Answer
Chapter 8.6, Problem 53PS
Solution
(a)
To explain : In the given case, when the intersection is not a
The point at which the upper cone ends and the lower cone starts will not be a circle.
Given information :
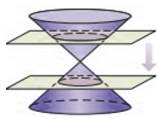
A plane perpendicular to the cone's axis passes through the cone, intersecting it in a circle whose radius decreases and then increases.
Explanation :
When a plane perpendicular to the cone's axis passes through the double napped cone and intersects the cone, it forms a
When the plane is moved from up to down then the point at which the upper cone ends and the lower cone starts, the radius will be zero, and therefore that point will not be a circle.
(b)
To explain : In the given case, when the intersection is not a hyperbola.
The point at which the two lines intersect is not a hyperbola.
Given information :
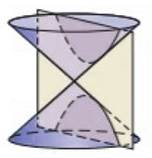
A plane parallel to the cone's axis passes through the cone, intersecting it in a hyperbola whose vertices get closer together and then farther apart.
Explanation :
When a plane parallel to the cone's axis passes through the double napped cone and intersects the cone, it forms a conic section called hyperbola.
When the plane passes through the axis of the double-napped cone, the point at which the two lines intersect is not a hyperbola.
Therefore the point at which the two lines intersect is not a hyperbola.
(c)
To explain : In the given case, when the intersection is not a parabola.
The point at which the plane gives a line is not a parabola.
Given information :
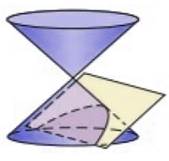
a plane parallel to the cone's nappe passes through the cone, intersecting it in a parabola that first gets narrower, then flips and gets wider.
Explanation :
When a plane parallel to the cone's nappe passes through the cone and intersects the cone, it forms a conic section called parabola.
When the plane moves from down to up of the double-napped cone, at the line of napping of the cones then the plane gives a line and this point is not a parabola.
Therefore the point at which the plane gives a line is not a parabola.
Chapter 8 Solutions
EBK ALGEBRA 2
Ch. 8.1 - Prob. 1GPCh. 8.1 - Prob. 2GPCh. 8.1 - Prob. 3GPCh. 8.1 - Prob. 4GPCh. 8.1 - Prob. 5GPCh. 8.1 - Prob. 6GPCh. 8.1 - Prob. 1ECh. 8.1 - Prob. 2ECh. 8.1 - Prob. 3ECh. 8.1 - Prob. 4E
Ch. 8.1 - Prob. 5ECh. 8.1 - Prob. 6ECh. 8.1 - Prob. 7ECh. 8.1 - Prob. 8ECh. 8.1 - Prob. 9ECh. 8.1 - Prob. 10ECh. 8.1 - Prob. 11ECh. 8.1 - Prob. 12ECh. 8.1 - Prob. 13ECh. 8.1 - Prob. 14ECh. 8.1 - Prob. 15ECh. 8.1 - Prob. 16ECh. 8.1 - Prob. 17ECh. 8.1 - Prob. 18ECh. 8.1 - Prob. 19ECh. 8.1 - Prob. 20ECh. 8.1 - Prob. 21ECh. 8.1 - Prob. 22ECh. 8.1 - Prob. 23ECh. 8.1 - Prob. 24ECh. 8.1 - Prob. 25ECh. 8.1 - Prob. 26ECh. 8.1 - Prob. 27ECh. 8.1 - Prob. 28ECh. 8.1 - Prob. 29ECh. 8.1 - Prob. 30ECh. 8.1 - Prob. 31ECh. 8.1 - Prob. 32ECh. 8.1 - Prob. 33ECh. 8.1 - Prob. 34ECh. 8.1 - Prob. 35ECh. 8.1 - Prob. 36ECh. 8.1 - Prob. 37ECh. 8.1 - Prob. 38ECh. 8.1 - Prob. 39ECh. 8.1 - Prob. 40ECh. 8.1 - Prob. 41ECh. 8.1 - Prob. 42ECh. 8.1 - Prob. 43ECh. 8.1 - Prob. 44ECh. 8.1 - Prob. 45ECh. 8.1 - Prob. 46ECh. 8.1 - Prob. 47PSCh. 8.1 - Prob. 48PSCh. 8.1 - Prob. 49PSCh. 8.1 - Prob. 50PSCh. 8.1 - Prob. 51PSCh. 8.1 - Prob. 52PSCh. 8.1 - Prob. 53PSCh. 8.1 - Prob. 54PSCh. 8.1 - Prob. 55PSCh. 8.1 - Prob. 56PSCh. 8.1 - Prob. 57PSCh. 8.2 - Prob. 1GPCh. 8.2 - Prob. 2GPCh. 8.2 - Prob. 3GPCh. 8.2 - Prob. 4GPCh. 8.2 - Prob. 5GPCh. 8.2 - Prob. 6GPCh. 8.2 - Prob. 7GPCh. 8.2 - Prob. 8GPCh. 8.2 - Prob. 9GPCh. 8.2 - Prob. 1ECh. 8.2 - Prob. 2ECh. 8.2 - Prob. 3ECh. 8.2 - Prob. 4ECh. 8.2 - Prob. 5ECh. 8.2 - Prob. 6ECh. 8.2 - Prob. 7ECh. 8.2 - Prob. 8ECh. 8.2 - Prob. 9ECh. 8.2 - Prob. 10ECh. 8.2 - Prob. 11ECh. 8.2 - Prob. 12ECh. 8.2 - Prob. 13ECh. 8.2 - Prob. 14ECh. 8.2 - Prob. 15ECh. 8.2 - Prob. 16ECh. 8.2 - Prob. 17ECh. 8.2 - Prob. 18ECh. 8.2 - Prob. 19ECh. 8.2 - Prob. 20ECh. 8.2 - Prob. 21ECh. 8.2 - Prob. 22ECh. 8.2 - Prob. 23ECh. 8.2 - Prob. 24ECh. 8.2 - Prob. 25ECh. 8.2 - Prob. 26ECh. 8.2 - Prob. 27ECh. 8.2 - Prob. 28ECh. 8.2 - Prob. 29ECh. 8.2 - Prob. 30ECh. 8.2 - Prob. 31ECh. 8.2 - Prob. 32ECh. 8.2 - Prob. 33ECh. 8.2 - Prob. 34ECh. 8.2 - Prob. 35ECh. 8.2 - Prob. 36ECh. 8.2 - Prob. 37ECh. 8.2 - Prob. 38ECh. 8.2 - Prob. 39ECh. 8.2 - Prob. 40ECh. 8.2 - Prob. 41ECh. 8.2 - Prob. 42ECh. 8.2 - Prob. 43ECh. 8.2 - Prob. 44ECh. 8.2 - Prob. 45ECh. 8.2 - Prob. 46ECh. 8.2 - Prob. 47ECh. 8.2 - Prob. 48ECh. 8.2 - Prob. 49ECh. 8.2 - Prob. 50ECh. 8.2 - Prob. 51ECh. 8.2 - Prob. 52ECh. 8.2 - Prob. 53ECh. 8.2 - Prob. 54ECh. 8.2 - Prob. 55PSCh. 8.2 - Prob. 56PSCh. 8.2 - Prob. 57PSCh. 8.2 - Prob. 58PSCh. 8.2 - Prob. 59PSCh. 8.2 - Prob. 60PSCh. 8.3 - Prob. 1GPCh. 8.3 - Prob. 2GPCh. 8.3 - Prob. 3GPCh. 8.3 - Prob. 4GPCh. 8.3 - Prob. 5GPCh. 8.3 - Prob. 6GPCh. 8.3 - Prob. 1ECh. 8.3 - Prob. 2ECh. 8.3 - Prob. 3ECh. 8.3 - Prob. 4ECh. 8.3 - Prob. 5ECh. 8.3 - Prob. 6ECh. 8.3 - Prob. 7ECh. 8.3 - Prob. 8ECh. 8.3 - Prob. 9ECh. 8.3 - Prob. 10ECh. 8.3 - Prob. 11ECh. 8.3 - Prob. 12ECh. 8.3 - Prob. 13ECh. 8.3 - Prob. 14ECh. 8.3 - Prob. 15ECh. 8.3 - Prob. 16ECh. 8.3 - Prob. 17ECh. 8.3 - Prob. 18ECh. 8.3 - Prob. 19ECh. 8.3 - Prob. 20ECh. 8.3 - Prob. 21ECh. 8.3 - Prob. 22ECh. 8.3 - Prob. 23ECh. 8.3 - Prob. 24ECh. 8.3 - Prob. 25ECh. 8.3 - Prob. 26ECh. 8.3 - Prob. 27ECh. 8.3 - Prob. 28ECh. 8.3 - Prob. 29ECh. 8.3 - Prob. 30ECh. 8.3 - Prob. 31ECh. 8.3 - Prob. 32ECh. 8.3 - Prob. 33ECh. 8.3 - Prob. 34ECh. 8.3 - Prob. 35ECh. 8.3 - Prob. 36ECh. 8.3 - Prob. 37ECh. 8.3 - Prob. 38ECh. 8.3 - Prob. 39ECh. 8.3 - Prob. 40ECh. 8.3 - Prob. 41ECh. 8.3 - Prob. 42ECh. 8.3 - Prob. 43ECh. 8.3 - Prob. 44ECh. 8.3 - Prob. 45ECh. 8.3 - Prob. 46ECh. 8.3 - Prob. 47ECh. 8.3 - Prob. 48ECh. 8.3 - Prob. 49ECh. 8.3 - Prob. 50ECh. 8.3 - Prob. 51ECh. 8.3 - Prob. 52ECh. 8.3 - Prob. 53ECh. 8.3 - Prob. 54ECh. 8.3 - Prob. 55ECh. 8.3 - Prob. 56ECh. 8.3 - Prob. 57ECh. 8.3 - Prob. 58ECh. 8.3 - Prob. 59ECh. 8.3 - Prob. 60ECh. 8.3 - Prob. 61ECh. 8.3 - Prob. 62PSCh. 8.3 - Prob. 63PSCh. 8.3 - Prob. 64PSCh. 8.3 - Prob. 65PSCh. 8.3 - Prob. 66PSCh. 8.3 - Prob. 67PSCh. 8.3 - Prob. 68PSCh. 8.3 - Prob. 69PSCh. 8.3 - Prob. 1QCh. 8.3 - Prob. 2QCh. 8.3 - Prob. 3QCh. 8.3 - Prob. 4QCh. 8.3 - Prob. 5QCh. 8.3 - Prob. 6QCh. 8.3 - Prob. 7QCh. 8.3 - Prob. 8QCh. 8.3 - Prob. 9QCh. 8.3 - Prob. 10QCh. 8.3 - Prob. 11QCh. 8.3 - Prob. 12QCh. 8.3 - Prob. 13QCh. 8.3 - Prob. 14QCh. 8.3 - Prob. 15QCh. 8.3 - Prob. 16QCh. 8.3 - Prob. 17QCh. 8.3 - Prob. 18QCh. 8.3 - Prob. 19QCh. 8.3 - Prob. 1PCh. 8.3 - Prob. 2PCh. 8.3 - Prob. 3PCh. 8.3 - Prob. 4PCh. 8.3 - Prob. 5PCh. 8.3 - Prob. 6PCh. 8.4 - Prob. 1GPCh. 8.4 - Prob. 2GPCh. 8.4 - Prob. 3GPCh. 8.4 - Prob. 4GPCh. 8.4 - Prob. 5GPCh. 8.4 - Prob. 6GPCh. 8.4 - Prob. 7GPCh. 8.4 - Prob. 8GPCh. 8.4 - Prob. 1ECh. 8.4 - Prob. 2ECh. 8.4 - Prob. 3ECh. 8.4 - Prob. 4ECh. 8.4 - Prob. 5ECh. 8.4 - Prob. 6ECh. 8.4 - Prob. 7ECh. 8.4 - Prob. 8ECh. 8.4 - Prob. 9ECh. 8.4 - Prob. 10ECh. 8.4 - Prob. 11ECh. 8.4 - Prob. 12ECh. 8.4 - Prob. 13ECh. 8.4 - Prob. 14ECh. 8.4 - Prob. 15ECh. 8.4 - Prob. 16ECh. 8.4 - Prob. 17ECh. 8.4 - Prob. 18ECh. 8.4 - Prob. 19ECh. 8.4 - Prob. 20ECh. 8.4 - Prob. 21ECh. 8.4 - Prob. 22ECh. 8.4 - Prob. 23ECh. 8.4 - Prob. 24ECh. 8.4 - Prob. 25ECh. 8.4 - Prob. 26ECh. 8.4 - Prob. 27ECh. 8.4 - Prob. 28ECh. 8.4 - Prob. 29ECh. 8.4 - Prob. 30ECh. 8.4 - Prob. 31ECh. 8.4 - Prob. 32ECh. 8.4 - Prob. 33ECh. 8.4 - Prob. 34ECh. 8.4 - Prob. 35ECh. 8.4 - Prob. 36ECh. 8.4 - Prob. 37ECh. 8.4 - Prob. 38ECh. 8.4 - Prob. 39ECh. 8.4 - Prob. 40ECh. 8.4 - Prob. 41ECh. 8.4 - Prob. 42ECh. 8.4 - Prob. 43ECh. 8.4 - Prob. 44ECh. 8.4 - Prob. 45ECh. 8.4 - Prob. 46ECh. 8.4 - Prob. 47ECh. 8.4 - Prob. 48PSCh. 8.4 - Prob. 49PSCh. 8.4 - Prob. 50PSCh. 8.4 - Prob. 51PSCh. 8.4 - Prob. 52PSCh. 8.4 - Prob. 53PSCh. 8.4 - Prob. 1PCh. 8.4 - Prob. 2PCh. 8.4 - Prob. 3PCh. 8.4 - Prob. 1MRPSCh. 8.4 - Prob. 2MRPSCh. 8.4 - Prob. 3MRPSCh. 8.4 - Prob. 4MRPSCh. 8.4 - Prob. 5MRPSCh. 8.4 - Prob. 6MRPSCh. 8.4 - Prob. 7MRPSCh. 8.4 - Prob. 8MRPSCh. 8.4 - Prob. 9MRPSCh. 8.5 - Prob. 1GPCh. 8.5 - Prob. 2GPCh. 8.5 - Prob. 3GPCh. 8.5 - Prob. 4GPCh. 8.5 - Prob. 5GPCh. 8.5 - Prob. 6GPCh. 8.5 - Prob. 1ECh. 8.5 - Prob. 2ECh. 8.5 - Prob. 3ECh. 8.5 - Prob. 4ECh. 8.5 - Prob. 5ECh. 8.5 - Prob. 6ECh. 8.5 - Prob. 7ECh. 8.5 - Prob. 8ECh. 8.5 - Prob. 9ECh. 8.5 - Prob. 10ECh. 8.5 - Prob. 11ECh. 8.5 - Prob. 12ECh. 8.5 - Prob. 13ECh. 8.5 - Prob. 14ECh. 8.5 - Prob. 15ECh. 8.5 - Prob. 16ECh. 8.5 - Prob. 17ECh. 8.5 - Prob. 18ECh. 8.5 - Prob. 19ECh. 8.5 - Prob. 20ECh. 8.5 - Prob. 21ECh. 8.5 - Prob. 22ECh. 8.5 - Prob. 23ECh. 8.5 - Prob. 24ECh. 8.5 - Prob. 25ECh. 8.5 - Prob. 26ECh. 8.5 - Prob. 27ECh. 8.5 - Prob. 28ECh. 8.5 - Prob. 29ECh. 8.5 - Prob. 30ECh. 8.5 - Prob. 31ECh. 8.5 - Prob. 32ECh. 8.5 - Prob. 33ECh. 8.5 - Prob. 34ECh. 8.5 - Prob. 35ECh. 8.5 - Prob. 36ECh. 8.5 - Prob. 37ECh. 8.5 - Prob. 38PSCh. 8.5 - Prob. 39PSCh. 8.5 - Prob. 40PSCh. 8.5 - Prob. 41PSCh. 8.5 - Prob. 42PSCh. 8.5 - Prob. 43PSCh. 8.5 - Prob. 44PSCh. 8.5 - Prob. 1QCh. 8.5 - Prob. 2QCh. 8.5 - Prob. 3QCh. 8.5 - Prob. 4QCh. 8.5 - Prob. 5QCh. 8.5 - Prob. 6QCh. 8.5 - Prob. 7QCh. 8.5 - Prob. 8QCh. 8.5 - Prob. 9QCh. 8.5 - Prob. 10QCh. 8.5 - Prob. 11QCh. 8.5 - Prob. 12QCh. 8.5 - Prob. 13QCh. 8.6 - Prob. 1GPCh. 8.6 - Prob. 2GPCh. 8.6 - Prob. 3GPCh. 8.6 - Prob. 4GPCh. 8.6 - Prob. 5GPCh. 8.6 - Prob. 6GPCh. 8.6 - Prob. 7GPCh. 8.6 - Prob. 8GPCh. 8.6 - Prob. 9GPCh. 8.6 - Prob. 10GPCh. 8.6 - Prob. 11GPCh. 8.6 - Prob. 12GPCh. 8.6 - Prob. 13GPCh. 8.6 - Prob. 14GPCh. 8.6 - Prob. 1ECh. 8.6 - Prob. 2ECh. 8.6 - Prob. 3ECh. 8.6 - Prob. 4ECh. 8.6 - Prob. 5ECh. 8.6 - Prob. 6ECh. 8.6 - Prob. 7ECh. 8.6 - Prob. 8ECh. 8.6 - Prob. 9ECh. 8.6 - Prob. 10ECh. 8.6 - Prob. 11ECh. 8.6 - Prob. 12ECh. 8.6 - Prob. 13ECh. 8.6 - Prob. 14ECh. 8.6 - Prob. 15ECh. 8.6 - Prob. 16ECh. 8.6 - Prob. 17ECh. 8.6 - Prob. 18ECh. 8.6 - Prob. 19ECh. 8.6 - Prob. 20ECh. 8.6 - Prob. 21ECh. 8.6 - Prob. 22ECh. 8.6 - Prob. 23ECh. 8.6 - Prob. 24ECh. 8.6 - Prob. 25ECh. 8.6 - Prob. 26ECh. 8.6 - Prob. 27ECh. 8.6 - Prob. 28ECh. 8.6 - Prob. 29ECh. 8.6 - Prob. 30ECh. 8.6 - Prob. 31ECh. 8.6 - Prob. 32ECh. 8.6 - Prob. 33ECh. 8.6 - Prob. 34ECh. 8.6 - Prob. 35ECh. 8.6 - Prob. 36ECh. 8.6 - Prob. 37ECh. 8.6 - Prob. 38ECh. 8.6 - Prob. 39ECh. 8.6 - Prob. 40ECh. 8.6 - Prob. 41ECh. 8.6 - Prob. 42ECh. 8.6 - Prob. 43ECh. 8.6 - Prob. 44ECh. 8.6 - Prob. 45ECh. 8.6 - Prob. 46ECh. 8.6 - Prob. 47ECh. 8.6 - Prob. 48PSCh. 8.6 - Prob. 49PSCh. 8.6 - Prob. 50PSCh. 8.6 - Prob. 51PSCh. 8.6 - Prob. 52PSCh. 8.6 - Prob. 53PSCh. 8.7 - Prob. 1GPCh. 8.7 - Prob. 2GPCh. 8.7 - Prob. 3GPCh. 8.7 - Prob. 4GPCh. 8.7 - Prob. 5GPCh. 8.7 - Prob. 6GPCh. 8.7 - Prob. 7GPCh. 8.7 - Prob. 8GPCh. 8.7 - Prob. 9GPCh. 8.7 - Prob. 10GPCh. 8.7 - Prob. 1ECh. 8.7 - Prob. 2ECh. 8.7 - Prob. 3ECh. 8.7 - Prob. 4ECh. 8.7 - Prob. 5ECh. 8.7 - Prob. 6ECh. 8.7 - Prob. 7ECh. 8.7 - Prob. 8ECh. 8.7 - Prob. 9ECh. 8.7 - Prob. 10ECh. 8.7 - Prob. 11ECh. 8.7 - Prob. 12ECh. 8.7 - Prob. 13ECh. 8.7 - Prob. 14ECh. 8.7 - Prob. 15ECh. 8.7 - Prob. 16ECh. 8.7 - Prob. 17ECh. 8.7 - Prob. 18ECh. 8.7 - Prob. 19ECh. 8.7 - Prob. 20ECh. 8.7 - Prob. 21ECh. 8.7 - Prob. 22ECh. 8.7 - Prob. 23ECh. 8.7 - Prob. 24ECh. 8.7 - Prob. 25ECh. 8.7 - Prob. 26ECh. 8.7 - Prob. 27ECh. 8.7 - Prob. 28ECh. 8.7 - Prob. 29ECh. 8.7 - Prob. 30ECh. 8.7 - Prob. 31ECh. 8.7 - Prob. 32ECh. 8.7 - Prob. 33ECh. 8.7 - Prob. 34ECh. 8.7 - Prob. 35ECh. 8.7 - Prob. 36ECh. 8.7 - Prob. 37ECh. 8.7 - Prob. 38ECh. 8.7 - Prob. 39PSCh. 8.7 - Prob. 40PSCh. 8.7 - Prob. 41PSCh. 8.7 - Prob. 42PSCh. 8.7 - Prob. 43PSCh. 8.7 - Prob. 44PSCh. 8.7 - Prob. 45PSCh. 8.7 - Prob. 1QCh. 8.7 - Prob. 2QCh. 8.7 - Prob. 3QCh. 8.7 - Prob. 4QCh. 8.7 - Prob. 5QCh. 8.7 - Prob. 6QCh. 8.7 - Prob. 7QCh. 8.7 - Prob. 8QCh. 8.7 - Prob. 9QCh. 8.7 - Prob. 10QCh. 8.7 - Prob. 11QCh. 8.7 - Prob. 12QCh. 8.7 - Prob. 13QCh. 8.7 - Prob. 14QCh. 8.7 - Prob. 1PCh. 8.7 - Prob. 2PCh. 8.7 - Prob. 3PCh. 8.7 - Prob. 4PCh. 8.7 - Prob. 5PCh. 8.7 - Prob. 6PCh. 8.7 - Prob. 7PCh. 8.7 - Prob. 8PCh. 8.7 - Prob. 9PCh. 8.7 - Prob. 10PCh. 8.7 - Prob. 11PCh. 8.7 - Prob. 12PCh. 8.7 - Prob. 13PCh. 8.7 - Prob. 14PCh. 8.7 - Prob. 15PCh. 8.7 - Prob. 1MRPSCh. 8.7 - Prob. 2MRPSCh. 8.7 - Prob. 3MRPSCh. 8.7 - Prob. 4MRPSCh. 8.7 - Prob. 5MRPSCh. 8.7 - Prob. 6MRPSCh. 8 - Prob. 1VECh. 8 - Prob. 2VECh. 8 - Prob. 3VECh. 8 - Prob. 4VECh. 8 - Prob. 5REAECh. 8 - Prob. 6REAECh. 8 - Prob. 7REAECh. 8 - Prob. 8REAECh. 8 - Prob. 9REAECh. 8 - Prob. 10REAECh. 8 - Prob. 11REAECh. 8 - Prob. 12REAECh. 8 - Prob. 13REAECh. 8 - Prob. 14REAECh. 8 - Prob. 15REAECh. 8 - Prob. 16REAECh. 8 - Prob. 17REAECh. 8 - Prob. 18REAECh. 8 - Prob. 19REAECh. 8 - Prob. 20REAECh. 8 - Prob. 21REAECh. 8 - Prob. 22REAECh. 8 - Prob. 23REAECh. 8 - Prob. 24REAECh. 8 - Prob. 25REAECh. 8 - Prob. 26REAECh. 8 - Prob. 27REAECh. 8 - Prob. 28REAECh. 8 - Prob. 29REAECh. 8 - Prob. 30REAECh. 8 - Prob. 31REAECh. 8 - Prob. 32REAECh. 8 - Prob. 33REAECh. 8 - Prob. 34REAECh. 8 - Prob. 35REAECh. 8 - Prob. 36REAECh. 8 - Prob. 37REAECh. 8 - Prob. 1TCh. 8 - Prob. 2TCh. 8 - Prob. 3TCh. 8 - Prob. 4TCh. 8 - Prob. 5TCh. 8 - Prob. 6TCh. 8 - Prob. 7TCh. 8 - Prob. 8TCh. 8 - Prob. 9TCh. 8 - Prob. 10TCh. 8 - Prob. 11TCh. 8 - Prob. 12TCh. 8 - Prob. 13TCh. 8 - Prob. 14TCh. 8 - Prob. 15TCh. 8 - Prob. 16TCh. 8 - Prob. 17TCh. 8 - Prob. 18TCh. 8 - Prob. 19TCh. 8 - Prob. 20TCh. 8 - Prob. 21TCh. 8 - Prob. 22TCh. 8 - Prob. 23TCh. 8 - Prob. 24TCh. 8 - Prob. 25TCh. 8 - Prob. 26TCh. 8 - Prob. 27TCh. 8 - Prob. 28TCh. 8 - Prob. 29TCh. 8 - Prob. 30TCh. 8 - Prob. 31TCh. 8 - Prob. 32TCh. 8 - Prob. 33TCh. 8 - Prob. 34TCh. 8 - Prob. 1PCh. 8 - Prob. 2PCh. 8 - Prob. 1TPCh. 8 - Prob. 2TPCh. 8 - Prob. 3TPCh. 8 - Prob. 4TPCh. 8 - Prob. 5TPCh. 8 - Prob. 6TPCh. 8 - Prob. 7TPCh. 8 - Prob. 8TPCh. 8 - Prob. 9TPCh. 8 - Prob. 10TPCh. 8 - Prob. 11TPCh. 8 - Prob. 12TPCh. 8 - Prob. 13TPCh. 8 - Prob. 14TPCh. 8 - Prob. 15TPCh. 8 - Prob. 16TPCh. 8 - Prob. 17TPCh. 8 - Prob. 18TP
Knowledge Booster
Similar questions
- I do not understand what to doarrow_forwardDirections: For problem 15: Determine the leading term, leading coefficient, and constant term of each polynomialarrow_forward3. Answer the questions for the quadratic function f(x) = -2x² + 8x - 10 a. Does the parabola opens up or down? b. Find the equation of the axis of symmetry. c. Find the vertex. d. Find the x and y intercepts. e. Graph the function on the axis provided. 12 13arrow_forward
- Practice: 2. Answer the questions for the quadratic function f(x) = x² + 8x+12 a. Does the parabola opens up or down? b. Find the equation of the axis of symmetry. c. Find the vertex. d. Find the x and y intercepts. e. Graph the function on the axis provided. -10 7arrow_forwardExercise 12.2. Let F be a field and n Є N. (1) For every subset S of F", show that I(S) := {f(x1, . ,xn) Є F[x1,...,xn] | f (a₁,, an) = 0, V(a1, ..., an) E S} is an ideal of the polynomial ring F[x1, ..., xn], called the vanishing ideal of S. (2) Given two subsets S and T of F", prove or disprove that I(SUT) = I(S)NI(T) · and I(SNT) = I(S) + I(T).arrow_forwardD2L Course Schedule × ⇒ Math 140 AC2 MT × G Google ☑ Untitled documen × G Google G square root sign ✗ adidas Samba OG X Math 140 AC2 MT X → C https://drive.google.com/file/d/1pXqJ_6k8OigXg6qML2lkxIM-bDwLhk5T/view 3. We're a little past the halfway point of the semester. I've been thinking about how we've been doing. I have noticed something interesting about the relationship between WAs and grades. I made a piecewise function to describe the situation. G(x) is the grade for someone who has completed x WAs. Use the function for parts a-c. (7.5 pts.) 20 for x=0 G(x)=15x+ 30 for 0arrow_forwardFor each of the following equations, a solution is given. Show whether the given solutionis correct by substituting the variable back into the equation. -9x = 18x = -2arrow_forwardA large mixing tank currently contains 200 gallons of water, into which 10 pounds of sugar have been mixed. A tap will open, pouring 10 gallons of water per minute into the tank at the same time sugar is poured into the tank at a rate of 3 pounds per minute. The concentration function has a vertical asymptote. Write the equation for the vertical asymptote. Does the vertical asymptote have any practical application in this problem? Why or why not?arrow_forward2 סוי vle.mathswatch.co.uk Queue | Oxf... X VIEWER XD... Submit a co... 18/28 Marks mplify 2a (a + 1) + 5(3a - 2) 2a+ 3 Answer + 5 البقاله بالهجه ..... W e r t y u # S £ יו 20 d f g harrow_forwardDraw a line representing the "rise" and a line representing the "run" of the line. State the slope of the line in simplest form. Click twice to plot each segment.Click a segment to delete it. 12345678910-1-2-3-4-5-6-7-8-9-1012345678910-1-2-3-4-5-6-7-8-9-10xy12345678910-1-2-3-4-5-6-7-8-9-1012345678910-1-2-3-4-5-6-7-8-9-10xy Answer Attempt 1 out of 2 Slope of the Line:arrow_forwardD2L Course Schedule × zm MATH 140 AC O Math 140 AC2 MT X Home - Google Dix Untitled documen X APznzaZOmoEKtr X adidas Samba OG × ✓ Math 140 AC2 MT × | + C doc-08-9s-prod-00-apps-viewer.googleusercontent.com/viewer2/prod-00/pdf/4b40ij3n5ssib10rfbdvgd4hcOnkjh9f/0972m4636 vecpvn6ctclvph34kfeur7... Q Update: APznzaZOmoEKtnM4eg2YA5rwDV97bDw0GG39cSO6IEGFlaYSOS4iHO... 2 / 3 - 90% + | 2. We spent a lot of time working with linear functions and distinguishing them from nonlinear functions. For a-c, if the function is linear, find its equation. If it is not linear, explain why it's not linear. (7.5 pts.) a) The UPass is a great program for students in college. You pay $80 for unlimited ‘L' and bus rides for the entire semester. U(x) is the cost for x 'L' and bus rides in one semester. b) X g(x) -1 -1 -2 -3 2 5 0 1 1 3 c) 4. 3. 2 1- 6 -5 -4 -3 -2 -1 0 1 2 4 5 6 7 8 -1- -2arrow_forwardD2L Course Sched X | zm MATH 140 ✗ Math 140 AC2 × Untitled docun X APznzaZOmoE X adidas Samba × |_ Math 140 AC2 × Home - Google × b Home | bartleb × | + С doc-08-9s-prod-00-apps-viewer.googleusercontent.com/viewer2/prod-00/pdf/4b40ij3n5ssib10rfbdvgd4hc0nkjh9f/0972m4636 vecpvn6ctclvph34kfeur7... Q Update: III APznzaZOmoEKtnM4eg2YA5rwDV97bDw0GG39cSO6IEGFlaYSoS4iHO... MT Check In Pat 1 2 20 for x=0 G(x)=15+ 300arrow_forwardarrow_back_iosSEE MORE QUESTIONSarrow_forward_iosRecommended textbooks for you
Algebra and Trigonometry (6th Edition)AlgebraISBN:9780134463216Author:Robert F. BlitzerPublisher:PEARSON
Contemporary Abstract AlgebraAlgebraISBN:9781305657960Author:Joseph GallianPublisher:Cengage Learning
Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning
Algebra And Trigonometry (11th Edition)AlgebraISBN:9780135163078Author:Michael SullivanPublisher:PEARSON
Introduction to Linear Algebra, Fifth EditionAlgebraISBN:9780980232776Author:Gilbert StrangPublisher:Wellesley-Cambridge Press
College Algebra (Collegiate Math)AlgebraISBN:9780077836344Author:Julie Miller, Donna GerkenPublisher:McGraw-Hill Education
Algebra and Trigonometry (6th Edition)AlgebraISBN:9780134463216Author:Robert F. BlitzerPublisher:PEARSONContemporary Abstract AlgebraAlgebraISBN:9781305657960Author:Joseph GallianPublisher:Cengage LearningLinear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage LearningAlgebra And Trigonometry (11th Edition)AlgebraISBN:9780135163078Author:Michael SullivanPublisher:PEARSONIntroduction to Linear Algebra, Fifth EditionAlgebraISBN:9780980232776Author:Gilbert StrangPublisher:Wellesley-Cambridge PressCollege Algebra (Collegiate Math)AlgebraISBN:9780077836344Author:Julie Miller, Donna GerkenPublisher:McGraw-Hill Education