Chapter 8.5, Problem 45E

To simulate roulette on a graphing calculator, a random integer between $- 1$ and 36 is selected $\left(-1\text{ represents }00;\text{ see Problem }35\right).$ The command in Figure A simulates 200 games.

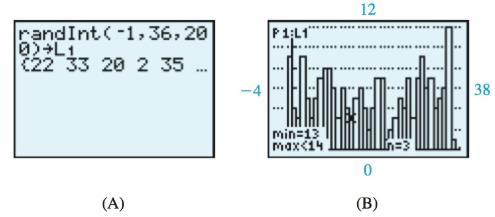
(A) Use the statistical plot in Figure B to determine the net gain or loss of placing a $\text{\$1}$ bet on the number 13 in each of the 200 games.

(B) Compare the results of part (A) with the expected value of the game.

(C) Use a graphing calculator to simulate betting $\text{\$1}$ on the number 7 in each of 500 games of roulette and compare the simulated and expected gains or losses.