
Concept explainers
(a)
Identify the positions of two particles at any instant t and explain about its motion.
(a)
Answer to Problem 8.3P
Equation to find the position of particles at any instant t are
Explanation of Solution
Write the general expression for position coordinate of centre of mass of system.
Here¸
Rearrange the above equation as follows.
Express
Here,
Rewrite equation (II) by adding
Write the equation for linear velocity of 1st mass from the above equation.
Here,
Rewrite equation (II) by adding
Draw the diagram showing the spring- mass system.
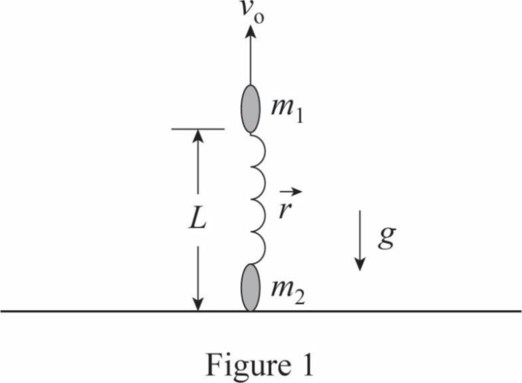
Write the law of conservation of momentum for spring-mass system.
Here,
Rewrite the expression for
Write the equation for position of initial centre of mass.
Write the equation of motion for coordinate of centre of mass.
Rewrite the above equation by substituting equations (IV) and (V).
Write the Lagrange equation in relative coordinates.
Replace
Write the Lagrange equation with respect to
Rewrite the above equation by substituting
Write the solution for above equation.
Replace
At time t=0, r=L.
Differentiate
At t=0,
Rewrite the previous equation by substituting
Rewrite the equation
Substitute
Replace
Substitute
Conclusion:
Therefore, the equation to find the position of particles at any instant t are
Want to see more full solutions like this?
Chapter 8 Solutions
Classical Mechanics
- Charge qi = -q is located at position (0, d). Charge q = −2q₁ is located at position (d,0). Charge q3 = located at position (2d, 2d). 5qi is y Determine the net electric field Ĕ net at the origin. Enter your expression using ij unit vector notation in terms of the given quantities, the permittivity of free space €0, and exact rational and irrational numbers. d 9₁ d TH net = 92 d d Xarrow_forwardsolve pleasearrow_forward= = R4 R5 = 12.5 Q. A - In the circuit shown, R₁ = R₂ = R 3 voltmeter measures the potential difference across the battery. When the switch is in position 1, the voltmeter measures V₁ = 13.8 V. When the switch is in position 2, the voltmeter measures V2 = 13.4 V. What is the emf ☐ of the battery? 14.93 = What is the battery's internal resistance r? r = V CH Ω R₁₂ V S R₁ 02 2 R₁ 4 R3 R 5arrow_forward
- Consider the arrangement of charges shown in the figure. Four charges of equal magnitude Q but varying sign are placed at the corners of a square as indicated. A positive charge q is placed in the center. What is the direction of the net force, if any, on the center charge? Indicate your answer by placing the appropriate label in the first box. Then, suppose that the charge q were to be displaced slightly from the center position. On the figure, label each box with the arrow that best indicates the direction of the net force that would act on q if it were moved to that location. Net Force Answer Bank no force ↑ +2 0 -Q -Q +Qarrow_forwardDon't use ai to answer I will report you answerarrow_forwardWhen an electromagnetic wave is reflected at normal incidence on a perfectly conducting surface, the electric fieldvector of the reflected wave at the reflecting surface is the negative of that of the incident wave.a) Explain why this should be so.b) Show that the superposition of the incident and reflected waves results in a standing wave.c) What is the relationship between the magnetic field vector of the incident and reflected waves at the reflectingsurface?arrow_forward
- Suppose there are two transformers between your house and the high-voltage transmission line that distributes the power. In addition, assume your house is the only one using electric power. At a substation the primary of a step-down transformer (turns ratio = 1:23) receives the voltage from the high-voltage transmission line. Because of your usage, a current of 51.1 mA exists in the primary of the transformer. The secondary is connected to the primary of another step- down transformer (turns ratio = 1:36) somewhere near your house, perhaps up on a telephone pole. The secondary of this transformer delivers a 240-V emf to your house. How much power is your house using? Remember that the current and voltage given in this problem are rms values.arrow_forwardIn some places, insect "zappers," with their blue lights, are a familiar sight on a summer's night. These devices use a high voltage to electrocute insects. One such device uses an ac voltage of 3970 V, which is obtained from a standard 120-V outlet by means of a transformer. If the primary coil has 27 turns, how many turns are in the secondary coil? hel lp?arrow_forwardHi, Does Quantum physics theory means all branches for example quantum relativity, Quantum mechanics, Quantum field theory, and string theory? Can you explain each one of them? Bestarrow_forward
- Dear Scientist in physics , How are doing, my name is Yahya from Saudi Arabia and currently in my first semester to pursue Master's degree in physics. I have been watching all interviews of some scientists in physics on YouTube Channel and somthing has got my mind. I studied my bachelor 's degree in biology and I have been contacting Professor's Bruce Lipton many times and he explained epigenatic well. He was talking about physics many times. He said if you want to understand who we are and how we think, you need to understand Physics well. So I have decided to study physics. I have some questions : Why is the community of physics are divided? What is the difference between Quantum physics, quantum field theory, Quantim theory, and classical physics? What is quantum consciousness theory as well. What do they mean by wave function collapse? Why professor Roger's always has another opinions in quantum consciousness theory?? Best Regards, Yahyaarrow_forwardGiven water's mass of 18g/mole and the value of the fundamental charge (charge magnitude of the electron and proton), use the largest charge density from the article to determine what fraction of water molecules became ionized (charged) due to triboelectric effects when it flows through the material that causes the largest charge transfer. Give your answer in e/molecule, or electrons transferred per molecule of water. For instance, a value of 0.2 means only one in five molecules of water loses an electron, or that 0.2=20% of water molecules become chargedarrow_forwardno AI, pleasearrow_forward
 College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning University Physics (14th Edition)PhysicsISBN:9780133969290Author:Hugh D. Young, Roger A. FreedmanPublisher:PEARSON
University Physics (14th Edition)PhysicsISBN:9780133969290Author:Hugh D. Young, Roger A. FreedmanPublisher:PEARSON Introduction To Quantum MechanicsPhysicsISBN:9781107189638Author:Griffiths, David J., Schroeter, Darrell F.Publisher:Cambridge University Press
Introduction To Quantum MechanicsPhysicsISBN:9781107189638Author:Griffiths, David J., Schroeter, Darrell F.Publisher:Cambridge University Press Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Lecture- Tutorials for Introductory AstronomyPhysicsISBN:9780321820464Author:Edward E. Prather, Tim P. Slater, Jeff P. Adams, Gina BrissendenPublisher:Addison-Wesley
Lecture- Tutorials for Introductory AstronomyPhysicsISBN:9780321820464Author:Edward E. Prather, Tim P. Slater, Jeff P. Adams, Gina BrissendenPublisher:Addison-Wesley College Physics: A Strategic Approach (4th Editio...PhysicsISBN:9780134609034Author:Randall D. Knight (Professor Emeritus), Brian Jones, Stuart FieldPublisher:PEARSON
College Physics: A Strategic Approach (4th Editio...PhysicsISBN:9780134609034Author:Randall D. Knight (Professor Emeritus), Brian Jones, Stuart FieldPublisher:PEARSON





