
Concept explainers
(a)
Decompose the Lagrange equation into equation 8.13.
(a)
Answer to Problem 8.2P
Lagrange equation is decomposed into equation 8.13.
Explanation of Solution
Write the general expression for position coordinate of centre of mass of system.
Here¸
Write the equation for the total mass of the system.
Draw the vector diagram showing the position vectors of individual masses and centre of mass.
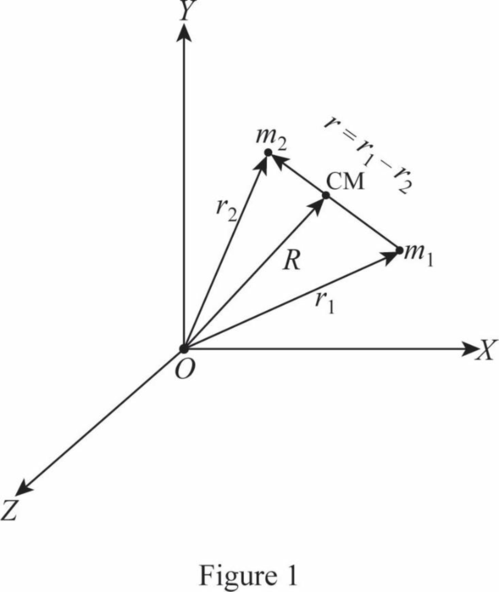
Express
Here,
Rewrite equation (II) by adding
Write the equation for linear velocity of 1st mass from the above equation.
Here,
Rewrite equation (II) by adding
Write the equation for linear velocity of 2nd mass from the above equation.
Here,
Write the expression for total kinetic energy.
Rewrite the above equation by substituting equations (III) and (IV).
Replace
Here,
Write the expression for total potential energy. It is the sum of interaction potential energy due to two masses and the gravitational potential energy.
Here,
Write the equation for
Rewrite the equation for
Write the general form of LaGrange of a system.
Rewrite the above equation by substituting equations (V) and (VI).
Express
Express
Here, x, y, z are relative coordinates.
Rewrite the equation for
Split the Lagrange into two parts-Lagrange of mass
Write the Lagrange of mass
Here,
Write the Lagrange of mass
Here,
So, the total Lagrange can be expressed as follows.
Conclusion:
Therefore, the Lagrange equation is decomposed into equation 8.13.
(b)
Write the Lagrange equation in cartesian coordinates, three Lagrange equation for relative coordinates and prove that the motion is equivalent to that of a single particle of mass equal to that of reduced mass of the system.
(b)
Answer to Problem 8.2P
Lagrange equation in cartesian coordinates are
Explanation of Solution
Write the Lagrange of mass
Write the Lagrange equation in x-direction of centre of mass.
Rewrite the above equation by substituting the previous equation.
Write the Lagrange equation in y-direction of centre of mass.
Rewrite the above equation by substituting
Write the Lagrange equation in z-direction of centre of mass.
Rewrite the above equation by substituting
Above results show that the system is moving at constant speed in x and y direction and is moving downwards with gravitational acceleration.
Write the Lagrange equation in relative coordinate x.
Rewrite the above equation by substituting
Write the Lagrange equation in relative coordinate y.
Rewrite the above equation by substituting
Write the Lagrange equation in relative coordinate z.
Rewrite the above equation by substituting
Conclusion:
Therefore, the Lagrange equation in cartesian coordinates are
Want to see more full solutions like this?
Chapter 8 Solutions
Classical Mechanics
- Suppose you have a diverging lens with a focal length of - 25 cm. You look through this lens at a sleeping squirrel, and notice that it forms a virtual image of the squirrel with di = - 18 cm. How far away from the lens is the squirrel? Give your answer as the number of centimeters (a positive number).arrow_forwardDiamond has an index of refraction of about 2.4. Suppose you cut a diamond so it has a flat surface, and shine a laser pointer beam so that it makes a 27 degree angle with respect to the normal line to that surface. What angle will the laser beam make with respect to the normal after it passes through the air-diamond boundary and is inside the diamond? Give your answer as the number of degrees.arrow_forwardFind current of each line of D,E, and F. Where V1 is 9V, V2 is 7V, R1 is 989 , R2 is 2160, R3 is 4630 , R4 is 5530, R5 is 6720, and E is 16V. Please explain all steps. Thank youarrow_forward
- You are tasked with designing a parallel-plate capacitor using two square metal plates, eachwith an area of 0.5 m², separated by a 0.1 mm thick layer of air. However, to increase the capacitance,you decide to insert a dielectric material with a dielectric constant κ = 3.0 between the plates. Describewhat happens (and why) to the E field between the plates when the dielectric is added in place of theair.arrow_forwardCalculate the work required to assemble a uniform charge Q on a thin spherical shell of radiusR. Start with no charge and add infinitesimal charges dq until the total charge reaches Q, assuming thecharge is always evenly distributed over the shell’s surface. Show all steps.arrow_forwardRod AB is fixed to a smooth collar D, which slides freely along the vertical guide shown in (Figure 1). Point C is located just to the left of the concentrated load P = 70 lb. Suppose that w= 17 lb/ft. Follow the sign convention. Part A Figure 3 ft -1.5 ft √30° 1 of 1 Determine the normal force at point C. Express your answer in pounds to three significant figures. ΜΕ ΑΣΦ Η vec Nc= Submit Request Answer Part B Determine the shear force at point C. Express your answer in pounds to three significant figures. VC= ΜΕ ΑΣΦΗ vec Submit Request Answer Part C Determine the moment at point C. Express your answer in pound-feet to three significant figures. Mc= Ο ΑΣΦ Η vec Submit Request Answer Provide Feedback ? ? lb lb ? lb-ftarrow_forward
- Consider a uniformly charged ring of radius R with total charge Q, centered at the origin inthe xy-plane. Find the electric field (as a vector) at a point on the z-axis at a distance z above thecenter of the ring. Assume the charge density is constant along the ring.arrow_forward3) If the slider block C is moving at 3m/s, determine the angular velocity of BC and the crank AB at the instant shown. (Use equation Vs Vc wx fuc, then use equation Vs VA + Ve/athen write it in terms of w and the appropriate r equate the two and solve) 0.5 m B 1 m 60° A 45° vc = 3 m/sarrow_forward3) If the slider block C is moving at 3m/s, determine the angular velocity of BC and the crank AB at the instant shown. (Use equation Vs Vc wxf, then use equation V, VA + Va/Athen write it in terms of w and the appropriate r equate the two and solve) f-3marrow_forward
- Pls help ASAParrow_forwardPls help ASAParrow_forward14. A boy is out walking his dog. From his house, he walks 30 m North, then 23 m East, then 120 cm South, then 95 m West, and finally 10 m East. Draw a diagram showing the path that the boy walked, his total displacement, and then determine the magnitude and direction of his total displacement.arrow_forward
 College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning University Physics (14th Edition)PhysicsISBN:9780133969290Author:Hugh D. Young, Roger A. FreedmanPublisher:PEARSON
University Physics (14th Edition)PhysicsISBN:9780133969290Author:Hugh D. Young, Roger A. FreedmanPublisher:PEARSON Introduction To Quantum MechanicsPhysicsISBN:9781107189638Author:Griffiths, David J., Schroeter, Darrell F.Publisher:Cambridge University Press
Introduction To Quantum MechanicsPhysicsISBN:9781107189638Author:Griffiths, David J., Schroeter, Darrell F.Publisher:Cambridge University Press Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Lecture- Tutorials for Introductory AstronomyPhysicsISBN:9780321820464Author:Edward E. Prather, Tim P. Slater, Jeff P. Adams, Gina BrissendenPublisher:Addison-Wesley
Lecture- Tutorials for Introductory AstronomyPhysicsISBN:9780321820464Author:Edward E. Prather, Tim P. Slater, Jeff P. Adams, Gina BrissendenPublisher:Addison-Wesley College Physics: A Strategic Approach (4th Editio...PhysicsISBN:9780134609034Author:Randall D. Knight (Professor Emeritus), Brian Jones, Stuart FieldPublisher:PEARSON
College Physics: A Strategic Approach (4th Editio...PhysicsISBN:9780134609034Author:Randall D. Knight (Professor Emeritus), Brian Jones, Stuart FieldPublisher:PEARSON





