
Electronics Fundamentals: Circuits, Devices & Applications
8th Edition
ISBN: 9780135072950
Author: Thomas L. Floyd, David Buchla
Publisher: Prentice Hall
expand_more
expand_more
format_list_bulleted
Concept explainers
Textbook Question
Chapter 8, Problem 41P
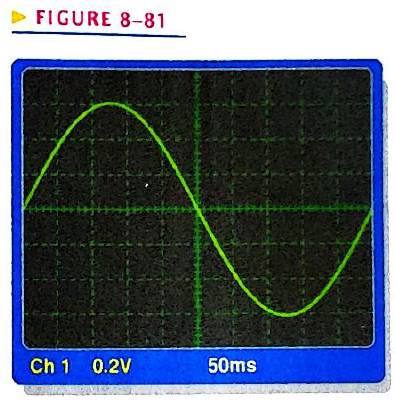
Determine the rms value and the frequency of the sine wave displayed on the scope screen in Figure 8-81.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
Please help me
Please help me
Can you check my connections and answers.
Chapter 8 Solutions
Electronics Fundamentals: Circuits, Devices & Applications
Ch. 8 - The period of a 60 Hz sine wave is 16.7 ms.Ch. 8 - The rms and average value of a sine wave are the...Ch. 8 - Prob. 3TFQCh. 8 - The peak value of a sine wave is the same as its...Ch. 8 - The number of radians in 360 is 2 .Ch. 8 - In a three-phase electrical system, the phases are...Ch. 8 - The purpose of an exciter is to supply dc rotor...Ch. 8 - In an automotive alternator, the output current is...Ch. 8 - Prob. 9TFQCh. 8 - A synchronous motor can be used when constant...
Ch. 8 - Prob. 1STCh. 8 - During each cycle, a sine wave reaches a peak...Ch. 8 - A sine wave with a frequency of 12 kHz is changing...Ch. 8 - Prob. 4STCh. 8 - When a sine wave has a frequency of 60 Hz, in 10 s...Ch. 8 - Prob. 6STCh. 8 - Prob. 7STCh. 8 - The average value of a 10 V peak sine wave over...Ch. 8 - Prob. 9STCh. 8 - Prob. 10STCh. 8 - The instantaneous value of a 15 A peak sine wave...Ch. 8 - If the rms curren through a 10k resistor is 5 m A,...Ch. 8 - Prob. 13STCh. 8 - Prob. 14STCh. 8 - Prob. 15STCh. 8 - Prob. 16STCh. 8 - Prob. 17STCh. 8 - Prob. 1TSCCh. 8 - Prob. 2TSCCh. 8 - Prob. 3TSCCh. 8 - Prob. 4TSCCh. 8 - Prob. 5TSCCh. 8 - Calculate the frequency for each of the following...Ch. 8 - Calculate the period for each of the following...Ch. 8 - A sine wave goes through 5 cycles in 10s. What is...Ch. 8 - A sine wave has a frequency of 50 kHz. How many...Ch. 8 - How long does it take a 10 kHz sine wave to...Ch. 8 - A sine wave has a peak value of 12 V. Determine...Ch. 8 - A sinusoidal current has an rms value of 5 mA....Ch. 8 - For the sine wave in Figure 8-74, determine the...Ch. 8 - If each horizontal division in Figure 8-74 is 1...Ch. 8 - In Figure 8-74, what is the instantaneous voltage...Ch. 8 - Sine wave A has a positive-going zero crossing at...Ch. 8 - One sine wave has a positive peak at 75 and...Ch. 8 - Draw two since waves as follows: Sline wave A is...Ch. 8 - Convert the following angular values from degrees...Ch. 8 - Convert the following angular values from radians...Ch. 8 - A certain sine wave has a positive-going zero...Ch. 8 - For a particular 0 reference sinusoidal current,...Ch. 8 - For a 0 reference sinw wave with an rms value of...Ch. 8 - Sine wave A lags sine wave B by 30. Both have peak...Ch. 8 - Repeat Problem 19 for the case when sine wave A...Ch. 8 - A sinusoidal voltage is applied to the resistive...Ch. 8 - Find the half-cycle average values of the voltages...Ch. 8 - Determine th rms voltage across R3 in Figure 8-77.Ch. 8 - A sine wave with an rms value of 10.6 V is riding...Ch. 8 - How much dc voltage must be added to a 3 V rms...Ch. 8 - A 6 V peak sine wave is riding on a dc voltage of...Ch. 8 - The conductive wire loop on the rotor of a simple...Ch. 8 - Prob. 28PCh. 8 - At what speed of rotation must a four-pole...Ch. 8 - A common frequency for alternators on aircraft is...Ch. 8 - Prob. 31PCh. 8 - Explain how the field in a three-phase motor...Ch. 8 - From the graph in Figure 8-78, determine the...Ch. 8 - Determine the duty cycle for each pulse waveform...Ch. 8 - Find the average value of each positive-going...Ch. 8 - What is the frequency of each waveform in Figure...Ch. 8 - What is the frequency of each sawtooth waveform in...Ch. 8 - A square wave has a period of 40s. List the first...Ch. 8 - What is the fundamental frequency of the square...Ch. 8 - Determine the peak value and the period of the...Ch. 8 - Determine the rms value and the frequency of the...Ch. 8 - Determine the rms value and the frequency of the...Ch. 8 - Find the amplitude, pulse width, and duty cycle...Ch. 8 - A certain sine wave has a frequency of 2.2 kHz and...Ch. 8 - Figure 8-84 shows a sinusoidal voltage source in...Ch. 8 - A nonsinusoidal waveform called a stairstep is...Ch. 8 - Refer to the oscilloscope screen in Figure 8-86....Ch. 8 - Accurately draw on a grid representing the scope...Ch. 8 - Accurately draw on a grid representing the scope...Ch. 8 - Based on the instrument settings and an...Ch. 8 - Examine the circuit board and the oscilloscope...Ch. 8 - Prob. 52PCh. 8 - www. prenhall.com/floyd. 53. Open file P08-53 and...Ch. 8 - www. prenhall.com/floyd. 54. Open file P08-54 and...Ch. 8 - www. prenhall.com/floyd. 55. Open file P08-55 and...Ch. 8 - www. prenhall.com/floyd. 56. Open file P08-56 and...
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, electrical-engineering and related others by exploring similar questions and additional content below.Similar questions
- A communication satellite is in stationary (synchronous) orbit about the earch (assume altitude of 22.300 statute miles). Its transmitter generates 8.00 W. Assume the transmit- ting antenna is isotropic. Its signal is received by the 210-ft diameter tracking parabo- loidal antenna on the earth at the NASA tracking station at Goldstone, California. Also assume no resistive loss in either antenna, perfect polarization match, and perfect impedance match at both antennas. At a frequency of 2 GHz, determine the: (a) power density (in watts/m²) incident on the receiving antenna. (b) power received by the ground-based antenna whose gain is 60 dB.arrow_forwardDetermine VO during the Negative Half Cycle of the input voltage, Vi 12 V f = 1 kHz -12 V C ... + 0.1 με Si R 56 ΚΩ Vo Vi 2 V - 0 +arrow_forward50mV and 10kHz from the function generator to the input. The mulitmeter postive is connected to the output and negative to a ground. Is the circuit connected correctly? Yes or No. Does the reading look correct? I don't need calculations but will take them. I just need to know if the connection is right. Connect a signal generator to the input and set it for 50 mV Sine wave with a frequency of 10 kHz. Connect the output to a multimeter set to RMS voltage. Record the output voltage and frequency in the following table. Repeat the measurement for all given frequency values in the table.arrow_forward
- The input reactance of an infinitesimal linear dipole of length A/60 and radius a=A/200 is given by Xin = – 120 [In(€/a) — 1] tan(ke) Assuming the wire of the dipole is copper with a conductivity of 5.7 x 10' S/m, determine at f = 1 GHz the (a) loss resistance (b) radiation resistance (c) radiation efficiency (d) VSWR when the antenna is connected to a 50-ohm linearrow_forwardExample Solve the octic polynomial 2x⁸-9x⁷+20x⁶-33x⁵+46x⁴-66x³+80x²-72x+32=0 Solution Divide by x⁴ 2x⁴-9x³+20x²-33x+46-66/x + 80/x² - 72/x³ + 32/x⁴=0 Combine and bring terms 2(x⁴+16/x⁴) - 9(x³+8/x³) +20(x²+4/x²)-33(x+2/x) + 46= 0 Let use substitution Let x+2/x =u (x+2/x)²= u² x²+2x*2/x + 4/x² = u² x²+4/x²= u²-4 (x+2/x)³= x³+8/x³+3x*2/x(x+2/x) u³= x³+8/x²+6u x³+8/x³= u³-6u (x²+4/x²)²= x⁴+2x²*4/x² + 16/x⁴ (u²-4)²= x⁴+16/x⁴ + 8 x⁴+16/x⁴ = (u²-4)²-8 x⁴+16/x⁴ = u⁴-8u²+8 2(u⁴-8u²+8)-9(u³-6u)+20(u²-4)-33u+46=0 Expand and simplify 2u⁴-9u³+4u²+21u-18=0 After checking (u-1)(u-2) Are factors Then 2u²-3u-9=0 u=3, u=-3/2 Assignment question Solve the octic polynomial 2s⁸+s⁷+2s⁶-31s⁴-16s³-32s²-160=0 using the above example question, please explain in detailarrow_forwardb) Another waveform g(t) is defined by =0 t≥0, α>0 otherwise g(t)= At exp(-at) and is plotted in Figure 1 (for representative values of 4 = 1 and α = 1). g(t) 0.4T 0.3+ 0.2 0.1+ 2 0 2 Figure 1 8 c) Show that its amplitude spectrum is |G(@)| = - A (a²+0²)² Describe briefly, with the aid of labelled sketches, how changing a affects the waveform in both the time and frequency domains. d) Deduce the Fourier transform H(@) of h(t) = g(t)+g(t+b)+g(t-b) and calculate its DC amplitude H(0).arrow_forward
- "I need an expert solution because the previous solution is incorrect." An antenna with a radiation impedance of 75+j10 ohm, with 10 ohm loss resistance, is connected to a generator with open-circuit voltage of 12 v and an internal impedance of 20 ohms via a 2/4-long transmission line with characteristic impedance of 75 ohms. (a) Draw the equivalent circuit (b) Determine the power supplied by the generator. (c) Determine the power radiated by the antenna. (d) Determine the reflection coefficient at the antenna terminals.arrow_forward--3/5- b) g(t) = 3 1441 g(t+mT) = g(t) -31 (i) Complex fourier coefficient Cn. (ii) Complex fourier coefficients - real fourier coefficient (the first 5 non-zero terms) of (iii) sketch the amplitude spectrum g(t) |Cal against n. n= -3 ⇒n=3 (labelling the axis).arrow_forwardQ4) (i) Calculate the fourier transform of : h(t) 2T (is) h(t) 2T -T о T 2T ·(-++T). cos2t ost≤T (iii) hro (4) ((-++T). cos otherwisearrow_forward
- Q2)a) consider the Circuit in figure 2 with initial conditions of Vc (o) = 5V, I₁ (o) = 1A, (i) redraw the circuit in the frequency domain using laplace Wansforms. (ii) using this circuit derive an equation for the Voltage across the inductor in the time domain.. 3.12 ww =V/3F ZH (figure 2) d) Solve the following second order differential equation using laplace transforms. d12 + 5 dx 3x=71 dt - with initial conditions x² (0) = 2, α(0) = 1arrow_forwardb) Another periodic waveform is defined by T c) g(t)= T with g(t+mT) = g(t) and m is an integer. (i) Sketch g(t) over two full cycles in the time domain, labelling the axes. (ii) Derive the formulae for the complex Fourier coefficients c₁ for g(t). For a periodic waveform h(t), if its complex Fourier coefficients are T T when n is odd T 2n²² T 4nn when n is even and not zero 4nn please derive the first five non-zero terms of the real Fourier series for h(t).arrow_forwardQ3)α) f(t) = (-+- 1 Isto f(t+mT) = f(t). L+- I Ost ST integer (i) sketch f(t) 2 full cycles time domain. (labelling the axis). (ii) Derive the formula for the real fourier Coefficients (i) Real Fourier series f(t), first 5 non-terms. an bn for f(t).arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
 Electricity for Refrigeration, Heating, and Air C...Mechanical EngineeringISBN:9781337399128Author:Russell E. SmithPublisher:Cengage Learning
Electricity for Refrigeration, Heating, and Air C...Mechanical EngineeringISBN:9781337399128Author:Russell E. SmithPublisher:Cengage Learning

Electricity for Refrigeration, Heating, and Air C...
Mechanical Engineering
ISBN:9781337399128
Author:Russell E. Smith
Publisher:Cengage Learning
What Is a Plane Wave? — Lesson 2; Author: EMViso;https://www.youtube.com/watch?v=ES2WFevGM0g;License: Standard Youtube License