Chapter 77, Problem 14A

To determine

(a)

Sketch the rectangular solid for the given views.

Explanation of Solution

The below figure represents the sketch of the sketch of a pyramid formed on surface of the extended sides of the rectangular solid surface block and identified angle.

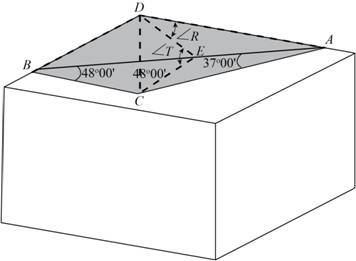
Figure-(1)

To determine

(b)

The angle of rotation $\angle R$.

Answer to Problem 14A

The angle of rotation $\angle R$ is $55°46^{\prime }$.

Explanation of Solution

The below figure represent the sketch of the sketch of a pyramid formed on surface of the extended sides of the rectangular solid surface block and identified angle.

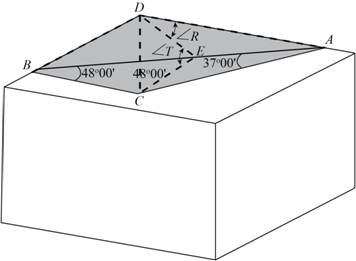
Figure-(1)

Here the side $DC$ is the common side of the triangle $\Delta ADC$, $\Delta BDC$ and $\Delta CDE$

Consider, the angle $\angle A$ of the triangle $\Delta ADC$ is $37°$, the angle $\angle B$ of the triangle $\Delta BDC$ is $48°$, the tilt angle of the triangle $\Delta CDE$ is $\angle T$ and side $DC$ is $1\text{unit}$.

Write the expression for the angle $\angle B$ using the triangle $\Delta BDC$.

  $\tan \angle B=\frac{DC}{DB}$ ...... (I)

Here, the length of the common side $DC$ is $DC$ and the length of the side $DB$ is $DB$.

Write the expression of the angle $\angle A$ using the triangle $\Delta ADC$.

  $\tan \angle A=\frac{DC}{AD}$ ........ (II)

Here, the length of the side $AD$ is $AD$.

Consider, the triangle $\Delta ADB$ is a right angle triangle.

Write the expression of the angle $\angle ADB$ of the triangle $\Delta ADB$.

  $\tan \left(\angle ADB\right)=\frac{DB}{AD}$ ......... (III)

Consider, the triangle $\Delta AED$ is a right angle triangle.

Write the expression of the rotation angle.

  $\angle R=90-\angle ADB$ ........ (IV)

Calculation:

Substitute $48°$ for $\angle B$ and $1\text{unit}$ for $DC$ in Equation (I).

  $\begin{array}{l}\tan \left(48°\right)=\frac{\left(1\text{unit}\right)}{DB}\\ DB=\frac{\left(1\text{unit}\right)}{\tan \left(48°\right)}\\ DB=0.9\text{unit}\end{array}$

Substitute $37°$ for $\angle A$ and $1\text{unit}$ for $DC$ in Equation (II).

  $\begin{array}{l}\tan \left(37°\right)=\frac{\left(1\text{unit}\right)}{AD}\\ AD=\frac{\left(1\text{unit}\right)}{\tan \left(37°\right)}\\ AD=1.32\text{unit}\end{array}$

Substitute $0.9\text{unit}$ for $DB$ and $1.32\text{unit}$ for $AD$ in Equation (III).

  $\begin{array}{l}\tan \left(\angle ADB\right)=\frac{\left(0.9\text{unit}\right)}{\left(1.32\text{unit}\right)}\\ \angle ADB={\tan }^{-1}\left(0.68\right)\\ \angle ADB=34.22°\end{array}$

Substitute $34.22°$ for $\angle ADB$ in Equation (IV).

  $\begin{array}{c}\angle R=90-34.22°\\ =55.78\\ =55°46^{\prime }\end{array}$

Conclusion:

The angle of rotation $\angle R$ is $55°46^{\prime }$.

To determine

(c)

The angle of tilt $\angle T$.

Answer to Problem 14A

The angle of tilt $\angle T$ is $52°51^{\prime }$.

Explanation of Solution

Write the expression of angle $\angle ADB$ of the triangle $\Delta DCE$.

  $\sin \left(\angle ADE\right)=\frac{DE}{AD}$ ........ (V)

Consider, the triangle $\Delta CDE$ is a right angle triangle.

Write the expression of tilt angle $\angle T$ by using triangle $\Delta CDE$.

  $\tan \angle T=\frac{DC}{DE}$ ........ (VI)

Calculation:

Substitute $34.22°$ for $\angle ADB$ and $2.25\text{unit}$ for $AD$ in Equation (V).

  $\begin{array}{l}\sin \left(34.22°\right)=\frac{DE}{\left(1.32\text{unit}\right)}\\ DE=\left(1.32\text{unit}\right)\sin \left(34.22°\right)\\ DE=0.76\text{unit}\end{array}$

Substitute $1\text{unit}$ for $DC$ and $0.76\text{unit}$ for $DE$ in Equation (VI).

  $\begin{array}{l}\tan \angle T=\frac{\left(1\text{unit}\right)}{\left(0.76\text{unit}\right)}\\ \angle T={\tan }^{-1}\left(1.32\right)\\ \angle T=52.85°\\ \angle T=52°51^{\prime }\end{array}$

Conclusion:

The angle of tilt $\angle T$ is $52°51^{\prime }$.