
Concept explainers
(a)
The number of deer after
(a)
Answer to Problem 52PPS
The number of deer after
Explanation of Solution
Given information: The number of Deer
Calculation: From (1) the number of deer after
(b)
Draw a table that shows the population of deer every year for next five years.
(b)
Answer to Problem 52PPS
| Time (t) | Number of Deer (D) |
| 0 | 100*20 = 100 |
| 1 | 100*20.5 = 141 |
| 2 | 100*21 = 200 |
| 3 | 100*21.5 = 282 |
| 4 | 100*22 = 400 |
| 5 | 100*22.5 = 565 |
Explanation of Solution
Given information: The number of Deer
Calculation: From (1) the number of deer for the next five years can be evaluated by plugging
| Time (t) | Number of Deer (D) |
| 0 | 100*20 = 100 |
| 1 | 100*20.5 = 141 |
| 2 | 100*21 = 200 |
| 3 | 100*21.5 = 282 |
| 4 | 100*22 = 400 |
| 5 | 100*22.5 = 565 |
(c)
Show the graph for number of deer verses time with the help of table obtained.
(c)
Explanation of Solution
Given information: The number of Deer
Graph: The graph for the number of deer verses the time is shown as,
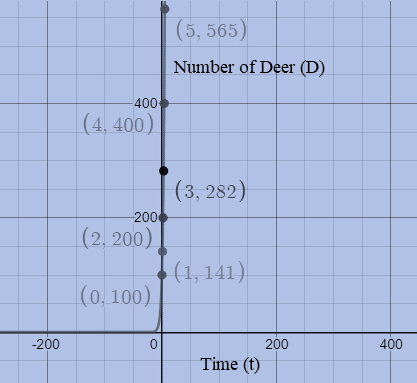
(d)
With the help of graph and table decide whether it is a reasonable trend over the long period. Explain.
(d)
Answer to Problem 52PPS
No, it is not reasonable trend over the long period.
Explanation of Solution
Given information: The number of Deer
Calculation: From the table and graph of the number of deer verses the time it can be said that the number of deer grows rapidly. And as seen from the number of deer graph it can be observed that the deer grows rapidly in small interval of time and the graph line for the population of deer is approaching to y-axis. Therefore, for the long term period it is not reasonable to say anything about the trend because there are many factors like environment conditions, temperature and rate of death etc. which can affect the deer population for long term.
Chapter 7 Solutions
Algebra 2
Additional Math Textbook Solutions
Algebra and Trigonometry (6th Edition)
Using and Understanding Mathematics: A Quantitative Reasoning Approach (6th Edition)
Thinking Mathematically (6th Edition)
Pre-Algebra Student Edition
Elementary Statistics (13th Edition)
Calculus: Early Transcendentals (2nd Edition)
- Safari File Edit View History Bookmarks Window Help Ο Ω OV O mA 0 mW ర Fri Apr 4 1 222 tv A F9 F10 DII 4 F6 F7 F8 7 29 8 00 W E R T Y U S D பட 9 O G H J K E F11 + 11 F12 O P } [arrow_forwardSo confused. Step by step instructions pleasearrow_forwardIn simplest terms, Sketch the graph of the parabola. Then, determine its equation. opens downward, vertex is (- 4, 7), passes through point (0, - 39)arrow_forward
- In simplest way, For each quadratic relation, find the zeros and the maximum or minimum. a) y = x 2 + 16 x + 39 b) y = 5 x2 - 50 x - 120arrow_forwardIn simplest terms and step by step Write each quadratic relation in standard form, then fi nd the zeros. y = - 4( x + 6)2 + 36arrow_forwardIn simplest terms and step by step For each quadratic relation, find the zeros and the maximum or minimum. 1) y = - 2 x2 - 28 x + 64 2) y = 6 x2 + 36 x - 42arrow_forward
- Write each relation in standard form a)y = 5(x + 10)2 + 7 b)y = 9(x - 8)2 - 4arrow_forwardIn simplest form and step by step Write the quadratic relation in standard form, then fi nd the zeros. y = 3(x - 1)2 - 147arrow_forwardStep by step instructions The path of a soccer ball can be modelled by the relation h = - 0.1 d 2 + 0.5 d + 0.6, where h is the ball’s height and d is the horizontal distance from the kicker. a) Find the zeros of the relation.arrow_forward
 Algebra and Trigonometry (6th Edition)AlgebraISBN:9780134463216Author:Robert F. BlitzerPublisher:PEARSON
Algebra and Trigonometry (6th Edition)AlgebraISBN:9780134463216Author:Robert F. BlitzerPublisher:PEARSON Contemporary Abstract AlgebraAlgebraISBN:9781305657960Author:Joseph GallianPublisher:Cengage Learning
Contemporary Abstract AlgebraAlgebraISBN:9781305657960Author:Joseph GallianPublisher:Cengage Learning Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning
Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning Algebra And Trigonometry (11th Edition)AlgebraISBN:9780135163078Author:Michael SullivanPublisher:PEARSON
Algebra And Trigonometry (11th Edition)AlgebraISBN:9780135163078Author:Michael SullivanPublisher:PEARSON Introduction to Linear Algebra, Fifth EditionAlgebraISBN:9780980232776Author:Gilbert StrangPublisher:Wellesley-Cambridge Press
Introduction to Linear Algebra, Fifth EditionAlgebraISBN:9780980232776Author:Gilbert StrangPublisher:Wellesley-Cambridge Press College Algebra (Collegiate Math)AlgebraISBN:9780077836344Author:Julie Miller, Donna GerkenPublisher:McGraw-Hill Education
College Algebra (Collegiate Math)AlgebraISBN:9780077836344Author:Julie Miller, Donna GerkenPublisher:McGraw-Hill Education





