
Concept explainers
Iris setosa is a beautiful wildflower that is found in such diverse places as Alaska, the Gulf of St. Lawrence, much of North America, and even in English meadows and parks. R. A. Fisher, with his colleague Dr. Edgar Anderson, studied these flowers extensively. Dr. Anderson described how he collected information on irises:
I have studied such irises as I could get to see, in as great detail as possible. measuring iris standard after iris standard and iris fall after iris fall, sitting squat-legged with record book and ruler in mountain meadows, in cypress swamps, on lake beaches, and in English parks. [E. Anderson. "The Irises of the Gaspé Peninsula." Bulletin. American IrisSociety, Vol. 59 pp. 2-5, 1935.]
The data in Table 7-10 were collected by Dr. Anderson and were published by his friend and colleague R. A. Fisher in a paper titled "The Use of Multiple Measurements in Taxonomic Problems" (Annals of Eugenics. part II. pp. 179-188, 1936). To find these data, visit the Carnegie Mellon University Data and Story Library (DASI.) web site. From the DASI. site, look under Biology and select Fisher's Irises Story.
Let x be a random variable representing petal length. Using a TI-84Plus/TI-83Plus/TI-n spire calculator, it was found that the sample
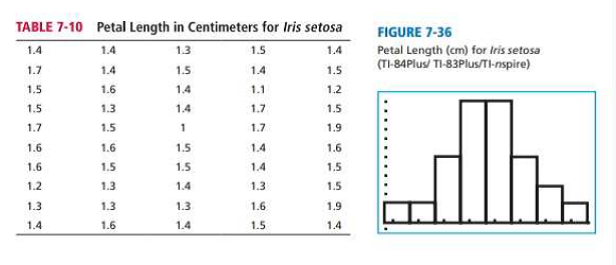
(a) Examine the histogram for petal lengths. Would you say that the distribution is approximately mound-shaped and symmetric? Our sample has only 50 irises; if many thousands of irises had been used, do you think the distribution would look even more like a normal curve? Let x be the petal length of Iris setosa. Research has shown that x has an approximately
(b) Use the
(c) Compute the
(d) Suppose that a random sample of 30 irises is obtained. Compute the probability that the average petal length for this sample is between 1.3 and 1.6 cm. Compute the probability that the average petal length is greater than 1.6 cm.
(e) Compare your answers to parts (c) and (d). Do you notice any differences? Why would these differences occur?
| TABLE 7-10 | Petal Length in Centimeters for Iris serosa | |||
| 1.4 | 14 | 1.3 | 1.5 | 1.4 |
| 1.7 | 1.4 | 1.5 | 14 | 1.5 |
| 1.5 | 1.6 | 14 | 1.1 | 1.2 |
| 1.5 | 1.3 | 1.4 | 1.7 | 1.5 |
| 1.7 | 1.5 | 1 | 1.7 | 1.9 |
| 1.6 | 16 | 1.5 | 1.4 | 16 |
| 1.5 | 1.5 | 1.4 | 1.5 | |
| 1.2 | 1.3 | 1.4 | 1.3 | 1.5 |
| 1.3 | 1.3 | 1.3 | 1.6 | 1.9 |
| 1.4 | 1.6 | 1.4 | 1.5 | 14 |
FIGURE 7-36
Petal Length (cm) for Iris setosa (TI-84Plus/TI-83Plus/TI-n spire)
(a)
To explain: Whether the distribution is approximately mound-shaped and symmetrical.
Answer to Problem DHGP
Solution: Yes, the distribution is approximately mound-shaped and symmetrical.
Explanation of Solution
Calculation:
From the histogram for petal lengths, the distribution is approximately bell-shaped or mound-shaped and symmetrical because approximately the left half of the graph being the mirror image of the right half of the graph.
Our sample has only 50 irises; if many thousands of irises had been used, the distribution would look more similar to normal curve because the sample is very largeand the distribution of the sample will be approximately normally distributed.
(b)
To find: The 68%, 95% and 99% interval and compare the computed percentages with those given by empirical rule..
Answer to Problem DHGP
Solution: The 68%, 95% and 99% interval are (1.3, 1.7), (1.1, 1.9), (0.9, 2.1) respectively.
Explanation of Solution
Let x be the petal length of Iris Setosa and x has an approximately normal distribution, with mean
We know that, 68% of the observations will fall within one standard deviation of mean.
The 68% interval is,
95% of the observations will fall within two standard deviation of mean.
The 95% interval is,
99.7% of the observations will fall within two standard deviation of mean.
The 99.7% interval is,
There are 33 data values fall within the interval 1.3 and 1.7, so the percentage of data within the interval 1.3 and 1.7 is
There are 46 data values fall within the interval 1.1 and 1.9, so the percentage of data within the interval 1.3 and 1.7 is
All data values fall within the interval 0.9 and 2.1, so the percentage of data within the interval 1.3 and 1.7 is
(c)
To find: The probability that a petal length is between 1.3 and 1.6 cm and the probability that a petal length is greater than 1.6 cm.
Answer to Problem DHGP
Solution: The probability that a petal length is between 1.3 and 1.6 cm is 0.5328. The probability that a petal length is greater than 1.6 cm is 0.3085.
Explanation of Solution
Let x be the petal length of Iris Setosa and x has an approximately normal distribution, with mean
We convert the interval
Using Table 3 from the Appendix to find the
Hence, the probability that a petal length is between 1.3 and 1.6 cm is 0.5328.
We convert the interval
Using Table 3 from the Appendix
Hence, the probability that a petal length is greater than 1.6 cm is 0.3085.
(d)
To find: The probability that average petal length is between 1.3 and 1.6 cm and the probability that average petal length is greater than 1.6 cm.
Answer to Problem DHGP
Solution: The probability that average petal length is between 1.3 and 1.6 cm is 0.9972. The probability that averagepetal length is greater than 1.6 cm is 0.0027.
Explanation of Solution
Let x has an approximately normal distribution, with mean
We convert the interval
Using Table 3 from the Appendix
Hence, the probability that average petal length is between 1.3 and 1.6 cm is 0.9972.
We convert the interval
Using Table 3 from the Appendix
Hence, the probability that a petal length is greater than 1.6 cm is 0.0027.
(e)
To explain: The comparison of part (c) and part (d).
Answer to Problem DHGP
Solution:
The standard deviation of the sample mean is much smaller than the population standard deviation.
Explanation of Solution
In part (c), x has a distribution that is approximately normal with
In part (b),
The central limit theorem tells us that the standard deviation of the sample mean is much smaller than the population standard deviation.
Want to see more full solutions like this?
Chapter 7 Solutions
Understanding Basic Statistics
- The following ordered data list shows the data speeds for cell phones used by a telephone company at an airport: A. Calculate the Measures of Central Tendency from the ungrouped data list. B. Group the data in an appropriate frequency table. C. Calculate the Measures of Central Tendency using the table in point B. 0.8 1.4 1.8 1.9 3.2 3.6 4.5 4.5 4.6 6.2 6.5 7.7 7.9 9.9 10.2 10.3 10.9 11.1 11.1 11.6 11.8 12.0 13.1 13.5 13.7 14.1 14.2 14.7 15.0 15.1 15.5 15.8 16.0 17.5 18.2 20.2 21.1 21.5 22.2 22.4 23.1 24.5 25.7 28.5 34.6 38.5 43.0 55.6 71.3 77.8arrow_forwardII Consider the following data matrix X: X1 X2 0.5 0.4 0.2 0.5 0.5 0.5 10.3 10 10.1 10.4 10.1 10.5 What will the resulting clusters be when using the k-Means method with k = 2. In your own words, explain why this result is indeed expected, i.e. why this clustering minimises the ESS map.arrow_forwardwhy the answer is 3 and 10?arrow_forward
- PS 9 Two films are shown on screen A and screen B at a cinema each evening. The numbers of people viewing the films on 12 consecutive evenings are shown in the back-to-back stem-and-leaf diagram. Screen A (12) Screen B (12) 8 037 34 7 6 4 0 534 74 1645678 92 71689 Key: 116|4 represents 61 viewers for A and 64 viewers for B A second stem-and-leaf diagram (with rows of the same width as the previous diagram) is drawn showing the total number of people viewing films at the cinema on each of these 12 evenings. Find the least and greatest possible number of rows that this second diagram could have. TIP On the evening when 30 people viewed films on screen A, there could have been as few as 37 or as many as 79 people viewing films on screen B.arrow_forwardQ.2.4 There are twelve (12) teams participating in a pub quiz. What is the probability of correctly predicting the top three teams at the end of the competition, in the correct order? Give your final answer as a fraction in its simplest form.arrow_forwardThe table below indicates the number of years of experience of a sample of employees who work on a particular production line and the corresponding number of units of a good that each employee produced last month. Years of Experience (x) Number of Goods (y) 11 63 5 57 1 48 4 54 5 45 3 51 Q.1.1 By completing the table below and then applying the relevant formulae, determine the line of best fit for this bivariate data set. Do NOT change the units for the variables. X y X2 xy Ex= Ey= EX2 EXY= Q.1.2 Estimate the number of units of the good that would have been produced last month by an employee with 8 years of experience. Q.1.3 Using your calculator, determine the coefficient of correlation for the data set. Interpret your answer. Q.1.4 Compute the coefficient of determination for the data set. Interpret your answer.arrow_forward
- Can you answer this question for mearrow_forwardTechniques QUAT6221 2025 PT B... TM Tabudi Maphoru Activities Assessments Class Progress lIE Library • Help v The table below shows the prices (R) and quantities (kg) of rice, meat and potatoes items bought during 2013 and 2014: 2013 2014 P1Qo PoQo Q1Po P1Q1 Price Ро Quantity Qo Price P1 Quantity Q1 Rice 7 80 6 70 480 560 490 420 Meat 30 50 35 60 1 750 1 500 1 800 2 100 Potatoes 3 100 3 100 300 300 300 300 TOTAL 40 230 44 230 2 530 2 360 2 590 2 820 Instructions: 1 Corall dawn to tha bottom of thir ceraan urina se se tha haca nariad in archerca antarand cubmit Q Search ENG US 口X 2025/05arrow_forwardThe table below indicates the number of years of experience of a sample of employees who work on a particular production line and the corresponding number of units of a good that each employee produced last month. Years of Experience (x) Number of Goods (y) 11 63 5 57 1 48 4 54 45 3 51 Q.1.1 By completing the table below and then applying the relevant formulae, determine the line of best fit for this bivariate data set. Do NOT change the units for the variables. X y X2 xy Ex= Ey= EX2 EXY= Q.1.2 Estimate the number of units of the good that would have been produced last month by an employee with 8 years of experience. Q.1.3 Using your calculator, determine the coefficient of correlation for the data set. Interpret your answer. Q.1.4 Compute the coefficient of determination for the data set. Interpret your answer.arrow_forward
 Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning, Functions and Change: A Modeling Approach to Coll...AlgebraISBN:9781337111348Author:Bruce Crauder, Benny Evans, Alan NoellPublisher:Cengage Learning
Functions and Change: A Modeling Approach to Coll...AlgebraISBN:9781337111348Author:Bruce Crauder, Benny Evans, Alan NoellPublisher:Cengage Learning



