
Concept explainers
(a)
The langrangian for two particles of equal masses.
(a)
Answer to Problem 7.8P
The Lagrangian of the two particle system is,
Explanation of Solution
The potential energy of the system is,
Here
Below figure shows the arrangement of the two particles of equal masses. These two particles are connected by a spring.
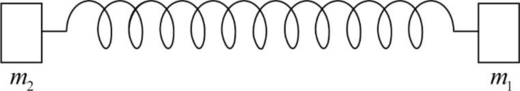
Here mass 1
The kinetic energy of the two masses is’
Here,
Substitute
The extension of the spring is,
Here,
Substitute
The Lagrangian of the system is,
Conclusion:
Substitute
Therefore, the Lagrangian of the two particle system is:
(b)
The Lagrange equations for new variable
(b)
Explanation of Solution
The position of the centre of mass of the system is,
Solve the equation for
The extension of the spring is,
Solve the equation for
Add the equations (1) and (2) to solve for
Substitute
The velocities of the first and second particle in terms of the position of the centre of mass of the system as follows.
The langrangian of two particle system is,
Substitute
Conclusion:
Therefore, the Lagrangian of the system of two particles in terms of centre of mass of the system is
The lagrangian equation of motion for the two-particle system in terms of
Determine the values of
Determine the value of
Substitute
Thus, the lagrange’s equation of motion for
The lagrangian equation motion for the two-particle system in terms of
Determine the value of
Determine the value of
Substitute
Thus, the lagrange’s equation of motion for
(c)
Solve for
(c)
Answer to Problem 7.8P
The value for
Explanation of Solution
Integrate the equation
Here,
Integrate the equation solve
Here,
Hence the value for
Conclusion:
Solve for the solution for the lagrange;s equation for
Compare the above equation of motion
So, the relative position oscillates with angular frequency
The centre of mass of the system moves as a free particle. This is because of the no external force acting on the system. Hence, two particles oscillate relative to each other.
Want to see more full solutions like this?
Chapter 7 Solutions
Classical Mechanics
- During a concentric loading of the quadriceps muscle in the upper leg, an athlete extends his lower leg from a vertical position (see figure (a)) to a fully extended horizontal position (see figure (b)) at a constant angular speed of 45.0° per second. Two of the four quadriceps muscles, the vastis intermedius and the rectus femoris, terminate at the patellar tendon which is attached to the top of the tibia in the lower leg. The distance from the point of attachment of the patellar tendon to the rotation axis of the tibia relative to the femur is 4.10 cm in this athlete. a b (a) The two quadriceps muscles can exert a maximum force of 225 N through the patellar tendon. This force is applied at an angle of 25.0° to the section of the tibia between the attachment point and the rotation axis. What is the torque (in N⚫ m) exerted by the muscle on the lower leg during this motion? (Enter the magnitude.) N⚫ m (b) What is the power (in W) generated by the athlete during the motion? W (c)…arrow_forward= A hanging weight, with a mass of m₁ = 0.365 kg, is attached by a rope to a block with mass m₂ 0.835 kg as shown in the figure below. The rope goes over a pulley with a mass of M = 0.350 kg. The pulley can be modeled as a hollow cylinder with an inner radius of R₁ = 0.0200 m, and an outer radius of R2 = 0.0300 m; the mass of the spokes is negligible. As the weight falls, the block slides on the table, and the coefficient of kinetic friction between the block and the table is μk = 0.250. At the instant shown, the block is moving with a velocity of v; = 0.820 m/s toward the pulley. Assume that the pulley is free to spin without friction, that the rope does not stretch and does not slip on the pulley, and that the mass of the rope is negligible. mq R₂ R₁ mi (a) Using energy methods, find the speed of the block (in m/s) after it has moved a distance of 0.700 m away from the initial position shown. m/s (b) What is the angular speed of the pulley (in rad/s) after the block has moved this…arrow_forwardno AI, pleasearrow_forward
- no AI, pleasearrow_forwardno AI, pleasearrow_forwardTwo astronauts, each having a mass of 95.5 kg, are connected by a 10.0-m rope of negligible mass. They are isolated in space, moving in circles around the point halfway between them at a speed of 4.60 m/s. Treating the astronauts as particles, calculate each of the following. CG × d (a) the magnitude of the angular momentum of the system kg m2/s (b) the rotational energy of the system KJ By pulling on the rope, the astronauts shorten the distance between them to 5.00 m. (c) What is the new angular momentum of the system? kg m2/s (d) What are their new speeds? m/s (e) What is the new rotational energy of the system? KJ (f) How much work is done by the astronauts in shortening the rope? KJarrow_forward
- A uniform horizontal disk of radius 5.50 m turns without friction at w = 2.55 rev/s on a vertical axis through its center, as in the figure below. A feedback mechanism senses the angular speed of the disk, and a drive motor at A ensures that the angular speed remain constant while a m = 1.20 kg block on top of the disk slides outward in a radial slot. The block starts at the center of the disk at time t = 0 and moves outward with constant speed v = 1.25 cm/s relative to the disk until it reaches the edge at t = 360 s. The sliding block experiences no friction. Its motion is constrained to have constant radial speed by a brake at B, producing tension in a light string tied to the block. (a) Find the torque as a function of time that the drive motor must provide while the block is sliding. Hint: The torque is given by t = 2mrvw. t N.m (b) Find the value of this torque at t = 360 s, just before the sliding block finishes its motion. N.m (c) Find the power which the drive motor must…arrow_forward(a) A planet is in an elliptical orbit around a distant star. At its closest approach, the planet is 0.670 AU from the star and has a speed of 54.0 km/s. When the planet is at its farthest distance from the star of 36.0 AU, what is its speed (in km/s)? (1 AU is the average distance from the Earth to the Sun and is equal to 1.496 × 1011 m. You may assume that other planets and smaller objects in the star system exert negligible forces on the planet.) km/s (b) What If? A comet is in a highly elliptical orbit around the same star. The comet's greatest distance from the star is 25,700 times larger than its closest distance to the star. The comet's speed at its greatest distance is 2.40 x 10-2 km/s. What is the speed (in km/s) of the comet at its closest approach? km/sarrow_forwardYou are attending a county fair with your friend from your physics class. While walking around the fairgrounds, you discover a new game of skill. A thin rod of mass M = 0.505 kg and length = 2.70 m hangs from a friction-free pivot at its upper end as shown in the figure. Pivot Velcro M Incoming Velcro-covered ball m The front surface of the rod is covered with Velcro. You are to throw a Velcro-covered ball of mass m = 1.25 kg at the rod in an attempt to make it swing backward and rotate all the way across the top. The ball must stick to the rod at all times after striking it. If you cause the rod to rotate over the top position (that is, rotate 180° opposite of its starting position), you win a stuffed animal. Your friend volunteers to try his luck. He feels that the most torque would be applied to the rod by striking it at its lowest end. While he prepares to aim at the lowest point on the rod, you calculate how fast he must throw the ball to win the stuffed animal with this…arrow_forward
- 56 is not the correct answer!arrow_forward81 SSM Figure 29-84 shows a cross section of an infinite conducting sheet carrying a current per unit x-length of 2; the current emerges perpendicularly out of the page. (a) Use the Biot-Savart law and symmetry to show that for all points B •P x B P'. Figure 29-84 Problem 81. P above the sheet and all points P' below it, the magnetic field B is parallel to the sheet and directed as shown. (b) Use Ampere's law to prove that B = ½µλ at all points P and P'.arrow_forward(λvacuum =640nm) red light (λ vacuum = 640 nm) and green light perpendicularly on a soap film (n=1.31) A mixture of (a vacuum = 512 nm) shines that has air on both side. What is the minimum nonzero thickness of the film, so that destructive interference to look red in reflected light? nm Causes itarrow_forward
 College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning University Physics (14th Edition)PhysicsISBN:9780133969290Author:Hugh D. Young, Roger A. FreedmanPublisher:PEARSON
University Physics (14th Edition)PhysicsISBN:9780133969290Author:Hugh D. Young, Roger A. FreedmanPublisher:PEARSON Introduction To Quantum MechanicsPhysicsISBN:9781107189638Author:Griffiths, David J., Schroeter, Darrell F.Publisher:Cambridge University Press
Introduction To Quantum MechanicsPhysicsISBN:9781107189638Author:Griffiths, David J., Schroeter, Darrell F.Publisher:Cambridge University Press Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Lecture- Tutorials for Introductory AstronomyPhysicsISBN:9780321820464Author:Edward E. Prather, Tim P. Slater, Jeff P. Adams, Gina BrissendenPublisher:Addison-Wesley
Lecture- Tutorials for Introductory AstronomyPhysicsISBN:9780321820464Author:Edward E. Prather, Tim P. Slater, Jeff P. Adams, Gina BrissendenPublisher:Addison-Wesley College Physics: A Strategic Approach (4th Editio...PhysicsISBN:9780134609034Author:Randall D. Knight (Professor Emeritus), Brian Jones, Stuart FieldPublisher:PEARSON
College Physics: A Strategic Approach (4th Editio...PhysicsISBN:9780134609034Author:Randall D. Knight (Professor Emeritus), Brian Jones, Stuart FieldPublisher:PEARSON





