Concept explainers
Videos
(a)
To Find: The classification of the shape of the distribution, or symmetric, skewed left or skewed right.
(a)
Answer to Problem 3CA
The shape is skewed to the right, the majority of the distribution to the left.
Given:
The given diagram is shown in Figure 1
Figure 1
Calculation:
From figure 1 the majority of the data is on the left side of the plot. Since, the right whisker is on the longer on the left whisker this shows that the distribution is skewed on the right and the median is used to describe the centre and use the and use the five number summary to determine the variation.
(b)
To Find: The classification of the shape of the distribution, or symmetric, skewed left or skewed right.
The shape is skewed to the left, the majority of the distribution to the right.
Given:
The given diagram is shown in Figure 2
Figure 2
Calculation:
From figure 1 the majority of the data is on the right side of the plot. Since, the left whisker is on the longer on the right whisker this shows that the distribution is skewed on the left and the median is used to describe the centre and use the and use the five number summary to determine the variation.
(c)
To Find: The classification of the shape of the distribution, or symmetric, skewed left or skewed right.
The shape is skewed to the left, the majority of the distribution to the right.
Given:
The given diagram is shown in Figure 3
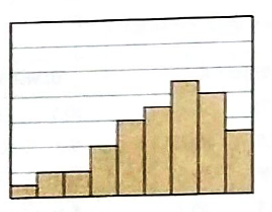
Figure 3
Calculation:
From figure 1 the majority of the data is on the right side of the plot. Since, the left whisker is on the longer on the right whisker this shows that the distribution is skewed on the left and the median is used to describe the centre and use the and use the five number summary to determine the variation.
(d)
To Find: The classification of the shape of the distribution, or symmetric, skewed left or skewed right.
The shape is skewed to the right, the majority of the distribution to the left.
Given:
The given diagram is shown in Figure 3
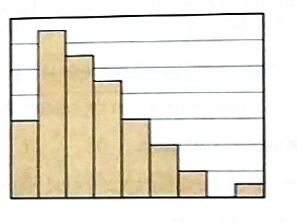
Figure 3
Calculation:
From figure 1 the majority of the data is on the left side of the plot. Since, the right whisker is on the longer on the left whisker this shows that the distribution is skewed on the right and the median is used to describe the centre and use the and use the five number summary to determine the variation.
Explanation of Solution
Given:
The given diagram is shown in Figure 1
Figure 1
Calculation:
From figure 1 the majority of the data is on the left side of the plot. Since, the right whisker is on the longer on the left whisker this shows that the distribution is skewed on the right and the median is used to describe the centre and use the and use the five number summary to determine the variation.
(b)
To Find: The classification of the shape of the distribution, or symmetric, skewed left or skewed right.
(b)
Answer to Problem 3CA
The shape is skewed to the left, the majority of the distribution to the right.
Given:
The given diagram is shown in Figure 2
Figure 2
Calculation:
From figure 1 the majority of the data is on the right side of the plot. Since, the left whisker is on the longer on the right whisker this shows that the distribution is skewed on the left and the median is used to describe the centre and use the and use the five number summary to determine the variation.
(c)
To Find: The classification of the shape of the distribution, or symmetric, skewed left or skewed right.
The shape is skewed to the left, the majority of the distribution to the right.
Given:
The given diagram is shown in Figure 3
Figure 3
Calculation:
From figure 1 the majority of the data is on the right side of the plot. Since, the left whisker is on the longer on the right whisker this shows that the distribution is skewed on the left and the median is used to describe the centre and use the and use the five number summary to determine the variation.
(d)
To Find: The classification of the shape of the distribution, or symmetric, skewed left or skewed right.
The shape is skewed to the right, the majority of the distribution to the left.
Given:
The given diagram is shown in Figure 3
Figure 3
Calculation:
From figure 1 the majority of the data is on the left side of the plot. Since, the right whisker is on the longer on the left whisker this shows that the distribution is skewed on the right and the median is used to describe the centre and use the and use the five number summary to determine the variation.
Explanation of Solution
Given:
The given diagram is shown in Figure 2
Figure 2
Calculation:
From figure 1 the majority of the data is on the right side of the plot. Since, the left whisker is on the longer on the right whisker this shows that the distribution is skewed on the left and the median is used to describe the centre and use the and use the five number summary to determine the variation.
(c)
To Find: The classification of the shape of the distribution, or symmetric, skewed left or skewed right.
(c)
Answer to Problem 3CA
The shape is skewed to the left, the majority of the distribution to the right.
Given:
The given diagram is shown in Figure 3
Figure 3
Calculation:
From figure 1 the majority of the data is on the right side of the plot. Since, the left whisker is on the longer on the right whisker this shows that the distribution is skewed on the left and the median is used to describe the centre and use the and use the five number summary to determine the variation.
(d)
To Find: The classification of the shape of the distribution, or symmetric, skewed left or skewed right.
The shape is skewed to the right, the majority of the distribution to the left.
Given:
The given diagram is shown in Figure 3
Figure 3
Calculation:
From figure 1 the majority of the data is on the left side of the plot. Since, the right whisker is on the longer on the left whisker this shows that the distribution is skewed on the right and the median is used to describe the centre and use the and use the five number summary to determine the variation.
Explanation of Solution
Given:
The given diagram is shown in Figure 3
Figure 3
Calculation:
From figure 1 the majority of the data is on the right side of the plot. Since, the left whisker is on the longer on the right whisker this shows that the distribution is skewed on the left and the median is used to describe the centre and use the and use the five number summary to determine the variation.
(d)
To Find: The classification of the shape of the distribution, or symmetric, skewed left or skewed right.
(d)
Answer to Problem 3CA
The shape is skewed to the right, the majority of the distribution to the left.
Given:
The given diagram is shown in Figure 3
Figure 3
Calculation:
From figure 1 the majority of the data is on the left side of the plot. Since, the right whisker is on the longer on the left whisker this shows that the distribution is skewed on the right and the median is used to describe the centre and use the and use the five number summary to determine the variation.
Explanation of Solution
Given:
The given diagram is shown in Figure 3
Figure 3
Calculation:
From figure 1 the majority of the data is on the left side of the plot. Since, the right whisker is on the longer on the left whisker this shows that the distribution is skewed on the right and the median is used to describe the centre and use the and use the five number summary to determine the variation.
Chapter 7 Solutions
BIG IDEAS MATH Integrated Math 1: Student Edition 2016
- A particle travels along a straight line path given by s=9.5t3-2.2t2-4.5t+9.9 (in meters). What time does it change direction? Report the higher of the answers to the nearest 2 decimal places in seconds.arrow_forward11:18 91 chisholminstitute.bksblive2.com.au 1.5 ACSF L5 SC Geometry and Measure: Pythagorus' Theorum Pythagorean Problems Calculate the lengths of all of this triangle's sides. x = 64 cm² y A ↑ ४ 225 cm² + ? Image not drawn accurately. 45 45arrow_forwardWhat is WX explain your reasoningarrow_forward
- Use the method of disks to find the volume of the solid that is obtained when the region under the curve y = over the interval [4,17] is rotated about the x-axis.arrow_forwardsearch P(QP) Q 1.-P:PR 2.-QP:PR 3. Q:MT 1, 2 4. Q:DNE 3 Submit 4.5 P. (QP) Q 1. P: PR 2. Q-P PR 3. -Q:AS 4. -P:MP 2, 3 5. Submit 17 A Previous Chapter Next Chapterarrow_forward1. Find the area of the region enclosed between the curves y = x and y = x. Sketch the region.arrow_forward
- for the given rectangular coordinates, find two sets of polar coordinates for which 0≤θ<2π, one with r>0 and the other with r<0. (-2sqrt(3),9)arrow_forward3) Find the general solution to the following differential equation. d²x = +4x sin² (2t) dt²arrow_forwardI circled the correct answer, could you show me how to do it using divergence and polar coordinatesarrow_forward
- The correct answer is D Could you explain and show the steps pleasearrow_forwardAre the two statements A and B equivalent? (A) p~q (B) ~pq ☐ Statement A and B are equivalent. ☐ Statement A and B are not equivalent as their values in three rows are not identical. ☐ Statement A and B are not equivalent as their values in one row is not identical. ☐ Statement A and B are not equivalent as their values in two row are not identical.arrow_forwardLet p, q and r to be True, False and True statements, respectively. What are the values of the statements below. A: B: [(p→q)^~q]→r (pvq) → ~r O O A: False B: False A: True B: True A: False B: True A: True B: Falsearrow_forward
Discrete Mathematics and Its Applications ( 8th I...MathISBN:9781259676512Author:Kenneth H RosenPublisher:McGraw-Hill Education
Mathematics for Elementary Teachers with Activiti...MathISBN:9780134392790Author:Beckmann, SybillaPublisher:PEARSON
Thinking Mathematically (7th Edition)MathISBN:9780134683713Author:Robert F. BlitzerPublisher:PEARSON
Discrete Mathematics With ApplicationsMathISBN:9781337694193Author:EPP, Susanna S.Publisher:Cengage Learning,
Pathways To Math Literacy (looseleaf)MathISBN:9781259985607Author:David Sobecki Professor, Brian A. MercerPublisher:McGraw-Hill Education