College Physics
OER 2016 Edition
ISBN: 9781947172173
Author: OpenStax
Publisher: OpenStax College
expand_more
expand_more
format_list_bulleted
Concept explainers
Textbook Question
Chapter 7, Problem 31PE
Suppose a star 1000 times brighter than our Sun (that is, emitting 1000 times the power) suddenly goes supernova. Using data from Table 7.3: (a) By what factor does its power output increase? (b) How many times brighter than our entire Milky Way galaxy is the supernova? (c) Based on your answers, discuss whether it should be possible to observe supernovas in distant galaxies. Note that there are on the order of 1011 observable galaxies, the average brightness of which is somewhat less than our own galaxy.
Expert Solution & Answer
Trending nowThis is a popular solution!
Students have asked these similar questions
Suppose a star 1000 times brighter than our Sun (that is, emitting 1000 times the power) suddenly goes supernova. Using data from Table 7.3: (a) By what factor does its power output increase? (b) How many times brighter than our entire Milky Way galaxy is the supernova? (c) Based on your answers, discuss whether it should be possible to observe supernovas in distant galaxies. Note that there are on the order of observable galaxies, the average brightness of which is somewhat less than our own galaxy.
The temperature (T) of a planet (ignoring any other sources of heating) is solely a function of
how far away the Sun is (d) and how bright it is (luminosity, L); planets are also reflective, so
the light that they reflect (A) will not change their temperature:
1/4
L(1-A)
T =
16σπα?
o here is just a constant, like TT.
10. How much hotter is the Earth at perihelion than at aphelion? To answer this, solve for
the ratio TT, where d, = r, (the perihelion distance) and d, = r, (the aphelion distance).
You should have found that r, = 1.034rp. If you did not, use this new value and redo the
previous problems.
The geometry of spacetime in the Universe on large scales is determined by the mean energy density of the matter in the Universe, ρ. The critical density of the Universe is denoted by ρ0 and can be used to define the parameter Ω0 = ρ/ρ0. Describe the geometry of space when: (i) Ω0 < 1; (ii) Ω0 = 1; (iii) Ω0 > 1. Explain how measurements of the angular sizes of the hot- and cold-spots in the CMB projected on the sky can inform us about the geometry of spacetime in our Universe. What do measurements of these angular sizes by the WMAP and PLANCK satellites tell us about the value of Ω0?
Chapter 7 Solutions
College Physics
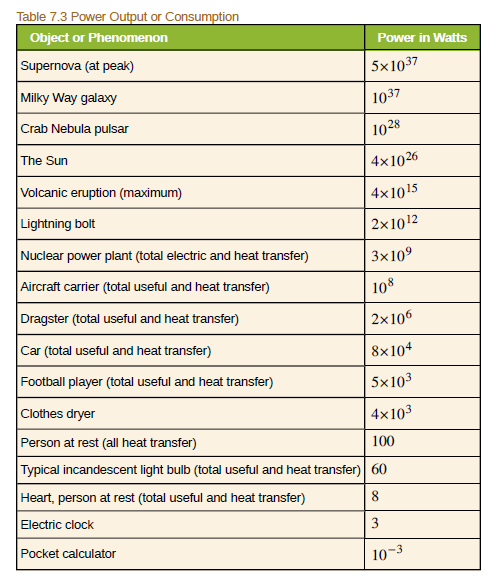
Ch. 7 - Give an example of something think of as work in...Ch. 7 - Give an example of a situation in which there is a...Ch. 7 - Describe a situation in which a force is exerted...Ch. 7 - The person in Figure 7.33 does work on the lawn...Ch. 7 - Work done on a system puts energy into it Work...Ch. 7 - When solving for speed in Example 7.4, we kept...Ch. 7 - In Example 7.7, we calculated the final speed of a...Ch. 7 - Does the work you do on a book when you lift it...Ch. 7 - What is a conservative force?Ch. 7 - The force exerted by a diving board is...
Ch. 7 - Define mechanical energy. What is the relationship...Ch. 7 - What is the relationship of potential energy to...Ch. 7 - Consider the following scenario. A car for which...Ch. 7 - Describe the energy transfers and transformations...Ch. 7 - Do devices with efficiencies of less than one...Ch. 7 - List four different forms or types of energy. Give...Ch. 7 - List the energy conversions that occur when riding...Ch. 7 - Most electrical appliances are rated in watts....Ch. 7 - Explain, in terms of the definition of power, why...Ch. 7 - A spark of static electricity, such as that you...Ch. 7 - Explain why it is easier to climb a mountain on a...Ch. 7 - Do you do work on the outside world when you rub...Ch. 7 - Shivering is an involuntary response to lowered...Ch. 7 - Discuss the relative effectiveness of dieting and...Ch. 7 - What is the difference between energy conservation...Ch. 7 - If the efficiency of a coal-fired electrical...Ch. 7 - How much work does a supermarket checkout...Ch. 7 - A 75.0-kg person climbs stairs, gaining 2.50...Ch. 7 - (a) Calculate the work done on a 1500-kg elevator...Ch. 7 - Suppose a car travels 108 km at a speed of 30.0...Ch. 7 - Calculate the work done by an 85.0-kg man who...Ch. 7 - How much work is done by the boy pulling his...Ch. 7 - A shopper pushes a grocery cart 20.0 m at constant...Ch. 7 - Suppose the ski patrol lowers a rescue sled and...Ch. 7 - Compare the kinetic energy of a 20,000-kg truck...Ch. 7 - (a) How fast must a 3000-kg elephant move to have...Ch. 7 - Confirm the value given for the kinetic energy of...Ch. 7 - (a) Calculate the force needed to bring a 950-kg...Ch. 7 - A car's bumper is designed to withstand a 4.0-km/h...Ch. 7 - Boxing gloves are padded to lessen the force of a...Ch. 7 - Using energy considerations, calculate the average...Ch. 7 - A hydroelectric power facility (see Figure 7.38)...Ch. 7 - (a) How much gravitational potential energy...Ch. 7 - Suppose a 350-g kookaburra (a large kingfisher...Ch. 7 - In Example 7.7, we found that the speed of a...Ch. 7 - A 100-g toy car is propelled by a compressed...Ch. 7 - In a downhill ski race, surprisingly, little...Ch. 7 - A 5.00105 -kg subway train is brought to a stop...Ch. 7 - A pogo stick has a spring with a force constant of...Ch. 7 - A 60.0-kg skier with an initial speed of 12.0 m/s...Ch. 7 - (a) How high a hill can a car coast up (engine...Ch. 7 - Using values from Table 7.1, how many DNA...Ch. 7 - Using energy considerations and assuming...Ch. 7 - If the energy in fusion bombs were used to supply...Ch. 7 - (a) Use of hydrogen fusion to supply energy is a...Ch. 7 - The Crab Nebula (see Figure 7.41) pulsar is the...Ch. 7 - Suppose a star 1000 times brighter than our Sun...Ch. 7 - A person in good physical condition can put out...Ch. 7 - What is the cost of operating a 3.00-W electric...Ch. 7 - A large household air conditioner may consume 15.0...Ch. 7 - (a) What is the average power consumption in watts...Ch. 7 - (a) What is the average useful power output of a...Ch. 7 - A 500-kg dragster accelerates from rest to a final...Ch. 7 - (a) How long will it take an 850-kg car with a...Ch. 7 - (a) Find the useful power output of an elevator...Ch. 7 - (a) What is the available energy content, in...Ch. 7 - (a) How long would it takea 1.50105 -kg airplane...Ch. 7 - Calculate the power output needed for a 950-kg car...Ch. 7 - (a) Calculate the power per square meter reaching...Ch. 7 - (a) How long can you rapidly climb stairs...Ch. 7 - (a) What is the power output in watts and...Ch. 7 - Calculate the power output in watts and horsepower...Ch. 7 - (a) What is the efficiency of an out-of-condition...Ch. 7 - Energy that is not utilized for work or heat...Ch. 7 - Using data from Table 7.5, calculate the daily...Ch. 7 - What is the efficiency of a subject on a treadmill...Ch. 7 - Shoveling snow can be extremely taxing because the...Ch. 7 - Very large forces are produced in joints when a...Ch. 7 - Jogging on hard surfaces with insufficiently...Ch. 7 - (a) Calculate the energy in kJ used by a 55.0-kg...Ch. 7 - Kanellos Kanellopoulos flew 119 km from Crete to...Ch. 7 - The swimmer shown in Figure 7.44 exerts an average...Ch. 7 - Mountain climbers carry bottled oxygen when at...Ch. 7 - The awe-inspiring Great Pyramid of Cheops was...Ch. 7 - (a) How long can you play tennis on the 800 kJ...Ch. 7 - Integrated Concepts (a) Calculate the force the...Ch. 7 - Integrated Concepts A 75.0-kg cross-country skier...Ch. 7 - Integrated Concepts The 70.0-kg swimmer in Figure...Ch. 7 - Integrated Concepts A toy gun uses a spring with a...Ch. 7 - Integrated Concepts (a) What force must be...Ch. 7 - Unreasonable Results A car advertisement claims...Ch. 7 - Unreasonable Results Body fat is metabolized,...Ch. 7 - Construct Your Own Problem Consider a person...Ch. 7 - Construct Your Own Problem Consider humans...Ch. 7 - Integrated Concepts A 105-kg basketball player...Ch. 7 - Prob. 1TPCh. 7 - Prob. 2TPCh. 7 - Prob. 3TPCh. 7 - Prob. 4TPCh. 7 - Prob. 5TPCh. 7 - Prob. 6TPCh. 7 - Prob. 7TPCh. 7 - Prob. 8TPCh. 7 - Prob. 9TPCh. 7 - Prob. 10TPCh. 7 - Prob. 11TPCh. 7 - Prob. 12TPCh. 7 - Prob. 13TPCh. 7 - Prob. 14TPCh. 7 - Prob. 15TPCh. 7 - Prob. 16TPCh. 7 - Prob. 17TPCh. 7 - Prob. 18TPCh. 7 - Prob. 19TPCh. 7 - Prob. 20TPCh. 7 - Prob. 21TPCh. 7 - Prob. 22TPCh. 7 - Prob. 23TPCh. 7 - Prob. 24TPCh. 7 - Prob. 25TPCh. 7 - Prob. 26TPCh. 7 - Prob. 27TPCh. 7 - Prob. 28TPCh. 7 - Prob. 29TPCh. 7 - Prob. 30TPCh. 7 - Prob. 31TPCh. 7 - Prob. 32TPCh. 7 - Prob. 33TPCh. 7 - Prob. 34TPCh. 7 - Prob. 35TPCh. 7 - Prob. 36TPCh. 7 - Prob. 37TPCh. 7 - Prob. 38TPCh. 7 - Prob. 39TPCh. 7 - Prob. 40TPCh. 7 - Prob. 41TPCh. 7 - Prob. 42TP
Additional Science Textbook Solutions
Find more solutions based on key concepts
1. Why is the quantum-mechanical model of the atom important for understanding chemistry?
Chemistry: Structure and Properties (2nd Edition)
1. If an object is not moving, does that mean that there are no forces acting on it? Explain.
College Physics: A Strategic Approach (3rd Edition)
15. A good scientific hypothesis is based on existing evidence and leads to testable predictions. What hypothes...
Campbell Biology: Concepts & Connections (9th Edition)
Police Captain Jeffers has suffered a myocardial infarction. a. Explain to his (nonmedically oriented) family w...
Human Physiology: An Integrated Approach (8th Edition)
5. When the phenotype of heterozygotes is intermediate between the phenotypes of the two homozygotes, this patt...
Biology: Life on Earth (11th Edition)
Define histology.
Fundamentals of Anatomy & Physiology (11th Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.Similar questions
- A cloud of gas has a temperature of 5,000 K. Estimate the width of the hydrogen H-alpha line with an intrinsic wavelength λ = 656 nm. (Note: the typical velocity of hydrogen atoms in a gas with temperature T is about (kT/mH)1/2, where k is Boltzmann constant and mH is the mass of a hydrogen atom, which is approximately the mass of a proton).arrow_forwardA mole contains 6.02 * 1023 particles (atoms, molecules, etc.). If you wanted to reach Alpha Centauri (4.367 light-years away) by creating a strand of carbon atoms (0.3 nm diameter), how many moles of carbon would you need? (Note: 1 light-year = 9.46 * 1012km)arrow_forwardCan you solve the problem?arrow_forward
- You can estimate a star’s radius R(meters) by its luminosity L(Watts) and its surface temperature T(Kelvin) using L = 4πR2σT4, where σ = 5.670 × 10−8 (Wm−2K−4) is the Stephen-Boltzmann constant. Perform a first order error analysis (approximate the error in the function) of an estimate of a star’s radius when L = 9.273×1027W, ∆L = ±0.05L,T = 9400K, ∆T = ±0.02T. Calculate both the error and relative percent error.arrow_forwardA star is formed when the gravitational attraction overcomes the pressure due to the gases inside.Thus we can write(in-picture) .... Assuming the ideal gas equation, we can write P = nkT, where n is the number of atoms/volume. Let M and m denote the total mass and the mass of each gas atom. Using the above equation, show that the condition for star formation is that the mass of the star obeys M > MJ, where : (in-picture)arrow_forwardProblem 2: Black hole – the ultimate blackbody A black hole emits blackbody radiation called Hawking radiation. A black hole with mass M has a total energy of Mc², a surface area of 167G²M² /c*, and a temperature of hc³/167²KGM. a) Estimate the typical wavelength of the Hawking radiation emitted by a 1 solar mass black hole (2 × 103ºkg). Compare your answer to the size of the black hole. b) Calculate the total power radiated by a one-solar mass black hole. c) Imagine a black hole in empty space, where it emits radiation but absorbs nothing. As it loses energy, its mass must decrease; one could say "evaporates". Derive a differential equation for the mass as a function of time, and solve to obtain an expression for the lifetime of a black hole in terms of its mass.arrow_forward
- Twelve out of the 61 ads (I watched all of them) that ran during a past Super Bowl were for cars/trucks/SUVs. Calculate the probability that three randomly selected ads (with replacement) are not for a car/truck/SUV. Round to three decimals as needed.arrow_forwardWhat is the wavelength in micrometers of peak emission for a black body at 33.5°C? (c = 3.0 × 108 m/s, Wien displacement law constant is 2.9 × 10-3 m ∙ K, σ = 5.67 × 10-8 W/m2 ∙ K4). Please give your answer with one decimal place.arrow_forwardModels of the first star-forming clouds indicate that they had a temperature of roughly 150 K and a particle density of roughly 400,000 particles per cubic centimeter at the time they started trapping their internal thermal energy. ▼ Part A Estimate the mass at which thermal pressure balances gravity for these values of pressure and temperature. Express your answer in kilograms. —| ΑΣΦ Mcloud Submit Part B = Mcloud How does that mass compare with the Sun's mass? Express your answer in solar masses. Submit Request Answer = ΤΙ ΑΣΦ Request Answer ? ? kg MSun Reviewarrow_forward
- suppose you collected a data set in which you measured fall-times for different fall-heights, you plotted the data and fit the mathemathical model, y=Ax^2, to match the physical hypothesis, y=0.5*g*t^2. From the best fit curve you are told that the value of your fit-parameter, A, is for 4.6 m/s^2 +/- 0.4 m/s^2. Determine the value of g +/- delta g for this fit parameter value.arrow_forwardPlease solve accurate. Thanksarrow_forwardanswer for 3arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
University Physics (14th Edition)PhysicsISBN:9780133969290Author:Hugh D. Young, Roger A. FreedmanPublisher:PEARSON
Introduction To Quantum MechanicsPhysicsISBN:9781107189638Author:Griffiths, David J., Schroeter, Darrell F.Publisher:Cambridge University Press
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Lecture- Tutorials for Introductory AstronomyPhysicsISBN:9780321820464Author:Edward E. Prather, Tim P. Slater, Jeff P. Adams, Gina BrissendenPublisher:Addison-Wesley
College Physics: A Strategic Approach (4th Editio...PhysicsISBN:9780134609034Author:Randall D. Knight (Professor Emeritus), Brian Jones, Stuart FieldPublisher:PEARSON
College Physics
Physics
ISBN:9781305952300
Author:Raymond A. Serway, Chris Vuille
Publisher:Cengage Learning
University Physics (14th Edition)
Physics
ISBN:9780133969290
Author:Hugh D. Young, Roger A. Freedman
Publisher:PEARSON
Introduction To Quantum Mechanics
Physics
ISBN:9781107189638
Author:Griffiths, David J., Schroeter, Darrell F.
Publisher:Cambridge University Press
Physics for Scientists and Engineers
Physics
ISBN:9781337553278
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
Lecture- Tutorials for Introductory Astronomy
Physics
ISBN:9780321820464
Author:Edward E. Prather, Tim P. Slater, Jeff P. Adams, Gina Brissenden
Publisher:Addison-Wesley
College Physics: A Strategic Approach (4th Editio...
Physics
ISBN:9780134609034
Author:Randall D. Knight (Professor Emeritus), Brian Jones, Stuart Field
Publisher:PEARSON