
Concept explainers
Expected return and standard deviation A game of chance offers the following odds and payoffs. Each play of the game costs $100, so the net profit per play is the payoff less $100.
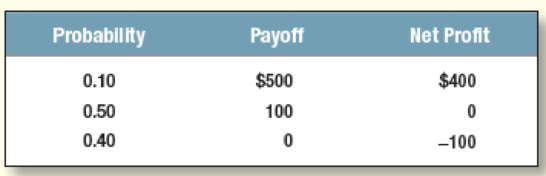
What are the expected cash payoff and expected
To compute: The expected payoff and expected rate of return.
Explanation of Solution
The formula to calculate expected payoff is as follows:
The computation of expected payoff is as follows:
Hence, the expected payoff is $100
The formula to calculate rate of return is as follows:
The calculation of rate of return is as follows:
The formula to calculate expected rate of return is as follows:
The calculation of expected rate of return is as follows:
Hence, the expected rate of return is 0%.
To compute: The variance and standard deviation of rate of return.
Explanation of Solution
The formula to calculate variance is as follows:
The computation of variance is as follows:
Hence, the variance is 20,000.
The formula to compute standard deviation is as follows:
The computation of standard deviation is as follows:
Hence, the standard deviation is 141.42%.
Want to see more full solutions like this?
Chapter 7 Solutions
Principles of Corporate Finance (Mcgraw-hill/Irwin Series in Finance, Insurance, and Real Estate)
- if image is blurr or data is not clear in image please comment i will write data or upload new image. Don't use chatgpt. confirm i will give unhelpful if answer with using incorrect data .arrow_forwardBright wood Seating sells reclining chairs for $55.00 per unit. The variable cost is 322 per unit. Each reclining chair requires 5 direct labor hours and 3 machine hours to produce. atribution margin pegmachine home* Wrightwood Manufacturing has a break-even point of 1,500 units. The sales price per unit is $18, and the variable cost per u 13. If the company sells 3,500 units, what will its net income be? Creatwood Industries provides the following budget data for its Processing Department for the year 2022: ⚫ Manufacturing Overhead Costs=$250,400 . Direct Labor Costs $1,234,500 Determine the manufacturing overhead application rate underthe base of Direct Labor Costs. Modesto Accessories manufactures two types of wallets leather and canvas. The company allocates manufacturing overhead using a single plant wide rate with direct labor cost as the allocation base. $48 Estimated Overhead Costs = 30,600 Direct Labor Cost per Leather Wallet Direct Labor Cost per Canvas Wallet = $52 Number of…arrow_forwardPlease don't use AI . Chatgpt giving wrong answer . confirm i will give unhelpharrow_forward
- Anti-Pandemic Pharma Co. Ltd. reports the following information inits income statement:Sales = $5,250,000;Costs = $2, 173,000;Other expenses = $187,400;Depreciation expense = $79,000;Interest expense= $53,555;Taxes = $76,000;Dividends = $69,000.$136,700 worth of new shares were also issued during the year andlong-term debt worth $65,300 was redeemed.a) Compute the cash flow from assetsb) Compute the net change in working capital Show all calculations. Please no spreadsheet so that I can clearly understandarrow_forwardAnswer this question Using the Google drive liink below it hhas the case study In the scenario, Sharp’s employer has been putting more emphasis on controlling costsfor the various businesses. With the slowing of overall spending in the construction sector,Travolta had ordered managers to closely monitor expenses. He had sold several companiesand has given vice presidents greater responsibility for statements of financial positions. Whatpositive and negative consequences might this pose to the company in future fraud prevention?Outline at least three of each type. Please use sources and insert intext citiations Apa 7 format in the answer and provide the links and references below. https://docs.google.com/document/d/1MpthrFl3eAnMKR-EprYRP9sMo8Ll0WtbhxhpGtLbdcw/edit?usp=sharingarrow_forwardfile:///C:/Users/rafan/Downloads/Assignment%201%20Paving%20Company%20Case%20S2%202024%20to%202025.pdf Using the link for the fraud case answer only this question below. b) As discussed in units 1 to 4, all frauds involve key elements. Identify and describe usingexamples, the elements of Sharp’s fraud.arrow_forward
- Option should be match experts are giving incorrect answer they are using AI /Chatgpt that is generating wrong answer. i will give unhelpful if answer will not match in option. dont use AI alsoarrow_forwardOption should be match. please don't use ai if option will not match means answer is incorrect . Ai giving incorrect answerarrow_forwardOption should be match. please don't use ai if option will not match means answer is incorrect . Ai giving incorrect answerarrow_forward
- all frauds involve key elements. Identify and describe usingexamples the elements of fraudarrow_forwardSolve for maturity value, discount period, bank discount, and proceeds. Assume a bank discount rate of 9%. Use the ordinary interest method. (Use Days in a year table.) Note: Do not round intermediate calculations. Round your final answers to the nearest cent. face value(principal) $50000rate interest:11%length of note: 95 days maturity value: ?date of note: june 10date note discounted: July 18discount period:?bank discount:?proceeds:?arrow_forwardWhat are the different types of audits and different types of auditors? WHat is an example of each type of audit? What is the significance of each from the perspective of different stakeholders?arrow_forward
 Intermediate Financial Management (MindTap Course...FinanceISBN:9781337395083Author:Eugene F. Brigham, Phillip R. DavesPublisher:Cengage Learning
Intermediate Financial Management (MindTap Course...FinanceISBN:9781337395083Author:Eugene F. Brigham, Phillip R. DavesPublisher:Cengage Learning Essentials of Business Analytics (MindTap Course ...StatisticsISBN:9781305627734Author:Jeffrey D. Camm, James J. Cochran, Michael J. Fry, Jeffrey W. Ohlmann, David R. AndersonPublisher:Cengage Learning
Essentials of Business Analytics (MindTap Course ...StatisticsISBN:9781305627734Author:Jeffrey D. Camm, James J. Cochran, Michael J. Fry, Jeffrey W. Ohlmann, David R. AndersonPublisher:Cengage Learning EBK CONTEMPORARY FINANCIAL MANAGEMENTFinanceISBN:9781337514835Author:MOYERPublisher:CENGAGE LEARNING - CONSIGNMENT
EBK CONTEMPORARY FINANCIAL MANAGEMENTFinanceISBN:9781337514835Author:MOYERPublisher:CENGAGE LEARNING - CONSIGNMENT


