Chapter 7, Problem 16PS

(a)

To determine

The possible values of x and y for a healthy person.

Answer to Problem 16PS

The possible values of x and y for a healthy person is $x\ge 0;y\ge 0$

Explanation of Solution

Given:

Given that for a healthy person who is 4 feet and 10 inches tall, the minimum weight is 91 pounds and increases by 3.65 pounds for every additional inch of height. The maximum weight is about 119 pounds and increases by 4.85 pounds for every additional inch of height.

Formula used:

Comparing and forming the equation

Calculation:

To calculate the range of permitted weight range for the given height range. The minimum and maximum permitted for a person having 4feet and 10 inches and permitted increase of weight for every increased height in inches.

Given that for a healthy person who is 4 feet and 10 inches tall, the minimum weight is 91 pounds and increases by 3.65 pounds for every additional inch of height. The maximum weight is about 119 pounds and increases by 4.85 pounds for every additional inch of height.

(a)

The system of inequalities describing the relation if x denotes every additional increase of height and y is the weight. Also weight and increase it height cannot be negative.

The system of inequalities can be given as:

  $\begin{array}{l}y\ge 91+3.65x\\ y\le 119+4.85x\\ x\ge 0\\ y\ge 0\end{array}$

Conclusion:

The weight and increase in height cannot be negative. The possible values of x and y for a healthy person is $x\ge 0;y\ge 0$

(b)

To determine

The graph of the system of inequalities.

Answer to Problem 16PS

The system of inequalities are

  $\begin{array}{l}y\ge 91+3.65x\\ y\le 119+4.85x\\ x\ge 0\\ y\ge 0\end{array}$

Explanation of Solution

Given:

Given that for a healthy person who is 4 feet and 10 inches tall, the minimum weight is 91 pounds and increases by 3.65 pounds for every additional inch of height. The maximum weight is about 119 pounds and increases by 4.85 pounds for every additional inch of height

Formula used:

The substitution method is used

Calculation:

The system of inequalities can be given as:

  $\begin{array}{l}y\ge 91+3.65x\\ y\le 119+4.85x\\ x\ge 0\\ y\ge 0\end{array}$

The graph can be as shown below:

  

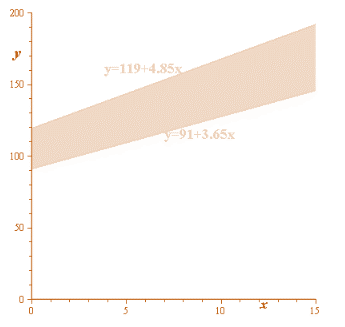
Conclusion:

The graph is the increasing function and it get increases.

(c)

To determine

The recommended weight range for a healthy person with 6 feet tall.

Answer to Problem 16PS

The Minimum weight is l42.l pounds and maximum weight as l86.9 pounds.

Explanation of Solution

Given:

The recommended weight range for a healthy person with 6 feet tall.

Formula used:

The substitution method is used.

Calculation:

To find the recommended weight range for a person with 6 feet height

From 4 feet 10 inches to 6 feet, the x value should be 14, hence to find the values of minimum and maximum weight at $x=14$

  $\begin{array}{l}y=91+3.65x\\ y=119+4.85x\end{array}$

At $x=14$ , the equation can be,

  $\begin{array}{l}y=91+3.65\left(14\right)=91+51.1=142.1\\ y=119+4.85\left(14\right)=119+67.9=186.9\end{array}$

The Minimum weight is l42.l pounds and maximum weight as l86.9 pounds.

Conclusion:

The Minimum weight is l42.l pounds and maximum weight as l86.9 pounds.