Chapter 6.3, Problem 42E

(a)

To determine

To find: The integral.

Answer to Problem 42E

The integral is: $e^x(x-1)+C$

Explanation of Solution

Given information:

The integration is $\int xe{}^{x}dx$

Calculation:

Put $n=1$ into the integral:

Use integration by parts $\int udv=ub-\int vdu$ Using LIPET (Log, Inv, Trig, Poly, Exp, Trig),

Let,

  $\begin{array}{c}u=x\\ dv=e^{x}dx\end{array}$

Then,

  $\begin{array}{c}du=dx\\ v=e^x\end{array}$

Evaluate the remaining integral after rewriting it using integration by parts.

  $\begin{array}{c}\int x^n{e}^{x}dx=\int x{e}^{x}dx\\ =x{e}^x-\int e^{x}dx\\ =x{e}^x-e^x+C\\ =e^x(x-1)+C\end{array}$

The required integral of the given integration $\int xe{}^{x}dx$ is $e^x(x-1)+C$ .

(b)

To determine

To find: The integral.

Answer to Problem 42E

The integral is: $e^x\left(x^2-2x+2\right)+C$

Explanation of Solution

Given information:

The integration is $\int xe{}^{x}dx$

Calculation:

Put $n=2$ into the integral:

Use integration by parts $\int udv=ub-\int vdu$ Using LIPET (Log, Inv, Trig, Poly, Exp, Trig),

Let,

  $\begin{array}{c}u=x^2\\ dv=e^{x}dx\end{array}$

Then,

  $\begin{array}{c}du=2xdx\\ v=e^x\end{array}$

Evaluate the remaining integral after rewriting it using integration by parts.

  $\begin{array}{c}\int x^n{e}^{x}dx=\int x^2{e}^{x}dx\\ =x^2{e}^x-\int e^x(2xdx)\\ =x^2{e}^x-2\int x{e}^{x}dx\end{array}$

From part (a) $\int x{e}^{x}dx=e^x(x-1)+C$ put in this and simplify:

  $\begin{array}{c}{x}^2{e}^x-2\left[e^x(x-1)+C\right]\\ =x^2{e}^x-2x{e}^x+2e^x+C\\ =e^x\left(x^2-2x+2\right)+C\end{array}$

The required integral of the given integration $\int xe{}^{x}dx$ is $e^x\left(x^2-2x+2\right)+C$ .

(c)

To determine

To find: The integral.

Answer to Problem 42E

The integral is: $e^x\left(x^3-3x^2+6x-6\right)+C$

Explanation of Solution

Given information:

The integration is $\int xe{}^{x}dx$

Calculation:

Put $n=3$ into the integral:

Use integration by parts $\int udv=ub-\int vdu$ Using LIPET (Log, Inv, Trig, Poly, Exp, Trig),

Let,

  $\begin{array}{c}u=x^3\\ dv=e^{x}dx\end{array}$

Then,

  $\begin{array}{c}du=3x^{2}dx\\ v=e^x\end{array}$

Evaluate the remaining integral after rewriting it using integration by parts.

  $\begin{array}{c}\int x^n{e}^{x}dx=\int x^3{e}^{x}dx\\ =x^3{e}^x-\int e^x\left(3x^{2}dx\right)\\ =x^3{e}^x-3\int x^2{e}^{x}dx\end{array}$

From part (b) $\int x^2{e}^{x}dx=e^x\left(x^2-2x+2\right)+C$ put in this and simplify:

  $\begin{array}{c}{x}^3{e}^x-3\left[e^x\left(x^2-2x+2\right)+C\right]\\ =x^3{e}^x-3x^2{e}^x+6x{e}^x-6e^x+C\\ =e^x\left(x^3-3x^2+6x-6\right)+C\end{array}$

The required integral of the given integration $\int xe{}^{x}dx$ is $e^x\left(x^3-3x^2+6x-6\right)+C$

(d)

To determine

To find: The value of the integral for any positive integer of $n$

Answer to Problem 42E

The integral is: $\int x^n{e}^{x}dx=e^x\left[x^n-\frac{d}{dx}\left(x^n\right)+\frac{d^2}{d{x}^2}\left(x^n\right)-\dots +{(-1)}^n\frac{d^n}{d{x}^n}\left(x^n\right)\right]+C$

Explanation of Solution

Given information:

The integration is $\int xe{}^{x}dx$

Calculation:

When $n=1$ , the result is $e^x(x-1)+C$

When $n=2$ , the result is $e^x\left(x^2-2x+2\right)+C$

When $n=3$ , the result is $e^x\left(x^3-3x^2+6x-6\right)+C$

The outcomes were of the form $e^x$ (polynomial of degree n) $+C$ in all three instances.

Each polynomial's terms contain alternating signs that begin with +. Aside from the + or -, each phrase is also the derivative of the one before it.

The polynomials are then of the form $x^n-\frac{d}{dx}\left(x^n\right)+\frac{d^2}{d{x}^2}\left(x^n\right)-\dots +{(-1)}^n\frac{d^n}{d{x}^n}\left(x^n\right)$

The integral's outcome can then be expressed in write as:

  $\int x^n{e}^{x}dx=e^x\left[x^n-\frac{d}{dx}\left(x^n\right)+\frac{d^2}{d{x}^2}\left(x^n\right)-\dots +{(-1)}^n\frac{d^n}{d{x}^n}\left(x^n\right)\right]+C$

Therefore the required value of the integral for any positive integer of $n$ is

  $\int x^n{e}^{x}dx=e^x\left[x^n-\frac{d}{dx}\left(x^n\right)+\frac{d^2}{d{x}^2}\left(x^n\right)-\dots +{(-1)}^n\frac{d^n}{d{x}^n}\left(x^n\right)\right]+C$

(e)

To determine

To check: The convincing argument that conjecture in part (d) is true or false,

Answer to Problem 42E

The option (d) is true.

Explanation of Solution

Given information:

The integration is $\int xe{}^{x}dx$

Calculation:

From part d)

  $\int x^n{e}^{x}dx=e^x\left[x^n-\frac{d}{dx}\left(x^n\right)+\frac{d^2}{d{x}^2}\left(x^n\right)-\dots +{(-1)}^n\frac{d^n}{d{x}^n}\left(x^n\right)\right]+C$

Use tubular integration

  $\begin{array}{c}f(x)=x^n\\ g(x)=e^x\end{array}$

Then gives,

  

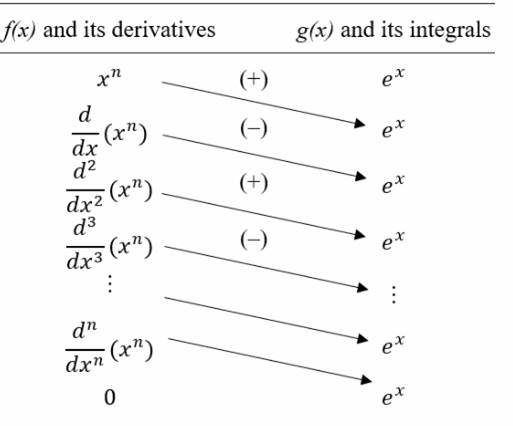
To use the operation signs above each arrow, combine the results of the functions connected by the arrows to produce:

  $\int x^n{e}^{x}dx=e^x{x}^n-e^x\frac{d}{dx}\left(x^n\right)+e^x\frac{d^2}{d{x}^2}\left(x^n\right)-e^x\frac{d^3}{d{x}^3}\left(x^n\right)+\dots +{(-1)}^n\frac{d^n}{d{x}^n}\left(x^n\right)+C$

The last term has a sign of ${(-1)}^n$ hence the first derivative term had a sign of ${(-1)}^1$ , second derivative term had a sign of ${(-1)}^2$ , third had ${(-1)}^3$ etc. so the $n$ th derivative term will have a sign of ${(-1)}^n$ .Factoring out $e^x$ then gives,

  $\int x^n{e}^{x}dx=e^x\left[x^n-\frac{d}{dx}\left(x^n\right)+\frac{d^2}{d{x}^2}\left(x^n\right)-e^x\frac{d^3}{d{x}^3}\left(x^n\right)\dots +{(-1)}^n\frac{d^n}{d{x}^n}\left(x^n\right)\right]+C$

Therefore, the required conjecture from part d) is true.