Chapter 6, Problem 20P

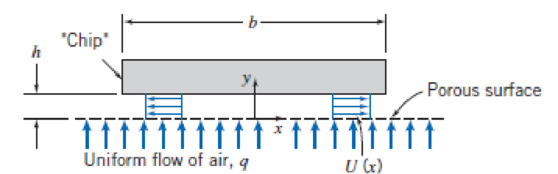
A rectangular computer chip floats on a thin layer of air, h = 0.5 mm thick, above a porous surface. The chip width is b = 40 mm, as shown. Its length, L, is very long in the direction perpendicular to the diagram. There is no flow in the z direction. Assume flow in the x direction in the gap under the chip is uniform. Flow is incompressible, and frictional effects may be neglected. Use a suitably chosen control volume to show that U(x) = qx/h in the gap. Find a general expression for the (2D) acceleration of a fluid particle in the gap in terms of q, h, x, and y. Obtain an expression for the pressure gradient ∂p/∂x. Assuming atmospheric pressure on the chip upper surface, find an expression for the net pressure force on the chip; is it directed upward or downward? Explain. Find the required flow rate q(m³/s/m²) and the maximum velocity, if the mass per unit length of the chip is 0:005 kg/m. Plot the pressure distribution as part of your explanation of the direction of the net force.

P6.20