
Concept explainers
Solve the following exercises based on Principles 18 through 21, although an exercise may require the application oftwo or more of any of the principles. Where necessary, round linear answers in inches to 3 decimal places and millimeters to 2 decimal places. Round angular answers in decimal degrees to 2 decimal places and degrees and minutes to the nearest minute.
a. If
(1) ∠EFD
(2)
(3) ∠1
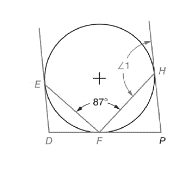
b. If
(1) ∠EFD
(2)
(3) ∠1
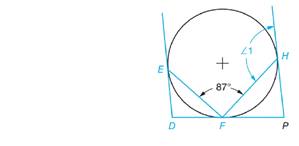
(a)
The value of
Answer to Problem 20A
The value of
Explanation of Solution
Given information:
The given figure is
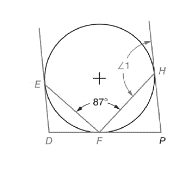
Calculation:
The angle drawn at the point of tangent between the tangent and the chord is equal to half the value of intercepted arc.
The angle EFD, is the angle between the tangent DF and the chord EF, thus, the value of angle EFD will be equal to
The line DFP is the straight line, the angle at the straight line is 180o. The value of angle FHP is to be calculated for calculating the value of arc HF.
The angle drawn at the point of tangent between the tangent and the chord is equal to half the value of intercepted arc. So,
To calculate the value of angle 1, let us first calculate the value of arc FEH.
Similarly, for angle 1, the angle drawn at the point of tangent between the tangent and the chord is equal to half the value of intercepted arc.
Conclusion:
Thus, the value of
(b)
The value of
Answer to Problem 20A
The value of
Explanation of Solution
Given information:
The given figure is
Calculation:
The angle drawn at the point of tangent between the tangent and the chord is equal to half the value of intercepted arc.
The angle EFD, is the angle between the tangent DF and the chord EF, thus, the value of angle EFD will be equal to
The line DFP is the straight line, the angle at the straight line is 180o. The value of angle FHP is to be calculated for calculating the value of arc HF.
The angle drawn at the point of tangent between the tangent and the chord is equal to half the value of intercepted arc. So,
To calculate the value of angle 1, let us first calculate the value of arc FEH.
Similarly, for angle 1, the angle drawn at the point of tangent between the tangent and the chord is equal to half the value of intercepted arc.
Conclusion:
Thus, the value of
Want to see more full solutions like this?
Chapter 54 Solutions
EBK MATHEMATICS FOR MACHINE TECHNOLOGY
- Consider the weighted voting system [16: 15, 8, 3, 1]Find the Banzhaf power distribution of this weighted voting system.List the power for each player as a fraction: P1: P2: P3: P4:arrow_forwardNo chatgpt pls willarrow_forwardConsider the weighted voting system [9: 7, 4, 1]Find the Shapley-Shubik power distribution of this weighted voting system.List the power for each player as a fraction:P1: P2: P3:arrow_forward
- Consider the weighted voting system [11: 7, 4, 1]Find the Shapley-Shubik power distribution of this weighted voting system.List the power for each player as a fraction: P1: P2: P3:arrow_forwardConsider the weighted voting system [18: 15, 8, 3, 1]Find the Banzhaf power distribution of this weighted voting system.List the power for each player as a fraction: P1: P2: P3: P4:arrow_forwardConsider the weighted voting system [18: 15, 8, 3, 1]Find the Banzhaf power distribution of this weighted voting system.List the power for each player as a fraction: P1 = P2 = P3 = P4 =arrow_forward
- Consider the weighted voting system [18: 15, 8, 3, 1]Find the Banzhaf power distribution of this weighted voting system.List the power for each player as a fraction: P1: P2: P3: P4:arrow_forwardConsider the weighted voting system [18: 15, 8, 3, 1]Find the Banzhaf power distribution of this weighted voting system.List the power for each player as a fraction: P1: P2: P3: P4:arrow_forwardFind the Banzhaf power distribution of the weighted voting system[26: 19, 15, 11, 6]Give each player's power as a fraction or decimal value P1 = P2 = P3 = P4 =arrow_forward
- solve it using augmented matrix. Also it is homeworkarrow_forward4. Now we'll look at a nonhomogeneous example. The general form for these is y' + p(x)y = f(x). For this problem, we will find solutions of the equation +2xy= xe (a) Identify p(x) and f(x) in the equation above. p(x) = f(x) = (b) The complementary equation is y' + p(x)y = 0. Write the complementary equation. (c) Find a solution for the complementary equation. We'll call this solution y₁. (You only need one particular solution, so you can let k = 0 here.) Y1 = (d) Check that y₁ satisfies the complementary equation, in other words, that y₁+ p(x)y₁ = 0.arrow_forwarddata managementarrow_forward
 Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning, Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,
Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage, Elementary Geometry for College StudentsGeometryISBN:9781285195698Author:Daniel C. Alexander, Geralyn M. KoeberleinPublisher:Cengage Learning
Elementary Geometry for College StudentsGeometryISBN:9781285195698Author:Daniel C. Alexander, Geralyn M. KoeberleinPublisher:Cengage Learning- Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
 Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning
Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal Littell
Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal Littell





