Chapter 5, Problem 3P

Determine the real root of $f\left(x\right)=-25+82x-90x^2+44x^3-8x^4+0.7x^5$:

(a) Graphically.

(b) Using bisection to determine the root to ${\epsilon }_s=10\%$. Employ initial guesses of $x_l=0.5\text{and }{x}_u=1.0$.

(c) Perform the same computation as in (b) but use the false-position method and ${\epsilon }_s=0.2\%$.

To determine

The real roots of the equation $f\left(x\right)=-25+82x-90x^2+44x^3-8x^4+0.7x^5$ using the graphical method.

Answer to Problem 3P

Solution:

The approximate real root of the equation is 0.6.

Explanation of Solution

Given Information:

The equation $f\left(x\right)=-25+82x-90x^2+44x^3-8x^4+0.7x^5$.

Calculation:

The graph of the function can be plotted using MATLAB.

Code:

% (a)

clc;

x=linspace(-1,1);

y1=0.7*x.^5−8*x.^4+44*x.^3−90*x.^2+82*x−25;

y2=0;

plot(x,y1);

hold on

line([-1,1],[y2,y2])

Output:

This gives the following plot:

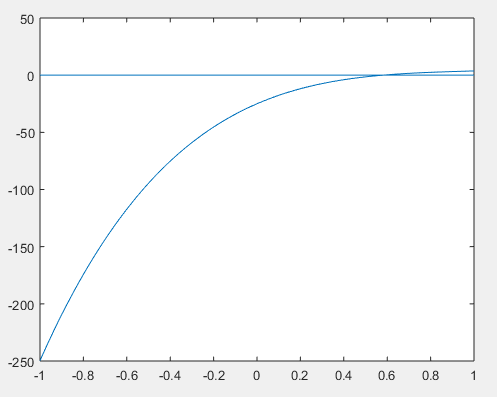
The roots of an equation can be represented graphically by the x-coordinate of the point where the graph cuts the x-axis. From the plot, the only zeros of the equation can be approximated as 0.6.

(b)

To calculate: The root of the equation $f\left(x\right)=-25+82x-90x^2+44x^3-8x^4+0.7x^5$ using the bisection method by assuming $x_l$ and $x_u$ to be 0.5 and 1 respectively with the stopping criteria being the iteration where ${\epsilon }_s$ falls below 10%.

Solution:

The root of the equation can be approximated as 0.59375.

Given Information:

The equation $f\left(x\right)=-25+82x-90x^2+44x^3-8x^4+0.7x^5$.

Formula Used:

A root of an equation can be obtained using the bisection method as follows:

1. Choose 2 values x, say a andb such that $f\left(a\right)f\left(b\right)<0$.

2. Now, estimate the root by $x_1=\frac{a+b}{2}$.

3. If, $f\left(a\right)f\left(x_1\right)<0$, the root would lie between a and $x_1$. Now assume the next root to be $x_2=\frac{a+x_1}{2}$. If, $f\left(a\right)f\left(x_1\right)>0$, the root would lie between b and $x_1$. Now assume the next root to be $x_2=\frac{b+x_1}{2}$ and if $f\left(a\right)f\left(x_1\right)=0$, the root is $x_1$.

Calculation:

For the provided function:

$\begin{array}{c}f\left(0.5\right)=-25+82\left(0.5\right)-90{\left(0.5\right)}^2+44{\left(0.5\right)}^3-8{\left(0.5\right)}^4+0.7{\left(0.5\right)}^5\\ =-1.47812\\ f\left(1\right)=-25+82\left(1\right)-90{\left(1\right)}^2+44{\left(1\right)}^3-8{\left(1\right)}^4+0.7{\left(1\right)}^5\\ =3.7\end{array}$

Hence,

$f\left(0.5\right)f\left(1\right)<0$

Now take the first root to be,

$\begin{array}{c}{x}_1=\frac{0.5+1}{2}\\ =0.75\end{array}$

Now,

$\begin{array}{c}f\left(0.75\right)=-25+82\left(0.75\right)-90{\left(0.75\right)}^2+44{\left(0.75\right)}^3-8{\left(0.75\right)}^4+0.7{\left(0.75\right)}^5\\ =2.07236\end{array}$

Thus, $f\left(0.5\right)f\left(0.75\right)<0$. This implies that the root would be between 0.5 and 0.75.

Now, the second root would be:

$\begin{array}{c}{x}_2=\frac{0.5+0.75}{2}\\ =0.625\end{array}$

The approximate error can be computed as:

$\begin{array}{c}{\epsilon }_a=\left(\left|\frac{0.625-0.75}{0.625}\right|\times 100\right)\%\\ =20\%\end{array}$

The approximate relative percentage error is 200%.

Now,

$\begin{array}{c}f\left(0.625\right)=-25+82\left(0.625\right)-90{\left(0.625\right)}^2+44{\left(0.625\right)}^3-8{\left(0.625\right)}^4+0.7{\left(0.625\right)}^5\\ =0.68199\end{array}$

Thus, $f\left(0.5\right)f\left(0.625\right)<0$. This implies that the root would be between 0.5 and 0.625.

Now, the third root would be:

$\begin{array}{c}{x}_3=\frac{0.5+0.625}{2}\\ =0.5625\end{array}$

The approximate error can be computed as:

$\begin{array}{c}{\epsilon }_a=\left(\left|\frac{0.5625-0.625}{0.5625}\right|\times 100\right)\%\\ =11.1\%\end{array}$

The approximate error is 11.1%.

Now,

$\begin{array}{c}f\left(0.5625\right)=-25+82\left(0.5625\right)-90{\left(0.5625\right)}^2+44{\left(0.5625\right)}^3-8{\left(0.5625\right)}^4+0.7{\left(0.5625\right)}^5\\ =-0.28199\end{array}$

Thus, $f\left(0.5625\right)f\left(0.625\right)<0$. This implies that the root would be between 0.375 and 0.625.

Now, the fourth root would be:

$\begin{array}{c}{x}_4=\frac{0.5625+0.625}{2}\\ =0.59375\end{array}$

The approximate error can be computed as:

$\begin{array}{c}{\epsilon }_a=\left(\left|\frac{0.59375-0.5625}{0.59375}\right|\times 100\right)\%\\ =5.26\%\end{array}$

The approximate error is 5.26%.

As ${\epsilon }_a$ is less than 10%, the process is stopped and root can be approximated as 0.59375.

(c)

To calculate: The root of the equation $f\left(x\right)=-25+82x-90x^2+44x^3-8x^4+0.7x^5$ using the false-position method by assuming $x_l$ and $x_u$ to be 0.5 and 1 respectively with the stopping criteria being the iteration where ${\epsilon }_s$ falls below 0.2%.

Solution:

The root of the equation can be approximated as 0.57956.

Given Information:

The equation $f\left(x\right)=-25+82x-90x^2+44x^3-8x^4+0.7x^5$.

Formula Used:

A root of an equation can be obtained using the false-position method as follows:

1. Choose 2 values x, say a andb such that $f\left(a\right)f\left(b\right)<0$.

2. Now, estimate the root by $x_1=b-\frac{f\left(b\right)\left(a-b\right)}{f\left(a\right)-f\left(b\right)}$.

3. If, $f\left(a\right)f\left(x_1\right)<0$, the root would lie between a and $x_1$. Now assume the next root to be $x_2=x_1-\frac{f\left(x_1\right)\left(a-x_1\right)}{f\left(a\right)-f\left(x_1\right)}$. If, $f\left(a\right)f\left(x_1\right)>0$, the root would lie between $x_1$ and b Now assume the next root to be $x_2=b-\frac{f\left(b\right)\left(x_1-b\right)}{f\left(x_i\right)-f\left(b\right)}$ and if $f\left(a\right)f\left(x_1\right)=0$, the root is $x_1$.

Calculation:

For the provided function:

$\begin{array}{c}f\left(0.5\right)=-25+82\left(0.5\right)-90{\left(0.5\right)}^2+44{\left(0.5\right)}^3-8{\left(0.5\right)}^4+0.7{\left(0.5\right)}^5\\ =-1.47812\\ f\left(1\right)=-25+82\left(1\right)-90{\left(1\right)}^2+44{\left(1\right)}^3-8{\left(1\right)}^4+0.7{\left(1\right)}^5\\ =3.7\end{array}$

Hence,

$f\left(0.5\right)f\left(1\right)<0$

Now take the first root to be,

$\begin{array}{c}{x}_1=0.5-\frac{\left(-1.47812\right)\left(0.5-1\right)}{-1.47812-3.7}\\ =0.64273\end{array}$

Now,

$\begin{array}{c}f\left(0.64273\right)=-25+82\left(0.64273\right)-90{\left(0.64273\right)}^2+44{\left(0.64273\right)}^3-8{\left(0.64273\right)}^4+0.7{\left(0.64273\right)}^5\\ =0.91879\end{array}$

Thus, $f\left(0.5\right)f\left(0.6473\right)<0$. This implies that the root would be between 0.5 and 0.64273.

Now, the second root would be:

$\begin{array}{c}{x}_2=0.5-\frac{\left(-1.47812\right)\left(0.5-0.64273\right)}{-1.47812-0.91879}\\ =0.58802\end{array}$

The approximate error can be computed as:

$\begin{array}{c}{\epsilon }_a=\left(\left|\frac{0.58802-0.64273}{0.58802}\right|\times 100\right)\%\\ =9.4\%\end{array}$

The approximate relative percentage error is 200%.

Now,

$\begin{array}{c}f\left(0.58802\right)=-25+82\left(0.58802\right)-90{\left(0.58802\right)}^2+44{\left(0.58802\right)}^3-8{\left(0.58802\right)}^4+0.7{\left(0.58802\right)}^5\\ =0.13729\end{array}$

Thus, $f\left(0.5\right)f\left(0.58802\right)<0$. This implies that the root would be between 0.5 and 0.58802.

Now, the third root would be:

$\begin{array}{c}{x}_3=0.5-\frac{\left(-1.47812\right)\left(0.5-0.58802\right)}{-1.47812-0.13729}\\ =0.58054\end{array}$

The approximate error can be computed as:

$\begin{array}{c}{\epsilon }_a=\left(\left|\frac{0.58054-0.58802}{0.58054}\right|\times 100\right)\%\\ =1.29\%\end{array}$

The approximate error is 1.29%.

Now,

$\begin{array}{c}f\left(0.58054\right)=-25+82\left(0.58054\right)-90{\left(0.58054\right)}^2+44{\left(0.58054\right)}^3-8{\left(0.58054\right)}^4+0.7{\left(0.58054\right)}^5\\ =0.01822\end{array}$

Thus, $f\left(0.5\right)f\left(0.58054\right)<0$. This implies that the root would be between 0.5 and 0.58054.

Now, the fourth root would be:

$\begin{array}{c}{x}_4=0.5-\frac{\left(-1.47812\right)\left(0.5-0.58054\right)}{-1.47812-0.01822}\\ =0.57956\end{array}$

The approximate error can be computed as:

$\begin{array}{c}{\epsilon }_a=\left(\left|\frac{0.57656-0.58054}{0.57956}\right|\times 100\right)\%\\ =0.17\%\end{array}$

The approximate error is 0.17%.

As ${\epsilon }_a$ is less than 0.2%, the process is stopped and root can be approximated as 0.57956.

To determine

To calculate: The root of the equation $f\left(x\right)=-25+82x-90x^2+44x^3-8x^4+0.7x^5$ using the bisection method by assuming $x_l$ and $x_u$ to be 0.5 and 1 respectively with the stopping criteria being the iteration where ${\epsilon }_s$ falls below 10%.

Answer to Problem 3P

Solution:

The root of the equation can be approximated as 0.59375.

Explanation of Solution

Given Information:

The equation $f\left(x\right)=-25+82x-90x^2+44x^3-8x^4+0.7x^5$.

Formula Used:

A root of an equation can be obtained using the bisection method as follows:

1. Choose 2 values x, say a andb such that $f\left(a\right)f\left(b\right)<0$.

2. Now, estimate the root by $x_1=\frac{a+b}{2}$.

3. If, $f\left(a\right)f\left(x_1\right)<0$, the root would lie between a and $x_1$. Now assume the next root to be $x_2=\frac{a+x_1}{2}$. If, $f\left(a\right)f\left(x_1\right)>0$, the root would lie between b and $x_1$. Now assume the next root to be $x_2=\frac{b+x_1}{2}$ and if $f\left(a\right)f\left(x_1\right)=0$, the root is $x_1$.

Calculation:

For the provided function:

$\begin{array}{c}f\left(0.5\right)=-25+82\left(0.5\right)-90{\left(0.5\right)}^2+44{\left(0.5\right)}^3-8{\left(0.5\right)}^4+0.7{\left(0.5\right)}^5\\ =-1.47812\\ f\left(1\right)=-25+82\left(1\right)-90{\left(1\right)}^2+44{\left(1\right)}^3-8{\left(1\right)}^4+0.7{\left(1\right)}^5\\ =3.7\end{array}$

Hence,

$f\left(0.5\right)f\left(1\right)<0$

Now take the first root to be,

$\begin{array}{c}{x}_1=\frac{0.5+1}{2}\\ =0.75\end{array}$

Now,

$\begin{array}{c}f\left(0.75\right)=-25+82\left(0.75\right)-90{\left(0.75\right)}^2+44{\left(0.75\right)}^3-8{\left(0.75\right)}^4+0.7{\left(0.75\right)}^5\\ =2.07236\end{array}$

Thus, $f\left(0.5\right)f\left(0.75\right)<0$. This implies that the root would be between 0.5 and 0.75.

Now, the second root would be:

$\begin{array}{c}{x}_2=\frac{0.5+0.75}{2}\\ =0.625\end{array}$

The approximate error can be computed as:

$\begin{array}{c}{\epsilon }_a=\left(\left|\frac{0.625-0.75}{0.625}\right|\times 100\right)\%\\ =20\%\end{array}$

The approximate relative percentage error is 200%.

Now,

$\begin{array}{c}f\left(0.625\right)=-25+82\left(0.625\right)-90{\left(0.625\right)}^2+44{\left(0.625\right)}^3-8{\left(0.625\right)}^4+0.7{\left(0.625\right)}^5\\ =0.68199\end{array}$

Thus, $f\left(0.5\right)f\left(0.625\right)<0$. This implies that the root would be between 0.5 and 0.625.

Now, the third root would be:

$\begin{array}{c}{x}_3=\frac{0.5+0.625}{2}\\ =0.5625\end{array}$

The approximate error can be computed as:

$\begin{array}{c}{\epsilon }_a=\left(\left|\frac{0.5625-0.625}{0.5625}\right|\times 100\right)\%\\ =11.1\%\end{array}$

The approximate error is 11.1%.

Now,

$\begin{array}{c}f\left(0.5625\right)=-25+82\left(0.5625\right)-90{\left(0.5625\right)}^2+44{\left(0.5625\right)}^3-8{\left(0.5625\right)}^4+0.7{\left(0.5625\right)}^5\\ =-0.28199\end{array}$

Thus, $f\left(0.5625\right)f\left(0.625\right)<0$. This implies that the root would be between 0.375 and 0.625.

Now, the fourth root would be:

$\begin{array}{c}{x}_4=\frac{0.5625+0.625}{2}\\ =0.59375\end{array}$

The approximate error can be computed as:

$\begin{array}{c}{\epsilon }_a=\left(\left|\frac{0.59375-0.5625}{0.59375}\right|\times 100\right)\%\\ =5.26\%\end{array}$

The approximate error is 5.26%.

As ${\epsilon }_a$ is less than 10%, the process is stopped and root can be approximated as 0.59375.

(c)

To calculate: The root of the equation $f\left(x\right)=-25+82x-90x^2+44x^3-8x^4+0.7x^5$ using the false-position method by assuming $x_l$ and $x_u$ to be 0.5 and 1 respectively with the stopping criteria being the iteration where ${\epsilon }_s$ falls below 0.2%.

Solution:

The root of the equation can be approximated as 0.57956.

Given Information:

The equation $f\left(x\right)=-25+82x-90x^2+44x^3-8x^4+0.7x^5$.

Formula Used:

A root of an equation can be obtained using the false-position method as follows:

1. Choose 2 values x, say a andb such that $f\left(a\right)f\left(b\right)<0$.

2. Now, estimate the root by $x_1=b-\frac{f\left(b\right)\left(a-b\right)}{f\left(a\right)-f\left(b\right)}$.

3. If, $f\left(a\right)f\left(x_1\right)<0$, the root would lie between a and $x_1$. Now assume the next root to be $x_2=x_1-\frac{f\left(x_1\right)\left(a-x_1\right)}{f\left(a\right)-f\left(x_1\right)}$. If, $f\left(a\right)f\left(x_1\right)>0$, the root would lie between $x_1$ and b Now assume the next root to be $x_2=b-\frac{f\left(b\right)\left(x_1-b\right)}{f\left(x_i\right)-f\left(b\right)}$ and if $f\left(a\right)f\left(x_1\right)=0$, the root is $x_1$.

Calculation:

For the provided function:

$\begin{array}{c}f\left(0.5\right)=-25+82\left(0.5\right)-90{\left(0.5\right)}^2+44{\left(0.5\right)}^3-8{\left(0.5\right)}^4+0.7{\left(0.5\right)}^5\\ =-1.47812\\ f\left(1\right)=-25+82\left(1\right)-90{\left(1\right)}^2+44{\left(1\right)}^3-8{\left(1\right)}^4+0.7{\left(1\right)}^5\\ =3.7\end{array}$

Hence,

$f\left(0.5\right)f\left(1\right)<0$

Now take the first root to be,

$\begin{array}{c}{x}_1=0.5-\frac{\left(-1.47812\right)\left(0.5-1\right)}{-1.47812-3.7}\\ =0.64273\end{array}$

Now,

$\begin{array}{c}f\left(0.64273\right)=-25+82\left(0.64273\right)-90{\left(0.64273\right)}^2+44{\left(0.64273\right)}^3-8{\left(0.64273\right)}^4+0.7{\left(0.64273\right)}^5\\ =0.91879\end{array}$

Thus, $f\left(0.5\right)f\left(0.6473\right)<0$. This implies that the root would be between 0.5 and 0.64273.

Now, the second root would be:

$\begin{array}{c}{x}_2=0.5-\frac{\left(-1.47812\right)\left(0.5-0.64273\right)}{-1.47812-0.91879}\\ =0.58802\end{array}$

The approximate error can be computed as:

$\begin{array}{c}{\epsilon }_a=\left(\left|\frac{0.58802-0.64273}{0.58802}\right|\times 100\right)\%\\ =9.4\%\end{array}$

The approximate relative percentage error is 200%.

Now,

$\begin{array}{c}f\left(0.58802\right)=-25+82\left(0.58802\right)-90{\left(0.58802\right)}^2+44{\left(0.58802\right)}^3-8{\left(0.58802\right)}^4+0.7{\left(0.58802\right)}^5\\ =0.13729\end{array}$

Thus, $f\left(0.5\right)f\left(0.58802\right)<0$. This implies that the root would be between 0.5 and 0.58802.

Now, the third root would be:

$\begin{array}{c}{x}_3=0.5-\frac{\left(-1.47812\right)\left(0.5-0.58802\right)}{-1.47812-0.13729}\\ =0.58054\end{array}$

The approximate error can be computed as:

$\begin{array}{c}{\epsilon }_a=\left(\left|\frac{0.58054-0.58802}{0.58054}\right|\times 100\right)\%\\ =1.29\%\end{array}$

The approximate error is 1.29%.

Now,

$\begin{array}{c}f\left(0.58054\right)=-25+82\left(0.58054\right)-90{\left(0.58054\right)}^2+44{\left(0.58054\right)}^3-8{\left(0.58054\right)}^4+0.7{\left(0.58054\right)}^5\\ =0.01822\end{array}$

Thus, $f\left(0.5\right)f\left(0.58054\right)<0$. This implies that the root would be between 0.5 and 0.58054.

Now, the fourth root would be:

$\begin{array}{c}{x}_4=0.5-\frac{\left(-1.47812\right)\left(0.5-0.58054\right)}{-1.47812-0.01822}\\ =0.57956\end{array}$

The approximate error can be computed as:

$\begin{array}{c}{\epsilon }_a=\left(\left|\frac{0.57656-0.58054}{0.57956}\right|\times 100\right)\%\\ =0.17\%\end{array}$

The approximate error is 0.17%.

As ${\epsilon }_a$ is less than 0.2%, the process is stopped and root can be approximated as 0.57956.

To determine

To calculate: The root of the equation $f\left(x\right)=-25+82x-90x^2+44x^3-8x^4+0.7x^5$ using the false-position method by assuming $x_l$ and $x_u$ to be 0.5 and 1 respectively with the stopping criteria being the iteration where ${\epsilon }_s$ falls below 0.2%.

Answer to Problem 3P

Solution:

The root of the equation can be approximated as 0.57956.

Explanation of Solution

Given Information:

The equation $f\left(x\right)=-25+82x-90x^2+44x^3-8x^4+0.7x^5$.

Formula Used:

A root of an equation can be obtained using the false-position method as follows:

1. Choose 2 values x, say a andb such that $f\left(a\right)f\left(b\right)<0$.

2. Now, estimate the root by $x_1=b-\frac{f\left(b\right)\left(a-b\right)}{f\left(a\right)-f\left(b\right)}$.

3. If, $f\left(a\right)f\left(x_1\right)<0$, the root would lie between a and $x_1$. Now assume the next root to be $x_2=x_1-\frac{f\left(x_1\right)\left(a-x_1\right)}{f\left(a\right)-f\left(x_1\right)}$. If, $f\left(a\right)f\left(x_1\right)>0$, the root would lie between $x_1$ and b Now assume the next root to be $x_2=b-\frac{f\left(b\right)\left(x_1-b\right)}{f\left(x_i\right)-f\left(b\right)}$ and if $f\left(a\right)f\left(x_1\right)=0$, the root is $x_1$.

Calculation:

For the provided function:

$\begin{array}{c}f\left(0.5\right)=-25+82\left(0.5\right)-90{\left(0.5\right)}^2+44{\left(0.5\right)}^3-8{\left(0.5\right)}^4+0.7{\left(0.5\right)}^5\\ =-1.47812\\ f\left(1\right)=-25+82\left(1\right)-90{\left(1\right)}^2+44{\left(1\right)}^3-8{\left(1\right)}^4+0.7{\left(1\right)}^5\\ =3.7\end{array}$

Hence,

$f\left(0.5\right)f\left(1\right)<0$

Now take the first root to be,

$\begin{array}{c}{x}_1=0.5-\frac{\left(-1.47812\right)\left(0.5-1\right)}{-1.47812-3.7}\\ =0.64273\end{array}$

Now,

$\begin{array}{c}f\left(0.64273\right)=-25+82\left(0.64273\right)-90{\left(0.64273\right)}^2+44{\left(0.64273\right)}^3-8{\left(0.64273\right)}^4+0.7{\left(0.64273\right)}^5\\ =0.91879\end{array}$

Thus, $f\left(0.5\right)f\left(0.6473\right)<0$. This implies that the root would be between 0.5 and 0.64273.

Now, the second root would be:

$\begin{array}{c}{x}_2=0.5-\frac{\left(-1.47812\right)\left(0.5-0.64273\right)}{-1.47812-0.91879}\\ =0.58802\end{array}$

The approximate error can be computed as:

$\begin{array}{c}{\epsilon }_a=\left(\left|\frac{0.58802-0.64273}{0.58802}\right|\times 100\right)\%\\ =9.4\%\end{array}$

The approximate relative percentage error is 200%.

Now,

$\begin{array}{c}f\left(0.58802\right)=-25+82\left(0.58802\right)-90{\left(0.58802\right)}^2+44{\left(0.58802\right)}^3-8{\left(0.58802\right)}^4+0.7{\left(0.58802\right)}^5\\ =0.13729\end{array}$

Thus, $f\left(0.5\right)f\left(0.58802\right)<0$. This implies that the root would be between 0.5 and 0.58802.

Now, the third root would be:

$\begin{array}{c}{x}_3=0.5-\frac{\left(-1.47812\right)\left(0.5-0.58802\right)}{-1.47812-0.13729}\\ =0.58054\end{array}$

The approximate error can be computed as:

$\begin{array}{c}{\epsilon }_a=\left(\left|\frac{0.58054-0.58802}{0.58054}\right|\times 100\right)\%\\ =1.29\%\end{array}$

The approximate error is 1.29%.

Now,

$\begin{array}{c}f\left(0.58054\right)=-25+82\left(0.58054\right)-90{\left(0.58054\right)}^2+44{\left(0.58054\right)}^3-8{\left(0.58054\right)}^4+0.7{\left(0.58054\right)}^5\\ =0.01822\end{array}$

Thus, $f\left(0.5\right)f\left(0.58054\right)<0$. This implies that the root would be between 0.5 and 0.58054.

Now, the fourth root would be:

$\begin{array}{c}{x}_4=0.5-\frac{\left(-1.47812\right)\left(0.5-0.58054\right)}{-1.47812-0.01822}\\ =0.57956\end{array}$

The approximate error can be computed as:

$\begin{array}{c}{\epsilon }_a=\left(\left|\frac{0.57656-0.58054}{0.57956}\right|\times 100\right)\%\\ =0.17\%\end{array}$

The approximate error is 0.17%.

As ${\epsilon }_a$ is less than 0.2%, the process is stopped and root can be approximated as 0.57956.