MyLab Math with Pearson eText -- Standalone Access Card -- for Calculus: Early Transcendentals (3rd Edition)
3rd Edition
ISBN: 9780134856926
Author: William L. Briggs, Lyle Cochran, Bernard Gillett, Eric Schulz
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 4.5, Problem 63E
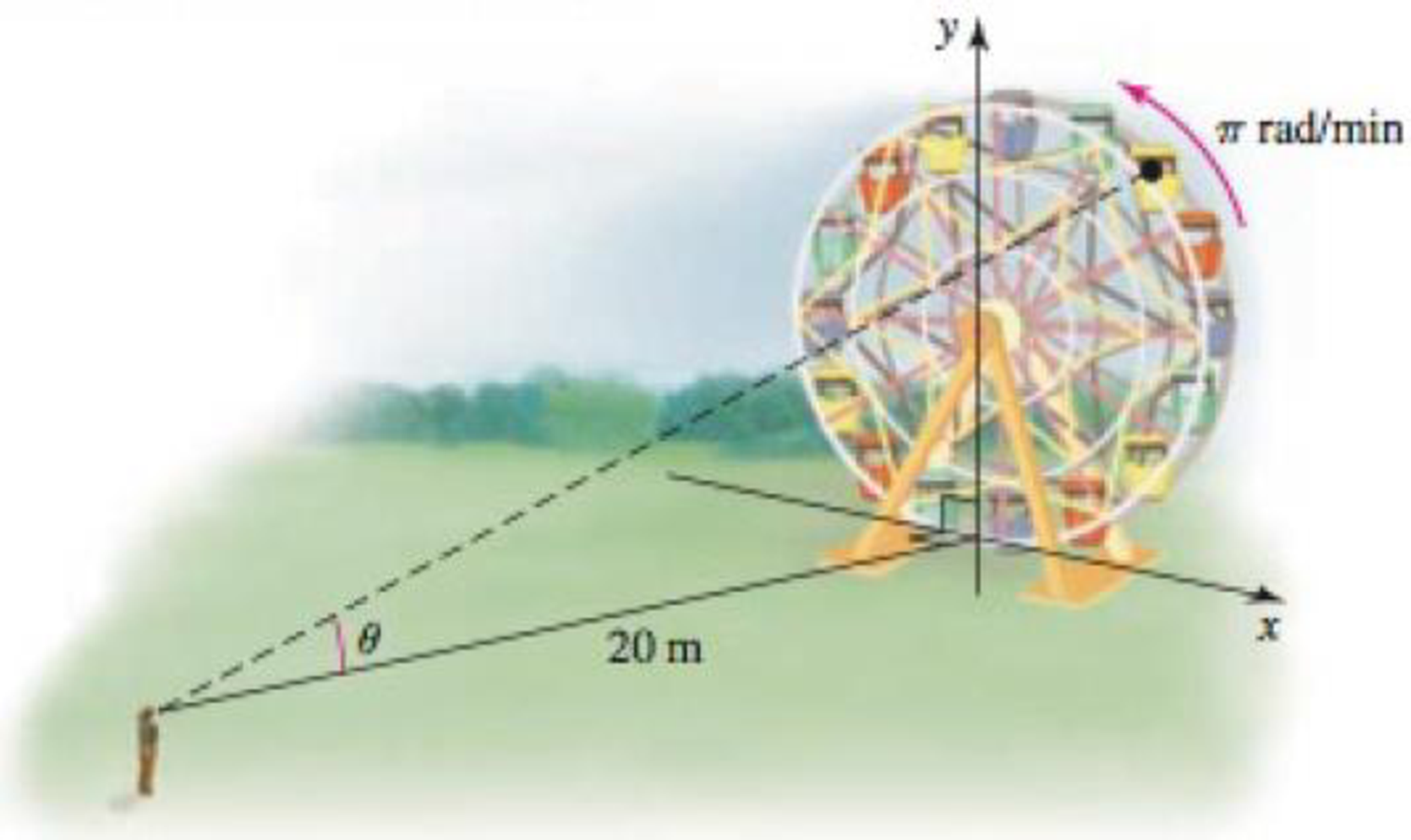
Watching a Ferris wheel An observer stands 20 m from the bottom of a Ferris wheel on a line that is perpendicular to the face of the wheel, with her eyes at the level of the bottom of the wheel. The wheel revolves at a rate of π rad/min, and the observer’s line of sight with a specific seat on the Ferris wheel makes an angle θ with the horizontal (see figure). At what time during a full revolution is θ changing most rapidly?
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Chapter 4 Solutions
MyLab Math with Pearson eText -- Standalone Access Card -- for Calculus: Early Transcendentals (3rd Edition)
Ch. 4.1 - Sketch the graph of a function that is continuous...Ch. 4.1 - Consider the function f(x) = x3. Where is the...Ch. 4.1 - Sketch the graph of a function that is continuous...Ch. 4.1 - Sketch the graph of a function that has an...Ch. 4.1 - What is a critical point of a function?Ch. 4.1 - Sketch the graph of a function f that has a local...Ch. 4.1 - Sketch the graph of a function f that has a local...Ch. 4.1 - Absolute maximum/minimum values Use the following...Ch. 4.1 - Absolute maximum/minimum values Use the following...Ch. 4.1 - Absolute maximum/minimum values Use the following...
Ch. 4.1 - Absolute maximum/minimum values Use the following...Ch. 4.1 - Local and absolute extreme values Use the...Ch. 4.1 - Local and absolute extreme values Use the...Ch. 4.1 - Local and absolute extreme values Use the...Ch. 4.1 - Local and absolute extreme values Use the...Ch. 4.1 - Locating critical points Find the critical points...Ch. 4.1 - Locating critical points Find the critical points...Ch. 4.1 - Locating critical points Find the critical points...Ch. 4.1 - Locating critical points Find the critical points...Ch. 4.1 - Locating critical points Find the critical points...Ch. 4.1 - Locating critical points Find the critical points...Ch. 4.1 - Locating critical points Find the critical points...Ch. 4.1 - Locating critical points Find the critical points...Ch. 4.1 - Locating critical points Find the critical points...Ch. 4.1 - Locating critical points Find the critical points...Ch. 4.1 - Locating critical points Find the critical points...Ch. 4.1 - Locating critical points Find the critical points...Ch. 4.1 - Locating critical points Find the critical points...Ch. 4.1 - Locating critical points Find the critical points...Ch. 4.1 - Locating critical points Find the critical points...Ch. 4.1 - Locating critical points Find the critical points...Ch. 4.1 - Locating critical points Find the critical points...Ch. 4.1 - Locating critical points Find the critical points...Ch. 4.1 - Locating critical points Find the critical points...Ch. 4.1 - Prob. 42ECh. 4.1 - Absolute maxima and minima Determine the location...Ch. 4.1 - Absolute maxima and minima Determine the location...Ch. 4.1 - Absolute maxima and minima Determine the location...Ch. 4.1 - Absolute maxima and minima Determine the location...Ch. 4.1 - Absolute maxima and minima Determine the location...Ch. 4.1 - Absolute maxima and minima Determine the location...Ch. 4.1 - Absolute maxima and minima Determine the location...Ch. 4.1 - Absolute maxima and minima Determine the location...Ch. 4.1 - Absolute maxima and minima Determine the location...Ch. 4.1 - Absolute maxima and minima Determine the location...Ch. 4.1 - Absolute maxima and minima Determine the location...Ch. 4.1 - Absolute maxima and minima Determine the location...Ch. 4.1 - Absolute maxima and minima Determine the location...Ch. 4.1 - Absolute maxima and minima Determine the location...Ch. 4.1 - Absolute maxima and minima Determine the location...Ch. 4.1 - Prob. 58ECh. 4.1 - Absolute maxima and minima Determine the location...Ch. 4.1 - Absolute maxima and minima Determine the location...Ch. 4.1 - Absolute maxima and minima Determine the location...Ch. 4.1 - Absolute maxima and minima Determine the location...Ch. 4.1 - Absolute maxima and minima Determine the location...Ch. 4.1 - Prob. 64ECh. 4.1 - Absolute maxima and minima Determine the location...Ch. 4.1 - Absolute maxima and minima Determine the location...Ch. 4.1 - Absolute maxima and minima Determine the location...Ch. 4.1 - Prob. 68ECh. 4.1 - Efficiency of wind turbines A wind Turbine...Ch. 4.1 - Derivation of wind turbine formula A derivation of...Ch. 4.1 - Suppose the position of an object moving...Ch. 4.1 - Minimum surface area box All boxes with a square...Ch. 4.1 - Trajectory high point A stone is launched...Ch. 4.1 - Maximizing revenue A sales analyst determines that...Ch. 4.1 - Maximizing profit Suppose a tour guide has a bus...Ch. 4.1 - Maximizing rectangle perimeters All rectangles...Ch. 4.1 - Explain why or why not Determine whether the...Ch. 4.1 - Absolute maxima and minima a. Find the critical...Ch. 4.1 - Absolute maxima and minima a. Find the critical...Ch. 4.1 - Critical points and extreme values a. Find the...Ch. 4.1 - Critical points and extreme values a. Find the...Ch. 4.1 - Prob. 82ECh. 4.1 - Prob. 83ECh. 4.1 - Absolute value functions Graph the following...Ch. 4.1 - Prob. 85ECh. 4.1 - Prob. 86ECh. 4.1 - Every second counts You must get from a point P on...Ch. 4.1 - Extreme values of parabolas Consider the function...Ch. 4.1 - Values of related functions Suppose f is...Ch. 4.1 - Prob. 90ECh. 4.1 - Proof of the Local Extreme Value Theorem Prove...Ch. 4.1 - Prob. 92ECh. 4.2 - Where on the interval [0, 4] does f(x) = 4x x2...Ch. 4.2 - Sketch the graph of a function that illustrates...Ch. 4.2 - Give two distinct linear functions f and g that...Ch. 4.2 - Explain Rolles Theorem with a sketch.Ch. 4.2 - Draw the graph of a function for which the...Ch. 4.2 - Explain why Rolles Theorem cannot be applied to...Ch. 4.2 - Explain the Mean Value Theorem with a sketch.Ch. 4.2 - For each function f and interval [a, b], a graph...Ch. 4.2 - For each function f and interval [a, b], a graph...Ch. 4.2 - For each function f and interval [a, b], a graph...Ch. 4.2 - At what points c does the conclusion of the Mean...Ch. 4.2 - Draw the graph of a function for which the...Ch. 4.2 - Letf(x)=x2/3. Show that there is no value of c in...Ch. 4.2 - Rolles Theorem Determine whether Rolles Theorem...Ch. 4.2 - Rolles Theorem Determine whether Rolles Theorem...Ch. 4.2 - Rolles Theorem Determine whether Rolles Theorem...Ch. 4.2 - Rolles Theorem Determine whether Rolles Theorem...Ch. 4.2 - Rolles Theorem Determine whether Rolles Theorem...Ch. 4.2 - Rolles Theorem Determine whether Rolles Theorem...Ch. 4.2 - Rolles Theorem Determine whether Rolles Theorem...Ch. 4.2 - Rolles Theorem Determine whether Rolles Theorem...Ch. 4.2 - Lapse rates in the atmosphere Concurrent...Ch. 4.2 - Drag racer acceleration The fastest drag racers...Ch. 4.2 - Mean Value Theorem Consider the following...Ch. 4.2 - Mean Value Theorem Consider the following...Ch. 4.2 - Mean Value Theorem Consider the following...Ch. 4.2 - Mean Value Theorem Consider the following...Ch. 4.2 - Mean Value Theorem Consider the following...Ch. 4.2 - Mean Value Theorem Consider the following...Ch. 4.2 - Mean Value Theorem Consider the following...Ch. 4.2 - Mean Value Theorem Consider the following...Ch. 4.2 - Mean Value Theorem Consider the following...Ch. 4.2 - Mean Value Theorem Consider the following...Ch. 4.2 - Mean Value Theorem Consider the following...Ch. 4.2 - Mean Value Theorem Consider the following...Ch. 4.2 - Explain why or why not Determine whether the...Ch. 4.2 - Prob. 34ECh. 4.2 - Another inverse tangent identity a.Use derivatives...Ch. 4.2 - Questions about derivatives 26. Without evaluating...Ch. 4.2 - Questions about derivatives 27. Without evaluating...Ch. 4.2 - Questions about derivatives 28. Find all functions...Ch. 4.2 - Mean Value Theorem and graphs By visual...Ch. 4.2 - Mean Value Theorem and graphs Find all points on...Ch. 4.2 - Mean Value Theorem and graphs Find all points on...Ch. 4.2 - Avalanche forecasting Avalanche forecasters...Ch. 4.2 - Mean Value Theorem and the police A state patrol...Ch. 4.2 - Mean Value Theorem and the police again Compare...Ch. 4.2 - Running pace Explain why if a runner completes a...Ch. 4.2 - Mean Value Theorem for linear functions Interpret...Ch. 4.2 - Mean Value Theorem for quadratic functions...Ch. 4.2 - Means a. Show that the point c guaranteed to exist...Ch. 4.2 - Equal derivatives Verify that the functions f(x) =...Ch. 4.2 - 100-m speed The Jamaican sprinter Usain Bolt set a...Ch. 4.2 - Verify the identity sec1x=cos1(1/x),forx0.Ch. 4.2 - Prob. 52ECh. 4.2 - Suppose f(x)2, for allx2, and f(2) = 7. Show that...Ch. 4.2 - Suppose f(x)1, for all x 0, and f(0) = 0. Show...Ch. 4.2 - Use the Mean Value Theorem to prove that 1+a21+a...Ch. 4.2 - Prove the following statements. a.|sinasinb||ab|,...Ch. 4.2 - Generalized Mean Value Theorem Suppose the...Ch. 4.2 - Prob. 58ECh. 4.3 - Explain why a positive derivative on an interval...Ch. 4.3 - Sketch a function f that is differentiable on (−∞,...Ch. 4.3 - Explain how the First Derivative Test determines...Ch. 4.3 - Verify that the function f(x) = x4 is concave up...Ch. 4.3 - Prob. 5QCCh. 4.3 - Explain how the first derivative of a function...Ch. 4.3 - Explain how to apply the First Derivative Test.Ch. 4.3 - Suppose the derivative of f isf(x)=x3. a.Find the...Ch. 4.3 - Suppose the derivative of f isf(x)=(x1)(x2)....Ch. 4.3 - Sketch the graph of a function that has neither a...Ch. 4.3 - The following graph of the derivative g' has...Ch. 4.3 - Functions from derivatives The following figures...Ch. 4.3 - Functions from derivatives The following figures...Ch. 4.3 - Sketches from properties Sketch a graph of a...Ch. 4.3 - f(x) 0 on (, 2); f(x) 0 on (2, 5); f(x) 0 on...Ch. 4.3 - Sketches from properties Sketch a graph of a...Ch. 4.3 - Sketches from properties Sketch a graph of a...Ch. 4.3 - Supposeg(x)=2x. a.On what intervals is g concave...Ch. 4.3 - The following graph of g has exactly three...Ch. 4.3 - Is it possible for a function to satisfy f(x) 0,...Ch. 4.3 - Sketch a function that changes from concave up to...Ch. 4.3 - Give a function that does not have an inflection...Ch. 4.3 - Suppose f is continuous on an interval containing...Ch. 4.3 - Increasing and decreasing functions Find the...Ch. 4.3 - Increasing and decreasing functions Find the...Ch. 4.3 - Increasing and decreasing functions Find the...Ch. 4.3 - Increasing and decreasing functions Find the...Ch. 4.3 - Increasing and decreasing functions Find the...Ch. 4.3 - Increasing and decreasing functions Find the...Ch. 4.3 - Increasing and decreasing functions Find the...Ch. 4.3 - Increasing and decreasing functions Find the...Ch. 4.3 - Increasing and decreasing functions Find the...Ch. 4.3 - Increasing and decreasing functions Find the...Ch. 4.3 - Increasing and decreasing functions Find the...Ch. 4.3 - Increasing and decreasing functions Find the...Ch. 4.3 - Increasing and decreasing functions Find the...Ch. 4.3 - Increasing and decreasing functions Find the...Ch. 4.3 - Increasing and decreasing functions Find the...Ch. 4.3 - Increasing and decreasing functions Find the...Ch. 4.3 - Increasing and decreasing functions Find the...Ch. 4.3 - Increasing and decreasing functions Find the...Ch. 4.3 - Increasing and decreasing functions Find the...Ch. 4.3 - Increasing and decreasing functions Find the...Ch. 4.3 - Increasing and decreasing functions Find the...Ch. 4.3 - Increasing and decreasing functions Find the...Ch. 4.3 - Increasing and decreasing functions Find the...Ch. 4.3 - Increasing and decreasing functions Find the...Ch. 4.3 - Increasing and decreasing functions Find the...Ch. 4.3 - Increasing and decreasing functions Find the...Ch. 4.3 - First Derivative Test a. Locale the critical...Ch. 4.3 - First Derivative Test a. Locale the critical...Ch. 4.3 - First Derivative Test a. Locale the critical...Ch. 4.3 - First Derivative Test a. Locale the critical...Ch. 4.3 - First Derivative Test a. Locale the critical...Ch. 4.3 - First Derivative Test a. Locale the critical...Ch. 4.3 - First Derivative Test a. Locale the critical...Ch. 4.3 - First Derivative Test a. Locale the critical...Ch. 4.3 - First Derivative Test a. Locale the critical...Ch. 4.3 - First Derivative Test a.Locate the critical points...Ch. 4.3 - Absolute extreme values Verify that the following...Ch. 4.3 - Absolute extreme values Verify that the following...Ch. 4.3 - Absolute extreme values Verify that the following...Ch. 4.3 - Absolute extreme values Verify that the following...Ch. 4.3 - Prob. 59ECh. 4.3 - Prob. 60ECh. 4.3 - Prob. 61ECh. 4.3 - Prob. 62ECh. 4.3 - Concavity Determine the intervals on which the...Ch. 4.3 - Concavity Determine the intervals on which the...Ch. 4.3 - Concavity Determine the intervals on which the...Ch. 4.3 - Concavity Determine the intervals on which the...Ch. 4.3 - Concavity Determine the intervals on which the...Ch. 4.3 - Concavity Determine the intervals on which the...Ch. 4.3 - Concavity Determine the intervals on which the...Ch. 4.3 - Concavity Determine the intervals on which the...Ch. 4.3 - Concavity Determine the intervals on which the...Ch. 4.3 - Concavity Determine the intervals on which the...Ch. 4.3 - Concavity Determine the intervals on which the...Ch. 4.3 - Concavity Determine the intervals on which the...Ch. 4.3 - Concavity Determine the intervals on which the...Ch. 4.3 - Concavity Determine the intervals on which the...Ch. 4.3 - Second Derivative Test Locate the critical points...Ch. 4.3 - Second Derivative Test Locate the critical points...Ch. 4.3 - Second Derivative Test Locate the critical points...Ch. 4.3 - Second Derivative Test Locate the critical points...Ch. 4.3 - Second Derivative Test Locate the critical points...Ch. 4.3 - Prob. 82ECh. 4.3 - Second Derivative Test Locate the critical points...Ch. 4.3 - Second Derivative Test Locate the critical points...Ch. 4.3 - Second Derivative Test Locate the critical points...Ch. 4.3 - Second Derivative Test Locate the critical points...Ch. 4.3 - Second Derivative Test Locate the critical points...Ch. 4.3 - Second Derivative Test Locate the critical points...Ch. 4.3 - Second Derivative Test Locate the critical points...Ch. 4.3 - Second Derivative Test Locate the critical points...Ch. 4.3 - Second Derivative Test Locate the critical points...Ch. 4.3 - Second Derivative Test Locate the critical points...Ch. 4.3 - Second Derivative Test Locate the critical points...Ch. 4.3 - Second Derivative Test Locate the critical points...Ch. 4.3 - Explain why or why not Determine whether the...Ch. 4.3 - Is it possible? Determine whether the following...Ch. 4.3 - Matching derivatives and functions The following...Ch. 4.3 - Prob. 100ECh. 4.3 - Prob. 101ECh. 4.3 - Designer functions Sketch the graph of a function...Ch. 4.3 - Prob. 103ECh. 4.3 - Designer functions Sketch the graph of a function...Ch. 4.3 - Designer functions Sketch the graph of a function...Ch. 4.3 - Graph carefully Graph the function f(x) = 60x5 ...Ch. 4.3 - Interpreting the derivative The graph of f on the...Ch. 4.3 - Prob. 108ECh. 4.3 - Prob. 109ECh. 4.3 - Prob. 110ECh. 4.3 - Population models The population of a species is...Ch. 4.3 - Tangent lines and concavity Give an argument to...Ch. 4.3 - General quartic Show that the general quartic...Ch. 4.3 - Properties of cubics Consider the general cubic...Ch. 4.3 - Concavity of parabolas Consider the general...Ch. 4.4 - Graph f(x) = x3/3 - 400x using various windows on...Ch. 4.4 - Explain why the functions f and f + C, where C is...Ch. 4.4 - Prob. 3QCCh. 4.4 - Why is it important to determine the domain of f...Ch. 4.4 - Prob. 2ECh. 4.4 - Prob. 3ECh. 4.4 - Where are the vertical asymptotes of a rational...Ch. 4.4 - How do you find the absolute maximum and minimum...Ch. 4.4 - Describe the possible end behavior of a...Ch. 4.4 - Shape of the curve Sketch a curve with the...Ch. 4.4 - Shape of the curve Sketch a curve with the...Ch. 4.4 - Designer functions Sketch a continuous function f...Ch. 4.4 - Designer functions Sketch a continuous function f...Ch. 4.4 - Designer functions Sketch a continuous function f...Ch. 4.4 - Designer functions Sketch a continuous function f...Ch. 4.4 - Let f(x)=(x3)(x+3)2. a.Verify that f(x)=3(x1)(x+3)...Ch. 4.4 - If , it can be shown that and . Use these...Ch. 4.4 - Graphing functions Use the guidelines of this...Ch. 4.4 - Graphing functions Use the guidelines of this...Ch. 4.4 - Graphing functions Use the guidelines of this...Ch. 4.4 - Graphing functions Use the guidelines of this...Ch. 4.4 - Graphing functions Use the guidelines of this...Ch. 4.4 - Graphing functions Use the guidelines of this...Ch. 4.4 - Graphing functions Use the guidelines of this...Ch. 4.4 - Graphing functions Use the guidelines of this...Ch. 4.4 - Graphing functions Use the guidelines of this...Ch. 4.4 - Graphing functions Use the guidelines of this...Ch. 4.4 - Graphing functions Use the guidelines of this...Ch. 4.4 - Graphing functions Use the guidelines of this...Ch. 4.4 - Graphing functions Use the guidelines of this...Ch. 4.4 - Graphing functions Use the guidelines of this...Ch. 4.4 - Graphing rational functions Use the guidelines of...Ch. 4.4 - Graphing rational functions Use the guidelines of...Ch. 4.4 - Graphing rational functions Use the guidelines of...Ch. 4.4 - Graphing rational functions Use the guidelines of...Ch. 4.4 - Graphing rational functions Use the guidelines of...Ch. 4.4 - Graphing functions Use the guidelines of this...Ch. 4.4 - Graphing functions Use the guidelines of this...Ch. 4.4 - Graphing functions Use the guidelines of this...Ch. 4.4 - Graphing functions Use the guidelines of this...Ch. 4.4 - Prob. 38ECh. 4.4 - Graphing functions Use the guidelines of this...Ch. 4.4 - Prob. 40ECh. 4.4 - Graphing functions Use the guidelines of this...Ch. 4.4 - Graphing functions Use the guidelines of this...Ch. 4.4 - Graphing functions Use the guidelines of this...Ch. 4.4 - Graphing functions Use the guidelines of this...Ch. 4.4 - Graphing functions Use the guidelines of this...Ch. 4.4 - Graphing functions Use the guidelines of this...Ch. 4.4 - Functions from graphs Use the graphs of f and f to...Ch. 4.4 - Functions from graphs Use the graphs of f and f to...Ch. 4.4 - Graphing with technology Make a complete graph of...Ch. 4.4 - Graphing with technology Make a complete graph of...Ch. 4.4 - Graphing with technology Make a complete graph of...Ch. 4.4 - Graphing with technology Make a complete graph of...Ch. 4.4 - Graphing with technology Make a complete graph of...Ch. 4.4 - Graphing with technology Make a complete graph of...Ch. 4.4 - Explain why or why not Determine whether the...Ch. 4.4 - Functions from derivatives Use the derivative f to...Ch. 4.4 - Functions from derivatives Use the derivative f to...Ch. 4.4 - Functions from derivatives Use the derivative f to...Ch. 4.4 - Functions from derivatives Use the derivative f to...Ch. 4.4 - e e Prove that e e by first finding the maximum...Ch. 4.4 - Oscillations Consider the function f(x) = cos (ln...Ch. 4.4 - Local max/min of x1/x Use analytical methods to...Ch. 4.4 - Local max/min of xx Use analytical methods to find...Ch. 4.4 - Derivative information Suppose a continuous...Ch. 4.4 - Prob. 66ECh. 4.4 - Combining technology with analytical methods Use a...Ch. 4.4 - Combining technology with analytical methods Use a...Ch. 4.4 - Combining technology with analytical methods Use a...Ch. 4.4 - Special curves The following classical curves have...Ch. 4.4 - Special curves The following classical curves have...Ch. 4.4 - Special curves The following classical curves have...Ch. 4.4 - Special curves The following classical curves have...Ch. 4.4 - Special curves The following classical curves have...Ch. 4.4 - Prob. 78ECh. 4.4 - Prob. 79ECh. 4.5 - Verify that in the example to the right, the same...Ch. 4.5 - Find the objective function in Example 1 (in terms...Ch. 4.5 - Find the objective function in Example 2 (in terms...Ch. 4.5 - Fill in the blanks: The goal of an optimization...Ch. 4.5 - Prob. 2ECh. 4.5 - Suppose the objective function is Q = x2y and you...Ch. 4.5 - Suppose you wish to minimize a continuous...Ch. 4.5 - Suppose the objective function P = xy is subject...Ch. 4.5 - Suppose S=x+2y is an objective function subject to...Ch. 4.5 - Maximum product What two nonnegative real numbers...Ch. 4.5 - Sum of squares What two nonnegative real numbers a...Ch. 4.5 - Minimum sum What two positive real numbers whose...Ch. 4.5 - Maximum product Find numbers x and y satisfying...Ch. 4.5 - Maximum area rectangles Of all rectangles with a...Ch. 4.5 - Maximum area rectangles Of all rectangles with a...Ch. 4.5 - Minimum perimeter rectangles Of all rectangles of...Ch. 4.5 - Minimum perimeter rectangles Of all rectangles...Ch. 4.5 - Minimum sum Find positive numbers x and y...Ch. 4.5 - Pen problems a. A rectangular pen is built with...Ch. 4.5 - Rectangles beneath a semicircle A rectangle is...Ch. 4.5 - Rectangles beneath a parabola A rectangle is...Ch. 4.5 - Minimum-surface-area box Of all boxes with a...Ch. 4.5 - Maximum-volume box Suppose an airline policy...Ch. 4.5 - Shipping crates A square-based, box-shaped...Ch. 4.5 - Closest point on a line What point on the line y =...Ch. 4.5 - Closest point on a curve What point on the...Ch. 4.5 - Minimum distance Find the point P on the curve y =...Ch. 4.5 - Minimum distance Find the point P on the line y =...Ch. 4.5 - Walking and rowing A boat on the ocean is 4 mi...Ch. 4.5 - Laying cable An island is 3.5 mi from the nearest...Ch. 4.5 - Laying cable again Solve the problem in Exercise...Ch. 4.5 - Shortest ladder A 10-ft-tall fence runs parallel...Ch. 4.5 - Shortest laddermore realistic An 8-ft-tall fence...Ch. 4.5 - Circle and square A piece of wire of length 60 is...Ch. 4.5 - Maximum-volume cone A cone is constructed by...Ch. 4.5 - Prob. 34ECh. 4.5 - Optimal soda can a. Classical problem Find the...Ch. 4.5 - Covering a marble Imagine a flat-bottomed...Ch. 4.5 - Optimal garden A rectangular flower garden with an...Ch. 4.5 - Rectangles beneath a line a. A rectangle is...Ch. 4.5 - Designing a box Two squares of length x are cut...Ch. 4.5 - Folded boxes a. Squares with sides of length x are...Ch. 4.5 - A window consists of rectangular pane of glass...Ch. 4.5 - Light transmission A window consists of a...Ch. 4.5 - Keplers wine barrel Several mathematical stories...Ch. 4.5 - Blood testing Suppose a blood test for a disease...Ch. 4.5 - Maximum-volume cylinder in a sphere Find the...Ch. 4.5 - Maximizing profit Suppose you own a tour bus and...Ch. 4.5 - Cone in a cone A right circular cone is inscribed...Ch. 4.5 - Cylinder in a sphere Find the height h, radius r,...Ch. 4.5 - Travel costs A simple model for travel costs...Ch. 4.5 - Do dogs know calculus? A mathematician stands on a...Ch. 4.5 - Viewing angles An auditorium with a flat floor has...Ch. 4.5 - Suspension system A load must be suspended 6 m...Ch. 4.5 - Light sources The intensity of a light source at a...Ch. 4.5 - Basketball shot A basketball is shot with an...Ch. 4.5 - Fermats Principle a. Two poles of heights m and n...Ch. 4.5 - Prob. 56ECh. 4.5 - Making silos A grain silo consists of a...Ch. 4.5 - Prob. 58ECh. 4.5 - Minimizing related functions Complete each of the...Ch. 4.5 - Searchlight problemnarrow beam A searchlight is...Ch. 4.5 - Metal rain gutters A rain gutter is made from...Ch. 4.5 - Gliding mammals Many species of small mammals...Ch. 4.5 - Watching a Ferris wheel An observer stands 20 m...Ch. 4.5 - Crease-length problem A rectangular sheet of paper...Ch. 4.5 - Crankshaft A crank of radius r rotates with an...Ch. 4.5 - Maximum angle Find the value of x that maximizes ...Ch. 4.5 - Sum of isosceles distances a. An isosceles...Ch. 4.5 - Cylinder and cones (Putnam Exam 1938) Right...Ch. 4.5 - Slowest shortcut Suppose you are standing in a...Ch. 4.5 - Rectangles in triangles Find the dimensions and...Ch. 4.5 - Prob. 71ECh. 4.5 - Another pen problem A rancher is building a horse...Ch. 4.5 - Minimum-length roads A house is located at each...Ch. 4.5 - The arbelos An arbelos is the region enclosed by...Ch. 4.5 - Prob. 75ECh. 4.5 - Turning a corner with a pole a. What is the length...Ch. 4.5 - Tree notch (Putnam Exam 1938, rephrased) A notch...Ch. 4.5 - Prob. 78ECh. 4.5 - A challenging pen problem A farmer uses 200 meters...Ch. 4.5 - Prob. 80ECh. 4.6 - Sketch the graph of a function f that is concave...Ch. 4.6 - In Example 1, suppose you travel one mile in 75...Ch. 4.6 - Prob. 3QCCh. 4.6 - Prob. 4QCCh. 4.6 - Prob. 5QCCh. 4.6 - Sketch the graph of a smooth function f and label...Ch. 4.6 - Suppose you find the linear approximation to a...Ch. 4.6 - How is linear approximation used to approximate...Ch. 4.6 - How can linear approximation be used to...Ch. 4.6 - Suppose f is differentiable on (,),f(1)=2, and...Ch. 4.6 - Suppose f is differentiable on (,) and the...Ch. 4.6 - Linear approximation Estimate f(3.85) given that...Ch. 4.6 - Linear approximation Estimate f(5.1) given that...Ch. 4.6 - Given a function f that is differentiable on its...Ch. 4.6 - Does the differential dy represent the change in f...Ch. 4.6 - Suppose f is differentiable on (,),...Ch. 4.6 - Suppose f is differentiable on (,), f(5.99)=7 and...Ch. 4.6 - Estimating speed Use the linear approximation...Ch. 4.6 - Prob. 14ECh. 4.6 - Estimating time Suppose you want to travel D miles...Ch. 4.6 - Prob. 16ECh. 4.6 - Estimating time Suppose you want to travel D miles...Ch. 4.6 - Estimating time Suppose you want to travel D miles...Ch. 4.6 - Linear approximation Find the linear approximation...Ch. 4.6 - Linear approximation Find the linear approximation...Ch. 4.6 - Linear approximation Find the linear approximation...Ch. 4.6 - Linear approximation Find the linear approximation...Ch. 4.6 - Linear approximation Find the linear approximation...Ch. 4.6 - Linear approximation Find the linear approximation...Ch. 4.6 - Linear approximation a.Write the equation of the...Ch. 4.6 - Linear approximation a.Write the equation of the...Ch. 4.6 - Linear approximation a.Write the equation of the...Ch. 4.6 - Linear approximation a.Write the equation of the...Ch. 4.6 - Linear approximation a.Write the equation of the...Ch. 4.6 - Linear approximation a.Write the equation of the...Ch. 4.6 - Linear approximation a.Write the equation of the...Ch. 4.6 - Linear approximation a.Write the equation of the...Ch. 4.6 - Linear approximation a.Write the equation of the...Ch. 4.6 - Linear approximation a.Write the equation of the...Ch. 4.6 - Linear approximation a.Write the equation of the...Ch. 4.6 - Linear approximation a.Write the equation of the...Ch. 4.6 - Estimations with linear approximation Use linear...Ch. 4.6 - Estimations with linear approximation Use linear...Ch. 4.6 - Estimations with linear approximation Use linear...Ch. 4.6 - Estimations with linear approximation Use linear...Ch. 4.6 - Estimations with linear approximation Use linear...Ch. 4.6 - Estimations with linear approximation Use linear...Ch. 4.6 - Estimations with linear approximation Use linear...Ch. 4.6 - Estimations with linear approximation Use linear...Ch. 4.6 - Estimations with linear approximation Use linear...Ch. 4.6 - Prob. 46ECh. 4.6 - Linear approximation and concavity Carry out the...Ch. 4.6 - Linear approximation and concavity Carry out the...Ch. 4.6 - Prob. 49ECh. 4.6 - Linear approximation and concavity Carry out the...Ch. 4.6 - Prob. 51ECh. 4.6 - Ideal Gas Law The pressure P, temperature T, and...Ch. 4.6 - Explain why or why not Determine whether the...Ch. 4.6 - Prob. 54ECh. 4.6 - Approximating changes 35. Approximate the change...Ch. 4.6 - Approximating changes 36. Approximate the change...Ch. 4.6 - Approximating changes 37. Approximate the change...Ch. 4.6 - Approximating changes 38. Approximate the change...Ch. 4.6 - Approximating changes 39. Approximate the change...Ch. 4.6 - Approximating changes 40. Approximate the change...Ch. 4.6 - Differentials Consider the following functions and...Ch. 4.6 - Differentials Consider the following functions and...Ch. 4.6 - Differentials Consider the following functions and...Ch. 4.6 - Differentials Consider the following functions and...Ch. 4.6 - Differentials Consider the following functions and...Ch. 4.6 - Differentials Consider the following functions and...Ch. 4.6 - Differentials Consider the following functions and...Ch. 4.6 - Differentials Consider the following functions and...Ch. 4.6 - Differentials Consider the following functions and...Ch. 4.6 - Differentials Consider the following functions and...Ch. 4.6 - Prob. 71ECh. 4.6 - Errors in approximations Suppose f(x) = 1/(1 + x)...Ch. 4.6 - Prob. 73ECh. 4.7 - Which of the following functions lead to an...Ch. 4.7 - Prob. 2QCCh. 4.7 - What is the form of the limit limx/2(x/2)(tanx)?...Ch. 4.7 - Explain why a limit of the form 0 is not an...Ch. 4.7 - Before proceeding, use your intuition and rank...Ch. 4.7 - Compare the growth rates of f(x)=x2 and g(x)=x3 as...Ch. 4.7 - Explain with examples what is meant by the...Ch. 4.7 - Why are special methods, such as lHpitals Rule,...Ch. 4.7 - Explain the steps used to apply lHpitals Rule to a...Ch. 4.7 - Give examples of each of the following. a.A limit...Ch. 4.7 - Give examples of each of the following. a. A limit...Ch. 4.7 - Which of the following limits can be evaluated...Ch. 4.7 - Explain how to convert a limit of the form 0 to...Ch. 4.7 - Give an example of a limit of the form / as x 0.Ch. 4.7 - Prob. 9ECh. 4.7 - Evaluate limx2x33x2+2xx2 using lHpitals Rule and...Ch. 4.7 - Explain why the form 1 is indeterminate and cannot...Ch. 4.7 - Give the two-step method for attacking an...Ch. 4.7 - In terms of limits, what does it mean for f to...Ch. 4.7 - In terms of limits, what does it mean for the...Ch. 4.7 - Rank the functions x3, ln x, xx, and 2x in order...Ch. 4.7 - Rank the functions x100, ln x10, xx, and 10x in...Ch. 4.7 - 0/0 form Evaluate the following limits using...Ch. 4.7 - 0/0 form Evaluate the following limits using...Ch. 4.7 - Limits Evaluate the following limits. Use lHpitals...Ch. 4.7 - Limits Evaluate the following limits. Use lHpitals...Ch. 4.7 - 0/0 form Evaluate the following limits using...Ch. 4.7 - 0/0 form Evaluate the following limits using...Ch. 4.7 - Prob. 23ECh. 4.7 - / form Evaluate the following limits. 38....Ch. 4.7 - 0/0 form Evaluate the following limits using...Ch. 4.7 - 0/0 form Evaluate the following limits using...Ch. 4.7 - Limits Evaluate the following limits. Use lHpitals...Ch. 4.7 - Limits Evaluate the following limits. Use lHpitals...Ch. 4.7 - Prob. 29ECh. 4.7 - 0/0 form Evaluate the following limits using...Ch. 4.7 - 0/0 form Evaluate the following limits using...Ch. 4.7 - 0/0 form Evaluate the following limits using...Ch. 4.7 - 0/0 form Evaluate the following limits. 23....Ch. 4.7 - 0/0 form Evaluate the following limits. 24....Ch. 4.7 - 0/0 form Evaluate the following limits. 25....Ch. 4.7 - 0/0 form Evaluate the following limits. 26....Ch. 4.7 - / form Evaluate the following limits. 39....Ch. 4.7 - Prob. 38ECh. 4.7 - 0/0 form Evaluate the following limits. 27....Ch. 4.7 - 0/0 form Evaluate the following limits. 28....Ch. 4.7 - Prob. 41ECh. 4.7 - 0/0 form Evaluate the following limits. 30....Ch. 4.7 - 0/0 form Evaluate the following limits. 31....Ch. 4.7 - 0/0 form Evaluate the following limits. 32....Ch. 4.7 - / form Evaluate the following limits. 41....Ch. 4.7 - / form Evaluate the following limits. 42....Ch. 4.7 - 0/0 form Evaluate the following limits. 33....Ch. 4.7 - 0/0 form Evaluate the following limits. 34....Ch. 4.7 - 0/0 form Evaluate the following limits. 35....Ch. 4.7 - 0/0 form Evaluate the following limits. 36....Ch. 4.7 - Prob. 51ECh. 4.7 - Miscellaneous limits by any means Use analytical...Ch. 4.7 - 0 form Evaluate the following limits. 45....Ch. 4.7 - 0 form Evaluate the following limits. 46....Ch. 4.7 - 0 form Evaluate the following limits. 47....Ch. 4.7 - 0 form Evaluate the following limits. 48....Ch. 4.7 - 0 form Evaluate the following limits. 49....Ch. 4.7 - 0 form Evaluate the following limits. 50....Ch. 4.7 - Limits Evaluate the following limits. Use lHpitals...Ch. 4.7 - Limits Evaluate the following limits. Use lHpitals...Ch. 4.7 - form Evaluate the following limits. 51....Ch. 4.7 - form Evaluate the following limits. 53....Ch. 4.7 - Limits Evaluate the following limits. Use lHpitals...Ch. 4.7 - Prob. 64ECh. 4.7 - Miscellaneous limits by any means Use analytical...Ch. 4.7 - Miscellaneous limits by any means Use analytical...Ch. 4.7 - Miscellaneous limits by any means Use analytical...Ch. 4.7 - Miscellaneous limits by any means Use analytical...Ch. 4.7 - Miscellaneous limits by any means Use analytical...Ch. 4.7 - Miscellaneous limits by any means Use analytical...Ch. 4.7 - Miscellaneous limits by any means Use analytical...Ch. 4.7 - Miscellaneous limits by any means Use analytical...Ch. 4.7 - Miscellaneous limits by any means Use analytical...Ch. 4.7 - Miscellaneous limits by any means Use analytical...Ch. 4.7 - Limits Evaluate the following limits. Use lHpitals...Ch. 4.7 - Limits Evaluate the following limits. Use lHpitals...Ch. 4.7 - Limits Evaluate the following limits. Use lHpitals...Ch. 4.7 - Limits Evaluate the following limits. Use lHpitals...Ch. 4.7 - Limits Evaluate the following limits. Use lHpitals...Ch. 4.7 - Limits Evaluate the following limits. Use lHpitals...Ch. 4.7 - Limits Evaluate the following limits. Use lHpitals...Ch. 4.7 - Limits Evaluate the following limits. Use lHpitals...Ch. 4.7 - Limits Evaluate the following limits. Use lHpitals...Ch. 4.7 - An optics limit The theory of interference of...Ch. 4.7 - Compound interest Suppose you make a deposit of P...Ch. 4.7 - Two methods Evaluate the following limits in two...Ch. 4.7 - Two methods Evaluate the following limits in two...Ch. 4.7 - More limits Evaluate the following limits. 88....Ch. 4.7 - More limits Evaluate the following limits. 89....Ch. 4.7 - More limits Evaluate the following limits. 90....Ch. 4.7 - 88-94. More limits Evaluate the following...Ch. 4.7 - More limits Evaluate the following limits. 92....Ch. 4.7 - More limits Evaluate the following limits. 93....Ch. 4.7 - More limits Evaluate the following limits. 94....Ch. 4.7 - Comparing growth rates Use limit methods to...Ch. 4.7 - Comparing growth rates Use limit methods to...Ch. 4.7 - Comparing growth rates Use limit methods to...Ch. 4.7 - Comparing growth rates Use limit methods to...Ch. 4.7 - Comparing growth rates Use limit methods to...Ch. 4.7 - Comparing growth rates Use limit methods to...Ch. 4.7 - Comparing growth rates Use limit methods to...Ch. 4.7 - Prob. 102ECh. 4.7 - Comparing growth rates Use limit methods to...Ch. 4.7 - Prob. 104ECh. 4.7 - Explain why or why not Determine whether the...Ch. 4.7 - Graphing functions Make a complete graph of the...Ch. 4.7 - Graphing functions Make a complete graph of the...Ch. 4.7 - Graphing functions Make a complete graph of the...Ch. 4.7 - Graphing functions Make a complete graph of the...Ch. 4.7 - Algorithm complexity The complexity of a computer...Ch. 4.7 - LHpital loops Consider the limit limx0ax+bcx+d,...Ch. 4.7 - General result Let a and b be positive real...Ch. 4.7 - Exponential functions and powers Show that any...Ch. 4.7 - Exponentials with different bases Show that f(x) =...Ch. 4.7 - Logs with different bases Show that f(x) = loga x...Ch. 4.7 - Factorial growth rate The factorial function is...Ch. 4.7 - A geometric limit Let f() be the area of the...Ch. 4.7 - Prob. 118ECh. 4.7 - Exponentials vs. super exponentials Show that xx...Ch. 4.7 - Exponential growth rates a. For what values of b ...Ch. 4.8 - Verity that setting y = 0 in the equation...Ch. 4.8 - What happens if you apply Newtons method to the...Ch. 4.8 - Give a geometric explanation of Newtons method.Ch. 4.8 - Prob. 2ECh. 4.8 - A graph of f and the lines tangent to f at x = 1,...Ch. 4.8 - A graph of f and the lines tangent to f at x = 3,...Ch. 4.8 - Let f(x)=2x36x2+4x. Use Newtons method to find x1...Ch. 4.8 - The function f(x)=4xx2+4 is differentiable and has...Ch. 4.8 - How do you decide when to terminate Newtons...Ch. 4.8 - Give the formula for Newtons method for the...Ch. 4.8 - Formulating Newtons method Write the formula for...Ch. 4.8 - Formulating Newtons method Write the formula for...Ch. 4.8 - Formulating Newtons method Write the formula for...Ch. 4.8 - Formulating Newtons method Write the formula for...Ch. 4.8 - Finding roots with Newtons method For the given...Ch. 4.8 - Finding roots with Newtons method For the given...Ch. 4.8 - Finding roots with Newtons method For the given...Ch. 4.8 - Finding roots with Newtons method For the given...Ch. 4.8 - Finding roots with Newtons method For the given...Ch. 4.8 - Finding roots with Newtons method For the given...Ch. 4.8 - Finding roots with Newtons method For the given...Ch. 4.8 - More root finding Find all the roots of the...Ch. 4.8 - More root finding Find all the roots of the...Ch. 4.8 - More root finding Find all the roots of the...Ch. 4.8 - More root finding Find all the roots of the...Ch. 4.8 - More root finding Find all the roots of the...Ch. 4.8 - More root finding Find all the roots of the...Ch. 4.8 - More root finding Find all the roots of the...Ch. 4.8 - Finding intersection points Use Newtons method to...Ch. 4.8 - Prob. 28ECh. 4.8 - Finding intersection points Use Newtons method to...Ch. 4.8 - Prob. 30ECh. 4.8 - Finding intersection points Use Newtons method to...Ch. 4.8 - Prob. 32ECh. 4.8 - Prob. 33ECh. 4.8 - Prob. 34ECh. 4.8 - Prob. 35ECh. 4.8 - Investment problem A one-time investment of 2500...Ch. 4.8 - Applications 45. A damped oscillator The...Ch. 4.8 - The sinc function The sinc function, sinc(x)=sinxx...Ch. 4.8 - Estimating roots The values of various roots can...Ch. 4.8 - Prob. 40ECh. 4.8 - Prob. 41ECh. 4.8 - Prob. 42ECh. 4.8 - Prob. 43ECh. 4.8 - Newtons method and curve sketching Use Newtons...Ch. 4.8 - Newtons method and curve sketching Use Newtons...Ch. 4.8 - Prob. 46ECh. 4.8 - Prob. 47ECh. 4.8 - Fixed points An important question about many...Ch. 4.8 - Fixed points An important question about many...Ch. 4.8 - Fixed points An important question about many...Ch. 4.8 - Fixed points An important question about many...Ch. 4.8 - Pitfalls of Newtons method Let f(x)=x1+x2, which...Ch. 4.8 - Prob. 53ECh. 4.8 - Approximating square roots Let a 0 be given and...Ch. 4.8 - Prob. 55ECh. 4.8 - Prob. 56ECh. 4.8 - An eigenvalue problem A certain kind of...Ch. 4.8 - Prob. 58ECh. 4.8 - Prob. 59ECh. 4.8 - Prob. 60ECh. 4.9 - Verify by differentiation that x4 is an...Ch. 4.9 - Find the family of antiderivatives for each of...Ch. 4.9 - Use differentiation to verify result 6 in Table...Ch. 4.9 - Prob. 4QCCh. 4.9 - Position is an antiderivative of velocity. But...Ch. 4.9 - Fill in the blanks with either of the words the...Ch. 4.9 - Describe the set of antiderivatives of f(x) = 0.Ch. 4.9 - Describe the set of antiderivatives of f(x) = 1.Ch. 4.9 - Why do two different antiderivatives of a function...Ch. 4.9 - Give the antiderivatives of xp. For what values of...Ch. 4.9 - Give the antiderivatives of a/1x2, where a is a...Ch. 4.9 - Give the antiderivatives of 1/x.Ch. 4.9 - Evaluate acosxdxand asinxdx, where a is a...Ch. 4.9 - If F(x) = x2 3x + C and F(1) = 4, what is the...Ch. 4.9 - For a given function f, explain the steps used to...Ch. 4.9 - Finding antiderivatives Find all the...Ch. 4.9 - Finding antiderivatives Find all the...Ch. 4.9 - Finding antiderivatives Find all the...Ch. 4.9 - Finding antiderivatives Find all the...Ch. 4.9 - Finding antiderivatives Find all the...Ch. 4.9 - Finding antiderivatives Find all the...Ch. 4.9 - Finding antiderivatives Find all the...Ch. 4.9 - Finding antiderivatives Find all the...Ch. 4.9 - Finding antiderivatives Find all the...Ch. 4.9 - Finding antiderivatives Find all the...Ch. 4.9 - Prob. 21ECh. 4.9 - Finding antiderivatives Find all the...Ch. 4.9 - Indefinite integrals Determine the following...Ch. 4.9 - Indefinite integrals Determine the following...Ch. 4.9 - Indefinite integrals Determine the following...Ch. 4.9 - Indefinite integrals Determine the following...Ch. 4.9 - Indefinite integrals Determine the following...Ch. 4.9 - Indefinite integrals Determine the following...Ch. 4.9 - Indefinite integrals Determine the following...Ch. 4.9 - Indefinite integrals Determine the following...Ch. 4.9 - Indefinite integrals Determine the following...Ch. 4.9 - Indefinite integrals Determine the following...Ch. 4.9 - Indefinite integrals Determine the following...Ch. 4.9 - Indefinite integrals Determine the following...Ch. 4.9 - Indefinite integrals Determine the following...Ch. 4.9 - Indefinite integrals Determine the following...Ch. 4.9 - Indefinite integrals Determine the following...Ch. 4.9 - Indefinite integrals Determine the following...Ch. 4.9 - Indefinite integrals Determine the following...Ch. 4.9 - Indefinite integrals Determine the following...Ch. 4.9 - Indefinite integrals Determine the following...Ch. 4.9 - Indefinite integrals Determine the following...Ch. 4.9 - Indefinite integrals Determine the following...Ch. 4.9 - Indefinite integrals Determine the following...Ch. 4.9 - Indefinite integrals involving trigonometric...Ch. 4.9 - Indefinite integrals Determine the following...Ch. 4.9 - Indefinite integrals involving trigonometric...Ch. 4.9 - Indefinite integrals Determine the following...Ch. 4.9 - Indefinite integrals Determine the following...Ch. 4.9 - Indefinite integrals Determine the following...Ch. 4.9 - Other indefinite integrate Determine the following...Ch. 4.9 - Indefinite integrals Determine the following...Ch. 4.9 - Indefinite integrals Determine the following...Ch. 4.9 - Indefinite integrals Determine the following...Ch. 4.9 - Indefinite integrals Determine the following...Ch. 4.9 - Indefinite integrals Determine the following...Ch. 4.9 - Indefinite integrals Determine the following...Ch. 4.9 - Indefinite integrals Determine the following...Ch. 4.9 - Other indefinite integrate Determine the following...Ch. 4.9 - Indefinite integrals Determine the following...Ch. 4.9 - Other indefinite integrate Determine the following...Ch. 4.9 - Other indefinite integrate Determine the following...Ch. 4.9 - Indefinite integrals Determine the following...Ch. 4.9 - Miscellaneous indefinite integrals Determine the...Ch. 4.9 - Miscellaneous indefinite integrals Determine the...Ch. 4.9 - Indefinite integrals Determine the following...Ch. 4.9 - Indefinite integrals Determine the following...Ch. 4.9 - Miscellaneous indefinite integrals Determine the...Ch. 4.9 - Particular antiderivatives For the following...Ch. 4.9 - Particular antiderivatives For the following...Ch. 4.9 - Particular antiderivatives For the following...Ch. 4.9 - Particular antiderivatives For the following...Ch. 4.9 - Particular antiderivatives For the following...Ch. 4.9 - Particular antiderivatives For the following...Ch. 4.9 - Particular antiderivatives For the following...Ch. 4.9 - Particular antiderivatives For the following...Ch. 4.9 - Solving initial value problems Find the solution...Ch. 4.9 - Solving initial value problems Find the solution...Ch. 4.9 - Solving initial value problems Find the solution...Ch. 4.9 - Solving initial value problems Find the solution...Ch. 4.9 - Solving initial value problems Find the solution...Ch. 4.9 - Solving initial value problems Find the solution...Ch. 4.9 - Solving initial value problems Find the solution...Ch. 4.9 - Solving initial value problems Find the solution...Ch. 4.9 - Solving initial value problems Find the solution...Ch. 4.9 - Solving initial value problems Find the solution...Ch. 4.9 - Graphing general solutions Graph several functions...Ch. 4.9 - Prob. 88ECh. 4.9 - Graphing general solutions Graph several functions...Ch. 4.9 - Graphing general solutions Graph several functions...Ch. 4.9 - Velocity to position Given the following velocity...Ch. 4.9 - Velocity to position Given the following velocity...Ch. 4.9 - Velocity to position Given the following velocity...Ch. 4.9 - Velocity to position Given the following velocity...Ch. 4.9 - Velocity to position Given the following velocity...Ch. 4.9 - Velocity to position Given the following velocity...Ch. 4.9 - Acceleration to position Given the following...Ch. 4.9 - Acceleration to position Given the following...Ch. 4.9 - Acceleration to position Given the following...Ch. 4.9 - Acceleration to position Given the following...Ch. 4.9 - Acceleration to position Given the following...Ch. 4.9 - Prob. 102ECh. 4.9 - A car starting at rest accelerates at 16 ft/s2-for...Ch. 4.9 - Prob. 104ECh. 4.9 - Races The velocity function and initial position...Ch. 4.9 - Prob. 106ECh. 4.9 - Motion with gravity Consider the following...Ch. 4.9 - Motion with gravity Consider the following...Ch. 4.9 - Motion with gravity Consider the following...Ch. 4.9 - Motion with gravity Consider the following...Ch. 4.9 - Explain why or why not Determine whether the...Ch. 4.9 - Prob. 112ECh. 4.9 - Functions from higher derivatives Find the...Ch. 4.9 - Functions from higher derivatives Find the...Ch. 4.9 - Prob. 115ECh. 4.9 - Prob. 116ECh. 4.9 - How rate A large tank is filled with water when an...Ch. 4.9 - Prob. 118ECh. 4.9 - Verifying indefinite integrals Verify the...Ch. 4.9 - Prob. 120ECh. 4.9 - Prob. 121ECh. 4.9 - Prob. 122ECh. 4 - Explain why or why not Determine whether the...Ch. 4 - Locating extrema Consider the graph of a function...Ch. 4 - Designer functions Sketch the graph of a function...Ch. 4 - Designer functions Sketch the graph of a function...Ch. 4 - Use the graphs of f and f to complete the...Ch. 4 - Critical points Find the critical points of the...Ch. 4 - Critical points Find the critical points of the...Ch. 4 - Critical points Find the critical points of the...Ch. 4 - Critical points Find the critical points of the...Ch. 4 - Critical points Find the critical points of the...Ch. 4 - Critical points Find the critical points of the...Ch. 4 - Critical points Find the critical points of the...Ch. 4 - Critical points Find the critical points of the...Ch. 4 - Critical points Find the critical points of the...Ch. 4 - Critical points Find the critical points of the...Ch. 4 - Critical points Find the critical points of the...Ch. 4 - Absolute values Consider the function f(x) = |x ...Ch. 4 - Use f and f to complete parts (a) and (b). a. Find...Ch. 4 - Use f and f to complete parts (a) and (b). a.Find...Ch. 4 - Use f and f to complete parts (a) and (b). a.Find...Ch. 4 - Inflection points Does f(x) = 2x5 10x4 + 20x3 + x...Ch. 4 - Does f(x)=x62+5x4415x2 have any inflection points?...Ch. 4 - Identify the critical points and the inflection...Ch. 4 - Curve sketching Use the guidelines given in...Ch. 4 - Curve sketching Use the guidelines given in...Ch. 4 - Curve sketching Use the guidelines given in...Ch. 4 - Curve sketching Use the guidelines given in...Ch. 4 - Curve sketching Use the guidelines given in...Ch. 4 - Curve sketching Use the guidelines given in...Ch. 4 - Curve sketching Use the guidelines given in...Ch. 4 - Curve sketching Use the guidelines given in...Ch. 4 - Prob. 32RECh. 4 - Curve sketching Use the guidelines given in...Ch. 4 - Prob. 34RECh. 4 - Optimal popcorn box A small popcorn box is created...Ch. 4 - Minimizing time Hannah is standing on the edge of...Ch. 4 - Minimizing sound intensity Two sound speakers are...Ch. 4 - Hockey problem A hockey player skates on a line...Ch. 4 - Optimization A right triangle has legs of length h...Ch. 4 - T 22. Rectangles beneath a curve A rectangle is...Ch. 4 - Maximum printable area A rectangular page in a...Ch. 4 - Nearest point What point on the graph of...Ch. 4 - Maximum area A line segment of length 10 joins the...Ch. 4 - Minimum painting surface A metal cistern in the...Ch. 4 - Linear approximation a. Find the linear...Ch. 4 - Linear approximation a. Find the linear...Ch. 4 - Estimations with linear approximation Use linear...Ch. 4 - Estimations with linear approximation Use linear...Ch. 4 - Change in elevation The elevation h (in feet above...Ch. 4 - Change in energy The energy E (in joules) released...Ch. 4 - Mean Value Theorem For the function f(x)=10x and...Ch. 4 - Mean Value Theorem Explain why the Mean Value...Ch. 4 - Mean Value Theorem The population of a culture of...Ch. 4 - Growth rate of bamboo Bamboo belongs to the grass...Ch. 4 - Newtons method Use Newtons method to approximate...Ch. 4 - Prob. 56RECh. 4 - Newtons method Use Newtons method to approximate...Ch. 4 - Two methods Evaluate the following limits in two...Ch. 4 - Two methods Evaluate the following limits in two...Ch. 4 - Limits Evaluate the following limits. Use lHpitals...Ch. 4 - Limits Evaluate the following limits. Use lHpitals...Ch. 4 - Limits Evaluate the following limits. Use lHpitals...Ch. 4 - Limits Evaluate the following limits. Use lHpitals...Ch. 4 - Limits Evaluate the following limits. Use lHpitals...Ch. 4 - Limits Evaluate the following limits. Use lHpitals...Ch. 4 - Limits Evaluate the following limits. Use lHpitals...Ch. 4 - Limits Evaluate the following limits. Use lHpitals...Ch. 4 - Prob. 68RECh. 4 - Prob. 69RECh. 4 - Limits Evaluate the following limits. Use lHpitals...Ch. 4 - Limits Evaluate the following limits. Use lHpitals...Ch. 4 - Limits Evaluate the following limits. Use lHpitals...Ch. 4 - Prob. 73RECh. 4 - Limits Evaluate the following limits. Use lHpitals...Ch. 4 - Limits Evaluate the following limits. Use lHpitals...Ch. 4 - Limits Evaluate the following limits. Use lHpitals...Ch. 4 - Limits Evaluate the following limits. Use lHpitals...Ch. 4 - Prob. 78RECh. 4 - Prob. 79RECh. 4 - Prob. 80RECh. 4 - Limits Evaluate the following limits. Use lHpitals...Ch. 4 - Comparing growth rates Determine which of the two...Ch. 4 - Prob. 83RECh. 4 - Prob. 84RECh. 4 - Comparing growth rates Determine which of the two...Ch. 4 - Comparing growth rates Determine which of the two...Ch. 4 - Prob. 87RECh. 4 - Comparing growth rates Determine which of the two...Ch. 4 - Comparing growth rates Determine which of the two...Ch. 4 - Indefinite integrals Determine the following...Ch. 4 - Indefinite integrals Determine the following...Ch. 4 - Indefinite integrals Determine the following...Ch. 4 - Indefinite integrals Determine the following...Ch. 4 - Indefinite integrals Determine the following...Ch. 4 - Indefinite integrals Determine the following...Ch. 4 - Indefinite integrals Determine the following...Ch. 4 - Prob. 97RECh. 4 - Indefinite integrals Determine the following...Ch. 4 - Prob. 99RECh. 4 - Indefinite integrals Determine the following...Ch. 4 - Prob. 101RECh. 4 - Prob. 102RECh. 4 - Prob. 103RECh. 4 - Prob. 104RECh. 4 - Prob. 105RECh. 4 - Functions from derivatives Find the function f...Ch. 4 - Functions from derivatives Find the function f...Ch. 4 - Prob. 108RECh. 4 - Prob. 109RECh. 4 - Distance traveled A car starting at rest...Ch. 4 - Prob. 111RECh. 4 - Logs of logs Compare the growth rates of ln x, ln...Ch. 4 - Prob. 113RECh. 4 - Prob. 114RECh. 4 - Prob. 115RECh. 4 - Prob. 116RECh. 4 - Prob. 117RECh. 4 - A family of super-exponential functions Let f(x) =...
Additional Math Textbook Solutions
Find more solutions based on key concepts
1. How many solutions are there to ax + b = 0 with ?
College Algebra with Modeling & Visualization (5th Edition)
CLT Shapes (Example 4) One of the histograms is a histogram of a sample (from a population with a skewed distri...
Introductory Statistics
The following set of data is from sample of n=5: a. Compute the mean, median, and mode. b. Compute the range, v...
Basic Business Statistics, Student Value Edition
The table by using the given graph of h.
Calculus for Business, Economics, Life Sciences, and Social Sciences (14th Edition)
To arrange the set of rational numbers from least to greatest. −11314,−1.9,−1911,−1.95.
Pre-Algebra Student Edition
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, calculus and related others by exploring similar questions and additional content below.Similar questions
- The circular blade on a saw has a radius of 4 inches and it rotates at 2400 revolutions per minute. a. Find the angular speed of the blade in radians per minute. b. Find the linear speed of the edge of the blade.arrow_forwardCycling When a cyclist pedals. he turns a gear, called a chainring. The angular velocity of the chainring will determine the linear speed at which the chain travels. The chain connects the chainring to a smaller gear, called a sprocket, winch is attached to the rear wheel (Figure 11). The angular velocity of the sprocket depends upon the linear speed of the chain. The sprocket and rear wheel rotate at the same rate, and the diameter of the rear wheel is 700 millimeters. The speed at which the cyclist travels is determined by the angular velocity of his rear wheel. Use this information to answer Problems 63 through 67. If a cyclist was using his 210-millimeter-diameter chainring and pedaling at a rate of 85 revolutions per minute, what diameter sprocket would he need in order to maintain a speed of 45 kilometers per hour?arrow_forwardCycling When a cyclist pedals. he turns a gear, called a chainring. The angular velocity of the chainring will determine the linear speed at which the chain travels. The chain connects the chainring to a smaller gear, called a sprocket, winch is attached to the rear wheel (Figure 11). The angular velocity of the sprocket depends upon the linear speed of the chain. The sprocket and rear wheel rotate at the same rate, and the diameter of the rear wheel is 700 millimeters. The speed at which the cyclist travels is determined by the angular velocity of his rear wheel. Use this information to answer Problems 63 through 67. Suppose a cyclist was using a 150-millimeter-diameter chainring and an 80-millimeter-diameter sprocket. How fast would she need to pedal, in revolutions per minute, in order to maintain a speed of 20 kilometers per hour?arrow_forward
- Cycling When a cyclist pedals. he turns a gear, called a chainring. The angular velocity of the chainring will determine the linear speed at which the chain travels. The chain connects the chainring to a smaller gear, called a sprocket, winch is attached to the rear wheel (Figure 11). The angular velocity of the sprocket depends upon the linear speed of the chain. The sprocket and rear wheel rotate at the same rate, and the diameter of the rear wheel is 700 millimeters. The speed at which the cyclist travels is determined by the angular velocity of his rear wheel. Use this information to answer Problems 63 through 67. A pro cyclist is climbing Mount Ventoux, equipped with a 150-millimeter-diameter chainring and a 95-millimeter-diameter sprocket. If he was pedaling at a rate of 90 revolutions per minute, find his speed in kilometers per hour. (1km=1,000,000mm)arrow_forwardCycling When a cyclist pedals. he turns a gear, called a chainring. The angular velocity of the chainring will determine the linear speed at which the chain travels. The chain connects the chainring to a smaller gear, called a sprocket, winch is attached to the rear wheel (Figure 11). The angular velocity of the sprocket depends upon the linear speed of the chain. The sprocket and rear wheel rotate at the same rate, and the diameter of the rear wheel is 700 millimeters. The speed at which the cyclist travels is determined by the angular velocity of his rear wheel. Use this information to answer Problems 63 through 67. On level ground, a pro cyclist would use a larger chainring and a smaller sprocket. If he shifted to a 210-millimeter-diameter chainring and a 40-millimeter-diameter sprocket, how fast would he be traveling in kilometers per hour if he pedaled at a rate of 80 revolutions per minute?arrow_forwardFan A ceiling fan with 16-in. blades rotates at 45rpm. a Find the angular speed of the fan in rad/min. b Find the linear speed of the tips of the blades in in./min.arrow_forward
- Fill in the blanks. The ________ speed of a particle is the ratio of the change in the central angle to the elapsed time.arrow_forwardLinear and Angular Speed A Blu-ray disc is approximately 12 centimeters in diameter. The drive motor of a Blu-ray player is able to rotate up to 10,000 revolutions per minute. (a) Find the maximum angular speed (in radians per second) of a Blu-ray disc as it rotates. (b) Find the maximum linear speed (in meters per second) of a point on the outermost track as the disc rotates.arrow_forwardAngular Speed and Linear Speed A potters wheel with radius 8 in. spins at 150rpm. Find the angular and linear speeds of a point on the rim of the wheel.arrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning
Trigonometry (MindTap Course List)TrigonometryISBN:9781305652224Author:Charles P. McKeague, Mark D. TurnerPublisher:Cengage Learning
Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
Algebra and Trigonometry (MindTap Course List)AlgebraISBN:9781305071742Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
Trigonometry (MindTap Course List)
Trigonometry
ISBN:9781337278461
Author:Ron Larson
Publisher:Cengage Learning
Trigonometry (MindTap Course List)
Trigonometry
ISBN:9781305652224
Author:Charles P. McKeague, Mark D. Turner
Publisher:Cengage Learning
Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:9781133382119
Author:Swokowski
Publisher:Cengage
Algebra and Trigonometry (MindTap Course List)
Algebra
ISBN:9781305071742
Author:James Stewart, Lothar Redlin, Saleem Watson
Publisher:Cengage Learning
The Law of Cosines; Author: Professor Dave Explains;https://www.youtube.com/watch?v=3wGQMyaWoLA;License: Standard YouTube License, CC-BY
Law of Sines and Law of Cosines (4 Examples); Author: Mario's Math Tutoring;https://www.youtube.com/watch?v=T--nPHdS1Vo;License: Standard YouTube License, CC-BY