Finite Mathematics for Business, Economics, Life Sciences, and Social Sciences (14th Edition)
Finite Mathematics for Business, Economics, Life Sciences, and Social Sciences (14th Edition)
14th Edition
ISBN: 9780134675985
Author: Raymond A. Barnett, Michael R. Ziegler, Karl E. Byleen, Christopher J. Stocker
Publisher: PEARSON
bartleby

Videos

Textbook Question
Book Icon
Chapter 4.3, Problem 83E

Nutrition. A dietitian in a hospital is to arrange a special diet composed of three basic foods. The diet is to include exactly 340 units of calcium, 180 units of iron, and 220 units of vitamin A. The number of units per ounce of each special ingredient for each of the foods is indicated in the table.

Chapter 4.3, Problem 83E, Nutrition. A dietitian in a hospital is to arrange a special diet composed of three basic foods. The

(A) How many ounces of each food must be used to meet the diet requirements?

(B) How is the diet in part (A) affected if food C is not used?

(C) How is the diet in part (A) affected if the vitamin A requirement is dropped?

Expert Solution & Answer
Check Mark

Want to see the full answer?

Check out a sample textbook solution
Blurred answer
Previous
Chapter 4.3, Problem 82E
Next
Chapter 4.3, Problem 84E
Students have asked these similar questions
5. [10 marks] Let G = (V,E) be a graph, and let X C V be a set of vertices. Prove that if |S||N(S)\X for every SCX, then G contains a matching M that matches every vertex of X (i.e., such that every x X is an end of an edge in M).
Q/show that 2" +4 has a removable discontinuity at Z=2i Z(≥2-21)
Refer to page 100 for problems on graph theory and linear algebra. Instructions: • Analyze the adjacency matrix of a given graph to find its eigenvalues and eigenvectors. • Interpret the eigenvalues in the context of graph properties like connectivity or clustering. Discuss applications of spectral graph theory in network analysis. Link: [https://drive.google.com/file/d/1wKSrun-GlxirS3IZ9qoHazb9tC440 AZF/view?usp=sharing]

Chapter 4 Solutions

Finite Mathematics for Business, Economics, Life Sciences, and Social Sciences (14th Edition)

Ch. 4.1 - Can a consistent and dependent system have exactly...Ch. 4.1 - Return to Example 2 and solve each system by...Ch. 4.1 - Solve by graphing and check: 2xy=3x+2y=4Ch. 4.1 - Solve each of the following systems by graphing:...Ch. 4.1 - Solve to two decimal places using graphical...Ch. 4.1 - Solve by substitution: 3x3y=22xy=6Ch. 4.1 - Solve the following system using elimination by...Ch. 4.1 - Dennis wants to use cottage cheese and yogurt to...Ch. 4.1 - Find the equilibrium quantity and equilibrium...Ch. 4.1 - In Problems 1-6, find the x and y coordinates of...
Ch. 4.1 - In Problems 1-6, find the x and y coordinates of...Ch. 4.1 - In Problems 1-6, find the x and y coordinates of...Ch. 4.1 - In Problems 1-6, find the x and y coordinates of...Ch. 4.1 - In Problems 1-6, find the x and y coordinates of...Ch. 4.1 - In Problems 1-6, find the x and y coordinates of...Ch. 4.1 - In Problems 7 and 8, find an equation in...Ch. 4.1 - In Problems 7 and 8, find an equation in...Ch. 4.1 - Match each system in Problems 9-12 with one of the...Ch. 4.1 - Match each system in Problems 9-12 with one of the...Ch. 4.1 - Match each system in Problems 9-12 with one of the...Ch. 4.1 - Match each system in Problems 9-12 with one of the...Ch. 4.1 - Solve Problem 13-16 by graphing. 3xy=2x+2y=10Ch. 4.1 - Solve Problem 13-16 by graphing. 3x2y=127x+2y=8Ch. 4.1 - Solve Problem 13-16 by graphing. m+2n=42m+4n=8Ch. 4.1 - Solve Problem 13-16 by graphing. 3u+5v=156u+10v=30Ch. 4.1 - Solve Problems 17-20 using substitution....Ch. 4.1 - Solve Problems 17-20 using substitution....Ch. 4.1 - Solve Problems 17-20 using substitution....Ch. 4.1 - Solve Problems 17-20 using substitution....Ch. 4.1 - Solve Problems 21-24 using elimination by...Ch. 4.1 - Solve Problems 21-24 using elimination by...Ch. 4.1 - Solve Problems 21-24 using elimination by...Ch. 4.1 - Solve Problems 21-24 using elimination by...Ch. 4.1 - Solve Problems 25-34 using substitution or...Ch. 4.1 - Solve Problems 25-34 using substitution or...Ch. 4.1 - Solve Problems 25-34 using substitution or...Ch. 4.1 - Prob. 28ECh. 4.1 - Solve Problems 25-34 using substitution or...Ch. 4.1 - Solve Problems 25-34 using substitution or...Ch. 4.1 - Solve Problems 25-34 using substitution or...Ch. 4.1 - Solve Problems 25-34 using substitution or...Ch. 4.1 - Solve Problems 25-34 using substitution or...Ch. 4.1 - Solve Problems 25-34 using substitution or...Ch. 4.1 - In Problems 35-42, solve the system. Note that...Ch. 4.1 - In Problems 35-42, solve the system. Note that...Ch. 4.1 - In Problems 35-42, solve the system. Note that...Ch. 4.1 - In Problems 35-42, solve the system. Note that...Ch. 4.1 - In Problems 35-42, solve the system. Note that...Ch. 4.1 - In Problems 35-42, solve the system. Note that...Ch. 4.1 - In Problems 35-42, solve the system. Note that...Ch. 4.1 - In Problems 35-42, solve the system. Note that...Ch. 4.1 - In a free competitive market, if the supply of a...Ch. 4.1 - In a free competitive market, if the demand for a...Ch. 4.1 - Problems 45-48 are concerned with the linear...Ch. 4.1 - Problems 45-48 are concerned with the linear...Ch. 4.1 - Problems 45-48 are concerned with the linear...Ch. 4.1 - Problems 45-48 are concerned with the linear...Ch. 4.1 - In Problems 49-56, use a graphing calculator to...Ch. 4.1 - In Problems 49-56, use a graphing calculator to...Ch. 4.1 - In Problems 49-56, use a graphing calculator to...Ch. 4.1 - In Problems 49-56, use a graphing calculator to...Ch. 4.1 - In Problems 49-56, use a graphing calculator to...Ch. 4.1 - In Problems 49-56, use a graphing calculator to...Ch. 4.1 - In Problems 49-56, use a graphing calculator to...Ch. 4.1 - In Problems 49-56, use a graphing calculator to...Ch. 4.1 - In Problems 57-62, graph the equations in the same...Ch. 4.1 - In Problems 57-62, graph the equations in the same...Ch. 4.1 - In Problems 57-62, graph the equations in the same...Ch. 4.1 - In Problems 57-62, graph the equations in the same...Ch. 4.1 - In Problems 57-62, graph the equations in the same...Ch. 4.1 - In Problems 57-62, graph the equations in the same...Ch. 4.1 - The coefficients of the three systems given below...Ch. 4.1 - Repeat Problem 63 for the following systems:...Ch. 4.1 - Supply and demand for T-shirts. Suppose that the...Ch. 4.1 - Supply and demand for baseball caps. Suppose that...Ch. 4.1 - Supply and demand for soybeans. At $4.80 per...Ch. 4.1 - Supply and demand for corn. At $2.13 per bushel,...Ch. 4.1 - Break-even analysis. A small plant manufactures...Ch. 4.1 - Break-even analysis. Repeat Problem 69 with the...Ch. 4.1 - Break-even analysis. A company markets exercise...Ch. 4.1 - Break-even analysis. Repeat Problem 71 if the...Ch. 4.1 - Delivery charges. United Express, a national...Ch. 4.1 - Delivery charges. Refer to Problem 73. Federated...Ch. 4.1 - Coffee blends. A coffee company uses Colombian and...Ch. 4.1 - Coffee blends. Refer to Problem 75. (A) If the...Ch. 4.1 - Animal diet. Animals in an experiment are to be...Ch. 4.1 - Fertilizer. A fruit grower uses two types of...Ch. 4.1 - Electronics. A supplier for the electronics...Ch. 4.1 - Sausage. A company produces Italian sausages and...Ch. 4.1 - Physics. An object dropped off the top of a tall...Ch. 4.1 - Physics. Repeat Problem 81 if the object is 240...Ch. 4.1 - Earthquakes. An earthquake emits a primary wave...Ch. 4.1 - Sound waves. A ship using sound-sensing devices...Ch. 4.1 - Psychology. People approach certain situations...Ch. 4.2 - The summary following the solution of Example 1...Ch. 4.2 - The solution of Example 3 involved three augmented...Ch. 4.2 - Solve using augmented matrix methods: 2x1x2=7Ch. 4.2 - Solve using augmented matrix methods:...Ch. 4.2 - Solve using augmented matrix methods:...Ch. 4.2 - Solve using augmented matrix methods:...Ch. 4.2 - Problems 1-14 refer to the following matrices: (If...Ch. 4.2 - Problems 1-14 refer to the following matrices: (If...Ch. 4.2 - Problems 1-14 refer to the following matrices: (If...Ch. 4.2 - Problems 1-14 refer to the following matrices: (If...Ch. 4.2 - Problems 1-14 refer to the following matrices: (If...Ch. 4.2 - Problems 1-14 refer to the following matrices: (If...Ch. 4.2 - Problems 1-14 refer to the following matrices: (If...Ch. 4.2 - Problems 1-14 refer to the following matrices: (If...Ch. 4.2 - Problems 1-14 refer to the following matrices: (If...Ch. 4.2 - Problems 1-14 refer to the following matrices: (If...Ch. 4.2 - Problems 1-14 refer to the following matrices: (If...Ch. 4.2 - Problems 1-14 refer to the following matrices: (If...Ch. 4.2 - Problems 1-14 refer to the following matrices: (If...Ch. 4.2 - Problems 1-14 refer to the following matrices: (If...Ch. 4.2 - In Problems 15-18, write the coefficient matrix...Ch. 4.2 - In Problems 15-18, write the coefficient matrix...Ch. 4.2 - In Problems 15-18, write the coefficient matrix...Ch. 4.2 - In Problems 15-18, write the coefficient matrix...Ch. 4.2 - In Problems 19-22, write the system of linear...Ch. 4.2 - In Problems 19-22, write the system of linear...Ch. 4.2 - In Problems 19-22, write the system of linear...Ch. 4.2 - In Problems 19-22, write the system of linear...Ch. 4.2 - Perform the row operations indicated in Problems...Ch. 4.2 - Perform the row operations indicated in Problems...Ch. 4.2 - Perform the row operations indicated in Problems...Ch. 4.2 - Perform the row operations indicated in Problems...Ch. 4.2 - Perform the row operations indicated in Problems...Ch. 4.2 - Perform the row operations indicated in Problems...Ch. 4.2 - Perform the row operations indicated in Problems...Ch. 4.2 - Perform the row operations indicated in Problems...Ch. 4.2 - Perform the row operations indicated in Problems...Ch. 4.2 - Perform the row operations indicated in Problems...Ch. 4.2 - Perform the row operations indicated in Problems...Ch. 4.2 - Perform the row operations indicated in Problems...Ch. 4.2 - Each of the matrices in Problems 35-42 is the...Ch. 4.2 - Each of the matrices in Problems 35-42 is the...Ch. 4.2 - Each of the matrices in Problems 35-42 is the...Ch. 4.2 - Each of the matrices in Problems 35-42 is the...Ch. 4.2 - Each of the matrices in Problems 35-42 is the...Ch. 4.2 - Each of the matrices in Problems 35-42 is the...Ch. 4.2 - Each of the matrices in Problems 35-42 is the...Ch. 4.2 - Each of the matrices in Problems 35-42 is the...Ch. 4.2 - Solve Problems 43-46 using augmented matrix...Ch. 4.2 - Solve Problems 43-46 using augmented matrix...Ch. 4.2 - Solve Problems 43-46 using augmented matrix...Ch. 4.2 - Solve Problems 43-46 using augmented matrix...Ch. 4.2 - Solve Problems 47 and 48 using augmented matrix...Ch. 4.2 - Solve Problems 47 and 48 using augmented matrix...Ch. 4.2 - Each of the matrices in Problems 49-54 is the...Ch. 4.2 - Each of the matrices in Problems 49-54 is the...Ch. 4.2 - Each of the matrices in Problems 49-54 is the...Ch. 4.2 - Each of the matrices in Problems 49-54 is the...Ch. 4.2 - Each of the matrices in Problems 49-54 is the...Ch. 4.2 - Each of the matrices in Problems 49-54 is the...Ch. 4.2 - Solve Problems 55-74 using augmented matrix...Ch. 4.2 - Solve Problems 55-74 using augmented matrix...Ch. 4.2 - Solve Problems 55-74 using augmented matrix...Ch. 4.2 - Solve Problems 55-74 using augmented matrix...Ch. 4.2 - Solve Problems 55-74 using augmented matrix...Ch. 4.2 - Solve Problems 55-74 using augmented matrix...Ch. 4.2 - Solve Problems 55-74 using augmented matrix...Ch. 4.2 - Solve Problems 55-74 using augmented matrix...Ch. 4.2 - Solve Problems 55-74 using augmented matrix...Ch. 4.2 - Solve Problems 55-74 using augmented matrix...Ch. 4.2 - Solve Problems 55-74 using augmented matrix...Ch. 4.2 - Solve Problems 55-74 using augmented matrix...Ch. 4.2 - Solve Problems 55-74 using augmented matrix...Ch. 4.2 - Solve Problems 55-74 using augmented matrix...Ch. 4.2 - Solve Problems 55-74 using augmented matrix...Ch. 4.2 - Solve Problems 55-74 using augmented matrix...Ch. 4.2 - Solve Problems 55-74 using augmented matrix...Ch. 4.2 - Solve Problems 55-74 using augmented matrix...Ch. 4.2 - Solve Problems 55-74 using augmented matrix...Ch. 4.2 - Solve Problems 55-74 using augmented matrix...Ch. 4.2 - Solve Problems 75-80 using augmented matrix...Ch. 4.2 - Solve Problems 75-80 using augmented matrix...Ch. 4.2 - Solve Problems 75-80 using augmented matrix...Ch. 4.2 - Solve Problems 75-80 using augmented matrix...Ch. 4.2 - Solve Problems 75-80 using augmented matrix...Ch. 4.2 - Solve Problems 75-80 using augmented matrix...Ch. 4.2 - Solve Problems 81-84 using augmented matrix...Ch. 4.2 - Solve Problems 81-84 using augmented matrix...Ch. 4.2 - Solve Problems 81-84 using augmented matrix...Ch. 4.2 - Solve Problems 81-84 using augmented matrix...Ch. 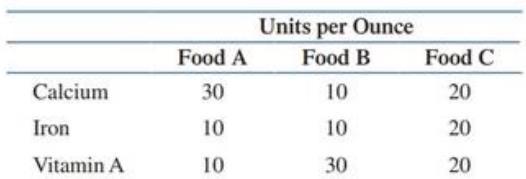4.3 - Explain why the definition of reduced form ensures...Ch. 4.3 - Refer to Example 6. The rental company charges...Ch. 4.3 - The matrices below are not in reduced form....Ch. 4.3 - Solve by Gauss-Jordan elimination:...Ch. 4.3 - Solve by Gauss-Jordan elimination:...Ch. 4.3 - Solve by Gauss-Jordan elimination:...Ch. 4.3 - Solve by Gauss-Jordan elimination:...Ch. 4.3 - A company that rents small moving trucks wants to...Ch. 4.3 - In Problems 1-4, write the augmented matrix of the...Ch. 4.3 - In Problems 1-4, write the augmented matrix of the...Ch. 4.3 - In Problems 1-4, write the augmented matrix of the...Ch. 4.3 - In Problems 14, write the augmented matrix of the...Ch. 4.3 - In Problems 5-8, write the system of linear...Ch. 4.3 - In Problems 5-8, write the system of linear...Ch. 4.3 - In Problems 5-8, write the system of linear...Ch. 4.3 - In Problems 5-8, write the system of linear...Ch. 4.3 - In Problems 9-18, if a matrix is in reduced form,...Ch. 4.3 - In Problems 9-18, if a matrix is in reduced form,...Ch. 4.3 - In Problems 9-18, if a matrix is in reduced form,...Ch. 4.3 - In Problems 9-18, if a matrix is in reduced form,...Ch. 4.3 - In Problems 9-18, if a matrix is in reduced form,...Ch. 4.3 - In Problems 9-18, if a matrix is in reduced form,...Ch. 4.3 - In Problems 9-18, if a matrix is in reduced form,...Ch. 4.3 - In Problems 9-18, if a matrix is in reduced form,...Ch. 4.3 - In Problems 9-18, if a matrix is in reduced form,...Ch. 4.3 - In Problems 9-18, if a matrix is in reduced form,...Ch. 4.3 - In Problems 19-28, write the solution of the...Ch. 4.3 - In Problems 19-28, write the solution of the...Ch. 4.3 - In Problems 19-28, write the solution of the...Ch. 4.3 - In Problems 19-28, write the solution of the...Ch. 4.3 - In Problems 19-28, write the solution of the...Ch. 4.3 - In Problems 19-28, write the solution of the...Ch. 4.3 - In Problems 19-28, write the solution of the...Ch. 4.3 - In Problems 19-28, write the solution of the...Ch. 4.3 - In Problems 19-28, write the solution of the...Ch. 4.3 - In Problems 19-28, write the solution of the...Ch. 4.3 - In which of Problems 19,21,23,25and27 is the...Ch. 4.3 - In which of Problems 20,22,24,26and28 is the...Ch. 4.3 - In which of Problems 19,21,23,25and27 is the...Ch. 4.3 - In which of Problems 20,22,24,26and28 is the...Ch. 4.3 - In Problems 33-38, discuss the validity of each...Ch. 4.3 - In Problems 33-38, discuss the validity of each...Ch. 4.3 - In Problems 33-38, discuss the validity of each...Ch. 4.3 - In Problems 33-38, discuss the validity of each...Ch. 4.3 - In Problems 33-38, discuss the validity of each...Ch. 4.3 - In Problems 33-38, discuss the validity of each...Ch. 4.3 - Use row operations to change each matrix in...Ch. 4.3 - Use row operations to change each matrix in...Ch. 4.3 - Use row operations to change each matrix in...Ch. 4.3 - Use row operations to change each matrix in...Ch. 4.3 - Use row operations to change each matrix in...Ch. 4.3 - Use row operations to change each matrix in...Ch. 4.3 - Use row operations to change each matrix in...Ch. 4.3 - Use row operations to change each matrix in...Ch. 4.3 - Solve Problems 47-62 using Gauss-Jordan...Ch. 4.3 - Solve Problems 47-62 using Gauss-Jordan...Ch. 4.3 - Solve Problems 47-62 using Gauss-Jordan...Ch. 4.3 - Solve Problems 47-62 using Gauss-Jordan...Ch. 4.3 - Solve Problems 47-62 using Gauss-Jordan...Ch. 4.3 - Solve Problems 47-62 using Gauss-Jordan...Ch. 4.3 - Solve Problems 47-62 using Gauss-Jordan...Ch. 4.3 - Solve Problems 47-62 using Gauss-Jordan...Ch. 4.3 - Solve Problems 47-62 using Gauss-Jordan...Ch. 4.3 - Solve Problems 47-62 using Gauss-Jordan...Ch. 4.3 - Solve Problems 47-62 using Gauss-Jordan...Ch. 4.3 - Solve Problems 4762 using Gauss-Jordan...Ch. 4.3 - Solve Problems 47-62 using Gauss-Jordan...Ch. 4.3 - Solve Problems 47-62 using Gauss-Jordan...Ch. 4.3 - Solve Problems 47-62 using Gauss-Jordan...Ch. 4.3 - Solve Problems 47-62 using Gauss-Jordan...Ch. 4.3 - Consider a consistent system of three linear...Ch. 4.3 - Consider a system of three linear equations in...Ch. 4.3 - Solve Problems 65-70 using Gauss-Jordan...Ch. 4.3 - Solve Problems 65-70 using Gauss-Jordan...Ch. 4.3 - Solve Problems 65-70 using Gauss-Jordan...Ch. 4.3 - Solve Problems 65-70 using Gauss-Jordan...Ch. 4.3 - Solve Problems 65-70 using Gauss-Jordan...Ch. 4.3 - Solve Problems 65-70 using Gauss-Jordan...Ch. 4.3 - Find a,bandc so that the graph of the quadratic...Ch. 4.3 - Find a,b,andc so that the graph of the quadratic...Ch. 4.3 - Boat production. A small manufacturing plant makes...Ch. 4.3 - Production scheduling. Repeat Problem 73 assuming...Ch. 4.3 - Tank car leases. A chemical manufacturer wants to...Ch. 4.3 - Airplane leases. A corporation wants to lease a...Ch. 4.3 - Tank car leases. Refer to Problem 75. The cost of...Ch. 4.3 - Airplane leases. Refer to Problem 76. The cost of...Ch. 4.3 - Income tax. A corporation has a taxable income of...Ch. 4.3 - Income tax. Repeat Problem 79 if local taxes are...Ch. 4.3 - Taxable income. As a result of several mergers and...Ch. 4.3 - Taxable income. Repeat Problem 81 if tax law is...Ch. 4.3 - Nutrition. A dietitian in a hospital is to arrange...Ch. 4.3 - Nutrition. Repeat Problem 83 if the diet is to...Ch. 4.3 - Plant food. A farmer can buy four types of plant...Ch. 4.3 - Animal feed. In a laboratory experiment, rats are...Ch. 4.3 - Plant food. Refer to Problem 85. The costs of the...Ch. 4.3 - Animal feed. Refer to Problem 86.The costs of the...Ch. 4.3 - Population growth. The U.S. population was...Ch. 4.3 - Population growth. The population of California...Ch. 4.3 - Female life expectancy. The life expectancy for...Ch. 4.3 - Male life expectancy. The life expectancy for...Ch. 4.3 - Female life expectancy. Refer to Problem 91....Ch. 4.3 - Male life expectancy. Refer to Problem 92....Ch. 4.3 - Sociology. Two sociologists have grant money to...Ch. 4.3 - Sociology. Repeat Problem 95 if 650 telephone...Ch. 4.3 - Traffic Wow. The rush-hour traffic flow for a...Ch. 4.3 - Traffic flow. Refer to Problem 97. Closing...Ch. 4.4 - In addition to the commutative and zero...Ch. 4.4 - Add: 321013+231212Ch. 4.4 - Subtract: 235321Ch. 4.4 - Find a,b,c,andd so that abcd4213=2582Ch. 4.4 - Find: 101.30.23.5Ch. 4.4 - Repeat Example 5 with...Ch. 4.4 - 10322341=?Ch. 4.4 - If the factory in Example 7 also produces a trick...Ch. 4.4 - Find each product, if it is defined:...Ch. 4.4 - Find a,b,c, and d so that 6053acbd=1624646Ch. 4.4 - Refer to Example 10. The company wants to know how...Ch. 4.4 - In Problems 1-14, perform the indicated operation,...Ch. 4.4 - In Problems 1-14, perform the indicated operation,...Ch. 4.4 - In Problems 1-14, perform the indicated operation,...Ch. 4.4 - In Problems 1-14, perform the indicated operation,...Ch. 4.4 - In Problems 1-14, perform the indicated operation,...Ch. 4.4 - In Problems 1-14, perform the indicated operation,...Ch. 4.4 - Prob. 7ECh. 4.4 - In Problems 1-14, perform the indicated operation,...Ch. 4.4 - In Problems 1-14, perform the indicated operation,...Ch. 4.4 - In Problems 1-14, perform the indicated operation,...Ch. 4.4 - In Problems 1-14, perform the indicated operation,...Ch. 4.4 - In Problems 1-14, perform the indicated operation,...Ch. 4.4 - In Problems 1-14, perform the indicated operation,...Ch. 4.4 - Prob. 14ECh. 4.4 - In Problems 15-22, find the matrix product. Note...Ch. 4.4 - In Problems 15-22, find the matrix product. Note...Ch. 4.4 - In Problems 15-22, find the matrix product. Note...Ch. 4.4 - In Problems 15-22, find the matrix product. Note...Ch. 4.4 - In Problems 15-22, find the matrix product. Note...Ch. 4.4 - In Problems 15-22, find the matrix product. Note...Ch. 4.4 - In Problems 15-22, find the matrix product. Note...Ch. 4.4 - In Problems 15-22, find the matrix product. Note...Ch. 4.4 - Find the products in Problems 23-30. 3254Ch. 4.4 - Find the products in Problems 23-30. 3524Ch. 4.4 - Find the products in Problems 23-30. 5432Ch. 4.4 - Find the products in Problems 23-30. 2435Ch. 4.4 - Find the products in Problems 23-30. 101213Ch. 4.4 - Find the products in Problems 23-30. 113201Ch. 4.4 - Find the products in Problems 23-30. 213101Ch. 4.4 - Find the products in Problems 23-30. 201113Ch. 4.4 - Problems 31-48 refer to the following matrices:...Ch. 4.4 - Problems 31-48 refer to the following matrices:...Ch. 4.4 - Problems 31-48 refer to the following matrices:...Ch. 4.4 - Problems 31-48 refer to the following matrices:...Ch. 4.4 - Problems 31-48 refer to the following matrices:...Ch. 4.4 - Problems 31-48 refer to the following matrices:...Ch. 4.4 - Problems 31-48 refer to the following matrices:...Ch. 4.4 - Problems 31-48 refer to the following matrices:...Ch. 4.4 - Problems 31-48 refer to the following matrices:...Ch. 4.4 - Problems 31-48 refer to the following matrices:...Ch. 4.4 - Problems 31-48 refer to the following matrices:...Ch. 4.4 - Problems 31-48 refer to the following matrices:...Ch. 4.4 - Problems 31-48 refer to the following matrices:...Ch. 4.4 - Problems 31-48 refer to the following matrices:...Ch. 4.4 - Problems 31-48 refer to the following matrices:...Ch. 4.4 - Problems 31-48 refer to the following matrices:...Ch. 4.4 - Problems 31-48 refer to the following matrices:...Ch. 4.4 - Problems 31-48 refer to the following matrices:...Ch. 4.4 - If aandb are nonzero real numbers A=aabb,andB=aaaa...Ch. 4.4 - If aandb are nonzero real numbers,...Ch. 4.4 - If aandb are nonzero real numbers and A=abb2a2ab...Ch. 4.4 - If aandb are nonzero real numbers and A=abab1ab2ab...Ch. 4.4 - In Problems 53-54, use a graphing calculator to...Ch. 4.4 - In Problems 53-54, use a graphing calculator to...Ch. 4.4 - Find a,b,c, and d so that abcd+2301=1234Ch. 4.4 - Find w,x,y, and z so that 4230+wxyz=2305Ch. 4.4 - Find a,b,c, and d so that 1223abcd=1032Ch. 4.4 - Find a,b,c, and d so that 1314abcd=6577Ch. 4.4 - In Problems 59-62, determine whether the statement...Ch. 4.4 - In Problems 59-62, determine whether the statement...Ch. 4.4 - In Problems 59-62, determine whether the statement...Ch. 4.4 - In Problems 59-62, determine whether the statement...Ch. 4.4 - A square matrix is a diagonal matrix if all...Ch. 4.4 - A square matrix is an upper triangular matrix if...Ch. 4.4 - Cost analysis. A company with two different plants...Ch. 4.4 - Cost analysis. If both labor and materials at...Ch. 4.4 - Markup. An import car dealer sells three models of...Ch. 4.4 - Markup. Referring to Problem 67, what is the...Ch. 4.4 - Labor costs. A company with manufacturing plants...Ch. 4.4 - Inventory value. A personal computer retail...Ch. 4.4 - Cereal. A nutritionist for a cereal company blends...Ch. 4.4 - Heredity. Gregor Mendel (1822-1884) made...Ch. 4.4 - Politics. In a local California election, a public...Ch. 4.4 - Test averages. A teacher has given four tests to a...Ch. 4.5 - The only real number solutions to the equation...Ch. 4.5 - (A) Suppose that the square matrix M has a row of...Ch. 4.5 - The inverse of A=abcd is...Ch. 4.5 - Multiply (a) 10012357and23571001 (b)...Ch. 4.5 - Let M=311110101 (A) Form the augmented matrix MI....Ch. 4.5 - Find M1, given M=2612Ch. 4.5 - Find N1,givenN=3162.Ch. 4.5 - The message below was also encoded with the matrix...Ch. 4.5 - In Problems 1-4, find the additive inverse and...Ch. 4.5 - In Problems 1-4, find the additive inverse and...Ch. 4.5 - Prob. 3ECh. 4.5 - Prob. 4ECh. 4.5 - In Problems 5-8, does the given matrix have a...Ch. 4.5 - In Problems 5-8, does the given matrix have a...Ch. 4.5 - In Problems 5-8, does the given matrix have a...Ch. 4.5 - In Problems 5-8, does the given matrix have a...Ch. 4.5 - In Problems 9-18, find the matrix products. Note...Ch. 4.5 - In Problems 9-18, find the matrix products. Note...Ch. 4.5 - In Problems 9-18, find the matrix products. Note...Ch. 4.5 - In Problems 9-18, find the matrix products. Note...Ch. 4.5 - In Problems 9-18, find the matrix products. Note...Ch. 4.5 - In Problems 9-18, find the matrix products. Note...Ch. 4.5 - In Problems 9-18, find the matrix products. Note...Ch. 4.5 - In Problems 9-18, find the matrix products. Note...Ch. 4.5 - In Problems 9-18, find the matrix products. Note...Ch. 4.5 - In Problems 9-18, find the matrix products. Note...Ch. 4.5 - In Problems 19-28, examine the product of the two...Ch. 4.5 - In Problems 19-28, examine the product of the two...Ch. 4.5 - In Problems 19-28, examine the product of the two...Ch. 4.5 - In Problems 19-28, examine the product of the two...Ch. 4.5 - In Problems 19-28, examine the product of the two...Ch. 4.5 - In Problems 19-28, examine the product of the two...Ch. 4.5 - In Problems 19-28, examine the product of the two...Ch. 4.5 - In Problems 19-28, examine the product of the two...Ch. 4.5 - In Problems 19-28, examine the product of the two...Ch. 4.5 - In Problems 19-28, examine the product of the two...Ch. 4.5 - Without performing any row operations, explain why...Ch. 4.5 - Without performing any row operations, explain why...Ch. 4.5 - Without performing any row operations, explain why...Ch. 4.5 - Without performing any row operations, explain why...Ch. 4.5 - Without performing any row operations, explain why...Ch. 4.5 - Without performing any row operations, explain why...Ch. 4.5 - Without performing any row operations, explain why...Ch. 4.5 - Without performing any row operations, explain why...Ch. 4.5 - Without performing any row operations, explain why...Ch. 4.5 - Without performing any row operations, explain why...Ch. 4.5 - Given M in Problems 39-48, Find M1 and show that...Ch. 4.5 - Given M in Problems 39-48, Find M1 and show that...Ch. 4.5 - Given M in Problems 39-48, Find M1 and show that...Ch. 4.5 - Given M in Problems 39-48, Find M1 and show that...Ch. 4.5 - Given M in Problems 39-48, Find M1 and show that...Ch. 4.5 - Given M in Problems 39-48, Find M1 and show that...Ch. 4.5 - Given M in Problems 39-48, Find M1 and show that...Ch. 4.5 - Given M in Problems 39-48, Find M1 and show that...Ch. 4.5 - Given M in Problems 39-48, Find M1 and show that...Ch. 4.5 - Given M in Problems 39-48, Find M1 and show that...Ch. 4.5 - Find the inverse of each matrix in Problems 49-56,...Ch. 4.5 - Find the inverse of each matrix in Problems 49-56,...Ch. 4.5 - Find the inverse of each matrix in Problems 49-56,...Ch. 4.5 - Find the inverse of each matrix in Problems 49-56,...Ch. 4.5 - Find the inverse of each matrix in Problems 49-56,...Ch. 4.5 - Find the inverse of each matrix in Problems 49-56,...Ch. 4.5 - Find the inverse of each matrix in Problems 49-56,...Ch. 4.5 - Find the inverse of each matrix in Problems 49-56,...Ch. 4.5 - In Problems 57-60, find the inverse. Note that...Ch. 4.5 - In Problems 57-60, find the inverse. Note that...Ch. 4.5 - In Problems 57-60, find the inverse. Note that...Ch. 4.5 - In Problems 57-60, find the inverse. Note that...Ch. 4.5 - Find the inverse of each matrix in Problems 61-68,...Ch. 4.5 - Find the inverse of each matrix in Problems 61-68,...Ch. 4.5 - Find the inverse of each matrix in Problems 61-68,...Ch. 4.5 - Find the inverse of each matrix in Problems 61-68,...Ch. 4.5 - Find the inverse of each matrix in Problems 61-68,...Ch. 4.5 - Find the inverse of each matrix in Problems 61-68,...Ch. 4.5 - Find the inverse of each matrix in Problems 61-68,...Ch. 4.5 - Find the inverse of each matrix in Problems 6168,...Ch. 4.5 - Show that A11=Afor;A=4332Ch. 4.5 - Show that AB1=B1A1for A=4332andB=2537Ch. 4.5 - Discuss the existence of M1 for 22 diagonal...Ch. 4.5 - Discuss the existence of M1for22 for upper...Ch. 4.5 - Prob. 73ECh. 4.5 - In Problems 73-75, find A1 and A2. 5465Ch. 4.5 - In Problems 73-75, find A1 and A2. 5385Ch. 4.5 - Based on your observations in Problems 73-75, if...Ch. 4.5 - Problems 77-80 refer to the encoding matrix A=1213...Ch. 4.5 - Problems 77-80 refer to the encoding matrix A=1213...Ch. 4.5 - Problems 77-80 refer to the encoding matrix A=1213...Ch. 4.5 - Problems 77-80 refer to the encoding matrix A=1213...Ch. 4.5 - Problems 81-84 require the use of a graphing...Ch. 4.5 - Problems 81-84 require the use of a graphing...Ch. 4.5 - Problems 81-84 require the use of a graphing...Ch. 4.5 - Problems 81-84 require the use of a graphing...Ch. 4.5 - Problems 85-88 require the use of a graphing...Ch. 4.5 - Problems 85-88 require the use of a graphing...Ch. 4.5 - Problems 85-88 require the use of a graphing...Ch. 4.5 - Problems 85-88 require the use of a graphing...Ch. 4.6 - Refer to the mathematical model in Example 4:...Ch. 4.6 - Given an nn matrix Aandn1 column matrices...Ch. 4.6 - Use matrix inverse methods to solve the system:...Ch. 4.6 - Use matrix inverse methods to solve each of the...Ch. 4.6 - Repeat Example 4 with investment A paying 4 and...Ch. 4.6 - In Problems 1-8, solve each equation for x, where...Ch. 4.6 - In Problems 1-8, solve each equation for x, where...Ch. 4.6 - In Problems 1-8, solve each equation for x, where...Ch. 4.6 - In Problems 1-8, solve each equation for x, where...Ch. 4.6 - In Problems 1-8, solve each equation for x, where...Ch. 4.6 - In Problems 1-8, solve each equation for x, where...Ch. 4.6 - In Problems 1-8, solve each equation for x, where...Ch. 4.6 - In Problems 1-8, solve each equation for x, where...Ch. 4.6 - Write Problems 9-12 as systems of linear equations...Ch. 4.6 - Write Problems 9-12 as systems of linear equations...Ch. 4.6 - Write Problems 9-12 as systems of linear equations...Ch. 4.6 - Write Problems 9-12 as systems of linear equations...Ch. 4.6 - Write each system in Problems 13-16 as a matrix...Ch. 4.6 - Write each system in Problems 13-16 as a matrix...Ch. 4.6 - Write each system in Problems 13-16 as a matrix...Ch. 4.6 - Write each system in Problems 13-16 as a matrix...Ch. 4.6 - Find x1andx2 in problems 17-20. x1x2=321421Ch. 4.6 - Find x1andx2 in problems 17-20. x1x2=211232Ch. 4.6 - Find x1andx2 in problems 17-20. x1x2=232132Ch. 4.6 - Find x1andx2 in problems 17-20. x1x2=310221Ch. 4.6 - In Problems 21-24, find x1 and x2. 1112x1x2=57Ch. 4.6 - In Problems 21-24, find x1 and x2. 1314x1x2=96Ch. 4.6 - In Problems 21-24, find x1 and x2. 1123x1x2=1510Ch. 4.6 - In Problems 21-24, find x1 and x2. 1132x1x2=1020Ch. 4.6 - In Problems 25-30, solve for x1andx2....Ch. 4.6 - In Problems 25-30, solve for x1andx2....Ch. 4.6 - In Problems 25-30, solve for x1andx2....Ch. 4.6 - In Problems 25-30, solve for x1andx2....Ch. 4.6 - In Problems 25-30, solve for x1andx2....Ch. 4.6 - In Problems 25-30, solve for x1andx2....Ch. 4.6 - In Problems 31-38, write each system as a matrix...Ch. 4.6 - In Problems 31-38, write each system as a matrix...Ch. 4.6 - In Problems 31-38, write each system as a matrix...Ch. 4.6 - In Problems 31-38, write each system as a matrix...Ch. 4.6 - In Problems 31-38, write each system as a matrix...Ch. 4.6 - In Problems 31-38, write each system as a matrix...Ch. 4.6 - In Problems 31-38, write each system as a matrix...Ch. 4.6 - In Problems 31-38, write each system as a matrix...Ch. 4.6 - In Problems 39-44, the matrix equation is not...Ch. 4.6 - In Problems 39-44, the matrix equation is not...Ch. 4.6 - In Problems 39-44, the matrix equation is not...Ch. 4.6 - In Problems 39-44, the matrix equation is not...Ch. 4.6 - In Problems 39-44, the matrix equation is not...Ch. 4.6 - In Problems 39-44, the matrix equation is not...Ch. 4.6 - In Problems 45-50, explain why the system cannot...Ch. 4.6 - In Problems 45-50, explain why the system cannot...Ch. 4.6 - In Problems 45-50, explain why the system cannot...Ch. 4.6 - In Problems 45-50, explain why the system cannot...Ch. 4.6 - In Problems 45-50, explain why the system cannot...Ch. 4.6 - In Problems 45-50, explain why the system cannot...Ch. 4.6 - For nn matrices A and B, and n1 column matrices...Ch. 4.6 - For nn matrices A and B, and n1 column matrices...Ch. 4.6 - For nn matrices A and B, and n1 column matrices...Ch. 4.6 - For nn matrices A and B, and n1 column matrices...Ch. 4.6 - For nn matrices A and B, and n1 column matrices...Ch. 4.6 - For nn matrices A and B, and n1 column matrices...Ch. 4.6 - In Problems 57 and 58, solve for x1andx2....Ch. 4.6 - In Problems 57 and 58, solve for x1andx2....Ch. 4.6 - In Problems 59-62. Write each system as a matrix...Ch. 4.6 - In Problems 59-62. Write each system as a matrix...Ch. 4.6 - In Problems 59-62. Write each system as a matrix...Ch. 4.6 - In Problems 59-62. Write each system as a matrix...Ch. 4.6 - Construct a mathematical model for each of the...Ch. 4.6 - Construct a mathematical model for each of the...Ch. 4.6 - Construct a mathematical model for each of the...Ch. 4.6 - Construct a mathematical model for each of the...Ch. 4.6 - Incentive plan. A small company provides an...Ch. 4.6 - Incentive plan. Repeat Problem 67 if the company...Ch. 4.6 - Diets. A biologist has available two commercial...Ch. 4.6 - Education. A stale university system is planning...Ch. 4.7 - If equations 2 and 3 are valid for an economy with...Ch. 4.7 - An economy is based on three sectors, coal, oil...Ch. 4.7 - In Problems 18, solve each equation for x, where x...Ch. 4.7 - In Problems 1-8, solve each equation for x, where...Ch. 4.7 - In Problems 1-8, solve each equation for x, where...Ch. 4.7 - In Problems 1-8, solve each equation for x, where...Ch. 4.7 - In Problems 1-8, solve each equation for x, where...Ch. 4.7 - In Problems 1-8, solve each equation for x, where...Ch. 4.7 - In Problems 1-8, solve each equation for x, where...Ch. 4.7 - In Problems 1-8, solve each equation for x, where...Ch. 4.7 - Problems 9-14 pertain to the following...Ch. 4.7 - Problems 9-14 pertain to the following...Ch. 4.7 - Problems 9-14 pertain to the following...Ch. 4.7 - Problems 9-14 pertain to the following...Ch. 4.7 - Problems 9-14 pertain to the following...Ch. 4.7 - Problems 9-14 pertain to the following...Ch. 4.7 - Problems 15-20 pertain to the following...Ch. 4.7 - Problems 15-20 pertain to the following...Ch. 4.7 - Problems 15-20 pertain to the following...Ch. 4.7 - Problems 15-20 pertain to the following...Ch. 4.7 - Problems 15-20 pertain to the following...Ch. 4.7 - Problems 15-20 pertain to the following...Ch. 4.7 - In Problems 21-26, find IM1 and X....Ch. 4.7 - In Problems 21-26, find IM1 and X....Ch. 4.7 - In Problems 21-26, find IM1 and X....Ch. 4.7 - In Problems 21-26, find IM1 and X....Ch. 4.7 - In Problems 21-26, find IM1 and X....Ch. 4.7 - In Problems 21-26, find IM1 and X....Ch. 4.7 - The technology matrix for an economy based on...Ch. 4.7 - The technology matrix for an economy based on...Ch. 4.7 - Refer to Problem 27. Fill in the elements in the...Ch. 4.7 - Refer lo Problem 28. Fill in the elements in the...Ch. 4.7 - The technology matrix for an economy based on...Ch. 4.7 - The technology matrix for an economy based on...Ch. 4.7 - All the technology matrices in the text have...Ch. 4.7 - The sum of the elements in a column of any of the...Ch. 4.7 - Coal, steel. An economy is based on two industrial...Ch. 4.7 - Transportation, manufacturing. An economy is based...Ch. 4.7 - Agriculture, tourism. The economy of a small...Ch. 4.7 - Agriculture, oil. The economy of a country is...Ch. 4.7 - Agriculture, manufacturing, energy. An economy is...Ch. 4.7 - Electricity, natural gas, oil. A large energy...Ch. 4.7 - Four sectors. An economy is based on four sectors,...Ch. 4.7 - Repeal Problem 41 with the following tableCh. 4 - Solve the following system by graphing 2xy=4x2y=4Ch. 4 - Solve the system in Problem 1 by substitution...Ch. 4 - If a matrix is in reduced form, say so. If not,...Ch. 4 - Given matrices A and B. A=5310248130 B=320417 (A)...Ch. 4 - Find x1andx2 : (A) 1213x1x2=42 (B)...Ch. 4 - In Problem 6-14, perform the operations that are...Ch. 4 - In Problem 6-14, perform the operations that are...Ch. 4 - In Problem 6-14, perform the operations that are...Ch. 4 - In Problem 6-14, perform the operations that are...Ch. 4 - In Problem 6-14, perform the operations that are...Ch. 4 - In Problem 6-14, perform the operations that are...Ch. 4 - In Problem 6-14, perform the operations that are...Ch. 4 - In Problem 6-14, perform the operations that are...Ch. 4 - In Problem 6-14, perform the operations that are...Ch. 4 - Find the inverse of the matrix A given below by...Ch. 4 - Solve the following system using elimination by...Ch. 4 - Solve the system in Problem 16 by performing...Ch. 4 - Solve the system in Problem 16 by writing the...Ch. 4 - In Problems 19-24, perform the operations that are...Ch. 4 - In Problems 19-24, perform the operations that are...Ch. 4 - In Problems 19-24, perform the operations that are...Ch. 4 - In Problems 1924, perform the operations that are...Ch. 4 - In Problems 19-24, perform the operations that are...Ch. 4 - In Problems 19-24, perform the operations that are...Ch. 4 - Find the inverse of the matrix A given below by...Ch. 4 - Solve by Gauss-Jordan elimination: (A)...Ch. 4 - Solve the system in Problem 26A by writing the...Ch. 4 - Discuss the relationship between the numbers of...Ch. 4 - An economy is based on two sectors, agriculture...Ch. 4 - Use the matrix M in Problem 29 to fill in the...Ch. 4 - An economy is based on two sectors, coal and...Ch. 4 - Use graphical approximation techniques on a...Ch. 4 - Find the inverse of the matrix A given below. Show...Ch. 4 - Solve the system...Ch. 4 - Solve Problem 34 by Gauss-Jordan elimination.Ch. 4 - Given the technology matrix M and the final demand...Ch. 4 - Discuss the number of solutions for a system of n...Ch. 4 - Discuss the number of solutions for the system...Ch. 4 - One solution to the input-output equation X=MX+D...Ch. 4 - Break-even analysis. A cookware manufacturer is...Ch. 4 - Resource allocation. An international mining...Ch. 4 - Resource allocation. (A) Set up Problem 41 as a...Ch. 4 - Business leases. A grain company wants to lease a...Ch. 4 - Material costs. A manufacturer wishes to make two...Ch. 4 - Labor costs. A company with manufacturing plants...Ch. 4 - Investment analysis. A person has $5,000 to...Ch. 4 - Investment analysis. Solve Problem 46 by using a...Ch. 4 - Investment analysis. In Problem 46, is it possible...Ch. 4 - Ticket prices. An outdoor amphitheater has 25,000...Ch. 4 - Ticket prices. Discuss the effect on the solutions...Ch. 4 - Input-output analysis. An economy is based on two...Ch. 4 - Cryptography. The following message was encoded...Ch. 4 - Traffic flow. The rush-hour traffic flow (in...

Additional Math Textbook Solutions

Find more solutions based on key concepts
1. Heights Heights of adult males are normally distributed. If a large sample of heights of adult males is rand...

Elementary Statistics (13th Edition)

In hypothesis testing, the common level of significance is =0.05. Some might argue for a level of significance ...

Basic Business Statistics, Student Value Edition

If n is a counting number, bn, read______, indicates that there are n factors of b. The number b is called the_...

Algebra and Trigonometry (6th Edition)

In a right triangle, with legs a and b and hypotenuse c , the Pythagorean Theorem states that _______. (p. A14)

Precalculus

Heights Refer to the dotplot in the previous question. a. What is the height of a woman with a z-score of -1? b...

Introductory Statistics

the below subtraction

Pre-Algebra Student Edition

Knowledge Booster
Background pattern image
Math
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, subject and related others by exploring similar questions and additional content below.
Similar questions
SEE MORE QUESTIONS
Recommended textbooks for you
Text book image
Linear Algebra: A Modern Introduction
Algebra
ISBN:9781285463247
Author:David Poole
Publisher:Cengage Learning
Text book image
Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:9781133382119
Author:Swokowski
Publisher:Cengage
Text book image
College Algebra
Algebra
ISBN:9781305115545
Author:James Stewart, Lothar Redlin, Saleem Watson
Publisher:Cengage Learning
Text book image
Algebra: Structure And Method, Book 1
Algebra
ISBN:9780395977224
Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. Cole
Publisher:McDougal Littell
Text book image
Algebra and Trigonometry (MindTap Course List)
Algebra
ISBN:9781305071742
Author:James Stewart, Lothar Redlin, Saleem Watson
Publisher:Cengage Learning
Text book image
College Algebra
Algebra
ISBN:9781337282291
Author:Ron Larson
Publisher:Cengage Learning
SEE MORE TEXTBOOKS
Matrix Factorization - Numberphile; Author: Numberphile;https://www.youtube.com/watch?v=wTUSz-HSaBg;License: Standard YouTube License, CC-BY