
Concept explainers
Mortgage payments: The following table presents interest rates, in percent, for 30-year and
15-year fixed-rate mortgages, for January through December, 2012.
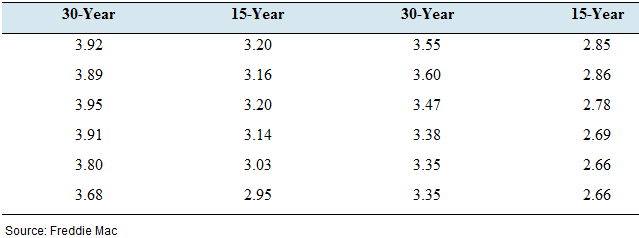
- Compute the least-squares regression line for predicting the 15-year rate from the 30-year rate.
- Construct a scatter-plot of the 15-year rate (y) versus the 30-year rate (x). Graph the least-squares regression line on the same axes.
- Is it possible to interpret die y-intercept? Explain.
- If the 30-year rate differs by 0.3 percent from one month to the next, by how much would you predict the 15-year rate to differ?
- Predict the 15-year rate for a month when the 30-year rate is 3.5 percent.
a.
To compute:The least squares regression line for the
Answer to Problem 26E
The least square regression line of the given data set is,
Explanation of Solution
The mortgage rate for
Calculation:
The least-square regression is given by the formula,
Where
The correlation coefficient is given by the formula,
Let

The correlation coefficient can be obtained by the following table.
Hence, the correlation coefficient is,
Then, the coefficient
Therefore,
Conclusion:
The least square regression line is found to be,
b.
To graph:The scatter plot for the two mortgage rates.
Explanation of Solution
Graph:
Taking the

Interpretation:
We can clearly observe that there is a strong linear relationship between these two parameters in the positive direction.
c.
To explain:The interpretation of the
Answer to Problem 26E
No, the
Explanation of Solution
The lease-square regression line has been computed in the part (a) s,
By the constant
The
Note that a rate of mortgage cannot be negative.
Conclusion:
Therefore, the
d.
To calculate:The difference in the percentage of the
Answer to Problem 26E
The
Explanation of Solution
The lease-square regression line has been computed in the part (a) s,
Calculation:
Let the initial
Also, the increased rate should be
Simplifying the obtained weight,
Therefore, the difference of two weights should be,
Interpretation:
According to the calculation, the
e.
To find:The predicted
Answer to Problem 26E
When
Explanation of Solution
Calculation:
When the
By substituting this value into the least-square regression line of the relationship, we can obtain the corresponding
Conclusion:
The predicted
Want to see more full solutions like this?
Chapter 4 Solutions
Elementary Statistics ( 3rd International Edition ) Isbn:9781260092561
- Theorem 3.5 Suppose that P and Q are probability measures defined on the same probability space (2, F), and that F is generated by a л-system A. If P(A) = Q(A) for all A = A, then P = Q, i.e., P(A) = Q(A) for all A = F.arrow_forward6. Show that, for any random variable, X, and a > 0, Lo P(x -00 P(x < xarrow_forward5. Suppose that X is an integer valued random variable, and let mЄ N. Show that 8 11118 P(narrow_forward食食假 6. Show that I(AUB) = max{1{A}, I{B}} = I{A} + I{B} - I{A} I{B}; I(AB)= min{I{A}, I{B}} = I{A} I{B}; I{A A B} = I{A} + I{B}-21{A} I{B} = (I{A} - I{B})². -arrow_forward11. Suppose that the events (An, n ≥ 1) are independent. Show that the inclusion- exclusion formula reduces to P(UAL)-1-(1-P(Ak)). k=1 k=1arrow_forward8. Show that, if {Xn, n≥ 1} are independent random variables, then sup X,, A) < ∞ for some A.arrow_forwardarrow_back_iosSEE MORE QUESTIONSarrow_forward_iosRecommended textbooks for you
 College AlgebraAlgebraISBN:9781305115545Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
College AlgebraAlgebraISBN:9781305115545Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning Algebra and Trigonometry (MindTap Course List)AlgebraISBN:9781305071742Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
Algebra and Trigonometry (MindTap Course List)AlgebraISBN:9781305071742Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
 Elementary Linear Algebra (MindTap Course List)AlgebraISBN:9781305658004Author:Ron LarsonPublisher:Cengage Learning
Elementary Linear Algebra (MindTap Course List)AlgebraISBN:9781305658004Author:Ron LarsonPublisher:Cengage Learning Functions and Change: A Modeling Approach to Coll...AlgebraISBN:9781337111348Author:Bruce Crauder, Benny Evans, Alan NoellPublisher:Cengage Learning
Functions and Change: A Modeling Approach to Coll...AlgebraISBN:9781337111348Author:Bruce Crauder, Benny Evans, Alan NoellPublisher:Cengage Learning Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
 College AlgebraAlgebraISBN:9781305115545Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
College AlgebraAlgebraISBN:9781305115545Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning Algebra and Trigonometry (MindTap Course List)AlgebraISBN:9781305071742Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
Algebra and Trigonometry (MindTap Course List)AlgebraISBN:9781305071742Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
 Elementary Linear Algebra (MindTap Course List)AlgebraISBN:9781305658004Author:Ron LarsonPublisher:Cengage Learning
Elementary Linear Algebra (MindTap Course List)AlgebraISBN:9781305658004Author:Ron LarsonPublisher:Cengage Learning Functions and Change: A Modeling Approach to Coll...AlgebraISBN:9781337111348Author:Bruce Crauder, Benny Evans, Alan NoellPublisher:Cengage Learning
Functions and Change: A Modeling Approach to Coll...AlgebraISBN:9781337111348Author:Bruce Crauder, Benny Evans, Alan NoellPublisher:Cengage Learning Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill