Concept explainers
Videos
Spirit Airlines kept track of the number of empty seats on flight 308 (DEN-DTW) for 10 consecutive trips on each weekday except Friday. (a) Sort the data for each day. (b) Find the
a.
Arrange the data.
Answer to Problem 16SE
The sorted data is:
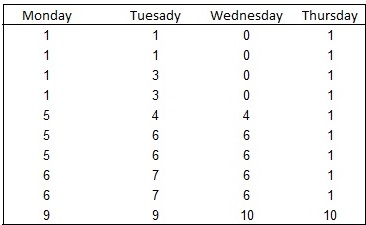
Explanation of Solution
Calculation:
The dataset represents the number of empty seats on flight 308 for 10 consecutive trips.
The data set of empty seats can be sorted either ascending or descending.
Here, the data is sorted in the ascending order.
Sorting:
Software procedure:
Step-by-step procedure to sort the data in ascending using the EXCEL is given below:
- Open an EXCEL file.
- Enter the data in the column in H cells H1 to H10, column in I cells I1 to I10, J cells J1 to J10 and column in K cells K1 to K10.
- Choose Data > Sort.
- In Column drop down box, select Column H.
- In Sort on drop down box, select Values.
- In Order drop down box, select “Smallest to Largest”.
- Click OK.
- In Column drop down box, select Column I.
- In Sort on drop down box, select Values.
- In Order drop down box, select “Smallest to Largest”.
- Click OK.
- In Column drop down box, select Column J.
- In Sort on drop down box, select Values.
- In Order drop down box, select “Smallest to Largest”.
- Click OK.
- In Column drop down box, select Column K.
- In Sort on drop down box, select Values.
- In Order drop down box, select “Smallest to Largest”.
- Click OK.
Thus, the sorted data has been stored in the column in H cells H1 to H10, column in I cells I1 to I10, J cells J1 to J10 and column in K cells K1 to K10.
b.
Find the Mean, Median, Mode, Midrange, Geometric mean, and 10% Trimmed mean.
Answer to Problem 16SE
The Mean, Median, Mode, Midrange, Geometric mean, and 10% Trimmed mean is:
| Descriptive measures | Monday | Tuesday | Wednesday | Thursday |
| Mean | 4 | 4.70 | 3.80 | 1.90 |
| Median | 5 | 5 | 5 | 1 |
| Mode | 1 | 1 | 6 | 1 |
| Geometric mean | 2.89 | 3.76 | Not applicable | 1.26 |
| Midrange | 5 | 5 | 5 | 5.50 |
| Trimmed mean | 3.75 | 4.63 | 3.50 | 1 |
Explanation of Solution
Calculation:
For Monday:
Mean:
Software procedure:
Step-by-step software procedure to obtain Mean using EXCEL software is as follows:
- Open an EXCEL file.
- Enter the data in the column I in cells I1 to I10.
- In cell A1, enter the formula “=AVERAGE(I1:I10)”.
- Output using EXCEL software is given below:
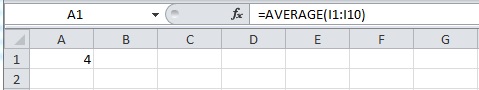
Thus, the mean number of empty seats on Monday is 4.
Median:
Software procedure:
Step-by-step software procedure to obtain Median using EXCEL software is as follows:
- Open an EXCEL file.
- Enter the data in the column I in cells I1 to I10.
- In cell A1, enter the formula “=MEDIAN(I1:110)”.
- Output using EXCEL software is given below:
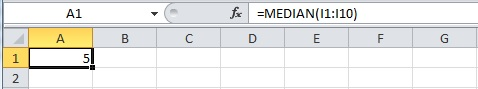
Thus, the median for the number of empty seats on Monday is 5.
Mode:
Software procedure:
Step-by-step software procedure to obtain Mode using EXCEL software is as follows:
- Open an EXCEL file.
- Enter the data in the column I in cells I1 to I10.
- In cell A1, enter the formula “=MODE.SNGL(I1:I10)”.
- Output using EXCEL software is given below:
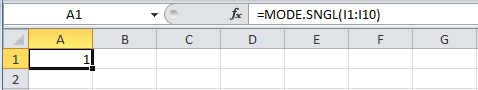
Thus, the mode for the number of empty seats on Monday is 1.
Geometric mean:
Software procedure:
Step-by-step software procedure to obtain Geometric mean using EXCEL software is as follows:
- Open an EXCEL file.
- Enter the data in the column I in cells I1 to I10.
- In cell A1, enter the formula “=GEOMEAN(I1:I10)”.
- Output using EXCEL software is given below:
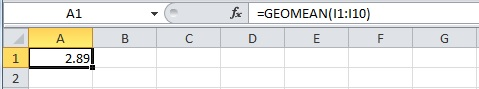
Thus, the geometric mean for the number of empty seats on Monday is 2.89.
Midrange:
Software procedure:
Step-by-step software procedure to obtain Midrange using EXCEL software is as follows:
- Open an EXCEL file.
- Enter the data in the column I in cells I1 to I10.
- In cell A1, enter the formula “=(MIN(I1:I10)+MAX(I1:I10))/2”.
- Output using EXCEL software is given below:
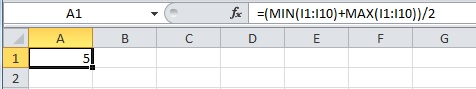
- Thus, the midrange for the number of empty seats on Monday is 5.
10% Trimmed mean:
Software procedure:
Step-by-step software procedure to obtain 10% Trimmed mean using EXCEL software is as follows:
- Open an EXCEL file.
- Enter the data in the column I in cells I1 to I10.
- In cell A1, enter the formula “=TRIMMEAN(I1:I10,0.2)”.
- Output using EXCEL software is given below:
- Thus, the 10% Trimmed mean for the number of empty seats on Monday is 3.75.
For Tuesday:
Mean:
Software procedure:
Step-by-step software procedure to obtain Mean using EXCEL software is as follows:
- Open an EXCEL file.
- Enter the data in the column J in cells J1 to J10.
- In cell A1, enter the formula “=AVERAGE(J1:J10)”.
- Output using EXCEL software is given below:
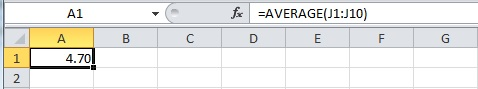
Thus, the mean number of empty seats on Tuesday is 4.70.
Median:
Software procedure:
Step-by-step software procedure to obtain Median using EXCEL software is as follows:
- Open an EXCEL file.
- Enter the data in the column J in cells J1 to J10.
- In cell A1, enter the formula “=MEDIAN(J1:J10)”.
- Output using EXCEL software is given below:
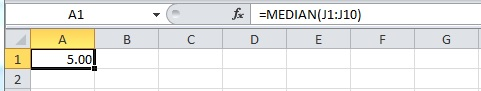
Thus, the median for the number of empty seats on Tuesday is 5.
Mode:
Software procedure:
Step-by-step software procedure to obtain Mode using EXCEL software is as follows:
- Open an EXCEL file.
- Enter the data in the column J in cells J1 to J10.
- In cell A1, enter the formula “=MODE.SNGL(J1:J10)”.
- Output using EXCEL software is given below:
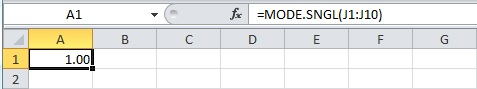
Thus, the mode for the number of empty seat on Tuesday is 1.
Geometric mean:
Software procedure:
Step-by-step software procedure to obtain Geometric mean using EXCEL software is as follows:
- Open an EXCEL file.
- Enter the data in the column J in cells J1 to J10.
- In cell A1, enter the formula “=GEOMEAN(J1:J10)”.
- Output using EXCEL software is given below:
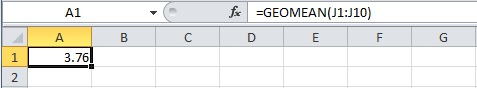
Thus, the geometric mean for the number of empty seats on Tuesday is 3.76.
Midrange:
Software procedure:
Step-by-step software procedure to obtain Midrange using EXCEL software is as follows:
- Open an EXCEL file.
- Enter the data in the column J in cells J1 to J10.
- In cell A1, enter the formula “=(MIN(J1:J10)+MAX(J1:J10))/2”.
- Output using EXCEL software is given below:
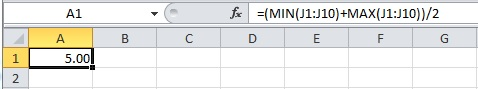
- Thus, the midrange for the number of empty seats on Tuesday is 5.
10% Trimmed mean:
Software procedure:
Step-by-step software procedure to obtain 10% Trimmed mean using EXCEL software is as follows:
- Open an EXCEL file.
- Enter the data in the column J in cells J1 to J10.
- In cell A1, enter the formula “=TRIMMEAN(J1:J10,0.2)”.
- Output using EXCEL software is given below:
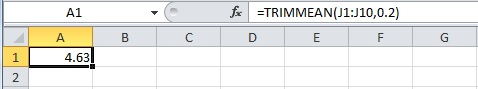
- Thus, the 10% Trimmed mean for the number of empty seats on Tuesday is 4.63.
For Wednesday:
Mean:
Software procedure:
Step-by-step software procedure to obtain Mean using EXCEL software is as follows:
- Open an EXCEL file.
- Enter the data in the column K in cells K1 to K10.
- In cell A1, enter the formula “=AVERAGE(K1:K10)”.
- Output using EXCEL software is given below:
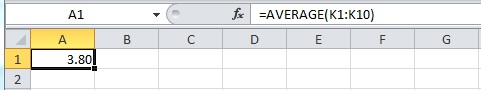
Thus, the mean number of empty seats on Wednesday is 3.80.
Median:
Software procedure:
Step-by-step software procedure to obtain Median using EXCEL software is as follows:
- Open an EXCEL file.
- Enter the data in the column K in cells K1 to K10.
- In cell A1, enter the formula “=MEDIAN(K1:K10)”.
- Output using EXCEL software is given below:
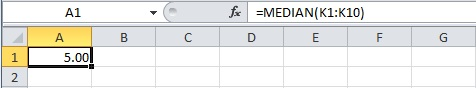
Thus, the median for the number of empty seats on Wednesday is 5.
Mode:
Software procedure:
Step-by-step software procedure to obtain Mode using EXCEL software is as follows:
- Open an EXCEL file.
- Enter the data in the column K in cells K1 to K10.
- In cell A1, enter the formula “=MODE.SNGL(K1:K10)”.
- Output using EXCEL software is given below:
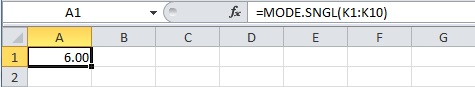
Thus, the mode for the number of empty seat on Wednesday is 6.
Geometric mean:
- Here, the data set on Wednesday contains zero, so, geometric mean is not applicable.
Midrange:
Software procedure:
Step-by-step software procedure to obtain Midrange using EXCEL software is as follows:
- Open an EXCEL file.
- Enter the data in the column K in cells K1 to K10.
- In cell A1, enter the formula “=(MIN(K1:K10)+MAX(K1:K10))/2”.
- Output using EXCEL software is given below:
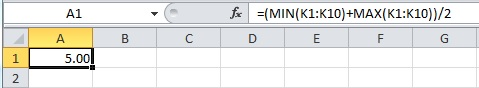
- Thus, the midrange for the number of empty seats on Wednesday is 5.
10% Trimmed mean:
Software procedure:
Step-by-step software procedure to obtain 10% Trimmed mean using EXCEL software is as follows:
- Open an EXCEL file.
- Enter the data in the column K in cells K1 to K10.
- In cell A1, enter the formula “=TRIMMEAN(K1:K10,0.2)”.
- Output using EXCEL software is given below:
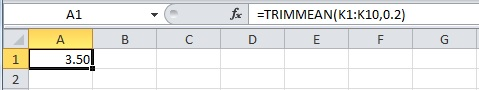
- Thus, the 10% Trimmed mean for the number of empty seats on Wednesday is 3.50.
For Thursday:
Mean:
Software procedure:
Step-by-step software procedure to obtain Mean using EXCEL software is as follows:
- Open an EXCEL file.
- Enter the data in the column L in cells L1 to L10.
- In cell A1, enter the formula “=AVERAGE(L1:L10)”.
- Output using EXCEL software is given below:
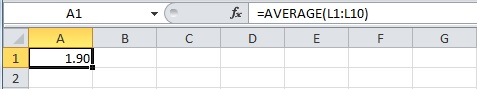
Thus, the mean number of empty seats on Thursday is 1.90.
Median:
Software procedure:
Step-by-step software procedure to obtain Median using EXCEL software is as follows:
- Open an EXCEL file.
- Enter the data in the column L in cells L1 to L10.
- In cell A1, enter the formula “=MEDIAN(L1:L10)”.
- Output using EXCEL software is given below:
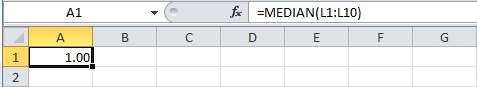
Thus, the median for the number of empty seats on Thursday is 5.
Mode:
Software procedure:
Step-by-step software procedure to obtain Mode using EXCEL software is as follows:
- Open an EXCEL file.
- Enter the data in the column L in cells L1 to L10.
- In cell A1, enter the formula “=MODE.SNGL(L1:L10)”.
- Output using EXCEL software is given below:
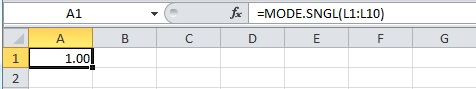
Thus, the mode for the number of empty seat on Thursday is 1.
Geometric mean:
Software procedure:
Step-by-step software procedure to obtain Geometric mean using EXCEL software is as follows:
- Open an EXCEL file.
- Enter the data in the column L in cells L1 to L10.
- In cell A1, enter the formula “=GEOMEAN(L1:L10)”.
- Output using EXCEL software is given below:
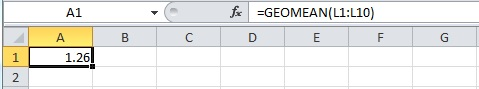
Thus, the geometric mean for the number of empty seats on Thursday is 1.26.
Midrange:
Software procedure:
Step-by-step software procedure to obtain Midrange using EXCEL software is as follows:
- Open an EXCEL file.
- Enter the data in the column L in cells L1 to L10.
- In cell A1, enter the formula “=(MIN(L1:L10)+MAX(L1:L10))/2”.
- Output using EXCEL software is given below:
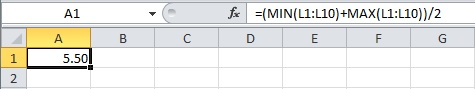
- Thus, the midrange for the number of empty seats on Thursday is 5.50.
10% Trimmed mean:
Software procedure:
Step-by-step software procedure to obtain 10% Trimmed mean using EXCEL software is as follows:
- Open an EXCEL file.
- Enter the data in the column L in cells L1 to L10.
- In cell A1, enter the formula “=TRIMMEAN(L1:L10,0.2)”.
- Output using EXCEL software is given below:
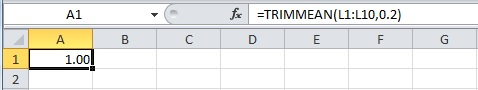
- Thus, the 10% Trimmed mean for the number of empty seats on Thursday is 1.
b.
Explain whether or not the measures of center agreed.
Answer to Problem 16SE
No, the measures of center are not agreed.
Explanation of Solution
The measures of center will not acceptable for the number of empty seats on flight 308. In this situation, the mode and geometric mean are totally different from the other measures. That is, the mean, median and the midrange for all days in a week has the similar values.
c.
Note the strength or weakness of the statistic of center.
Explanation of Solution
Strength of the statistic of the center:
Mean: it is more preferable for any statistical analysis which uses all information about the sample data.
Median: median will be more unaffected by the extreme values.
Mode: Of all the observations, mode is the undependable measure of center.
Weakness of the statistic of the center:
Mean: it is affected by the extreme values.
Median: it is affected by the gaps in the data value.
Mode: it is not helpful for continuous data.
When the strength or weakness is observed for each day, it is known that mode is the undependable for all the days. It is observed from the previous part (b), the mean and median are equal for all the days, so it acts as the strength of the statistic of center.
Want to see more full solutions like this?
Chapter 4 Solutions
Gen Combo Ll Applied Statistics In Business & Economics; Connect Access Card
- Suppose that the chance that an elementary student eats hot lunch is 30 percent. What’s the chance that, among 20 randomly selected students, between 6 and 8 students eat hot lunch (inclusive)?arrow_forwardBob’s commuting times to work are varied. He makes it to work on time 80 percent of the time. On 12 randomly selected trips to work, what’s the chance that Bob makes it on time at least 10 times?arrow_forwardYour chance of winning a small prize in a scratch-off ticket is 10 percent. You buy five tickets. What’s the chance you will win at least one prize?arrow_forward
- Suppose that 60 percent of families own a pet. You randomly sample four families. What is the chance that two or three of them own a pet?arrow_forwardIf 40 percent of university students purchase their textbooks online, in a random sample of five students, what’s the chance that exactly one of them purchased their textbooks online?arrow_forwardA stoplight is green 40 percent of the time. If you stop at this light eight random times, what is the chance that it’s green exactly five times?arrow_forward
- If 10 percent of the parts made by a certain company are defective and have to be remade, what is the chance that a random sample of four parts has one that is defective?arrow_forwardQuestion 4 Fourteen individuals were given a complex puzzle to complete. The times in seconds was recorded for their first and second attempts and the results provided below: 1 2 3 first attempt 172 255 second attempt 70 4 5 114 248 218 194 270 267 66 6 7 230 219 341 174 8 10 9 210 261 347 218 200 281 199 308 268 243 236 300 11 12 13 14 140 302 a. Calculate a 95% confidence interval for the mean time taken by each individual to complete the (i) first attempt and (ii) second attempt. [la] b. Test the hypothesis that the difference between the two mean times for both is 100 seconds. Use the 5% level of significance. c. Subsequently, it was learnt that the times for the second attempt were incorrecly recorded and that each of the values is 50 seconds too large. What, if any, difference does this make to the results of the test done in part (b)? Show all steps for the hypothesis testarrow_forwardQuestion 3 3200 students were asked about the importance of study groups in successfully completing their courses. They were asked to provide their current majors as well as their opinion. The results are given below: Major Opinion Psychology Sociology Economics Statistics Accounting Total Agree 144 183 201 271 251 1050 Disagree 230 233 254 227 218 1162 Impartial 201 181 196 234 176 988 Total 575 597 651 732 645 3200 a. State both the null and alternative hypotheses. b. Provide the decision rule for making this decision. Use an alpha level of 5%. c. Show all of the work necessary to calculate the appropriate statistic. | d. What conclusion are you allowed to draw? c. Would your conclusion change at the 10% level of significance? f. Confirm test results in part (c) using JASP. Note: All JASP input files and output tables should be providedarrow_forward
- Question 1 A tech company has acknowledged the importance of having records of all meetings conducted. The meetings are very fast paced and requires equipment that is able to capture the information in the shortest possible time. There are two options, using a typewriter or a word processor. Fifteen administrative assistants are selected and the amount of typing time in hours was recorded. The results are given below: 1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 typewriter 8.0 6.5 5.0 6.7 7.8 8.5 7.2 5.7 9.2 5.7 6.5 word processor 7.2 5.7 8.3 7.5 9.2 7.2 6.5 7.0 6.9 34 7.0 6.9 8.8 6.7 8.8 9.4 8.6 5.5 7.2 8.4 a. Test the hypothesis that the mean typing time in hours for typewriters is less than 7.0. Use the 1% level of significance. b. Construct a 90% confidence interval for the difference in mean typing time in hours, where a difference is equal to the typing time in hours of word processors minus typing time in hours of typewriter. c. Using the 5% significance level, determine whether there is…arrow_forwardIllustrate 2/7×4/5 using a rectangular region. Explain your work. arrow_forwardWrite three other different proportions equivalent to the following using the same values as in the given proportion 3 foot over 1 yard equals X feet over 5 yardsarrow_forward
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Big Ideas Math A Bridge To Success Algebra 1: Stu...AlgebraISBN:9781680331141Author:HOUGHTON MIFFLIN HARCOURTPublisher:Houghton Mifflin Harcourt
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning