
Bus and subway ridership for the summer months in London, England, is believed to be tied heavily to the number of tourists visiting the city. During the past 12 years, the data on the next page have been obtained:
a) Plot these data and decide if a linear model is reasonable.
b) Develop a regression relationship.
c) What is expected ridership if 10 million tourists visit London in a year?
d) Explain the predicted ridership if there are no tourists at all.
e) What is the standard error of the estimate?
f) What is the model’s correlation coefficient and coefficient of determination?
a)
To determine: To plot the data and decide whether the linear model is reasonable.
Introduction: Forecasting is used to predict future changes or demand patterns. It involves different approaches and varies with different periods.
Answer to Problem 52P
The graph for the given data is plotted and it can be observed that the data points are scattered around.
Explanation of Solution
Given information:
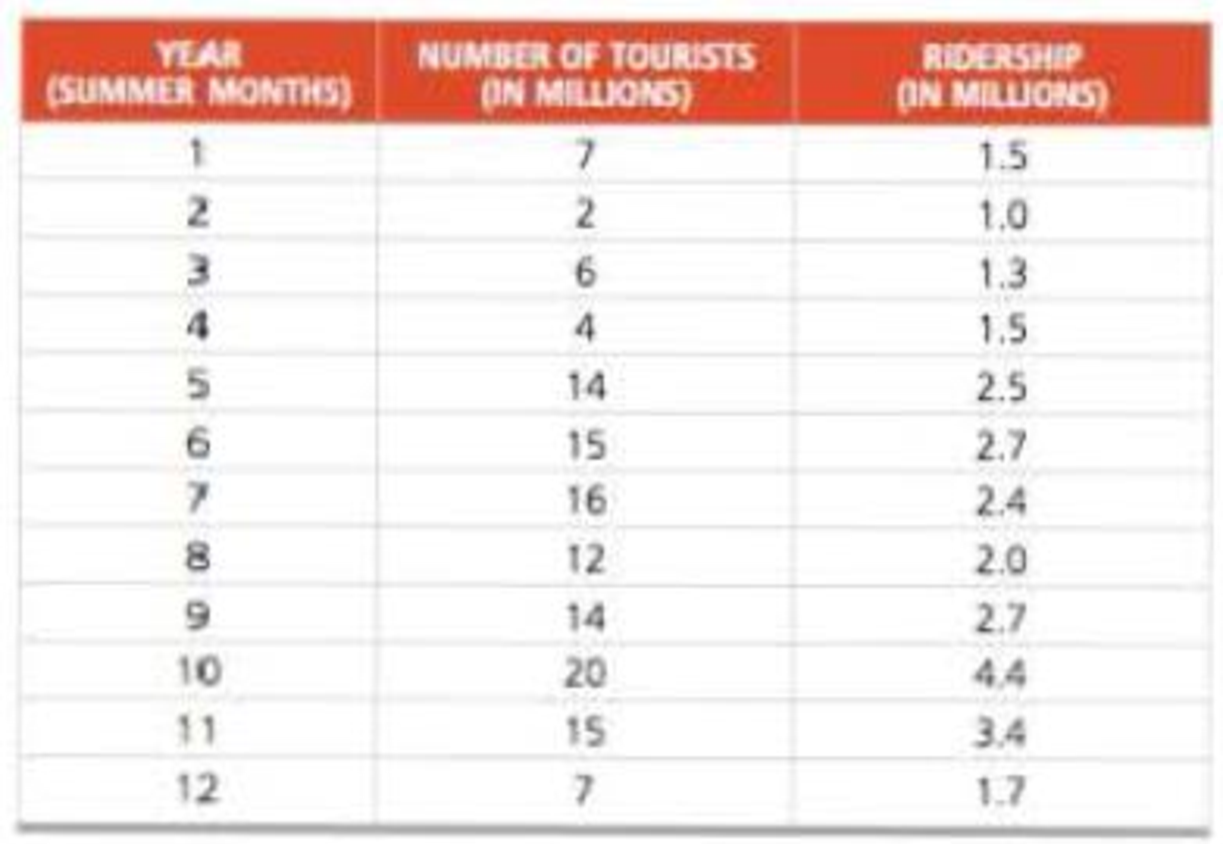
| Year (Summer Months) | Number of tourist (in millions) | Ridership (in millions) |
| 1 | 7 | 1.5 |
| 2 | 2 | 1 |
| 3 | 6 | 1.3 |
| 4 | 4 | 1.5 |
| 5 | 14 | 2.5 |
| 6 | 15 | 2.7 |
| 7 | 16 | 2.4 |
| 8 | 12 | 2 |
| 9 | 14 | 2.7 |
| 10 | 20 | 4.4 |
| 11 | 15 | 3.4 |
| 12 | 7 | 1.7 |
Table 1
Graphical representation:
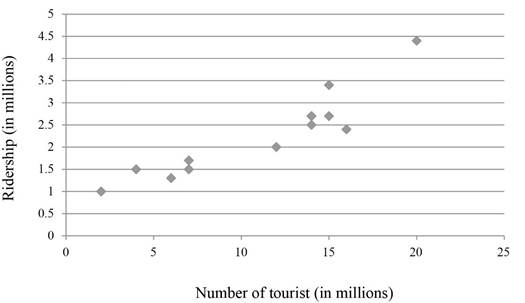
The data to plot the graph is taken from Table 1.
Hence, the graph for the given data is plotted and it can be observed that the data points are scattered around.
b)
To determine: A regression relationship.
Answer to Problem 52P
The linear regression equation is
Explanation of Solution
Given information:
| Year (Summer Months) | Number of tourist (in millions) | Ridership (in millions) |
| 1 | 7 | 1.5 |
| 2 | 2 | 1 |
| 3 | 6 | 1.3 |
| 4 | 4 | 1.5 |
| 5 | 14 | 2.5 |
| 6 | 15 | 2.7 |
| 7 | 16 | 2.4 |
| 8 | 12 | 2 |
| 9 | 14 | 2.7 |
| 10 | 20 | 4.4 |
| 11 | 15 | 3.4 |
| 12 | 7 | 1.7 |
Formula of least square regression:
Where,
Where,
| Year (Summer Months) | Number of tourist (in millions) (x) | Ridership (in millions) (y) | xy | x^2 | y^2 |
| 1 | 7 | 1.5 | 10.5 | 49 | 2.25 |
| 2 | 2 | 1 | 2 | 4 | 1 |
| 3 | 6 | 1.3 | 7.8 | 36 | 1.69 |
| 4 | 4 | 1.5 | 6 | 16 | 2.25 |
| 5 | 14 | 2.5 | 35 | 196 | 6.25 |
| 6 | 15 | 2.7 | 40.5 | 225 | 7.29 |
| 7 | 16 | 2.4 | 38.4 | 256 | 5.76 |
| 8 | 12 | 2 | 24 | 144 | 4 |
| 9 | 14 | 2.7 | 37.8 | 196 | 7.29 |
| 10 | 20 | 4.4 | 88 | 400 | 19.36 |
| 11 | 15 | 3.4 | 51 | 225 | 11.56 |
| 12 | 7 | 1.7 | 11.9 | 49 | 2.89 |
| Total | 132 | 27.1 | 352.9 | 1796 | 71.59 |
Table 2
Excel worksheet:
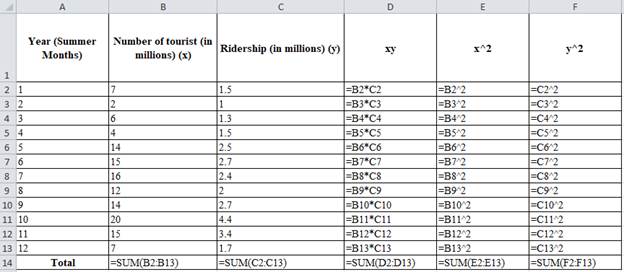
Substitute the values in the above formula.
Calculation of the average of x values
The average of x values is obtained by dividing the summation of x values with the number of periods n=12, the value of
Calculation of the average of y values
The average of y values is obtained by dividing the summation of sales with the number of periods n=12. The value of
Calculation ofthe slope of regression line‘b’:
The summation of the product of sales (y) with x values is ∑xy = 352.9, the product of number of period (n), the average of x values and the average of y values is obtained;
The summation of the square of x values, 1796, is subtracted from the product of the number of periods, 10with the average of x values, 11. The resultant value is 344. The slope of the regression line is obtained by dividing 1796 with 344. The value of ‘b’ is 0.159.
Calculation of the y-axis intercept ‘a’:
The y-axis intercept is obtained by the difference between the average of y values and values obtained by the product of the slope of regression line with the average of x values. The resultant value of ‘a’ is 0.511.
Least Square Regression forecasting equation:
Substitute the slope of regression line and they axis intercept in the regression equation which gives the liner regression equation for the data.
Hence, the linear regression equation is
c)
To determine: The expected ridership when 10 million tourists visit in a year.
Answer to Problem 52P
There is a 2.101 million ridership when 10 million tourists visit in a year.
Explanation of Solution
Given information:
| Year (Summer Months) | Number of tourist (in millions) | Ridership (in millions) |
| 1 | 7 | 1.5 |
| 2 | 2 | 1 |
| 3 | 6 | 1.3 |
| 4 | 4 | 1.5 |
| 5 | 14 | 2.5 |
| 6 | 15 | 2.7 |
| 7 | 16 | 2.4 |
| 8 | 12 | 2 |
| 9 | 14 | 2.7 |
| 10 | 20 | 4.4 |
| 11 | 15 | 3.4 |
| 12 | 7 | 1.7 |
Formula of least square regression:
Where,
Where,
Calculation of number of ridership when 10 million touristsvisit in a year:
Equation (1) provides the linear regression equation for the data and substitutes the number of tourists visiting in the regression equation. Substituting 10 million in the equation, the resultant value is found to be 2.101 million ridership.
Hence, there are 2.101 million ridership when 10 million touristsvisit in a year.
d)
To determine: The expected ridership when no tourists visit in a year.
Answer to Problem 52P
There is a 511,000 ridership when no touristsvisit in a year.
Explanation of Solution
Given information:
| Year (Summer Months) | Number of tourist (in millions) | Ridership (in millions) |
| 1 | 7 | 1.5 |
| 2 | 2 | 1 |
| 3 | 6 | 1.3 |
| 4 | 4 | 1.5 |
| 5 | 14 | 2.5 |
| 6 | 15 | 2.7 |
| 7 | 16 | 2.4 |
| 8 | 12 | 2 |
| 9 | 14 | 2.7 |
| 10 | 20 | 4.4 |
| 11 | 15 | 3.4 |
| 12 | 7 | 1.7 |
Formula of least square regression:
Where,
Where,
Calculation of the number of ridership when notouristsvisit in a year:
Equation (1) provides the linear regression equation for the data and substitutes the number of tourists visiting in the regression equation. Substituting 0 in the equation, the resultant value is 0.511 million ridership.
Hence, there is a 511,000 ridership when notouristsvisit in a year.
e)
To determine: The standard error of estimate.
Answer to Problem 52P
The standard error of estimate is0.4037.
Explanation of Solution
Given information:
| Year (Summer Months) | Number of tourists(in millions) | Ridership (in millions) |
| 1 | 7 | 1.5 |
| 2 | 2 | 1 |
| 3 | 6 | 1.3 |
| 4 | 4 | 1.5 |
| 5 | 14 | 2.5 |
| 6 | 15 | 2.7 |
| 7 | 16 | 2.4 |
| 8 | 12 | 2 |
| 9 | 14 | 2.7 |
| 10 | 20 | 4.4 |
| 11 | 15 | 3.4 |
| 12 | 7 | 1.7 |
Formula to compute the standard error of estimate:
Calculation of standard error of estimate:
The values to be substituted in the standard error of estimate formula are given inTable 2. Substitute the values from the table in the formula. This results in a standard error of estimate of 0.4037.
Hence, the standard error of estimate is 0.4037.
f)
To determine: The coefficient of correlation (r) and coefficient of determination (r2).
Answer to Problem 52P
The coefficient of correlation (r) and coefficient of determination (r2) are 717.41 & 0.840, respectively.
Explanation of Solution
Given information:
| Year (Summer Months) | Number of tourists(in millions) | Ridership (in millions) |
| 1 | 7 | 1.5 |
| 2 | 2 | 1 |
| 3 | 6 | 1.3 |
| 4 | 4 | 1.5 |
| 5 | 14 | 2.5 |
| 6 | 15 | 2.7 |
| 7 | 16 | 2.4 |
| 8 | 12 | 2 |
| 9 | 14 | 2.7 |
| 10 | 20 | 4.4 |
| 11 | 15 | 3.4 |
| 12 | 7 | 1.7 |
Formula to calculate the correlation coefficient:
Calculation of the correlation coefficient (r):
Table (2) provides the values to calculate the correlation coefficient (r).
Calculation of the correlation of determination (r2):
Hence, the coefficient of correlation and coefficient of determination are 717.41 and 0.840, respectively.
Want to see more full solutions like this?
Chapter 4 Solutions
Principles Of Operations Management
- Do you feel there is anything positive about rework?arrow_forwardDo you think technology can achieve faster setup times? How would it be implemented in the hospital workforce?arrow_forwardIn your experience or opinion, do you think process changes like organizing workspaces make a bigger difference, or is investing in technology usually the better solution for faster setups?arrow_forward
- Have you seen rework done in your business, and what was done to prevent that from occurring again?arrow_forwardResearch a company different than case studies examined and search the internet and find an example of a business that had to rework a process. How was the organization affected to rework a process in order to restore a good flow unit? Did rework hurt a process or improve the organization's operational efficiency? • Note: Include a reference with supportive citations in the discussion reply in your post.arrow_forwardSetup time is very important in affecting a process and the capacity of a process. How do you reduce setup time? Give examples of reducing setup time. Please Provide a referenecearrow_forward
- Do you think TPS was successful? If so, how? Are there other companies that have used TPS? If so, give examples. Please provide a referencearrow_forwardGiven the significant impact on finances, production timelines, and even equipment functionality, as you pointed out, what do you believe is the most effective single strategy a company can implement to significantly reduce the occurrence of rework within their operations?arrow_forwardDurban woman, Nombulelo Mkumla, took to social media last week to share how she discovered the rodent.In a lengthy Facebook post, she said she purchased the loaf of bread from a local shop after work on August 27.For the next days, Mkumla proceeded to use slices of bread from the load to make toast."Then, on the morning of August 31, I took the bread out of the fridge to make toast and noticed something disgusting andscary. I took a picture and sent it to my friends, and one of them said, 'Yi mpuku leyo tshomi' [That's a rat friend]“."I was in denial and suggested it might be something else, but the rat scenario made sense - it's possible the rat got into thebread at the factory, and no one noticed," Mkumla said.She went back to the shop she'd bought the bread from and was told to lay a complaint directly with the supplier.She sent an email with a video and photographs of the bread.Mkumla said she was later contacted by a man from Sasko who apologised for the incident.According to…arrow_forward
- PepsiCo South Africa says the incident where a woman discovered part of a rodent in her loaf of bread, is anisolated occurrence.Durban woman, Nombulelo Mkumla, took to social media last week to share how she discovered the rodent.In a lengthy Facebook post, she said she purchased the loaf of bread from a local shop after work on August 27.For the next days, Mkumla proceeded to use slices of bread from the load to make toast."Then, on the morning of August 31, I took the bread out of the fridge to make toast and noticed something disgusting andscary. I took a picture and sent it to my friends, and one of them said, 'Yi mpuku leyo tshomi' [That's a rat friend]“."I was in denial and suggested it might be something else, but the rat scenario made sense - it's possible the rat got into thebread at the factory, and no one noticed," Mkumla said.She went back to the shop she'd bought the bread from and was told to lay a complaint directly with the supplier.She sent an email with a video and…arrow_forwardDurban woman, Nombulelo Mkumla, took to social media last week to share how she discovered the rodent.In a lengthy Facebook post, she said she purchased the loaf of bread from a local shop after work on August 27.For the next days, Mkumla proceeded to use slices of bread from the load to make toast."Then, on the morning of August 31, I took the bread out of the fridge to make toast and noticed something disgusting andscary. I took a picture and sent it to my friends, and one of them said, 'Yi mpuku leyo tshomi' [That's a rat friend]“."I was in denial and suggested it might be something else, but the rat scenario made sense - it's possible the rat got into thebread at the factory, and no one noticed," Mkumla said.She went back to the shop she'd bought the bread from and was told to lay a complaint directly with the supplier.She sent an email with a video and photographs of the bread.Mkumla said she was later contacted by a man from Sasko who apologised for the incident.According to…arrow_forwardRead the project statement and answer ALL of the questions that follow PROJECT STATEMENT The African Integrated High-Speed Railway Network (AIHSRN). African nations are preparing to invest billions in a significant overhaul of their rail infrastructure as part of an ambitious plan for the continent. One of the key projects underway is the African Integrated High-Speed Railway Network (AIHSRN), which aims to connect Africa's capital cities and major commercial centres with a high-speed railway network to enhance continental trade and competition. This network will span 2,000 km (1,243 miles) and connect 60 cities, including Nairobi, Lagos, Cairo, and Dakar. It will improve access to essential markets, enhance economic cooperation, and encourage regional collaboration. The plan is poised to revolutionise intra-African trade by reducing travel times and lowering transportation costs, making trade between African nations more competitive. The trains will be capable of reaching speeds of up…arrow_forward
 Practical Management ScienceOperations ManagementISBN:9781337406659Author:WINSTON, Wayne L.Publisher:Cengage,MarketingMarketingISBN:9780357033791Author:Pride, William MPublisher:South Western Educational Publishing
Practical Management ScienceOperations ManagementISBN:9781337406659Author:WINSTON, Wayne L.Publisher:Cengage,MarketingMarketingISBN:9780357033791Author:Pride, William MPublisher:South Western Educational Publishing Contemporary MarketingMarketingISBN:9780357033777Author:Louis E. Boone, David L. KurtzPublisher:Cengage Learning
Contemporary MarketingMarketingISBN:9780357033777Author:Louis E. Boone, David L. KurtzPublisher:Cengage Learning Purchasing and Supply Chain ManagementOperations ManagementISBN:9781285869681Author:Robert M. Monczka, Robert B. Handfield, Larry C. Giunipero, James L. PattersonPublisher:Cengage Learning
Purchasing and Supply Chain ManagementOperations ManagementISBN:9781285869681Author:Robert M. Monczka, Robert B. Handfield, Larry C. Giunipero, James L. PattersonPublisher:Cengage Learning




