
Inside a distillation column (see Problem 4.8), a downward-flowing liquid and an upward-flowing vapor maintain contact with each other. For reasons we will discuss in greater detail in Chapter 6. the vapor stream becomes increasingly rich in the more volatile components of the mixture as it moves up the column, and the liquid stream is enriched in the less volatile components as it moves down. The vapor leaving the lop of the column goes to a condenser. A portion of the condensate is taken off as a product (the overhead product), and the remainder (the reflux) is returned to the top of the column to begin its downward journey as the liquid stream. The condensation process can be represented as shown below:
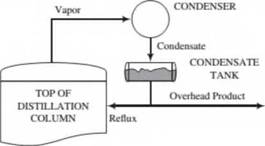
A distillation column is being used to separate a liquid mixture of ethanol (more volatile) and water (less volatile). A vapor mixture containing 89.0 moleSt ethanol and the balance water enters the overhead condenser at a rate of 100 lb-mole/h. The liquid condensate has a density of 49.0 lbnl/ft’. and
the reflux ratio is 3 lbm reflux/lbm overhead product. When the system is operating at steady state, the tank collecting the condensate is half full of liquid and the mean residence time in the tank (volume of liquid/volumetrie flow rate of liquid) is 10.0 minutes. Determine the overhead product volumetric flow rate (ft3/min) and the condenser tank volume (gal).
Trending nowThis is a popular solution!
Learn your wayIncludes step-by-step video

Chapter 4 Solutions
Elementary Principles of Chemical Processes
Additional Engineering Textbook Solutions
Java How to Program, Early Objects (11th Edition) (Deitel: How to Program)
Degarmo's Materials And Processes In Manufacturing
Computer Science: An Overview (13th Edition) (What's New in Computer Science)
Electric Circuits. (11th Edition)
Management Information Systems: Managing The Digital Firm (16th Edition)
Java: An Introduction to Problem Solving and Programming (8th Edition)
- help with this practise question on phase transformations.arrow_forwardDifferentiate between an ideal and a regular solution consisting of a mixture of A and B atoms. Which of these solutions, is likely to contain a random mixture of atoms at all temperatures? For the binary A-B ideal-solution, differentiate the equation for the configurational entropy of mixing with respect to concentration. Hence show that the slope of the free energy of mixing versus concentration curve is towards tinfinity when the mole fraction is 0 or 1. Does this make it easy or hard to purify materials? [50%] (ii) How can a phase that has a limited solubility for a particular solute be forced to accept larger concentrations which far exceed its equilibrium solubility? [20%] (iii) Atoms of A and B are arranged in a straight line at random, with the mole fraction of B equal to x. What is the probability of finding two A atoms next to each other? How would your calculation be modified if this were to be a two-dimensional array of A and B atoms? [20%] (iv) An alloy is to be made,…arrow_forwardCan the method steps be given for these questions please 10 answer given is 0.01m/s 11 answer given is 0.067e Cnm where e is charge of electron divided by volume of unit cell, giving 0.165 C/m^2 12 answer is 0.08%arrow_forward
- 3. Differentiate f(x) = x² sin(x). 4. Evaluate the limit: lim x 0 sin(2x) Xarrow_forwardDifferentiate between an ideal and a regular solution consisting of a mixture of A andB atoms. Which of these solutions, is likely to contain a random mixture of atomsat all temperatures? For the binary A-B ideal-solution, differentiate the equationfor the configurational entropy of mixing with respect to concentration. Hence showthat the slope of the free energy of mixing versus concentration curve is towards±infinity when the mole fraction is 0 or 1. Does this make it easy or hard to purifymaterials?arrow_forwardQuestion During the solidification of a binary alloy, with a positive temperature gradient in the melt, a planar solid-liquid interface is moving at the steady state, Fig. Q1(i). The variation of the solute concentration, C, in the melt ahead of the interface is given by, b) If m is the liquidus gradient, or the slope of liquidus, Fig.Q1 (iv), how does the equilibrium temperature, T, vary with the melt composition C? T₁ = C=C1+ exp R.x D (equation 1.1) T L Solid Melt (iv) T₁ S S+L where Co is the nominal solute concentration in the alloy, Ko is the equilibrium distribution coefficient, R is the solid-liquid interface moving rate, D is the solute diffusivity in the melt and x is distance into the liquid phase, Fig. Q1(ii). Answer the questions in the steps below, to show that the level of constitutional supercooling is governed by both the actual temperature, T, and the composition, C, in the solidification front. a) Consider a point in the melt at a distance x away from the solid/melt…arrow_forward
- Practise question of phase transformations topic that I need help on thank you.arrow_forwardPractise question:arrow_forwardBarium titanate can exist in both the cubic and tetragonal crystal structures. Explain why the tetragonal form exhibits piezoelectricity whereas its cubic form does not. The transformation from cubic to tetragonal BaTiO3 occurs when the former is cooled below the Curie temperature of 120 ◦C. Describe how the change can be verified experimentally based on measurements made at ambient temperature.arrow_forward
- Calleb Sandl Soda ash 5% crushing Recycling melting furnace C float bath 600 annealing Mixing Sec Packing cutting Lime Salt Care Dolomite Powder Coal Glass plant has the follownig manufacturing process in figure is the glass where the produced glass contain the following composition: SiO2 72.5%, CaO 12.3% Na20 14.0%, Fe203 0.8%, MgO 0.4% and the production capacity of the plant is 32000 ton /year and the melting furnace consist the following reaction equations: Na CO,+ aSiO, NagO aSiO + CO₂ (1) CaCOy + b$iO, → CaO - bsiO, + CO, (2) Na,SO, + cSiO2 + C-Nago cSiO, + SO₂ + CO (3) The last reaction may take place as in equations (4) or (5), and (6): Na₂SO, C Na₂SO3 + CO 2Na2SO4 + C Na,SO+cSiO, 2Na₂SO3 + CO2 - Na₂O eSiO2 + SO2 (5) (6) The ratios Na₂O/SiO, and CaO/SiO, are not molar ratios. The ratio may be of the type Na O/1.8SiO,, for example. In an ordinary window glass the molar ratios are approximately 1.5 mol Nago, I mol CaO, and 5 mol SiO,. Other glasses vary widely (Table 11.2).…arrow_forward1-A counterflow, concentric tube heat exchanger is used to cool the lubricating oil for a large industrial gas turbine engine. The flow rate of cooling water through the inner tube (Di = 25 mm) is 0.2 kg/s, while the flow rate of oil through the outer annulus (Do = 45 mm) is 0.1 kg/s. The oil and water enter at temperatures of 100 and 30 C, respectively. The exit temperature of hot fluid and cold fluid is 60 and 40 C. calculate the LMTD. Calculate the surface area of the heat exchanger. How long must the tube? Properties: unused engine oil: cp = 2131 J/kg K, μ=3.25 ×10^-2 N.s/m^2, k = 0.138 W/m.K, 4178 J/kg .K, u= 725×10^-6 N.s/m2, k = 0.625 W/m.K, Pr = 4.85 water: cp neer in which steam is condensarrow_forwardplease... please. , how can I use the boundary conditions for this question (two fixed pates and the distance between plates is h).arrow_forward
 Introduction to Chemical Engineering Thermodynami...Chemical EngineeringISBN:9781259696527Author:J.M. Smith Termodinamica en ingenieria quimica, Hendrick C Van Ness, Michael Abbott, Mark SwihartPublisher:McGraw-Hill Education
Introduction to Chemical Engineering Thermodynami...Chemical EngineeringISBN:9781259696527Author:J.M. Smith Termodinamica en ingenieria quimica, Hendrick C Van Ness, Michael Abbott, Mark SwihartPublisher:McGraw-Hill Education Elementary Principles of Chemical Processes, Bind...Chemical EngineeringISBN:9781118431221Author:Richard M. Felder, Ronald W. Rousseau, Lisa G. BullardPublisher:WILEY
Elementary Principles of Chemical Processes, Bind...Chemical EngineeringISBN:9781118431221Author:Richard M. Felder, Ronald W. Rousseau, Lisa G. BullardPublisher:WILEY Elements of Chemical Reaction Engineering (5th Ed...Chemical EngineeringISBN:9780133887518Author:H. Scott FoglerPublisher:Prentice Hall
Elements of Chemical Reaction Engineering (5th Ed...Chemical EngineeringISBN:9780133887518Author:H. Scott FoglerPublisher:Prentice Hall
 Industrial Plastics: Theory and ApplicationsChemical EngineeringISBN:9781285061238Author:Lokensgard, ErikPublisher:Delmar Cengage Learning
Industrial Plastics: Theory and ApplicationsChemical EngineeringISBN:9781285061238Author:Lokensgard, ErikPublisher:Delmar Cengage Learning Unit Operations of Chemical EngineeringChemical EngineeringISBN:9780072848236Author:Warren McCabe, Julian C. Smith, Peter HarriottPublisher:McGraw-Hill Companies, The
Unit Operations of Chemical EngineeringChemical EngineeringISBN:9780072848236Author:Warren McCabe, Julian C. Smith, Peter HarriottPublisher:McGraw-Hill Companies, The





