
Concept explainers
(a)
Interpretation:
It is to be identified whether the two structures in a given pair are constitutional isomers.
Concept introduction:
Constitutional isomers are also knows as structural isomers having the same molecular formula but differing in their connectivity. In order to identify if the two given structures are constitutional isomers, some steps needs to be followed. Start by identifying the longest continuous chain or largest ring of carbons that contains the
Answer to Problem 4.50P
The two structures in a given pair are not constitutional isomers as they have the same connectivity of atoms.
Explanation of Solution
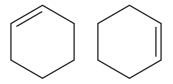
The two structures in the given pair are

Both the above structures contain the largest carbon ring consisting of six carbon atoms. The ring contains one double bond. Both the structures have the same molecular formula.
Both the structures do not have any substituent attached to the root. For cyclic structures containing
The two structures do not differ in their connectivity and, hence, are not constitutional isomers.
Constitutional isomers have the same molecular formula but differ in their connectivity.
(b)
Interpretation:
It is to be identified whether the two structures in a given pair are constitutional isomers.
Concept introduction:
Constitutional isomers are also knows as structural isomers having the same molecular formula but differing in their connectivity. In order to identify if the two given structures are constitutional isomers, some steps needs to be followed. Start by identifying the longest continuous chain or largest ring of carbons that contains the functional group. Number the carbon atoms involved in the root including the functional group such that the carbon atoms in the functional group receive the lowest possible locator numbers. Also, the substituents attached to the root get the lower possible locator numbers. If two given structures differ in the size of the longest continuous chain, ring or the numbers assigned to the carbon atoms involved in the multiple bonds, the numbers assigned to the carbon atoms to which any substituent is attached, or the identities of the substituents attached to the same-numbered carbon atoms, then the two structures must be constitutional isomers of each other. Otherwise, the molecules have the same connectivity and are not constitutional isomers.
Answer to Problem 4.50P
The two structures in a given pair are constitutional isomers as they have different connectivity of atoms.
Explanation of Solution
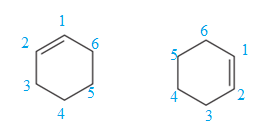
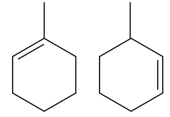
The two structures in the given pair are

Both the above structures contain the largest carbon ring consisting of six carbon atoms. The ring contains one double bond and one methyl substituent. Both the structures have the same molecular formula. For cycloalkenes, the carbon atoms bearing
For the structure on the left, the numbering can be done so as to give the lowest possible number for the substituent attached. It can be done as
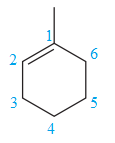
For the structure on the right, the numbering can be done so as to give the lowest possible number for the substituent attached. It can be done as
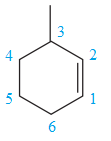
Note that the position of the substituent is different in both the structures. Thus, the two must be constitutional isomers of each other.
Constitutional isomers have the same molecular formula but differ in their connectivity.
(c)
Interpretation:
It is to be identified whether the two structures in a given pair are constitutional isomers.
Concept introduction:
Constitutional isomers are also knows as structural isomers having the same molecular formula but differing in their connectivity. In order to identify if the two given structures are constitutional isomers, some steps needs to be followed. Start by identifying the longest continuous chain or largest ring of carbons that contains the functional group. Number the carbon atoms involved in the root including the functional group such that the carbon atoms in the functional group receive the lowest possible locator numbers. Also, the substituents attached to the root get the lower possible locator numbers. If two given structures differ in the size of the longest continuous chain, ring or the numbers assigned to the carbon atoms involved in the multiple bonds, the numbers assigned to the carbon atoms to which any substituent is attached, or the identities of the substituents attached to the same-numbered carbon atoms, then the two structures must be constitutional isomers of each other. Otherwise, the molecules have the same connectivity and are not constitutional isomers.
Answer to Problem 4.50P
The two structures in a given pair are not constitutional isomers as they have the same connectivity of atoms.
Explanation of Solution
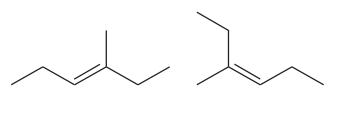
The two structures in the given pair are

The longest continuous chain of carbon atoms in each molecule has six carbons. The molecular formula for both the structures is the same. The carbon atoms are numbered 1 through 6 so that the first methyl group is encountered on C3 carbon atom as shown below:
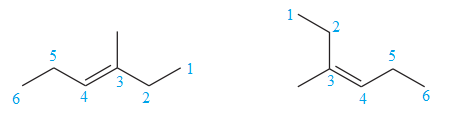
Because the methyl group is attached to C3 carbon atoms in both the molecules, the molecules have the same connectivity of atoms and therefore are not constitutional isomers.
Constitutional isomers have the same molecular formula but differ in their connectivity.
(d)
Interpretation:
It is to be identified whether the two structures in a given pair are constitutional isomers.
Concept introduction:
Constitutional isomers are also knows as structural isomers having the same molecular formula but differing in their connectivity. In order to identify if the two given structures are constitutional isomers, some steps needs to be followed. Start by identifying the longest continuous chain or largest ring of carbons that contains the functional group. Number the carbon atoms involved in the root including the functional group such that the carbon atoms in the functional group receive the lowest possible locator numbers. Also, the substituents attached to the root get the lower possible locator numbers. If two given structures differ in the size of the longest continuous chain, ring or the numbers assigned to the carbon atoms involved in the multiple bonds, the numbers assigned to the carbon atoms to which any substituent is attached, or the identities of the substituents attached to the same-numbered carbon atoms, then the two structures must be constitutional isomers of each other. Otherwise, the molecules have the same connectivity and are not constitutional isomers.
Answer to Problem 4.50P
The two structures in a given pair are not constitutional isomers as they have the same connectivity of atoms.
Explanation of Solution
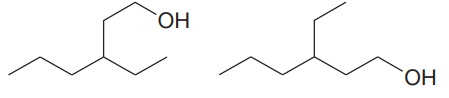
The two structures in the given pair are

The longest continuous chain of carbon atoms in each molecule has six carbons. The molecular formula for both the structures is the same. The carbon atoms are numbered 1 through 6 so that the carbon atoms to which
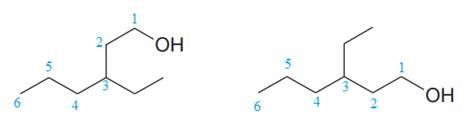
Because the ethyl group is attached to C3 carbon atoms in both the molecules, the molecules have the same connectivity of atoms and therefore are not constitutional isomers.
Constitutional isomers have the same molecular formula but differ in their connectivity.
(e)
Interpretation:
It is to be identified whether the two structures in a given pair are constitutional isomers.
Concept introduction:
Constitutional isomers are also knows as structural isomers having the same molecular formula but differing in their connectivity. In order to identify if the two given structures are constitutional isomers, some steps needs to be followed. Start by identifying the longest continuous chain or largest ring of carbons that contains the functional group. Number the carbon atoms involved in the root including the functional group such that the carbon atoms in the functional group receive the lowest possible locator numbers. Also, the substituents attached to the root get the lower possible locator numbers. If two given structures differ in the size of the longest continuous chain, ring or the numbers assigned to the carbon atoms involved in the multiple bonds, the numbers assigned to the carbon atoms to which any substituent is attached, or the identities of the substituents attached to the same-numbered carbon atoms, then the two structures must be constitutional isomers of each other. Otherwise, the molecules have the same connectivity and are not constitutional isomers.
Answer to Problem 4.50P
The two structures in a given pair are constitutional isomers as they have different connectivity of atoms.
Explanation of Solution
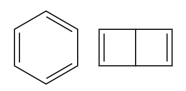
The two structures in the given pair are

Both the above structures contain the largest carbon ring consisting of six carbon atoms. The ring contains double bonds. Both the structures have the same molecular formula.
Both the structures do not have any substituent attached to the root. For cyclic structures containing
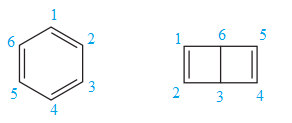
Note that there are three double bonds for the ring on the left and two for the ring on the right. The two structures differ in their connectivity and, hence, are constitutional isomers.
Constitutional isomers have the same molecular formula but differ in their connectivity.
(g)
Interpretation:
It is to be identified whether the two structures in a given pair are constitutional isomers.
Concept introduction:
Constitutional isomers are also knows as structural isomers having the same molecular formula but differing in their connectivity. In order to identify if the two given structures are constitutional isomers, some steps needs to be followed. Start by identifying the longest continuous chain or largest ring of carbons that contains the functional group. Number the carbon atoms involved in the root including the functional group such that the carbon atoms in the functional group receive the lowest possible locator numbers. Also, the substituents attached to the root get the lower possible locator numbers. If two given structures differ in the size of the longest continuous chain, ring or the numbers assigned to the carbon atoms involved in the multiple bonds, the numbers assigned to the carbon atoms to which any substituent is attached, or the identities of the substituents attached to the same-numbered carbon atoms, then the two structures must be constitutional isomers of each other. Otherwise, the molecules have the same connectivity and are not constitutional isomers.
Answer to Problem 4.50P
The two structures in a given pair are not constitutional isomers as they have different molecular formula.
Explanation of Solution
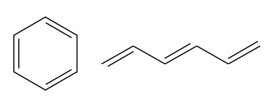
The two structures in the given pair are

Out of the two gives compounds, one is a ring of six carbon atoms, while the other is a straight chain of six carbon atoms. Though both the structures have the root with six carbon
Constitutional isomers have the same molecular formula but differ in their connectivity.
(h)
Interpretation:
It is to be identified whether the two structures in a given pair are constitutional isomers.
Concept introduction:
Constitutional isomers are also knows as structural isomers having the same molecular formula but differing in their connectivity. In order to identify if the two given structures are constitutional isomers, some steps needs to be followed. Start by identifying the longest continuous chain or largest ring of carbons that contains the functional group. Number the carbon atoms involved in the root including the functional group such that the carbon atoms in the functional group receive the lowest possible locator numbers. Also, the substituents attached to the root get the lower possible locator numbers. If two given structures differ in the size of the longest continuous chain, ring or the numbers assigned to the carbon atoms involved in the multiple bonds, the numbers assigned to the carbon atoms to which any substituent is attached, or the identities of the substituents attached to the same-numbered carbon atoms, then the two structures must be constitutional isomers of each other. Otherwise, the molecules have the same connectivity and are not constitutional isomers.
Answer to Problem 4.50P
The two structures in a given pair are constitutional isomers as they have different connectivity of atoms.
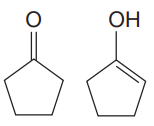
Explanation of Solution
The two structures in the given pair are

Both the above structures contain the largest carbon ring consisting of five carbon atoms. Both the structures have the same molecular formula.
The ring on the left can be numbered in such a way so that the double bonded carbon atom gets the lowest possible locator number.
The ring on the right can be numbered in such a way that each of the double bonded carbon atom gets C1 and C2 numbers. The numbering for both the structures is shown below:
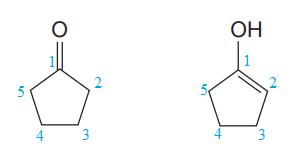
The ring on the left has
Note that the connectivity of the two structures is different.
Thus, the two must be constitutional isomers of each other.
Constitutional isomers have the same molecular formula but differ in their connectivity.
Want to see more full solutions like this?
Chapter 4 Solutions
EBK ORGANIC CHEMISTRY: PRINCIPLES AND M
- 个 CHEM&131 9267 - $25 - Intro to Mail - Hutchison, Allison (Student x Aktiv Learnin https://app.aktiv.com Draw the product of the reaction shown below. Ignore inorganic byproducts. + Na2Cr2O7 Acetone, H2SO4 Type here to search Dryng OH W Prarrow_forwardPredict the products of this organic reaction: OH + NaOH A? Specifically, in the drawing area below draw the skeletal ("line") structure of the product, or products, of this reaction. (If there's more than one product, draw them in any arrangement you like, so long as they aren't touching.) If there aren't any products because this reaction won't happen, check the No reaction box under the drawing area. No reaction Click and drag to start drawing a structure. ✓ Sarrow_forwardPredict the products of this organic reaction: CH3-C-O-CH2-CH2-C-CH3 + H₂O ? A Specifically, in the drawing area below draw the condensed structure of the product, or products, of this reaction. (If there's more than one product, draw them in any arrangement you like, so long as they aren't touching.) If there aren't any products because this reaction won't happen, check the No reaction box under the drawing area. No reaction Click anywhere to draw the first atom of your structure. :☐ darrow_forward
- DE d. Draw an arrow pushing mechanism for the following IN O CI N fo 人 P Polle DELL prt sc home end ins F5 F6 F7 F8 F9 F10 F11 F12arrow_forwardPredict the products of this organic reaction: + H₂O H* ? A Specifically, in the drawing area below draw the skeletal ("line") structure of the product, or products, of this reaction. (If there's more than one product, draw them in any arrangement you like, so long as they aren't touching.) If there aren't any products because this reaction won't happen, check the No reaction box under the drawing area. No Reaction Click and drag to start drawing a structure.arrow_forwardPredict the major organic products of the reaction below and draw them on right side of the arrow. If there will be no significant reaction, check the box below the drawing area instead. C Cl CH, OH There will be no significant reaction. + pyridine G Click and drag to start drawing a structure.arrow_forward
- What is the missing reactant in this organic reaction? H R+ H2O Δ OH 0= CH3-CH-O-CH3 + CH3-C-OH Specifically, in the drawing area below draw the condensed structure of R. If there is more than one reasonable answer, you can draw any one of them. If there is no reasonable answer, check the No answer box under the drawing area. No Answer Click anywhere to draw the first atom of your structure. dyarrow_forwardYou are trying to determine whether the following organic reaction can be done in a single synthesis step. If so, add any missing reagents or conditions in the drawing area below. If it isn't possible to do this reaction in a single synthesis step, check the box below the drawing area instead. Note for advanced students: if you have a choice of reagents to add, you should choose the least reactive and most economical reagents possible. Cl It isn't possible to do this reaction in a single synthesis step. + T OHarrow_forwardPredict the products of this organic reaction: CH3 O CH3-CH-C-O-CH2-CH2-CH3 + H₂OH+ Η ? A Specifically, in the drawing area below draw the condensed structure of the product, or products, of this reaction. (If there's more than one product, draw them in any arrangement you like, so long as they aren't touching.) If there aren't any products because this reaction won't happen, check the No reaction box under the drawing area. No Reaction Click anywhere to draw the first atom of your structure.arrow_forward
- € CH3-CH-C-O-CH2-CH2-CH3 + NaOH A? Specifically, in the drawing area below draw the condensed structure of the product, or products, of this reaction. (If there's more than one product, draw them in any arrangement you like, so long as they aren't touching.) If there aren't any products because this reaction won't happen, check the No reaction box under the drawing area. Predict the products of this organic reaction: CH3 O Click anywhere to draw the first atom of your structure. No reaction ✓ Garrow_forwardA molecule can have a temporary or permanent depending on the structure and the way the electrons can move. True Falsearrow_forwardedict the products of this organic reaction: CH3 O A CH3-CH-C-NH2 + H2O + HCI ? Specifically, in the drawing area below draw the condensed structure of the product, or products, of this reaction. If there's more than one product, draw them in any arrangement you like, so long as they aren't touching. If there aren't any products because this reaction won't happen, check the No reaction box under the drawing area. Click anywhere to draw the first atom of your structure. No Reaction planation Check 2025 McGraw Hill LLC. All Rights Reserved. Terms of Use | Privacy Center +arrow_forward
 Living By Chemistry: First Edition TextbookChemistryISBN:9781559539418Author:Angelica StacyPublisher:MAC HIGHERChemistry: Matter and ChangeChemistryISBN:9780078746376Author:Dinah Zike, Laurel Dingrando, Nicholas Hainen, Cheryl WistromPublisher:Glencoe/McGraw-Hill School Pub Co
Living By Chemistry: First Edition TextbookChemistryISBN:9781559539418Author:Angelica StacyPublisher:MAC HIGHERChemistry: Matter and ChangeChemistryISBN:9780078746376Author:Dinah Zike, Laurel Dingrando, Nicholas Hainen, Cheryl WistromPublisher:Glencoe/McGraw-Hill School Pub Co Chemistry for Today: General, Organic, and Bioche...ChemistryISBN:9781305960060Author:Spencer L. Seager, Michael R. Slabaugh, Maren S. HansenPublisher:Cengage Learning
Chemistry for Today: General, Organic, and Bioche...ChemistryISBN:9781305960060Author:Spencer L. Seager, Michael R. Slabaugh, Maren S. HansenPublisher:Cengage Learning
 Introductory Chemistry: A FoundationChemistryISBN:9781337399425Author:Steven S. Zumdahl, Donald J. DeCostePublisher:Cengage Learning
Introductory Chemistry: A FoundationChemistryISBN:9781337399425Author:Steven S. Zumdahl, Donald J. DeCostePublisher:Cengage Learning





