Chapter 3.3, Problem 36E

a)

To determine

To find Median, first quartile and third quartile for Cubs data

Answer to Problem 36E

Median = 1.8, First quartile = 1.15, Third quartile =3.1 and IQR = 1.95

Explanation of Solution

Formula:

Median:

  $Median={\left(\frac{n+1}{2}\right)}^{th}value$

First quartile Q1:

  $Q_1=\frac{1}{4}{(n+1)}^{th}value$

Third quartile Q3:

  $Q_3=\frac{3}{4}{(n+1)}^{th}value$

IQR:

  $IQR=Q_3-Q_1$

Calculation:

Data sorted in ascending order:

$x$
0.3	1.2	1.8	3.1
0.5	1.2	1.8	3.1
0.5	1.2	1.9	3.3
0.6	1.2	2.1	3.3
0.6	1.3	2.1	3.4
0.9	1.3	2.1	3.5
0.9	1.3	2.2	3.5
0.9	1.3	2.4	3.5
0.9	1.4	2.5	3.6
1	1.4	2.5	3.7
1.1	1.5	2.6	3.8
1.1	1.5	2.7	4
1.1	1.5	2.7	4.2
1.1	1.6	2.7	4.6
1.1	1.6	3	5.9
1.1	1.6	3.1	6.6
			6.6

Here, n = 65

For finding outlier, first need to find First quartile, Third quartile and IQR.

Median:

  $\begin{array}{l}Median={\left(\frac{n+1}{2}\right)}^{th}value\\ Median={\left(\frac{65+1}{2}\right)}^{th}value\\ Median={\left(33\right)}^{th}value\\ Median=1.8\end{array}$

First Quartile:

  $\begin{array}{l}$ Q_1=\frac{1}{4}{(65+1)}^{th}value$$ Q_1=\frac{1}{4}{(66)}^{th}value$$ Q_1={(16.5)}^{th}value$Q_1=1.1+0.5\times (1.2-1.1)\\ Q_1=1.15\end{array}$

First quartile is 1.15

Third quartile:

  $\begin{array}{l}$ Q_3=\frac{3}{4}{(n+1)}^{th}value$$ Q_3=\frac{3}{4}{(65+1)}^{th}value$$ Q_3=\frac{3}{4}{(66)}^{th}value$$ Q_3={(49.5)}^{th}value$Q_3=3.1+0.5\times (3.1-3.1)\\ Q_3=3.1\end{array}$

Third quartile is 3.1

  $\begin{array}{l}IQR=Q_3-Q_1\hfill \\ IQR=\text{ 3}\text{.1-1}\text{.15}\hfill \\ IQR=\text{ 1}\text{.95}\hfill \end{array}$

b)

To determine

To find Median, first quartile, third quartile and the IQR for Indians data.

Answer to Problem 36E

Median = 5.6, First quartile = 2.7, Third quartile =6.5 and IQR = 3.8

Explanation of Solution

Formula:

Median:

  $Median={\left(\frac{n+1}{2}\right)}^{th}value$

First quartile Q1:

  $Q_1=\frac{1}{4}{(n+1)}^{th}value$

Third quartile Q3:

  $Q_3=\frac{3}{4}{(n+1)}^{th}value$

IQR:

  $IQR=Q_3-Q_1$

Calculation:

Data sorted in ascending order:

$y$
1.1	3.8	6.3
1.5	3.8	6.3
1.8	4.4	6.5
1.9	4.4	6.5
2	4.7	7.3
2.1	5.3	7.6
2.1	5.3	8.7
2.4	5.6	8.9
2.7	5.6	9.1
2.9	5.6	9.2
3.3	5.8	9.5
3.6	5.9

Here, n = 35

For finding outlier, first need to find First quartile, Third quartile and IQR.

  $\begin{array}{l}Median={\left(\frac{35+1}{2}\right)}^{th}value\\ Median={\left(\frac{36}{2}\right)}^{th}value\\ Median={\left(18\right)}^{th}value\\ Median=5.6\end{array}$

First Quartile:

  $\begin{array}{l}$ Q_1=\frac{1}{4}{(35+1)}^{th}value$$ Q_1=\frac{1}{4}{(36)}^{th}value$$ Q_1={(9)}^{th}value$Q_1=2.7\end{array}$

First quartile is 2.7

Third quartile:

  $\begin{array}{l}$ Q_3=\frac{3}{4}{(n+1)}^{th}value$$ Q_3=\frac{3}{4}{(35+1)}^{th}value$$ Q_3=\frac{3}{4}{(36)}^{th}value$$ Q_3={(27)}^{th}value$Q_3=6.5\end{array}$

Third quartile is 6.5

  $\begin{array}{l}IQR=Q_3-Q_1\hfill \\ IQR=\text{ 6}\text{.5-2}\text{.7}\hfill \\ IQR=\text{ 3}\text{.8}\hfill \end{array}$

c)

To determine

To find upper and lower outlier limits for sea level

Answer to Problem 36E

Lower outlier limit= -1.775 and upper limit = 6.025

Explanation of Solution

Outliers are those values which are less than Q1-1.5 x IQR and greater than Q3+1.5 x IQR.

Here

Lower outlier boundary is $1.15-1.5\times 1.95=-1.775$

Upper outlier boundary is $3.1+1.5\times 1.95=6.025$

d)

To determine

To find upper and lower outlier limits for sea level.

Answer to Problem 36E

Lower outlier limit= -3.38 and upper limit = 12.2

Explanation of Solution

Outliers are those values which are less than Q1-1.5 x IQR and greater than Q3+1.5 x IQR.

Here,

Lower outlier boundary is $2.7-1.5\times 3.8=-3.38$

Upper outlier boundary is $6.5+1.5\times 3.8=12.2$

e)

To determine

To construct box plot for both data set.

Explanation of Solution

Box plot for a data set of sea level:

  

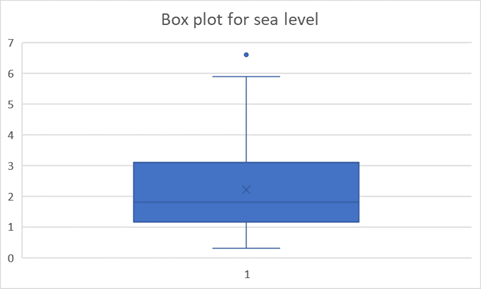
From above Boxplot, it is concluded that 6.6 is the outlier values which is greater than upper outlier limit.

Box plot for a data set High Attitude:

  

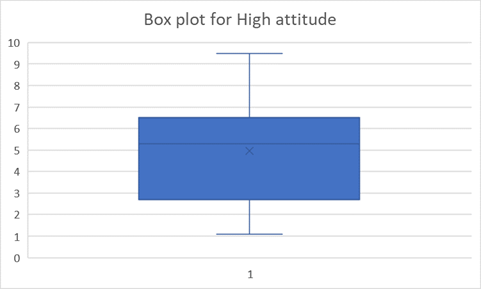
From above Boxplot, it is concluded that, there are no any outlier value in the data set.