
Obtaining exact, or approximate, expressions for eigenvalues and eigenvectors in terms of the model parameters is often useful for understanding the qualitative behavior of solutions to a dynamical system. We illustrate using Example
(a) Show that the general solution of Eqs.
where
(b) Assuming that
(c) Show that approximations to the corresponding eigenvectors are
(d) Use the approximations obtained in parts (b) and (c) and the equilibrium solution
(e) Show that when
where
(f) Give a physical explanation of the significance of the eigenvalues on the dynamical behavior of the solution
Example
Consider the schematic diagram of the greenhouse/rockbed system in Figure
Rocks are a good material for storing heat since they have a high energy-storage capacity, are inexpensive, and have a long life.
Using
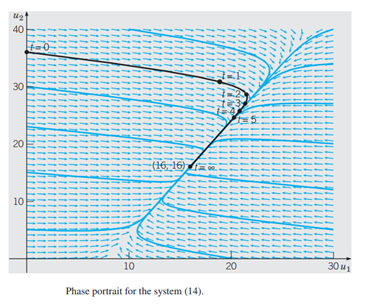
Section
Find the equilibrium solution, or critical point, of Eqs.
In the limiting case,
Thus, if initial conditions
Want to see the full answer?
Check out a sample textbook solution
Chapter 3 Solutions
DIFFERENTIAL EQUATIONS(LL) W/WILEYPLUS
Additional Math Textbook Solutions
Basic Business Statistics, Student Value Edition
Thinking Mathematically (6th Edition)
College Algebra with Modeling & Visualization (5th Edition)
A First Course in Probability (10th Edition)
Precalculus: Mathematics for Calculus (Standalone Book)
Elementary Statistics: Picturing the World (7th Edition)
- Q1. A group of five applicants for a pair of identical jobs consists of three men and two women. The employer is to select two of the five applicants for the jobs. Let S denote the set of all possible outcomes for the employer's selection. Let A denote the subset of outcomes corresponding to the selection of two men and B the subset corresponding to the selection of at least one woman. List the outcomes in A, B, AUB, AN B, and An B. (Denote the different men and women by M₁, M2, M3 and W₁, W2, respectively.)arrow_forwardFor the following function, find the full power series centered at a of convergence. 0 and then give the first 5 nonzero terms of the power series and the open interval = f(2) Σ 8 1(x)--(-1)*(3)* n=0 ₤(x) = + + + ++... The open interval of convergence is: 1 1 3 f(x)= = 28 3x6 +1 (Give your answer in help (intervals) .)arrow_forwardQ3 (8 points) Q3. A survey classified a large number of adults according to whether they were diag- nosed as needing eyeglasses to correct their reading vision and whether they use eyeglasses when reading. The proportions falling into the four resulting categories are given in the following table: Use Eyeglasses for Reading Needs glasses Yes No Yes 0.44 0.14 No 0.02 0.40 If a single adult is selected from the large group, find the probabilities of the events defined below. The adult (a) needs glasses. (b) needs glasses but does not use them. (c) uses glasses whether the glasses are needed or not.arrow_forward
- 4. (i) Let a discrete sample space be given by N = {W1, W2, W3, W4}, and let a probability measure P on be given by P(w1) = 0.2, P(w2) = 0.2, P(w3) = 0.5, P(wa) = 0.1. Consider the random variables X1, X2 → R defined by X₁(w1) = 1, X₁(w2) = 2, X2(w1) = 2, X2 (w2) = 2, Find the joint distribution of X1, X2. (ii) X1(W3) = 1, X₁(w4) = 1, X2(W3) = 1, X2(w4) = 2. [4 Marks] Let Y, Z be random variables on a probability space (, F, P). Let the random vector (Y, Z) take on values in the set [0, 1] x [0,2] and let the joint distribution of Y, Z on [0, 1] x [0,2] be given by 1 dPy,z (y, z) ==(y²z+yz2) dy dz. harks 12 Find the distribution Py of the random variable Y. [8 Marks]arrow_forwardNeed help answering wuestionarrow_forwardFor the following function, find the full power series centered at x = 0 and then give the first 5 nonzero terms of the power series and the open interval of convergence. f(x) = Σ| n=0 9 f(x) = 6 + 4x f(x)− + + + ++··· The open interval of convergence is: ☐ (Give your answer in help (intervals) .)arrow_forward
- marks 11 3 3/4 x 1/4 1. There are 4 balls in an urn, of which 3 balls are white and 1 ball is black. You do the following: draw a ball from the urn at random, note its colour, do not return the ball to the urn; draw a second ball, note its colour, return the ball to the urn; finally draw a third ball and note its colour. (i) Describe the corresponding discrete probability space (Q, F, P). [9 Marks] (ii) Consider the following event, A: Among the first and the third balls, one ball is white, the other is black. Write down A as a subset of the sample space and find its probability, P(A). [2 Marks]arrow_forwardThere are 4 balls in an urn, of which 3 balls are white and 1 ball isblack. You do the following:• draw a ball from the urn at random, note its colour, do not return theball to the urn;• draw a second ball, note its colour, return the ball to the urn;• finally draw a third ball and note its colour.(i) Describe the corresponding discrete probability space(Ω, F, P). [9 Marks](ii) Consider the following event,A: Among the first and the third balls, one ball is white, the other is black.Write down A as a subset of the sample space Ω and find its probability, P(A)arrow_forwardLet (Ω, F, P) be a probability space and let X : Ω → R be a randomvariable whose probability density function is given by f(x) = 12 |x|e−|x| forx ∈ R.(i) Find the characteristic function of the random variable X.[8 Marks](ii) Using the result of (i), calculate the first two moments of therandom variable X, i.e., E(Xn) for n = 1, 2. [6 Marks]Total marks 16 (iii) What is the variance of X?arrow_forward
- Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
