EBK PHYSICS FOR SCIENTISTS AND ENGINEER
9th Edition
ISBN: 8220100581557
Author: Jewett
Publisher: Cengage Learning US
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 32, Problem 32.78CP
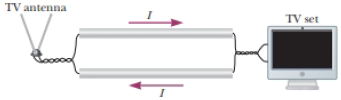
In earlier times when many households received nondigital television signals from an antenna, the lead-in wires from the antenna were often constructed in the form of two parallel wires (Fig. P31.50). The two wires carry currents of equal magnitude in opposite directions. The center-to-center separation of the wires is w, and a is their radius. Assume w is large enough compared with a that the wires carry the current uniformly distributed over their surfaces and negligible magnetic field exists inside the wires. (a) Why does this configuration of conductors have an inductance? (b) What constitutes the flux loop for this configuration? (c) Show that the inductance of a length x of this type of lead-in is
Figure P31.50
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
A long straight wire is held fixed in a horizontal position. A second parallel wire is 2.6 mm below the first but is free to fall
under its own weight. The second wire is copper (density 8920 kg/m3), with diameter 1.6 mm. What equal current in both
wires will suspend the lower wire against gravity
Question #1. Consider a current-carrying circular wire with radius r. Find the angle between the infinitesimal length dl and some distance h?
+z
dl
h
dB
+y
y
Current-carrying circular wire
Note: radius r is perpendicular to the screen
Select one:
O 90°
O 0°
O 180°
O 270°
In earlier times when many households received nondigital
television signals from an antenna, the lead-in wires from
the antenna were often constructed in the form of two paral-
lel wires (Fig. P31.50). The two wires carry currents of equal
magnitude in opposite directions. The center-to-center
separation of the wires is w, and a is their radius. Assume
w is large enough compared with a that the wires carry the
current uniformly distributed over their surfaces and negli-
gible magnetic field exists inside the wires. (a) Why does this
configuration of conductors have an inductance? (b) What
TV antenna
TV set
Figure P31.50
constitutes the flux loop for this configuration? (c) Show
that the inductance of a length x of this type of lead-in is
w -
a
L
In
a
Chapter 32 Solutions
EBK PHYSICS FOR SCIENTISTS AND ENGINEER
Ch. 32 - A coil with zero resistance has its ends labeled a...Ch. 32 - Prob. 32.2QQCh. 32 - Prob. 32.3QQCh. 32 - Prob. 32.4QQCh. 32 - (i) At an instant of time during the oscillations...Ch. 32 - Prob. 32.1OQCh. 32 - Prob. 32.2OQCh. 32 - Prob. 32.3OQCh. 32 - In Figure OQ32.4, the switch is left in position a...Ch. 32 - Prob. 32.5OQ
Ch. 32 - Prob. 32.6OQCh. 32 - Prob. 32.7OQCh. 32 - Prob. 32.1CQCh. 32 - Prob. 32.2CQCh. 32 - A switch controls the current in a circuit that...Ch. 32 - Prob. 32.4CQCh. 32 - Prob. 32.5CQCh. 32 - Prob. 32.6CQCh. 32 - The open switch in Figure CQ32.7 is thrown closed...Ch. 32 - After the switch is dosed in the LC circuit shown...Ch. 32 - Prob. 32.9CQCh. 32 - Discuss the similarities between the energy stored...Ch. 32 - Prob. 32.1PCh. 32 - Prob. 32.2PCh. 32 - Prob. 32.3PCh. 32 - Prob. 32.4PCh. 32 - An emf of 24.0 mV Ls induced in a 500-turn coil...Ch. 32 - Prob. 32.6PCh. 32 - Prob. 32.7PCh. 32 - Prob. 32.8PCh. 32 - Prob. 32.9PCh. 32 - Prob. 32.10PCh. 32 - Prob. 32.11PCh. 32 - A toroid has a major radius R and a minor radius r...Ch. 32 - Prob. 32.13PCh. 32 - Prob. 32.14PCh. 32 - Prob. 32.15PCh. 32 - Prob. 32.16PCh. 32 - Prob. 32.17PCh. 32 - Prob. 32.18PCh. 32 - Prob. 32.19PCh. 32 - When the switch in Figure P32.18 is closed, the...Ch. 32 - Prob. 32.21PCh. 32 - Show that i = Iiet/ is a solution of the...Ch. 32 - Prob. 32.23PCh. 32 - Consider the circuit in Figure P32.18, taking =...Ch. 32 - Prob. 32.25PCh. 32 - The switch in Figure P31.15 is open for t 0 and...Ch. 32 - Prob. 32.27PCh. 32 - Prob. 32.28PCh. 32 - Prob. 32.29PCh. 32 - Two ideal inductors, L1 and L2, have zero internal...Ch. 32 - Prob. 32.31PCh. 32 - Prob. 32.32PCh. 32 - Prob. 32.33PCh. 32 - Prob. 32.34PCh. 32 - Prob. 32.35PCh. 32 - Complete the calculation in Example 31.3 by...Ch. 32 - Prob. 32.37PCh. 32 - A flat coil of wire has an inductance of 40.0 mH...Ch. 32 - Prob. 32.39PCh. 32 - Prob. 32.40PCh. 32 - Prob. 32.41PCh. 32 - Prob. 32.42PCh. 32 - Prob. 32.43PCh. 32 - Prob. 32.44PCh. 32 - Prob. 32.45PCh. 32 - Prob. 32.46PCh. 32 - In the circuit of Figure P31.29, the battery emf...Ch. 32 - A 1.05-H inductor is connected in series with a...Ch. 32 - A 1.00-F capacitor is charged by a 40.0-V power...Ch. 32 - Calculate the inductance of an LC circuit that...Ch. 32 - An LC circuit consists of a 20.0-mH inductor and a...Ch. 32 - Prob. 32.52PCh. 32 - Prob. 32.53PCh. 32 - Prob. 32.54PCh. 32 - An LC circuit like the one in Figure CQ32.8...Ch. 32 - Show that Equation 32.28 in the text Ls Kirchhoffs...Ch. 32 - In Figure 31.15, let R = 7.60 , L = 2.20 mH, and C...Ch. 32 - Consider an LC circuit in which L = 500 mH and C=...Ch. 32 - Electrical oscillations are initiated in a series...Ch. 32 - Review. Consider a capacitor with vacuum between...Ch. 32 - Prob. 32.61APCh. 32 - An inductor having inductance I. and a capacitor...Ch. 32 - A capacitor in a series LC circuit has an initial...Ch. 32 - Prob. 32.64APCh. 32 - When the current in the portion of the circuit...Ch. 32 - At the moment t = 0, a 24.0-V battery is connected...Ch. 32 - Prob. 32.67APCh. 32 - Prob. 32.68APCh. 32 - Prob. 32.69APCh. 32 - At t = 0, the open switch in Figure P31.46 is...Ch. 32 - Prob. 32.71APCh. 32 - Prob. 32.72APCh. 32 - Review. A novel method of storing energy has been...Ch. 32 - Prob. 32.74APCh. 32 - Review. The use of superconductors has been...Ch. 32 - Review. A fundamental property of a type 1...Ch. 32 - Prob. 32.77APCh. 32 - In earlier times when many households received...Ch. 32 - Assume the magnitude of the magnetic field outside...Ch. 32 - Prob. 32.80CPCh. 32 - To prevent damage from arcing in an electric...Ch. 32 - One application of an RL circuit is the generation...Ch. 32 - Prob. 32.83CP
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.Similar questions
- Chapter 32, Problem 006 A capacitor with square plates of edge length L is being discharged by a current of 0.59 A. The figure is a head-on view of one of the plates from inside the capacitor. A dashed rectangular path is shown. If L = 8.3 cm, W = 5.1 cm, and H = 2.4 cm, what is the value of ф8-ds around the dashed path?arrow_forwardCoaxial cables are used extensively for cable television and other electronic applications. A coaxial cable consists of two concentric cylindrical conductors. The region between the conductors is completely filled with polyethylene plastic as shown in 26.8a. Current leakage through the plastic, in the radial direction, is unwanted. (The cable is designed to conduct current along its length, but that is not the current being considered here.) The radius of the inner conductor is a = 0.500 cm, the radius of the outer conductor is b = 1.75 cm, and the length is L = 15.0 cm. The resistivity of the plastic is 1.0 x 1013 Ω ⋅ m. Calculate the radial resistance of the plastic between the two conductors.arrow_forwardA portion of a long, cylindrical coaxial cable is shown in the figure below. An electrical current I = 3.0 amps flows down the center conductor, and this same current is returned in the outer conductor. Assume the current is distributed uniformly over the cross sections of the two parts of the cable. The values of the radii in the figure are r1 = 1.5 mm, r2 = 4.0 mm, and r3 = 7.0 mm. Using Ampere’s Law, find the magnitude of the magnetic field at the following distances from the center of the inner wire: a. 1.0 mm. b. 3.0 mm. c. 5.5 mm. d. 9.0 mm.arrow_forward
- E13P5arrow_forwardTwo very long coaxial cylindrical conductors are shown in cross-section below. The inner cylinder has radius a = 2 cm and caries a total current of I1 = 1.0 A in the positive z-direction (pointing out of the page). The outer cylinder has an inner radius b = 4 cm, outer radius c = 6 cm and carries a current of I2 = 2.0 A in the negative z-direction (pointing into the page). You may assume that the current is uniformly distributed over the cross-sectional area of the conductors. a) Calculate the magnitude and direction of the magnetic field at a radius of r=20 cm. b) Calculate the magnitude and direction of the magnetic field at a radius of r=3 cm.arrow_forwardA closed curve encircles several conductors. The line integral PB.dL around this curve is 3,83x104 T.m (a) What is the net current in the conductors? (b) If you were to integrate around the curve in the opposite direction, what would be the value of the line integral? Select one: lenci=D 305 A, 0.0 T.m lencl = 502 A, -3.83 x 10 4T.m lenci = 502 A, -7.66 x 104 T.m lencl = 600 A, -7.66 x 104T.m lencl = 305 A, -3.83 x 10 4 T.m lencl = 502 A, 0.0 T.m %3Darrow_forward
- Problem 1: Two long thin parallel wires are 0.1 m apart and each wire carries a current of 12 A. The current for the wire on the left is into the page and the current for the wire on the right is out of the page. What is B at point P, 0.08 m from one wire and 0.06 m from the other wire? Hint: Point P is on the corner of the right triangle with the right angle. Answer: B = −(1.4 × 10−5 T)î − (4.8 × 10-5 T)ĵ. 0.08 m - X 0.1 m- I -0.06 marrow_forwardBiot-savart lawarrow_forwardHELPPParrow_forward
- The xy-plane serves as the interface between two different media. Medium 1 (z 0) is filled with a material whose H, = 4. If the interface carries current (1/H) a, mA/m, and B, = 5a, + 8a, mWb/m², find H, and B,. a12 =a, K O H1 =6arrow_forwardA long straight wire in the z-axis carries a current of 6.0 A in the positive z direction, and a circular loop of 10 cm radius in the xy-plane also carries 1.0-A current as shown in the figure. Point P in the center of the ring is 25 cm from the z-axis. An electron is ejected from P at a velocity of 1.0 × 106 m / s in the negative x direction. What is the y component of the force acting on the electron? (e = 1.60 × 10-19 C, μ0 = 4π × 10-7 T m / A)arrow_forward7. A sheet of copper foil 1 mm thick, 5 cm wide and 1.75 m long is connected to a voltage source as shown. There is a magnetic field B = 2.5 T into the page. Assume copper has conductivity σ = 5.96 x 107 mho/m and a charge carrier density n = 1028 electrons/m³. a) What is the current density J in the foil? b) What is the Hall voltage as measured by the voltmeter? c) Which point is at a higher potential, a or b? Voltmeter 20 Varrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...
Physics
ISBN:9781133939146
Author:Katz, Debora M.
Publisher:Cengage Learning
Principles of Physics: A Calculus-Based Text
Physics
ISBN:9781133104261
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
What is Electromagnetic Induction? | Faraday's Laws and Lenz Law | iKen | iKen Edu | iKen App; Author: Iken Edu;https://www.youtube.com/watch?v=3HyORmBip-w;License: Standard YouTube License, CC-BY