Calculus: Early Transcendentals (2nd Edition)
2nd Edition
ISBN: 9780321947345
Author: William L. Briggs, Lyle Cochran, Bernard Gillett
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 3.11, Problem 29E
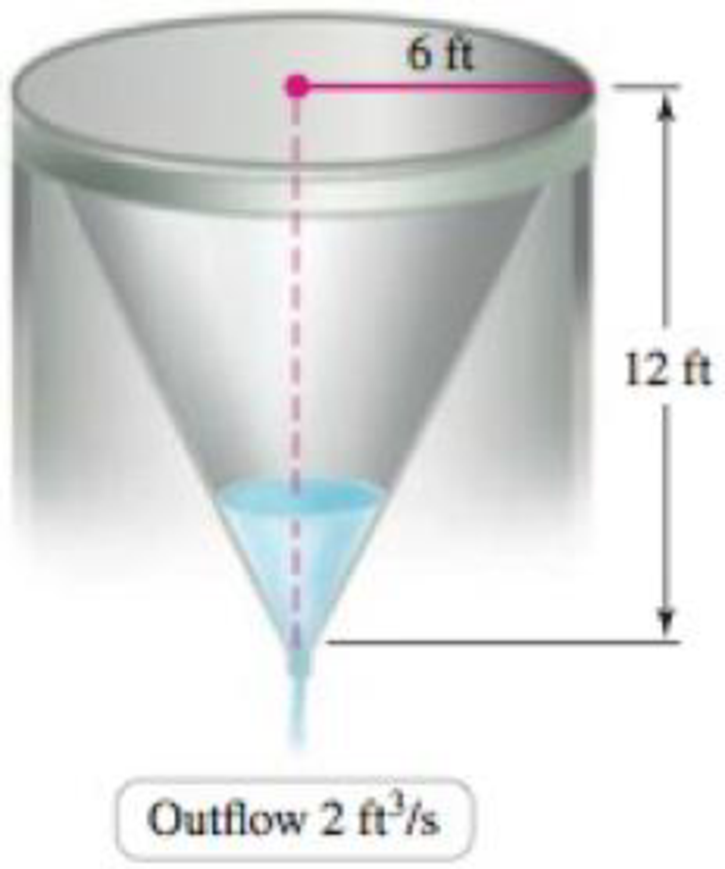
Draining a tank An inverted conical water tank with a height of 12 ft and a radius of 6 ft is drained through a hole in the vertex at a rate of 2 ft3/s (see figure). What is the rate of change of the water depth when the water depth is 3 ft? (Hint: Use similar triangles.)
Expert Solution & Answer
Learn your wayIncludes step-by-step video
schedule05:19
Students have asked these similar questions
A homeware company has been approached to manufacture a cake tin in the shape
of a "ghost" from the Pac-Man video game to celebrate the 45th Anniversary of the
games launch. The base of the cake tin has a characteristic dimension / and is
illustrated in Figure 1 below, you should assume the top and bottom of the shape
can be represented by semi-circles. The vertical sides of the cake tin have a height of
h. As the company's resident mathematician, you need to find the values of r and h
that minimise the internal surface area of the cake tin given that the volume of the
tin is Vfixed-
2r
Figure 1 - Plan view of the "ghost" cake tin base.
(a) Show that the Volume (V) of the cake tin as a function of r and his
2(+1)²h
V = 2
15. Please solve this and show each and every step please. PLEASE no chatgpt can I have a real person solve it please!! I am stuck. I am doing pratice problems and I do not even know where to start with this. The question is Please compute the indicated functional value.
Use a graph of f to estimate lim f(x) or to show that the limit does not exist. Evaluate f(x) near x = a to support your conjecture. Complete parts (a) and (b).
x-a
f(x)=
1 - cos (4x-4)
3(x-1)²
; a = 1
a. Use a graphing utility to graph f. Select the correct graph below..
A.
W
→
✓
Each graph is displayed in a [- 1,3] by [0,5] window.
B.
in
✓
○ C.
und
☑
Use the graphing utility to estimate lim f(x). Select the correct choice below and, if necessary, fill in the answer box to complete your choice.
x-1
○ A. The limit appears to be approximately ☐ .
(Round to the nearest tenth as needed.)
B. The limit does not exist.
b. Evaluate f(x) for values of x near 1 to support your conjecture.
X
0.9
0.99
0.999
1.001
1.01
1.1
f(x)
○ D.
+
☑
(Round to six decimal places as needed.)
Does the table from the previous step support your conjecture?
A. No, it does not. The function f(x) approaches a different value in the table of values than in the graph, after the approached values are rounded to the…
Chapter 3 Solutions
Calculus: Early Transcendentals (2nd Edition)
Ch. 3.1 - Use definition (1) (p. 127) for the slope of a...Ch. 3.1 - Explain why the slope of a secant line can be...Ch. 3.1 - Explain why the slope of the tangent line can be...Ch. 3.1 - Prob. 4ECh. 3.1 - Given a function f and a point a in its domain,...Ch. 3.1 - Explain the relationships among the slope of a...Ch. 3.1 - Prob. 7ECh. 3.1 - Prob. 8ECh. 3.1 - Equations of tangent lines by definition (1) a....Ch. 3.1 - Equations of tangent lines by definition (1) a....
Ch. 3.1 - Equations of tangent lines by definition (1) a....Ch. 3.1 - Prob. 12ECh. 3.1 - Equations of tangent lines by definition (1) a....Ch. 3.1 - Equations of tangent lines by definition (1) a....Ch. 3.1 - Equations of tangent lines by definition (2) a....Ch. 3.1 - Equations of tangent lines by definition (2) a....Ch. 3.1 - Equations of tangent lines by definition (2) a....Ch. 3.1 - Equations of tangent lines by definition (2) a....Ch. 3.1 - Equations of tangent lines by definition (2) a....Ch. 3.1 - Equations of tangent lines by definition (2) a....Ch. 3.1 - Equations of tangent lines by definition (2) a....Ch. 3.1 - Equations of tangent lines by definition (2) a....Ch. 3.1 - Equations of tangent lines by definition (2) a....Ch. 3.1 - Equations of tangent lines by definition (2) a....Ch. 3.1 - Equations of tangent lines by definition (2) a....Ch. 3.1 - Prob. 26ECh. 3.1 - Derivatives and tangent lines a. For the following...Ch. 3.1 - Derivatives and tangent lines a. For the following...Ch. 3.1 - Derivatives and tangent lines a. For the following...Ch. 3.1 - Derivatives and tangent lines a. For the following...Ch. 3.1 - Derivatives and tangent lines a. For the following...Ch. 3.1 - Derivatives and tangent lines a. For the following...Ch. 3.1 - Derivatives and tangent lines a. For the following...Ch. 3.1 - Derivatives and tangent lines a. For the following...Ch. 3.1 - Derivatives and tangent lines a. For the following...Ch. 3.1 - Derivatives and tangent lines a. For the following...Ch. 3.1 - Prob. 37ECh. 3.1 - Prob. 38ECh. 3.1 - Prob. 39ECh. 3.1 - Prob. 40ECh. 3.1 - A derivative formula a. Use the definition of the...Ch. 3.1 - A derivative formula a. Use the definition of the...Ch. 3.1 - Prob. 43ECh. 3.1 - Prob. 44ECh. 3.1 - Prob. 45ECh. 3.1 - Prob. 46ECh. 3.1 - Explain why or why not Determine whether the...Ch. 3.1 - Slope of a line Consider the line f(x) = mx + b,...Ch. 3.1 - Calculating derivatives a. For the following...Ch. 3.1 - Calculating derivatives a. For the following...Ch. 3.1 - Calculating derivatives a. For the following...Ch. 3.1 - Calculating derivatives a. For the following...Ch. 3.1 - Analyzing slopes Use the points A, B, C, D, and E...Ch. 3.1 - Analyzing slopes Use the points A, B, C, D, and E...Ch. 3.1 - Power and energy Energy is the capacity to do...Ch. 3.1 - Population of Las Vegas Let p(t) represent the...Ch. 3.1 - Find the function The following limits represent...Ch. 3.1 - Find the function The following limits represent...Ch. 3.1 - Find the function The following limits represent...Ch. 3.1 - Find the function The following limits represent...Ch. 3.1 - Prob. 61ECh. 3.1 - Looking ahead: Derivative of xn Use the definition...Ch. 3.1 - Prob. 63ECh. 3.1 - Approximating derivatives Assuming the limit...Ch. 3.1 - Approximating derivatives Assuming the limit...Ch. 3.1 - Prob. 66ECh. 3.1 - Approximating derivatives Assuming the limit...Ch. 3.2 - Explain why f(x) could be positive or negative at...Ch. 3.2 - Prob. 2ECh. 3.2 - If f is differentiable at a, must f be continuous...Ch. 3.2 - If f is continuous at a, must f be differentiable...Ch. 3.2 - Derivatives from graphs Use the graph of f to...Ch. 3.2 - Derivatives from graphs Use the graph of f to...Ch. 3.2 - Matching functions with derivatives Match graphs...Ch. 3.2 - Matching derivatives with functions Match graphs...Ch. 3.2 - Matching functions with derivatives Match the...Ch. 3.2 - Sketching derivatives Reproduce the graph of f and...Ch. 3.2 - Sketching derivatives Reproduce the graph of f and...Ch. 3.2 - Sketching derivatives Reproduce the graph of f and...Ch. 3.2 - Graphing the derivative with asymptotes Sketch a...Ch. 3.2 - Graphing the derivative with asymptotes Sketch a...Ch. 3.2 - Where is the function continuous? Differentiable?...Ch. 3.2 - Where is the function continuous? Differentiable?...Ch. 3.2 - Explain why or why not Determine whether the...Ch. 3.2 - Prob. 18ECh. 3.2 - Finding f from f Sketch the graph of f(x) = x....Ch. 3.2 - Prob. 20ECh. 3.2 - Normal lines A line perpendicular to another line...Ch. 3.2 - Normal lines A line perpendicular to another line...Ch. 3.2 - Normal lines A line perpendicular to another line...Ch. 3.2 - Prob. 24ECh. 3.2 - Aiming a tangent line Given the function f and the...Ch. 3.2 - Aiming a tangent line Given the function f and the...Ch. 3.2 - Prob. 27ECh. 3.2 - Prob. 28ECh. 3.2 - Voltage on a capacitor A capacitor is a device in...Ch. 3.2 - Logistic growth A common model for population...Ch. 3.2 - One-sided derivatives The right-sided and...Ch. 3.2 - One-sided derivatives The right-sided and...Ch. 3.2 - Prob. 33ECh. 3.2 - Prob. 34ECh. 3.2 - Prob. 35ECh. 3.2 - Vertical tangent lines If a function f is...Ch. 3.2 - Continuity is necessary for differentiability a....Ch. 3.3 - Assume the derivatives of f and g exist in...Ch. 3.3 - Assume the derivatives of f and g exist in...Ch. 3.3 - Assume the derivatives of f and g exist in...Ch. 3.3 - Prob. 4ECh. 3.3 - Assume the derivatives of f and g exist in...Ch. 3.3 - Prob. 6ECh. 3.3 - Prob. 7ECh. 3.3 - Derivatives of power and constant functions Find...Ch. 3.3 - Prob. 9ECh. 3.3 - Derivatives of power and constant functions Find...Ch. 3.3 - Derivatives of power and constant functions Find...Ch. 3.3 - Prob. 12ECh. 3.3 - Derivatives of constant multiples of functions...Ch. 3.3 - Prob. 14ECh. 3.3 - Prob. 15ECh. 3.3 - Derivatives of constant multiples of functions...Ch. 3.3 - Prob. 17ECh. 3.3 - Prob. 18ECh. 3.3 - Derivatives of the sum of functions Find the...Ch. 3.3 - Prob. 20ECh. 3.3 - Prob. 21ECh. 3.3 - Prob. 22ECh. 3.3 - Prob. 23ECh. 3.3 - Prob. 24ECh. 3.3 - Derivatives of products Find the derivative of the...Ch. 3.3 - Derivatives of products Find the derivative of the...Ch. 3.3 - Prob. 27ECh. 3.3 - Prob. 28ECh. 3.3 - Derivatives of products Find the derivative of the...Ch. 3.3 - Derivatives of products Find the derivative of the...Ch. 3.3 - Derivatives of products Find the derivative of the...Ch. 3.3 - Derivatives of products Find the derivative of the...Ch. 3.3 - Derivatives of products Find the derivative of the...Ch. 3.3 - Derivatives of products Find the derivative of the...Ch. 3.3 - Equations of tangent lines a. Find an equation of...Ch. 3.3 - Equations of tangent lines a. Find an equation of...Ch. 3.3 - Equations of tangent lines a. Find an equation of...Ch. 3.3 - Equations of tangent lines a. Find an equation of...Ch. 3.3 - Finding slope locations Let f(x) = x3 6x + 5. a....Ch. 3.3 - Finding slope locations Let f(t) = t3 27t + 5. a....Ch. 3.3 - Finding slope locations Let f(x) = 2x3 3x2 12x +...Ch. 3.3 - Finding slope locations Let f(x) = 2ex 6x. a....Ch. 3.3 - Finding slope locations Let f(x)=4xx. a. Find all...Ch. 3.3 - Higher-order derivatives Find f(x), f(x), and f(x)...Ch. 3.3 - Higher-order derivatives Find f(x), f(x), and f(x)...Ch. 3.3 - Higher-order derivatives Find f(x), f(x), and f(x)...Ch. 3.3 - Higher-order derivatives Find f(x), f(x), and f(x)...Ch. 3.3 - Higher-order derivatives Find f(x), f(x), and f(x)...Ch. 3.3 - Explain why or why not Determine whether the...Ch. 3.3 - Tangent lines Suppose f(3) = 1 and f(3) = 4. Let...Ch. 3.3 - Derivatives from tangent lines Suppose the line...Ch. 3.3 - Tangent line Find the equation of the line tangent...Ch. 3.3 - Tangent line given Determine the constants b and c...Ch. 3.3 - Derivatives from a graph Let F = f + g and G = 3f ...Ch. 3.3 - Derivatives from a graph Let F = f + g and G = 3f ...Ch. 3.3 - Derivatives from a graph Let F = f + g and G = 3f ...Ch. 3.3 - Derivatives from a graph Let F = f + g and G = 3f ...Ch. 3.3 - Derivatives from a table Use the table to find the...Ch. 3.3 - Derivatives from a table Use the table to find the...Ch. 3.3 - Derivatives from a table Use the table to find the...Ch. 3.3 - Derivatives from limits The following limits...Ch. 3.3 - Derivatives from limits The following limits...Ch. 3.3 - Derivatives from limits The following limits...Ch. 3.3 - Prob. 64ECh. 3.3 - Prob. 65ECh. 3.3 - Calculator limits Use a calculator to approximate...Ch. 3.3 - Prob. 67ECh. 3.3 - Prob. 68ECh. 3.3 - Prob. 69ECh. 3.3 - Projectile trajectory The position of a small...Ch. 3.3 - Prob. 71ECh. 3.3 - Cell growth When observations begin at t = 0, a...Ch. 3.3 - City urbanization City planners model the size of...Ch. 3.3 - Constant Rule proof For the constant function f(x)...Ch. 3.3 - Prob. 75ECh. 3.3 - Looking ahead: Power Rule for negative integers...Ch. 3.3 - Prob. 77ECh. 3.3 - Computing the derivative of f(x) = ex a. Use the...Ch. 3.3 - Prob. 79ECh. 3.3 - Computing the derivative of f(x) = x2ex a. Use the...Ch. 3.4 - How do you find the derivative of the product of...Ch. 3.4 - How do you find the derivative of the quotient of...Ch. 3.4 - Prob. 3ECh. 3.4 - Show two ways to differentiate f(x) = 1/x10.Ch. 3.4 - Prob. 5ECh. 3.4 - Prob. 6ECh. 3.4 - Derivatives of products Find the derivative of the...Ch. 3.4 - Derivatives of products Find the derivative of the...Ch. 3.4 - Derivatives of products Find the derivative of the...Ch. 3.4 - Derivatives of products Find the derivative of the...Ch. 3.4 - Derivatives of products Find the derivative of the...Ch. 3.4 - Derivatives of products Find the derivative of the...Ch. 3.4 - Derivatives of products Find the derivative of the...Ch. 3.4 - Prob. 14ECh. 3.4 - Prob. 15ECh. 3.4 - Prob. 16ECh. 3.4 - Derivatives by two different methods a. Use the...Ch. 3.4 - Derivatives by two different methods a. Use the...Ch. 3.4 - Derivatives of quotients Find the derivative of...Ch. 3.4 - Derivatives of quotients Find the derivative of...Ch. 3.4 - Derivatives of quotients Find the derivative of...Ch. 3.4 - Derivatives of quotients Find the derivative of...Ch. 3.4 - Derivatives of quotients Find the derivative of...Ch. 3.4 - Prob. 24ECh. 3.4 - Derivatives of quotients Find the derivative of...Ch. 3.4 - Derivatives of quotients Find the derivative of...Ch. 3.4 - Derivatives of quotients Find the derivative of...Ch. 3.4 - Derivatives of quotients Find the derivative of...Ch. 3.4 - Derivatives by two different methods a. Use the...Ch. 3.4 - Prob. 30ECh. 3.4 - Derivatives by two different methods a. Use the...Ch. 3.4 - Derivatives by two different methods a. Use the...Ch. 3.4 - Equations of tangent lines a. Find an equation of...Ch. 3.4 - Equations of tangent lines a. Find an equation of...Ch. 3.4 - Equations of tangent lines a. Find an equation of...Ch. 3.4 - Equations of tangent lines a. Find an equation of...Ch. 3.4 - Extended Power Rule Find the derivative of the...Ch. 3.4 - Extended Power Rule Find the derivative of the...Ch. 3.4 - Extended Power Rule Find the derivative of the...Ch. 3.4 - Extended Power Rule Find the derivative of the...Ch. 3.4 - Extended Power Rule Find the derivative of the...Ch. 3.4 - Extended Power Rule Find the derivative of the...Ch. 3.4 - Prob. 43ECh. 3.4 - Prob. 44ECh. 3.4 - Prob. 45ECh. 3.4 - Prob. 46ECh. 3.4 - Prob. 47ECh. 3.4 - Prob. 48ECh. 3.4 - Prob. 49ECh. 3.4 - Prob. 50ECh. 3.4 - Population growth Consider the following...Ch. 3.4 - Prob. 52ECh. 3.4 - Antibiotic decay The half-life of an antibiotic in...Ch. 3.4 - Bank account A 200 investment in a savings account...Ch. 3.4 - Finding slope locations Let f(x) = xe2x. a. Find...Ch. 3.4 - Prob. 56ECh. 3.4 - Combining rules Compute the derivative of the...Ch. 3.4 - Combining rules Compute the derivative of the...Ch. 3.4 - Combining rules Compute the derivative of the...Ch. 3.4 - Combining rules Compute the derivative of the...Ch. 3.4 - Prob. 61ECh. 3.4 - Higher-order derivatives Find f(x), f(x), and...Ch. 3.4 - Prob. 63ECh. 3.4 - First and second derivatives Find f(x) and f(x)....Ch. 3.4 - First and second derivatives Find f(x) and f(x)....Ch. 3.4 - Choose your method Use any method to evaluate the...Ch. 3.4 - Choose your method Use any method to evaluate the...Ch. 3.4 - Prob. 68ECh. 3.4 - Choose your method Use any method to evaluate the...Ch. 3.4 - Choose your method Use any method to evaluate the...Ch. 3.4 - Choose your method Use any method to evaluate the...Ch. 3.4 - Tangent lines Suppose f(2) = 2 and f(2) = 3. Let...Ch. 3.4 - The Witch of Agnesi The graph of y=a3x2+a2, where...Ch. 3.4 - Derivatives from a table Use the following table...Ch. 3.4 - Derivatives from a table Use the following table...Ch. 3.4 - Derivatives from a table Use the following table...Ch. 3.4 - Derivatives from a table Use the following table...Ch. 3.4 - Derivatives from a table Use the following table...Ch. 3.4 - Derivatives from a table Use the following table...Ch. 3.4 - Derivatives from tangent lines Suppose the line...Ch. 3.4 - Electrostatic force The magnitude of the...Ch. 3.4 - Gravitational force The magnitude of the...Ch. 3.4 - Prob. 83ECh. 3.4 - Prob. 84ECh. 3.4 - Prob. 85ECh. 3.4 - Proof of the Quotient Rule Let F = f/g be the...Ch. 3.4 - Product Rule for the second derivative Assuming...Ch. 3.4 - Prob. 88ECh. 3.4 - Derivative of ekx for negative integers k Use the...Ch. 3.4 - Prob. 90ECh. 3.4 - Product Rule for three functions Assume that f, g,...Ch. 3.4 - One of the Leibniz Rules One of several Leibniz...Ch. 3.5 - Why is it not possible to evaluate limx0sinxx by...Ch. 3.5 - How is limx0sinxx used in this section?Ch. 3.5 - Explain why the Quotient Rule is used to determine...Ch. 3.5 - How can you use the derivatives ddx(sinx)=cosx,...Ch. 3.5 - Let f(x) = sin x. What is the value of f()?Ch. 3.5 - Where does the graph of sin x have a horizontal...Ch. 3.5 - Trigonometric limits Use Theorem 3.11 to evaluate...Ch. 3.5 - Trigonometric limits Use Theorem 3.11 to evaluate...Ch. 3.5 - Trigonometric limits Use Theorem 3.11 to evaluate...Ch. 3.5 - Trigonometric limits Use Theorem 3.11 to evaluate...Ch. 3.5 - Trigonometric limits Use Theorem 3.11 to evaluate...Ch. 3.5 - Trigonometric limits Use Theorem 3.11 to evaluate...Ch. 3.5 - Trigonometric limits Use Theorem 3.11 to evaluate...Ch. 3.5 - Trigonometric limits Use Theorem 3.11 to evaluate...Ch. 3.5 - Trigonometric limits Use Theorem 3.11 to evaluate...Ch. 3.5 - Trigonometric limits Use Theorem 3.11 to evaluate...Ch. 3.5 - Calculating derivatives Find dy/dx for the...Ch. 3.5 - Calculating derivatives Find dy/dx for the...Ch. 3.5 - Calculating derivatives Find dy/dx for the...Ch. 3.5 - Calculating derivatives Find dy/dx for the...Ch. 3.5 - Calculating derivatives Find dy/dx for the...Ch. 3.5 - Prob. 22ECh. 3.5 - Calculating derivatives Find dy/dx for the...Ch. 3.5 - Calculating derivatives Find dy/dx for the...Ch. 3.5 - Calculating derivatives Find dy/dx for the...Ch. 3.5 - Prob. 26ECh. 3.5 - Calculating derivatives Find dy/dx for the...Ch. 3.5 - Calculating derivatives Find dy/dx for the...Ch. 3.5 - Derivatives of other trigonometric functions...Ch. 3.5 - Derivatives of other trigonometric functions...Ch. 3.5 - Derivatives of other trigonometric functions...Ch. 3.5 - Derivatives involving other trigonometric...Ch. 3.5 - Derivatives involving other trigonometric...Ch. 3.5 - Derivatives involving other trigonometric...Ch. 3.5 - Derivatives involving other trigonometric...Ch. 3.5 - Derivatives involving other trigonometric...Ch. 3.5 - Derivatives involving other trigonometric...Ch. 3.5 - Derivatives involving other trigonometric...Ch. 3.5 - Derivatives involving other trigonometric...Ch. 3.5 - Derivatives involving other trigonometric...Ch. 3.5 - Second-order derivatives Find y for the following...Ch. 3.5 - Prob. 42ECh. 3.5 - Second-order derivatives Find y for the following...Ch. 3.5 - Second-order derivatives Find y for the following...Ch. 3.5 - Second-order derivatives Find y for the following...Ch. 3.5 - Second-order derivatives Find y for the following...Ch. 3.5 - Second-order derivatives Find y for the following...Ch. 3.5 - Second-order derivatives Find y for the following...Ch. 3.5 - Explain why or why not Determine whether the...Ch. 3.5 - Trigonometric limits Evaluate the following limits...Ch. 3.5 - Trigonometric limits Evaluate the following limits...Ch. 3.5 - Trigonometric limits Evaluate the following limits...Ch. 3.5 - Prob. 53ECh. 3.5 - Prob. 54ECh. 3.5 - Prob. 55ECh. 3.5 - Calculating derivatives Find dy/dx for the...Ch. 3.5 - Calculating derivatives Find dy/dx for the...Ch. 3.5 - Calculating derivatives Find dy/dx for the...Ch. 3.5 - Prob. 59ECh. 3.5 - Prob. 60ECh. 3.5 - Calculating derivatives Find dy/dx for the...Ch. 3.5 - Equations of tangent lines a. Find an equation of...Ch. 3.5 - Equations of tangent lines a. Find an equation of...Ch. 3.5 - Equations of tangent lines a. Find an equation of...Ch. 3.5 - Equations of tangent lines a. Find an equation of...Ch. 3.5 - Locations of tangent lines a. For what values of x...Ch. 3.5 - Locations of horizontal tangent lines For what...Ch. 3.5 - Matching Match the graphs of the functions in ad...Ch. 3.5 - Velocity of an oscillator An object oscillates...Ch. 3.5 - Prob. 70ECh. 3.5 - A differential equation A differential equation is...Ch. 3.5 - Using identities Use the identity sin 2x = 2 sin x...Ch. 3.5 - Prob. 73ECh. 3.5 - Prob. 74ECh. 3.5 - Proof of ddx(cosx)=sinx Use the definition of the...Ch. 3.5 - Continuity of a piecewise function Let...Ch. 3.5 - Continuity of a piecewise function Let...Ch. 3.5 - Prob. 78ECh. 3.5 - Prob. 79ECh. 3.5 - Prob. 80ECh. 3.5 - Prob. 81ECh. 3.5 - Prob. 82ECh. 3.5 - Prob. 83ECh. 3.5 - Prob. 84ECh. 3.5 - Prob. 85ECh. 3.5 - Prob. 86ECh. 3.6 - Explain the difference between the average rate of...Ch. 3.6 - Complete the following statement. If dydx is...Ch. 3.6 - Complete the following statement: If dydx is...Ch. 3.6 - What is the difference between the velocity and...Ch. 3.6 - Define the acceleration of an object moving in a...Ch. 3.6 - An object moving along a line has a constant...Ch. 3.6 - Prob. 7ECh. 3.6 - Explain why a decreasing demand function has a...Ch. 3.6 - Highway travel A state patrol station is located...Ch. 3.6 - Airline travel The following figure shows the...Ch. 3.6 - Position, velocity, and acceleration Suppose the...Ch. 3.6 - Position, velocity, and acceleration Suppose the...Ch. 3.6 - Position, velocity, and acceleration Suppose the...Ch. 3.6 - Position, velocity, and acceleration Suppose the...Ch. 3.6 - Position, velocity, and acceleration Suppose the...Ch. 3.6 - Position, velocity, and acceleration Suppose the...Ch. 3.6 - A stone thrown vertically on Mars Suppose a stone...Ch. 3.6 - Prob. 18ECh. 3.6 - Population growth in Georgia The population of the...Ch. 3.6 - Consumer price index The U.S. consumer price index...Ch. 3.6 - Average and marginal cost Consider the following...Ch. 3.6 - Average and marginal cost Consider the following...Ch. 3.6 - Average and marginal cost Consider the following...Ch. 3.6 - Average and marginal cost Consider the following...Ch. 3.6 - Demand and elasticity Based on sales data over the...Ch. 3.6 - Demand and elasticity The economic advisor of a...Ch. 3.6 - Prob. 27ECh. 3.6 - Prob. 28ECh. 3.6 - Explain why or why not Determine whether the...Ch. 3.6 - A feather dropped on the moon On the moon, a...Ch. 3.6 - Comparing velocities A stone is thrown vertically...Ch. 3.6 - Comparing velocities Two stones are thrown...Ch. 3.6 - Matching heights A stone is thrown from the edge...Ch. 3.6 - Velocity of a car The graph shows the position s =...Ch. 3.6 - Velocity from position The graph of s = f(t)...Ch. 3.6 - Fish length Assume the length L (in cm) of a...Ch. 3.6 - Average and marginal profit Let C(x) represent the...Ch. 3.6 - Average and marginal profit Let C(x) represent the...Ch. 3.6 - Average and marginal profit Let C(x) represent the...Ch. 3.6 - Average and marginal profit Let C(x) represent the...Ch. 3.6 - Prob. 41ECh. 3.6 - Average and marginal production Economists use...Ch. 3.6 - Velocity of a marble The position (in meters) of a...Ch. 3.6 - Tree growth Let b represent the base diameter of a...Ch. 3.6 - Prob. 45ECh. 3.6 - Diminishing returns A cost function of the form...Ch. 3.6 - Revenue function A store manager estimates that...Ch. 3.6 - Fuel economy Suppose you own a fuel-efficient...Ch. 3.6 - Spring oscillations A spring hangs from the...Ch. 3.6 - Pressure and altitude Earths atmospheric pressure...Ch. 3.6 - A race Jean and Juan run a one-lap race on a...Ch. 3.6 - Power and energy Power and energy are often used...Ch. 3.6 - Flow from a tank A cylindrical tank is full at...Ch. 3.6 - Prob. 54ECh. 3.6 - Bungee jumper A woman attached to a bungee cord...Ch. 3.6 - Spring runoff The flow of a small stream is...Ch. 3.6 - Temperature distribution A thin copper rod, 4...Ch. 3.7 - Two equivalent forms of the Chain Rule for...Ch. 3.7 - Let h(x) = f(g(x)), where f and g are...Ch. 3.7 - Fill in the blanks. The derivative of f(g(x))...Ch. 3.7 - Prob. 4ECh. 3.7 - Identify the inner and outer functions in the...Ch. 3.7 - Express Q(x) = cos4 (x2 + 1) as the composition of...Ch. 3.7 - Version 1 of the Chain Rule Use Version 1 of the...Ch. 3.7 - Version 1 of the Chain Rule Use Version 1 of the...Ch. 3.7 - Version 1 of the Chain Rule Use Version 1 of the...Ch. 3.7 - Prob. 10ECh. 3.7 - Version 1 of the Chain Rule Use Version 1 of the...Ch. 3.7 - Version 1 of the Chain Rule Use Version 1 of the...Ch. 3.7 - Version 1 of the Chain Rule Use Version 1 of the...Ch. 3.7 - Version 1 of the Chain Rule Use Version 1 of the...Ch. 3.7 - Version 1 of the Chain Rule Use Version 1 of the...Ch. 3.7 - Version 1 of the Chain Rule Use Version 1 of the...Ch. 3.7 - Version 1 of the Chain Rule Use Version 1 of the...Ch. 3.7 - Prob. 18ECh. 3.7 - Version 2 of the Chain Rule Use Version 2 of the...Ch. 3.7 - Version 2 of the Chain Rule Use Version 2 of the...Ch. 3.7 - Version 2 of the Chain Rule Use Version 2 of the...Ch. 3.7 - Prob. 22ECh. 3.7 - Version 2 of the Chain Rule Use Version 2 of the...Ch. 3.7 - Version 2 of the Chain Rule Use Version 2 of the...Ch. 3.7 - Version 2 of the Chain Rule Use Version 2 of the...Ch. 3.7 - Version 2 of the Chain Rule Use Version 2 of the...Ch. 3.7 - Version 2 of the Chain Rule Use Version 2 of the...Ch. 3.7 - Version 2 of the Chain Rule Use Version 2 of the...Ch. 3.7 - Version 2 of the Chain Rule Use Version 2 of the...Ch. 3.7 - Version 2 of the Chain Rule Use Version 2 of the...Ch. 3.7 - Prob. 31ECh. 3.7 - Version 2 of the Chain Rule Use Version 2 of the...Ch. 3.7 - Version 2 of the Chain Rule Use Version 2 of the...Ch. 3.7 - Version 2 of the Chain Rule Use Version 2 of the...Ch. 3.7 - Prob. 35ECh. 3.7 - Prob. 36ECh. 3.7 - Chain Rule using a table Let h(x)= f(g(x)) and...Ch. 3.7 - Prob. 38ECh. 3.7 - Applying the Chain Rule Use the data in Tables 3.4...Ch. 3.7 - Chain Rule for powers Use the Chain Rule to find...Ch. 3.7 - Chain Rule for powers Use the Chain Rule to find...Ch. 3.7 - Prob. 43ECh. 3.7 - Chain Rule for powers Use the Chain Rule to find...Ch. 3.7 - Repeated use of the Chain Rule Calculate the...Ch. 3.7 - Prob. 46ECh. 3.7 - Repeated use of the Chain Rule Calculate the...Ch. 3.7 - Repeated use of the Chain Rule Calculate the...Ch. 3.7 - Repeated use of the Chain Rule Calculate the...Ch. 3.7 - Prob. 50ECh. 3.7 - Prob. 51ECh. 3.7 - Repeated use of the Chain Rule Calculate the...Ch. 3.7 - Repeated use of the Chain Rule Calculate the...Ch. 3.7 - Repeated use of the Chain Rule Calculate the...Ch. 3.7 - Repeated use of the Chain Rule Calculate the...Ch. 3.7 - Repeated use of the Chain Rule Calculate the...Ch. 3.7 - Combining rules Use the Chain Rule combined with...Ch. 3.7 - Combining rules Use the Chain Rule combined with...Ch. 3.7 - Combining rules Use the Chain Rule combined with...Ch. 3.7 - Combining rules Use the Chain Rule combined with...Ch. 3.7 - Combining rules Use the Chain Rule combined with...Ch. 3.7 - Combining rules Use the Chain Rule combined with...Ch. 3.7 - Combining rules Use the Chain Rule combined with...Ch. 3.7 - Combining rules Use the Chain Rule combined with...Ch. 3.7 - Prob. 65ECh. 3.7 - Combining rules Use the Chain Rule combined with...Ch. 3.7 - Prob. 67ECh. 3.7 - Prob. 68ECh. 3.7 - Explain why or why not Determine whether the...Ch. 3.7 - Second derivatives Find d2ydx2 for the following...Ch. 3.7 - Second derivatives Find d2ydx2 for the following...Ch. 3.7 - Second derivatives Find d2ydx2 for the following...Ch. 3.7 - Second derivatives Find d2ydx2 for the following...Ch. 3.7 - Prob. 74ECh. 3.7 - Square root derivatives Find the derivative of the...Ch. 3.7 - Prob. 76ECh. 3.7 - Prob. 77ECh. 3.7 - Tangent lines Determine equations of the lines...Ch. 3.7 - Tangent lines Assume f and g are differentiable on...Ch. 3.7 - Prob. 80ECh. 3.7 - Tangent lines Find the equation of the line...Ch. 3.7 - Prob. 82ECh. 3.7 - Composition containing sin x Suppose f is...Ch. 3.7 - Prob. 84ECh. 3.7 - Prob. 85ECh. 3.7 - Prob. 86ECh. 3.7 - A damped oscillator The displacement of a mass on...Ch. 3.7 - Oscillator equation A mechanical oscillator (such...Ch. 3.7 - Prob. 89ECh. 3.7 - Prob. 90ECh. 3.7 - Prob. 91ECh. 3.7 - Deriving trigonometric identities a. Differentiate...Ch. 3.7 - Prob. 93ECh. 3.7 - Prob. 94ECh. 3.7 - Prob. 95ECh. 3.7 - Prob. 96ECh. 3.7 - Prob. 97ECh. 3.7 - Prob. 98ECh. 3.7 - Prob. 99ECh. 3.7 - Prob. 100ECh. 3.7 - Prob. 101ECh. 3.7 - Prob. 102ECh. 3.7 - Prob. 103ECh. 3.8 - For some equations, such as x2 + y2 = l or x y2 =...Ch. 3.8 - Explain the differences between computing the...Ch. 3.8 - Why are both the x-coordinate and the y-coordinate...Ch. 3.8 - In this section, for what values of n did we prove...Ch. 3.8 - Implicit differentiation Carry out the following...Ch. 3.8 - Implicit differentiation Carry out the following...Ch. 3.8 - Implicit differentiation Carry out the following...Ch. 3.8 - Implicit differentiation Carry out the following...Ch. 3.8 - Implicit differentiation Carry out the following...Ch. 3.8 - Implicit differentiation Carry out the following...Ch. 3.8 - Implicit differentiation Carry out the following...Ch. 3.8 - Implicit differentiation Carry out the following...Ch. 3.8 - Implicit differentiation Use implicit...Ch. 3.8 - Implicit differentiation Use implicit...Ch. 3.8 - Implicit differentiation Use implicit...Ch. 3.8 - Implicit differentiation Use implicit...Ch. 3.8 - Implicit differentiation Use implicit...Ch. 3.8 - Implicit differentiation Use implicit...Ch. 3.8 - Implicit differentiation Use implicit...Ch. 3.8 - Implicit differentiation Use implicit...Ch. 3.8 - Implicit differentiation Use implicit...Ch. 3.8 - Implicit differentiation Use implicit...Ch. 3.8 - Implicit differentiation Use implicit...Ch. 3.8 - Implicit differentiation Use implicit...Ch. 3.8 - Tangent lines Carry out the following steps. a....Ch. 3.8 - Tangent lines Carry out the following steps. a....Ch. 3.8 - Prob. 27ECh. 3.8 - Tangent lines Carry out the following steps. a....Ch. 3.8 - Tangent lines Carry out the following steps. a....Ch. 3.8 - Tangent lines Carry out the following steps. a....Ch. 3.8 - Second derivatives Find d2ydx2. 31. x + y2 = 1Ch. 3.8 - Second derivatives Find d2ydx2. 32. 2x2 + y2 = 4Ch. 3.8 - Second derivatives Find d2ydx2. 33. x + y = sin yCh. 3.8 - Second derivatives Find d2ydx2. 34. x4 + y4 = 64Ch. 3.8 - Second derivatives Find d2ydx2. 35. e2y + x = yCh. 3.8 - Second derivatives Find d2ydx2 36. sin x + x2y =...Ch. 3.8 - Prob. 37ECh. 3.8 - Prob. 38ECh. 3.8 - Prob. 39ECh. 3.8 - Prob. 40ECh. 3.8 - Prob. 41ECh. 3.8 - Prob. 42ECh. 3.8 - Prob. 43ECh. 3.8 - Prob. 44ECh. 3.8 - Prob. 45ECh. 3.8 - Prob. 46ECh. 3.8 - Prob. 47ECh. 3.8 - Prob. 48ECh. 3.8 - Prob. 49ECh. 3.8 - Prob. 50ECh. 3.8 - Explain why or why not Determine whether the...Ch. 3.8 - Multiple tangent lines Complete the following...Ch. 3.8 - Multiple tangent lines Complete the following...Ch. 3.8 - Multiple tangent lines Complete the following...Ch. 3.8 - Witch of Agnesi Let y(x2 + 4) = 8 (see figure). a....Ch. 3.8 - Vertical tangent lines a. Determine the points at...Ch. 3.8 - Vertical tangent lines a. Determine the points...Ch. 3.8 - Tangent lines for ellipses Find the equations of...Ch. 3.8 - Tangent lines for ellipses Find the equations of...Ch. 3.8 - Prob. 60ECh. 3.8 - Identifying functions from an equation The...Ch. 3.8 - Prob. 62ECh. 3.8 - Prob. 63ECh. 3.8 - Prob. 64ECh. 3.8 - Normal lines A normal line at a point P on a curve...Ch. 3.8 - Normal lines A normal line at a point P on a curve...Ch. 3.8 - Prob. 67ECh. 3.8 - Normal lines A normal line at a point P on a curve...Ch. 3.8 - Prob. 69ECh. 3.8 - Normal lines A normal line at a point P on a curve...Ch. 3.8 - Prob. 71ECh. 3.8 - Visualizing tangent and normal lines a. Determine...Ch. 3.8 - Visualizing tangent and normal lines a. Determine...Ch. 3.8 - Prob. 74ECh. 3.8 - Cobb-Douglas production function The output of an...Ch. 3.8 - Surface area of a cone The lateral surface area of...Ch. 3.8 - Volume of a spherical cap Imagine slicing through...Ch. 3.8 - Volume of a torus The volume of a torus (doughnut...Ch. 3.8 - Orthogonal trajectories Two curves are orthogonal...Ch. 3.8 - Orthogonal trajectories Two curves are orthogonal...Ch. 3.8 - Orthogonal trajectories Two curves are orthogonal...Ch. 3.8 - Finding slope Find the slope of the curve...Ch. 3.8 - A challenging derivative Find dydx, where (x2 +...Ch. 3.8 - Prob. 84ECh. 3.8 - A challenging derivative Find d2ydx2, where...Ch. 3.8 - Work carefully Proceed with caution when using...Ch. 3.8 - Work carefully Proceed with caution when using...Ch. 3.8 - Work carefully Proceed with caution when using...Ch. 3.8 - Work carefully Proceed with caution when using...Ch. 3.9 - Use x = ey to explain why ddx(lnx)=1x, for x 0.Ch. 3.9 - Prob. 2ECh. 3.9 - Show that ddx(lnkx)=ddx(lnx), where x 0 and k is...Ch. 3.9 - State the derivative rule for the exponential...Ch. 3.9 - State the derivative rule for the logarithmic...Ch. 3.9 - Explain why bx = ex ln bCh. 3.9 - Prob. 7ECh. 3.9 - Prob. 8ECh. 3.9 - Derivatives involving ln x Find the following...Ch. 3.9 - Derivatives involving ln x Find the following...Ch. 3.9 - Derivatives involving ln x Find the following...Ch. 3.9 - Derivatives involving ln x Find the following...Ch. 3.9 - Derivatives involving ln x Find the following...Ch. 3.9 - Derivatives involving ln x Find the following...Ch. 3.9 - Derivatives involving ln x Find the following...Ch. 3.9 - Derivatives involving ln x Find the following...Ch. 3.9 - Derivatives involving ln x Find the following...Ch. 3.9 - Derivatives involving ln x Find the following...Ch. 3.9 - Derivatives involving ln x Find the following...Ch. 3.9 - Derivatives involving ln x Find the following...Ch. 3.9 - Derivatives involving ln x Find the following...Ch. 3.9 - Derivatives involving ln x Find the following...Ch. 3.9 - Derivatives of bx Find the derivatives of the...Ch. 3.9 - Derivatives of bx Find the derivatives of the...Ch. 3.9 - Derivatives of bx Find the derivatives of the...Ch. 3.9 - Derivatives of bx Find the derivatives of the...Ch. 3.9 - Derivatives of bx Find the derivatives of the...Ch. 3.9 - Derivatives of bx Find the derivatives of the...Ch. 3.9 - Derivatives of bx Find the derivatives of the...Ch. 3.9 - Prob. 30ECh. 3.9 - Exponential model The following table shows the...Ch. 3.9 - Magnitude of an earthquake The energy (in joules)...Ch. 3.9 - Diagnostic scanning Iodine-123 is a radioactive...Ch. 3.9 - Prob. 34ECh. 3.9 - Prob. 35ECh. 3.9 - Prob. 36ECh. 3.9 - Prob. 37ECh. 3.9 - General Power Rule Use the General Power Rule...Ch. 3.9 - Prob. 39ECh. 3.9 - General Power Rule Use the General Power Rule...Ch. 3.9 - Prob. 41ECh. 3.9 - Prob. 42ECh. 3.9 - General Power Rule Use the General Power Rule...Ch. 3.9 - General Power Rule Use the General Power Rule...Ch. 3.9 - Derivatives of Tower Functions (or gh) Find the...Ch. 3.9 - Derivatives of Tower Functions (or gh) Find the...Ch. 3.9 - Derivatives of Tower Functions (or gh) Find the...Ch. 3.9 - Derivatives of Tower Functions (or gh) Find the...Ch. 3.9 - Derivatives of Tower Functions (or gh) Find the...Ch. 3.9 - Derivatives of Tower Functions (or gh) Find the...Ch. 3.9 - Find an equation of the line tangent to y = xsin x...Ch. 3.9 - Determine whether the graph of y=xx has any...Ch. 3.9 - The graph of y = (x2)x has two horizontal tangent...Ch. 3.9 - The graph of y = xln x has one horizontal tangent...Ch. 3.9 - Derivatives of logarithmic functions Calculate the...Ch. 3.9 - Derivatives of logarithmic functions Calculate the...Ch. 3.9 - Derivatives of logarithmic functions Calculate the...Ch. 3.9 - Derivatives of logarithmic functions Calculate the...Ch. 3.9 - Derivatives of logarithmic functions Calculate the...Ch. 3.9 - Derivatives of logarithmic functions Calculate the...Ch. 3.9 - Logarithmic differentiation Use logarithmic...Ch. 3.9 - Logarithmic differentiation Use logarithmic...Ch. 3.9 - Logarithmic differentiation Use logarithmic...Ch. 3.9 - Logarithmic differentiation Use logarithmic...Ch. 3.9 - Logarithmic differentiation Use logarithmic...Ch. 3.9 - Logarithmic differentiation Use logarithmic...Ch. 3.9 - Logarithmic differentiation Use logarithmic...Ch. 3.9 - Prob. 68ECh. 3.9 - Prob. 69ECh. 3.9 - Prob. 70ECh. 3.9 - Higher-order derivatives Find the following...Ch. 3.9 - Higher-order derivatives Find the following...Ch. 3.9 - Higher-order derivatives Find the following...Ch. 3.9 - Derivatives by different methods Calculate the...Ch. 3.9 - Derivatives by different methods Calculate the...Ch. 3.9 - Prob. 76ECh. 3.9 - Derivatives of logarithmic functions Use the...Ch. 3.9 - Derivatives of logarithmic functions Use the...Ch. 3.9 - Derivatives of logarithmic functions Use the...Ch. 3.9 - Derivatives of logarithmic functions Use the...Ch. 3.9 - Derivatives of logarithmic functions Use the...Ch. 3.9 - Derivatives of logarithmic functions Use the...Ch. 3.9 - Tangent lines Find the equation of the line...Ch. 3.9 - Horizontal tangents The graph of y = cos x ln...Ch. 3.9 - General logarithmic and exponential derivatives...Ch. 3.9 - General logarithmic and exponential derivatives...Ch. 3.9 - Prob. 87ECh. 3.9 - Prob. 88ECh. 3.9 - Prob. 89ECh. 3.9 - General logarithmic and exponential derivatives...Ch. 3.9 - Prob. 91ECh. 3.9 - Prob. 92ECh. 3.9 - Logistic growth Scientists often use the logistic...Ch. 3.9 - Logistic growth Scientists often use the logistic...Ch. 3.9 - Prob. 95ECh. 3.9 - Logistic growth Scientists often use the logistic...Ch. 3.9 - Savings plan Beginning at age 30, a self-employed...Ch. 3.9 - Tangency question It is easily verified that the...Ch. 3.9 - Tangency question It is easily verified that the...Ch. 3.9 - Triple intersection Graph the functions f(x) = x3,...Ch. 3.9 - Calculating limits exactly Use the definition of...Ch. 3.9 - Calculating limits exactly Use the definition of...Ch. 3.9 - Calculating limits exactly Use the definition of...Ch. 3.9 - Calculating limits exactly Use the definition of...Ch. 3.9 - Derivative of u(x)v(x) Use logarithmic...Ch. 3.9 - Tangent lines and exponentials. Assume b is given...Ch. 3.10 - State the derivative formulas for sin1 x, tan1 x,...Ch. 3.10 - What is the slope of the line tangent to the graph...Ch. 3.10 - What is the slope of the line tangent to the graph...Ch. 3.10 - How are the derivatives of sin1 x and cos1 x...Ch. 3.10 - Suppose f is a one-to-one function with f(2) = 8...Ch. 3.10 - Explain how to find (f1)(y0), given that y0 =...Ch. 3.10 - Derivatives of inverse sine Evaluate the...Ch. 3.10 - Derivatives of inverse sine Evaluate the...Ch. 3.10 - Derivatives of inverse sine Evaluate the...Ch. 3.10 - Derivatives of inverse sine Evaluate the...Ch. 3.10 - Derivatives of inverse sine Evaluate the...Ch. 3.10 - Derivatives of inverse sine Evaluate the...Ch. 3.10 - Derivatives Evaluate the derivatives of the...Ch. 3.10 - Prob. 14ECh. 3.10 - Prob. 15ECh. 3.10 - Derivatives Evaluate the derivatives of the...Ch. 3.10 - Derivatives Evaluate the derivatives of the...Ch. 3.10 - Derivatives Evaluate the derivatives of the...Ch. 3.10 - Derivatives Evaluate the derivatives of the...Ch. 3.10 - Prob. 20ECh. 3.10 - Derivatives Evaluate the derivatives of the...Ch. 3.10 - Derivatives Evaluate the derivatives of the...Ch. 3.10 - Derivatives Evaluate the derivatives of the...Ch. 3.10 - Derivatives Evaluate the derivatives of the...Ch. 3.10 - Derivatives Evaluate the derivatives of the...Ch. 3.10 - Derivatives Evaluate the derivatives of the...Ch. 3.10 - Derivatives Evaluate the derivatives of the...Ch. 3.10 - Derivatives Evaluate the derivatives of the...Ch. 3.10 - Derivatives Evaluate the derivatives of the...Ch. 3.10 - Derivatives Evaluate the derivatives of the...Ch. 3.10 - Tangent lines Find an equation of the line tangent...Ch. 3.10 - Tangent lines Find an equation of the line tangent...Ch. 3.10 - Tangent lines Find an equation of the line tangent...Ch. 3.10 - Tangent lines Find an equation of the line tangent...Ch. 3.10 - Angular size A boat sails directly toward a...Ch. 3.10 - Prob. 36ECh. 3.10 - Derivatives of inverse functions at a point Find...Ch. 3.10 - Derivatives of inverse functions at a point Find...Ch. 3.10 - Prob. 39ECh. 3.10 - Derivatives of inverse functions at a point Find...Ch. 3.10 - Derivatives of inverse functions at a point Find...Ch. 3.10 - Derivatives of inverse functions at a point Find...Ch. 3.10 - Prob. 43ECh. 3.10 - Prob. 44ECh. 3.10 - Slopes of tangent lines Given the function f, find...Ch. 3.10 - Prob. 46ECh. 3.10 - Prob. 47ECh. 3.10 - Prob. 48ECh. 3.10 - Prob. 49ECh. 3.10 - Prob. 50ECh. 3.10 - Derivatives of inverse functions from a table Use...Ch. 3.10 - Derivatives of inverse functions from a table Use...Ch. 3.10 - Explain why or why not Determine whether the...Ch. 3.10 - Prob. 54ECh. 3.10 - Graphing f and f a. Graph f with a graphing...Ch. 3.10 - Prob. 56ECh. 3.10 - Prob. 57ECh. 3.10 - Graphing with inverse trigonometric functions a....Ch. 3.10 - Prob. 59ECh. 3.10 - Prob. 60ECh. 3.10 - Prob. 61ECh. 3.10 - Prob. 62ECh. 3.10 - Prob. 63ECh. 3.10 - Prob. 64ECh. 3.10 - Prob. 65ECh. 3.10 - Prob. 66ECh. 3.10 - Towing a boat A boat is towed toward a dock by a...Ch. 3.10 - Tracking a dive A biologist standing at the bottom...Ch. 3.10 - Angle to a particle, part I A particle travels...Ch. 3.10 - Prob. 70ECh. 3.10 - Prob. 71ECh. 3.10 - Prob. 72ECh. 3.10 - Prob. 73ECh. 3.10 - Prob. 74ECh. 3.10 - Identity proofs Prove the following identities and...Ch. 3.10 - Identity proofs Prove the following identities and...Ch. 3.10 - Identity proofs Prove the following identities and...Ch. 3.10 - Prob. 78ECh. 3.10 - Prob. 79ECh. 3.11 - Give an example in which one dimension of a...Ch. 3.11 - Prob. 2ECh. 3.11 - If two opposite sides of a rectangle increase in...Ch. 3.11 - Prob. 4ECh. 3.11 - Prob. 5ECh. 3.11 - Shrinking square The sides of a square decrease in...Ch. 3.11 - Expanding isosceles triangle The legs of an...Ch. 3.11 - Shrinking isosceles triangle The hypotenuse of an...Ch. 3.11 - Expanding circle The area of a circle increases at...Ch. 3.11 - Prob. 10ECh. 3.11 - Shrinking circle A circle has an initial radius of...Ch. 3.11 - Prob. 12ECh. 3.11 - Balloons A spherical balloon is inflated and its...Ch. 3.11 - Piston compression A piston is seated at the top...Ch. 3.11 - Melting snowball A spherical snowball melts at a...Ch. 3.11 - Prob. 16ECh. 3.11 - Prob. 17ECh. 3.11 - Expanding rectangle A rectangle initially has...Ch. 3.11 - Prob. 19ECh. 3.11 - Altitude of a jet A jet ascends at a 10 angle from...Ch. 3.11 - Rate of dive of a submarine A surface ship is...Ch. 3.11 - Prob. 22ECh. 3.11 - Ladder against the wall A 13-foot ladder is...Ch. 3.11 - Ladder against the wall again A 12-foot ladder is...Ch. 3.11 - Moving shadow A 5-foot-tall woman walks at 8 ft/s...Ch. 3.11 - Baseball runners Runners stand at first and second...Ch. 3.11 - Growing sandpile Sand falls from an overhead bin...Ch. 3.11 - Draining a water heater A water heater that has...Ch. 3.11 - Draining a tank An inverted conical water tank...Ch. 3.11 - Drinking a soda At what rate is soda being sucked...Ch. 3.11 - Prob. 31ECh. 3.11 - Filling a hemispherical tank A hemispherical tank...Ch. 3.11 - Prob. 33ECh. 3.11 - Observing a launch An observer stands 300 ft from...Ch. 3.11 - Another balloon story A hot-air balloon is 150 ft...Ch. 3.11 - Prob. 36ECh. 3.11 - Another fishing story An angler hooks a trout and...Ch. 3.11 - Flying a kite Once Kates kite reaches a height of...Ch. 3.11 - Rope on a boat A rope passing through a capstan on...Ch. 3.11 - Parabolic motion An arrow is shot into the air and...Ch. 3.11 - Time-lagged flights An airliner passes over an...Ch. 3.11 - Disappearing triangle An equilateral triangle...Ch. 3.11 - Clock hands The hands of the clock in the tower of...Ch. 3.11 - Filling two pools Two cylindrical swimming pools...Ch. 3.11 - Filming a race A camera is set up at the starting...Ch. 3.11 - Two tanks A conical tank with an upper radius of 4...Ch. 3.11 - Oblique tracking A port and a radar station are 2...Ch. 3.11 - Oblique tracking A ship leaves port traveling...Ch. 3.11 - Watching an elevator An observer is 20 m above the...Ch. 3.11 - A lighthouse problem A lighthouse stands 500 m off...Ch. 3.11 - Prob. 51ECh. 3.11 - Watching a Ferris wheel An observer stands 20 m...Ch. 3.11 - Viewing angle The bottom of a large theater screen...Ch. 3.11 - Searchlightwide beam A revolving searchlight,...Ch. 3.11 - Draining a trough A trough in the shape of a half...Ch. 3.11 - Divergent paths Two boats leave a port at the same...Ch. 3 - Explain why or why not Determine whether the...Ch. 3 - Prob. 2RECh. 3 - Prob. 3RECh. 3 - Prob. 4RECh. 3 - Prob. 5RECh. 3 - Prob. 6RECh. 3 - Prob. 7RECh. 3 - Growth rate of bacteria Suppose the following...Ch. 3 - Velocity of a skydiver Assume the graph represents...Ch. 3 - Prob. 10RECh. 3 - Prob. 11RECh. 3 - Sketching a derivative graph Sketch a graph of f...Ch. 3 - Sketching a derivative graph Sketch a graph of g...Ch. 3 - Matching functions and derivatives Match the...Ch. 3 - Evaluating derivatives Evaluate and simplify the...Ch. 3 - Evaluating derivatives Evaluate and simplify the...Ch. 3 - Evaluating derivatives Evaluate and simplify the...Ch. 3 - Evaluating derivatives Evaluate and simplify the...Ch. 3 - Evaluating derivatives Evaluate and simplify the...Ch. 3 - Evaluating derivatives Evaluate and simplify the...Ch. 3 - Evaluating derivatives Evaluate and simplify the...Ch. 3 - Evaluating derivatives Evaluate and simplify the...Ch. 3 - Evaluating derivatives Evaluate and simplify the...Ch. 3 - Prob. 24RECh. 3 - Prob. 25RECh. 3 - Evaluating derivatives Evaluate and simplify the...Ch. 3 - Evaluating derivatives Evaluate and simplify the...Ch. 3 - Prob. 28RECh. 3 - Evaluating derivatives Evaluate and simplify the...Ch. 3 - Evaluating derivatives Evaluate and simplify the...Ch. 3 - Evaluating derivatives Evaluate and simplify the...Ch. 3 - Prob. 32RECh. 3 - Evaluating derivatives Evaluate and simplify the...Ch. 3 - Evaluating derivatives Evaluate and simplify the...Ch. 3 - Evaluating derivatives Evaluate and simplify the...Ch. 3 - Evaluating derivatives Evaluate and simplify the...Ch. 3 - Implicit differentiation Calculate y(x) for the...Ch. 3 - Implicit differentiation Calculate y(x) for the...Ch. 3 - Implicit differentiation Calculate y(x) for the...Ch. 3 - Quadratic functions a. Show that if (a, f(a)) is...Ch. 3 - Prob. 41RECh. 3 - Prob. 42RECh. 3 - Prob. 43RECh. 3 - Prob. 44RECh. 3 - Prob. 45RECh. 3 - A parabola property Let f(x) = x2. a. Show that...Ch. 3 - Prob. 47RECh. 3 - Prob. 48RECh. 3 - Derivative formulas Evaluate the following...Ch. 3 - Prob. 50RECh. 3 - Derivative formulas Evaluate the following...Ch. 3 - Derivative formulas Evaluate the following...Ch. 3 - Prob. 53RECh. 3 - Limits The following limits represent the...Ch. 3 - Limits The following limits represent the...Ch. 3 - Derivative of the inverse at a point Consider the...Ch. 3 - Derivative of the inverse at a point Consider the...Ch. 3 - Derivative of the inverse Find the derivative of...Ch. 3 - Derivative of the inverse Find the derivative of...Ch. 3 - A function and its inverse function The function...Ch. 3 - Prob. 61RECh. 3 - Derivatives from a graph If possible, evaluate the...Ch. 3 - Derivatives from a graph If possible, evaluate the...Ch. 3 - Velocity of a probe A small probe is launched...Ch. 3 - Prob. 65RECh. 3 - Marginal and average cost Suppose a company...Ch. 3 - Population growth Suppose p(t) = 1.7t3 + 72t2 +...Ch. 3 - Position of a piston The distance between the head...Ch. 3 - Boat rates Two boats leave a dock at the same...Ch. 3 - Rate of inflation of a balloon A spherical balloon...Ch. 3 - Rate of descent of a hot-air balloon A rope is...Ch. 3 - Filling a tank Water flows into a conical tank at...Ch. 3 - Angle of elevation A jet flies horizontally 500 ft...Ch. 3 - Viewing angle A man whose eye level is 6 ft above...
Additional Math Textbook Solutions
Find more solutions based on key concepts
If 8 identical blackboards are to be divided among 4 schools, how many divisions are possible? How many if each...
A First Course in Probability (10th Edition)
Which of the series in Exercises 13–46 converge, and which diverge? Give reasons for your answers. (When you ch...
University Calculus: Early Transcendentals (4th Edition)
Identify f as being linear, quadratic, or neither. If f is quadratic, identify the leading coefficient a and ...
College Algebra with Modeling & Visualization (5th Edition)
Fill in each blank so that the resulting statement is true. An equation that expresses a relationship between t...
Algebra and Trigonometry (6th Edition)
Standard Normal Distribution. In Exercises 13–16, find the indicated z score. The graph depicts the standard no...
Elementary Statistics (13th Edition)
The solution of the equation
Pre-Algebra Student Edition
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, calculus and related others by exploring similar questions and additional content below.Similar questions
- x²-19x+90 Let f(x) = . Complete parts (a) through (c) below. x-a a. For what values of a, if any, does lim f(x) equal a finite number? Select the correct choice below and, if necessary, fill in the answer box to complete your choice. x→a+ ○ A. a= (Type an integer or a simplified fraction. Use a comma to separate answers as needed.) B. There are no values of a for which the limit equals a finite number. b. For what values of a, if any, does lim f(x) = ∞o? Select the correct choice below and, if necessary, fill in the answer boxes to complete your choice. x→a+ A. (Type integers or simplified fractions) C. There are no values of a that satisfy lim f(x) = ∞. + x-a c. For what values of a, if any, does lim f(x) = -∞0? Select the correct choice below and, if necessary, fill in the answer boxes to complete your choice. x→a+ A. Either a (Type integers or simplified fractions) B.arrow_forwardSketch a possible graph of a function f, together with vertical asymptotes, that satisfies all of the following conditions. f(2)=0 f(4) is undefined lim f(x)=1 X-6 lim f(x) = -∞ x-0+ lim f(x) = ∞ lim f(x) = ∞ x-4 _8arrow_forwardDetermine the following limit. lim 35w² +8w+4 w→∞ √49w+w³ 3 Select the correct choice below, and, if necessary, fill in the answer box to complete your choice. ○ A. lim W→∞ 35w² +8w+4 49w+w3 (Simplify your answer.) B. The limit does not exist and is neither ∞ nor - ∞.arrow_forwardCalculate the limit lim X-a x-a 5 using the following factorization formula where n is a positive integer and x-➡a a is a real number. x-a = (x-a) (x1+x-2a+x lim x-a X - a x-a 5 = n- + xa an-2 + an−1)arrow_forwardThe function s(t) represents the position of an object at time t moving along a line. Suppose s(1) = 116 and s(5)=228. Find the average velocity of the object over the interval of time [1,5]. The average velocity over the interval [1,5] is Vav = (Simplify your answer.)arrow_forwardFor the position function s(t) = - 16t² + 105t, complete the following table with the appropriate average velocities. Then make a conjecture about the value of the instantaneous velocity at t = 1. Time Interval Average Velocity [1,2] Complete the following table. Time Interval Average Velocity [1, 1.5] [1, 1.1] [1, 1.01] [1, 1.001] [1,2] [1, 1.5] [1, 1.1] [1, 1.01] [1, 1.001] ப (Type exact answers. Type integers or decimals.) The value of the instantaneous velocity at t = 1 is (Round to the nearest integer as needed.)arrow_forwardFind the following limit or state that it does not exist. Assume b is a fixed real number. (x-b) 40 - 3x + 3b lim x-b x-b ... Select the correct choice below and, if necessary, fill in the answer box to complete your choice. (x-b) 40 -3x+3b A. lim x-b x-b B. The limit does not exist. (Type an exact answer.)arrow_forwardx4 -289 Consider the function f(x) = 2 X-17 Complete parts a and b below. a. Analyze lim f(x) and lim f(x), and then identify the horizontal asymptotes. x+x X--∞ lim 4 X-289 2 X∞ X-17 X - 289 lim = 2 ... X∞ X - 17 Identify the horizontal asymptotes. Select the correct choice and, if necessary, fill in the answer box(es) to complete your choice. A. The function has a horizontal asymptote at y = B. The function has two horizontal asymptotes. The top asymptote is y = and the bottom asymptote is y = ☐ . C. The function has no horizontal asymptotes. b. Find the vertical asymptotes. For each vertical asymptote x = a, evaluate lim f(x) and lim f(x). Select the correct choice and, if necessary, fill in the answer boxes to complete your choice. earrow_forwardExplain why lim x²-2x-35 X-7 X-7 lim (x+5), and then evaluate lim X-7 x² -2x-35 x-7 x-7 Choose the correct answer below. A. x²-2x-35 The limits lim X-7 X-7 and lim (x+5) equal the same number when evaluated using X-7 direct substitution. B. Since each limit approaches 7, it follows that the limits are equal. C. The numerator of the expression X-2x-35 X-7 simplifies to x + 5 for all x, so the limits are equal. D. Since x²-2x-35 X-7 = x + 5 whenever x 7, it follows that the two expressions evaluate to the same number as x approaches 7. Now evaluate the limit. x²-2x-35 lim X-7 X-7 = (Simplify your answer.)arrow_forwardA function f is even if f(x) = f(x) for all x in the domain of f. If f is even, with lim f(x) = 4 and x-6+ lim f(x)=-3, find the following limits. X-6 a. lim f(x) b. +9-←x lim f(x) X-6 a. lim f(x)= +9-←x (Simplify your answer.) b. lim f(x)= X→-6 (Simplify your answer.) ...arrow_forwardEvaluate the following limit. lim X-X (10+19) Select the correct answer below and, if necessary, fill in the answer box within your choice. 10 A. lim 10+ = 2 ☐ (Type an integer or a simplified fraction.) X-∞ B. The limit does not exist.arrow_forwardFind the following limit or state that it does not exist. x² +x-20 lim x-4 x-4 Select the correct choice below and, if necessary, fill in the answer box to complete your choice. A. lim x²+x-20 x-4 (Type an exact answer.) x→4 B. The limit does not exist.arrow_forwardarrow_back_iosSEE MORE QUESTIONSarrow_forward_iosRecommended textbooks for you
Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
Algebra and Trigonometry (MindTap Course List)AlgebraISBN:9781305071742Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal Littell
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Trigonometry (MindTap Course List)TrigonometryISBN:9781305652224Author:Charles P. McKeague, Mark D. TurnerPublisher:Cengage Learning
Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:CengageAlgebra and Trigonometry (MindTap Course List)AlgebraISBN:9781305071742Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage LearningAlgebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal LittellMathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGALTrigonometry (MindTap Course List)TrigonometryISBN:9781305652224Author:Charles P. McKeague, Mark D. TurnerPublisher:Cengage LearningTrigonometric Ratios; Author: The Organic Chemistry Tutor;https://www.youtube.com/watch?v=9-eHMMpQC2k;License: Standard YouTube License, CC-BY