Physics for Scientists and Engineers
10th Edition
ISBN: 9781337553278
Author: Raymond A. Serway, John W. Jewett
Publisher: Cengage Learning
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 3, Problem 39AP
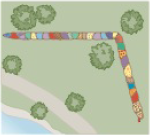
Review. The biggest stuffed animal in the world is a snake 420 m long, constructed by Norwegian children. Suppose the snake is laid out in a park as shown in Figure P3.39, forming two straight sides of a 105° angle, with one side 240 m long. Olaf and Inge run a race they invent. Inge runs directly from the tail of the snake to its head, and Olaf starts from the same place at the same moment but runs along the snake. (a) If both children run steadily at 12.0 km/h. Inge reaches the head of the snake how much earlier than Olaf? (b) If Inge runs the race again at a constant speed of 12.0 km/h. at what constant speed must Olaf run to reach the end of the snake at the same time as Inge?
Figure P3.39
Expert Solution & Answer
Trending nowThis is a popular solution!
Students have asked these similar questions
No chatgpt pls will upvote
No chatgpt pls will upvote
Solve and answer the question correctly please. Thank you!!
Chapter 3 Solutions
Physics for Scientists and Engineers
Ch. 3.2 - Which of the following are vector quantities and...Ch. 3.3 - The magnitudes of two vectors A and B are A = 12...Ch. 3.3 - If vector B is added to vector A, which two of the...Ch. 3.4 - Choose the correct response to make the sentence...Ch. 3.4 - For which of the following vectors is the...Ch. 3 - Two points in the xy plane have Cartesian...Ch. 3 - Prob. 2PCh. 3 - The polar coordinates of a certain point are (r =...Ch. 3 - Let the polar coordinates of the point (x, y) be...Ch. 3 - Why is the following situation impossible? A...
Ch. 3 - Vector A has a magnitude of 29 units and points in...Ch. 3 - A force F1 of magnitude 6.00 units acts on an...Ch. 3 - Three displacements are A=200m due south, B=250m...Ch. 3 - The displacement vectors A and B shown in Figure...Ch. 3 - A roller-coaster car moves 200 ft horizontally and...Ch. 3 - A minivan travels straight north in the right lane...Ch. 3 - A person walks 25.0 north of east for 3.10 km. How...Ch. 3 - Your dog is running around the grass in your back...Ch. 3 - Given the vectors A=2.00i+6.00j and B=3.00i2.00j,...Ch. 3 - The helicopter view in Fig. P3.15 shows two people...Ch. 3 - A snow-covered ski slope makes an angle of 35.0...Ch. 3 - Consider the three displacement vectors m, m,...Ch. 3 - Vector A has x and y components of 8.70 cm and...Ch. 3 - Prob. 19PCh. 3 - Given the displacement vectors A=(3i4j+4k)m and...Ch. 3 - Vector A has a negative x component 3.00 units in...Ch. 3 - Three displacement vectors of a croquet ball are...Ch. 3 - Prob. 23PCh. 3 - Prob. 24PCh. 3 - Use the component method to add the vectors A and...Ch. 3 - A girl delivering newspapers covers her route by...Ch. 3 - A man pushing a mop across a floor causes it to...Ch. 3 - Figure P3.28 illustrates typical proportions of...Ch. 3 - Review. As it passes over Grand Bahama Island, the...Ch. 3 - In an assembly operation illustrated in Figure...Ch. 3 - Review. You are standing on the ground at the...Ch. 3 - Why is the following situation impossible? A...Ch. 3 - In Figure P3.33, the line segment represents a...Ch. 3 - You are spending the summer as an assistant...Ch. 3 - A person going for a walk follows the path shown...Ch. 3 - A ferry transports tourists between three islands....Ch. 3 - Two vectors A and B have precisely equal...Ch. 3 - Two vectors A and B have precisely equal...Ch. 3 - Review. The biggest stuffed animal in the world is...Ch. 3 - Ecotourists use their global positioning system...Ch. 3 - A vector is given by R=2i+j+3k. Find (a) the...Ch. 3 - You are working as an assistant to an air-traffic...Ch. 3 - Review. The instantaneous position of an object is...Ch. 3 - Vectors A and B have equal magnitudes of 5.00. The...Ch. 3 - A rectangular parallelepiped has dimensions a, b,...Ch. 3 - A pirate has buried his treasure on an island with...
Additional Science Textbook Solutions
Find more solutions based on key concepts
Choose the best answer to each of the following. Explain your reasoning. If Earth were twice as far as it actua...
Cosmic Perspective Fundamentals
Define histology.
Fundamentals of Anatomy & Physiology (11th Edition)
How does the removal of hydrogen atoms from nutrient molecules result in a loss of energy from the nutrient mol...
SEELEY'S ANATOMY+PHYSIOLOGY
What process causes the Mediterranean intermediate Water MIW to become more dense than water in the adjacent At...
Applications and Investigations in Earth Science (9th Edition)
Describe the evolution of mammals, tracing their synapsid lineage from early amniote ancestors to true mammals....
Loose Leaf For Integrated Principles Of Zoology
Give the IUPAC name for each compound.
Organic Chemistry
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.Similar questions
- ་ The position of a particle is described by r = (300e 0.5t) mm and 0 = (0.3t²) rad, where t is in seconds. Part A Determine the magnitude of the particle's velocity at the instant t = 1.5 s. Express your answer to three significant figures and include the appropriate units. v = Value Submit Request Answer Part B ? Units Determine the magnitude of the particle's acceleration at the instant t = 1.5 s. Express your answer to three significant figures and include the appropriate units. a = Value A ? Unitsarrow_forwardSolve and answer the question correctly please. Thank you!!arrow_forwardSolve and answer the question correctly please. Thank you!!arrow_forward
- A spiral transition curve is used on railroads to connect a straight portion of the track with a curved portion. (Figure 1) Part A v = v₁ft/s 600 ft y = (106) x³ If the spiral is defined by the equation y = (106)³, where x and y are in feet, determine the magnitude of the acceleration of a train engine moving with a constant speed of v₁ = 30 ft/s when it is at point x = 600 ft. Express your answer to three significant figures and include the appropriate units. ? a = Value Unitsarrow_forwardsolve and answer the problem correctly please. Thank you!!arrow_forwardSolve and answer the question correctly please. Thank you!!arrow_forward
- Solve and answer the question correctly please. Thank you!!arrow_forwardWhen the motorcyclist is at A, he increases his speed along the vertical circular path at the rate of = (0.3t) ft/s², where t is in seconds. Take p = 360 ft. (Figure 1) Part A 60° Ρ B If he starts from rest at A, determine the magnitude of his velocity when he reaches B. Express your answer to three significant figures and include the appropriate units. v = Value Submit Request Answer ་ Part B ? Units If he starts from rest at A, determine the magnitude of his acceleration when he reaches B. Express your answer to three significant figures and include the appropriate units. 11 ? a = Value Unitsarrow_forwardThe car starts from rest at s = 0 and increases its speed at a₁ = 7 m/s². (Figure 1) Part A = 40 m Determine the time when the magnitude of acceleration becomes 20 m/s². Express your answer to three significant figures and include the appropriate units. ? t = Value Units Part B At what position s does this occur? Express your answer to three significant figures and include the appropriate units. s = Value Submit Request Answer ? Unitsarrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
College PhysicsPhysicsISBN:9781285737027Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
University Physics Volume 1PhysicsISBN:9781938168277Author:William Moebs, Samuel J. Ling, Jeff SannyPublisher:OpenStax - Rice University
Physics for Scientists and Engineers: Foundations...
Physics
ISBN:9781133939146
Author:Katz, Debora M.
Publisher:Cengage Learning
Physics for Scientists and Engineers, Technology ...
Physics
ISBN:9781305116399
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
Principles of Physics: A Calculus-Based Text
Physics
ISBN:9781133104261
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
College Physics
Physics
ISBN:9781285737027
Author:Raymond A. Serway, Chris Vuille
Publisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...
Physics
ISBN:9781337553292
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
University Physics Volume 1
Physics
ISBN:9781938168277
Author:William Moebs, Samuel J. Ling, Jeff Sanny
Publisher:OpenStax - Rice University
Introduction to Vectors and Their Operations; Author: Professor Dave Explains;https://www.youtube.com/watch?v=KBSCMTYaH1s;License: Standard YouTube License, CC-BY