Chapter 3, Problem 3.29P

To determine

Expression for vehicle’s speed as a function of time ($\dot{v}$).

Answer to Problem 3.29P

The vehicle’s speed as a function of time, $\dot{v}=1.906ft/s$.

Explanation of Solution

Given:

Weight of a rear wheel = 500 lb.

Weight of front wheel = 800 lb.

Weight of the body = 9000 lb.

Radius of rear wheel = 4ft.

Radius of front wheel = 2ft.

Concept used:

The object in question can translate in two dimensions and can rotate only about an axis that is perpendicular to the plane.

Object in question is a rigid body that moves in a plane passing through its mass center.

The motion of this object is defined by its translational motion in the plane and its rotational motion about an axis perpendicular to the plane. Two force equations describe the translational motion, and a moment equation is needed to describe the rotational motion.

The two force equations of the translational motion:

Using Newton’s laws for plane motion,

$\begin{array}{l}{f}_x=m{a}_{G_x}\\ f_y=m{a}_{G_y}\end{array}$

Where, $f_x$ and $f_y$ are the net forces acting on the object in the $x$ and $y$ directions, respectively. The mass center is located at point G. The quantities $m{a}_{G_x}$ and $m{a}_{G_y}$ are the accelerations of the mass center in the $x$ and $y$ directions relative to the ?xed $x$ - $y$ coordinate system.

For an objects’ planar motion which rotates only about an axis perpendicular to the plane, the equation of motion can be written down using Newton’s Second Law.

Equation of Motion: $I_o\dot{\omega }=M_o$

Where $I_o$ = Mass moment of Inertia of the body about point O.

$\dot{\omega }$ = Angular acceleration of the mass about an axis through a point O.

$M_o$ = Sum of the moments applied to the body about the point O.

Here the rotation is about the mass center, G, and the angular acceleration is taken as $\alpha$.

$\begin{array}{l}{I}_o\dot{\omega }=M_o\\ I_G\alpha =M_G\end{array}$

Where $I_G$ = Mass moment of Inertia of the body about point G.

$\alpha$ = Angular acceleration of the mass about an axis through a point G.

$M_G$ = Net moments acting on the body about the point G.

Derivation of Equation of motion:

Taking the body and the wheels as separate systems with axle,

Free body diagram of the body of the road roller:

$R_x$ and $R_y$ are the reaction forces between the axle and the body of the road roller.

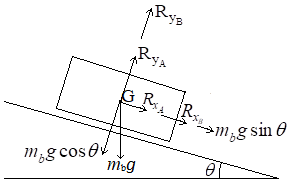
Force equations of the translational motion of body:

In the x -direction,

$\begin{array}{l}{f}_x=m{a}_{G_x}\\ R_{x_A}+R_{x_B}+m_{b}g\sin \theta =m_b\dot{v}\to \left(1\right)\end{array}$

The acceleration, $a_{G{}_x}$ is the same as $\dot{v}$.

In the y -direction,

$\begin{array}{l}{f}_y=m{a}_{G_y}\\ R_{y_A}+R_{y_B}-m_{b}g\cos \theta =m_b\dot{v}\\ R_{y_A}+R_{y_B}-m_{b}g\cos \theta =0\to \left(2\right)\end{array}$

The acceleration, $a_{G{}_y}$ is zero as the wheels do not bounce.

Free body diagram of the front wheel:

$f_t$ is the tangential component of the effect of the ground on the wheel.

$N$ is the tangential component of the effect of the ground on the wheel.

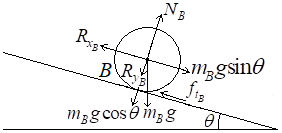
Sum of force in x -direction:

$\begin{array}{l}{\displaystyle \sum f_x}=m_B\dot{v}\\ m_{B}g\sin \theta -R_{x_B}-f_{t_B}=m_B\dot{v}\to \left(3\right)\end{array}$

Sum of force in y -direction:

$\begin{array}{l}{\displaystyle \sum f_y}=m_B\dot{v}\\ N_B-m_{B}g\cos \theta -R_{y_B}=m_B\dot{v}\\ N_B-m_{B}g\cos \theta -R_{y_B}=0\to \left(4\right)\end{array}$

The acceleration, $\dot{v}$ is zero as the wheels do not bounce.

Moments equation:

$\begin{array}{l}{I}_o\dot{\omega }=M_o\\ I_B\dot{\omega }=M_B\\ I_B\dot{\omega }=-r_B{f}_{t_B}\to \left(5\right)\end{array}$

Where, $r_B$ is the radius of the front wheel.

Assuming the wheels are rolling without slipping,

$\begin{array}{l}v=r_B\omega \\ \dot{v}=r_B\dot{\omega }\\ \dot{\omega }=\frac{\dot{v}}{r_B}\end{array}$

Modelling the wheels as a cylinder of radius $r_B$ ,

$\begin{array}{l}I=\frac{m{r}^2}{2}\\ I_B=\frac{m_B{r}_B{}^2}{2}\end{array}$

Substituting $\dot{\omega }$ and $I_B$ in equation $\left(5\right)$ ,

$\begin{array}{l}{I}_B\dot{\omega }=-r_B{f}_{t_B}\\ \frac{m_B{r}_B{}^2}{2}\times \frac{\dot{v}}{r_B}=-r_B{f}_{t_B}\\ \frac{m_B{r}_B{}^{\bcancel{2}}}{2}\times \frac{\dot{v}}{{\bcancel{r}}_B}=-r_B{f}_{t_B}\\ \frac{m_B{r}_B}{2}\times \dot{v}=-r_B{f}_{t_B}\\ f_{t_B}=-\frac{1}{r_B}\times \frac{m_B{r}_B}{2}\times \dot{v}\\ f_{t_B}=-\frac{1}{{\bcancel{r}}_B}\times \frac{m_B{\bcancel{r}}_B}{2}\times \dot{v}\\ f_{t_B}=-\frac{m_B}{2}\times \dot{v}\\ f_{t_B}=-\frac{m_B\dot{v}}{2}\end{array}$

Substitute $f_{t_B}$ in equation $\left(3\right)$ and find $R_{x_B}$.

$\begin{array}{l}{m}_{B}g\sin \theta -R_{x_B}-f_{t_B}=m_B\dot{v}\to \left(3\right)\\ f_{t_B}=-\frac{m_B\dot{v}}{2}\\ m_{B}g\sin \theta -R_{x_B}-\left(-\frac{m_B\dot{v}}{2}\right)=m_B\dot{v}\\ m_{B}g\sin \theta -R_{x_B}+\frac{m_B\dot{v}}{2}=m_B\dot{v}\\ R_{x_B}=m_{B}g\sin \theta +\frac{m_B\dot{v}}{2}-m_B\dot{v}\\ R_{x_B}=m_{B}g\sin \theta -\frac{m_B\dot{v}}{2}\end{array}$

Free body diagram of the rear wheels:

$f_t$ is the tangential component of the effect of the ground on the wheel.

$N$ is the tangential component of the effect of the ground on the wheel.

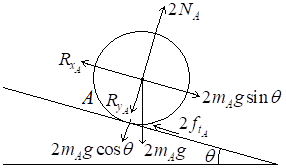
Sum of force in x -direction:

$\begin{array}{l}{\displaystyle \sum f_x}=m_A\dot{v}\\ 2m_{A}g\sin \theta -R_{x_A}-2f_{t_A}=2m_A\dot{v}\to \left(6\right)\end{array}$

Sum of force in y -direction:

$\begin{array}{l}{\displaystyle \sum f_y}=m_A\dot{v}\\ 2N_A-2m_{A}g\cos \theta -R_{y_A}=m_A\dot{v}\\ 2N_A-2m_{A}g\cos \theta -R_{y_A}=0\to \left(7\right)\end{array}$

The acceleration, $\dot{v}$ is zero as the wheels do not bounce.

Moments equation:

$\begin{array}{l}{I}_o\dot{\omega }=M_o\\ I_A\dot{\omega }=M_A\\ I_A\dot{\omega }=-2r_A{f}_{t_A}\to \left(8\right)\end{array}$

Where, $r_A$ is the radius of the rear wheel.

Assuming the wheels are rolling without slipping,

$\begin{array}{l}v=r_A\omega \\ \dot{v}=r_A\dot{\omega }\\ \dot{\omega }=\frac{\dot{v}}{r_A}\end{array}$

Modelling the wheels as a cylinder of radius $r_A$ ,

$\begin{array}{l}I=\frac{m{r}^2}{2}\\ I_A=\frac{m_A{r}_A{}^2}{2}\end{array}$

Substituting $\dot{\omega }$ and $I_A$ in equation $\left(8\right)$ ,

$\begin{array}{l}{I}_A\dot{\omega }=-2r_A{f}_{t_A}\\ \frac{m_A{r}_A{}^2}{2}\times \frac{\dot{v}}{r_A}=-2r_A{f}_{t_A}\\ \frac{m_A{r}_A{}^{\bcancel{2}}}{2}\times \frac{\dot{v}}{{\bcancel{r}}_A}=-2r_A{f}_{t_A}\\ \frac{m_A{r}_A}{2}\times \dot{v}=-2r_A{f}_{t_A}\\ f_{t_A}=-\frac{1}{2r_A}\times \frac{m_A{r}_A}{2}\times \dot{v}\\ f_{t_A}=-\frac{1}{2{\bcancel{r}}_A}\times \frac{m_A{\bcancel{r}}_A}{2}\times \dot{v}\\ f_{t_A}=-\frac{m_A}{4}\times \dot{v}\\ f_{t_A}=-\frac{m_A\dot{v}}{4}\end{array}$

Substitute $f_{t_A}$ in equation $\left(6\right)$ and find $R_{x_A}$.

$\begin{array}{l}2m_{A}g\sin \theta -R_{x_A}-2f_{t_A}=m_A\dot{v}\to \left(6\right)\\ f_{t_A}=-\frac{m_A\dot{v}}{4}\\ 2m_{A}g\sin \theta -R_{x_A}-2\left(-\frac{m_A\dot{v}}{4}\right)=m_A\dot{v}\\ 2m_{A}g\sin \theta -R_{x_A}+\frac{m_A\dot{v}}{2}=m_A\dot{v}\\ R_{x_A}=2m_{A}g\sin \theta +\frac{m_A\dot{v}}{2}-m_A\dot{v}\\ R_{x_A}=2m_{A}g\sin \theta -\frac{m_A\dot{v}}{2}\end{array}$

Substitute $R_{x_A}$ and $R_{x_B}$ in equation $\left(1\right)$

$\begin{array}{l}{R}_{x_A}+R_{x_B}+m_{b}g\sin \theta =m_b\dot{v}\to \left(1\right)\\ R_{x_A}=2m_{A}g\sin \theta -\frac{m_A\dot{v}}{2}{R}_{x_B}=m_{B}g\sin \theta -\frac{m_B\dot{v}}{2}\\ 2m_{A}g\sin \theta -\frac{m_A\dot{v}}{2}+m_{B}g\sin \theta -\frac{m_B\dot{v}}{2}+m_{b}g\sin \theta =m_b\dot{v}\\ 2m_{A}g\sin \theta +m_{B}g\sin \theta +m_{b}g\sin \theta =m_b\dot{v}+\frac{m_A\dot{v}}{2}+\frac{m_B\dot{v}}{2}\\ \dot{v}\left(m_b+\frac{m_A}{2}+\frac{m_B}{2}\right)=g\sin \theta \left(2m_A+m_B+m_b\right)\to \left(9\right)\end{array}$

Substitute the given values to equation $\left(9\right)$

$m_A$ = 500 lb.

$m_B$ = 800 lb.

$m_b$ = 9000 lb.

$\theta =10°$

$\begin{array}{l}\dot{v}\left(m_b+\frac{m_A}{2}+\frac{m_B}{2}\right)=g\sin \theta \left(2m_A+m_B+m_b\right)\\ \dot{v}\left(9000+\frac{500}{2}+\frac{800}{2}\right)=g\sin 10\left(2\times 500+800+9000\right)\\ 9650\dot{v}=10800g\sin 10\end{array}$

$\begin{array}{l}9650\dot{v}=10800g\sin 10\\ \dot{v}=\frac{10800g\sin 10}{9650}=1.905843993\\ \dot{v}=1.906ft/s\end{array}$.

Conclusion:

The vehicle’s speed as a function of time, $\dot{v}=1.906ft/s$.