Concept explainers
Videos
Refer to Figure 27-7. Dimension A with its tolerance is given in each of the following problems. Determine the maximum dimension (maximum limit) and the minimum dimension (minimum limit) for each.
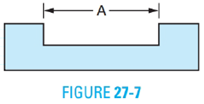
a. Dimension
maximum________.
minimum________.
b. Dimension
maximum________.
minimum________.
c. Dimension
maximum________.
minimum________.
d. Dimension
maximum________.
minimum________.
e. Dimension
maximum________.
minimum________.
f. Dimension
maximum________.
minimum________.
g. Dimension
maximum________.
minimum________.
h. Dimension
maximum________.
minimum________.
i. Dimension
maximum________.
minimum________.
j. Dimension
maximum________.
minimum________.
Want to see the full answer?
Check out a sample textbook solution
Chapter 27 Solutions
EBK MATHEMATICS FOR MACHINE TECHNOLOGY
- 2) Consider the matrix M = [1 2 3 4 5 0 2 3 4 5 00345 0 0 0 4 5 0 0 0 0 5 Determine whether the following statements are True or False. A) M is invertible. B) If R5 and Mx = x, then x = 0. C) The last row of M² is [0 0 0 0 25]. D) M can be transformed into the 5 × 5 identity matrix by a sequence of elementary row operations. E) det (M) 120 =arrow_forward3) Find an equation of the plane containing (0,0,0) and perpendicular to the line of intersection of the planes x + y + z = 3 and x y + z = 5. -arrow_forward1) In the xy-plane, what type of conic section is given by the equation - √√√(x − 1)² + (y − 1)² + √√√(x + 1)² + (y + 1)² : - = 3?arrow_forward
- 3) Let V be the vector space of all functions f: RR. Prove that each W below is a subspace of V. A) W={f|f(1) = 0} B) W = {f|f(1) = ƒ(3)} C) W={ff(x) = − f(x)}arrow_forwardTranslate the angument into symbole from Then determine whether the argument is valid or Invalid. You may use a truth table of, it applicable compare the argument’s symbolic form to a standard valid or invalid form. pot out of bed. The morning I did not get out of bed This moring Mat woke up. (1) Cidt the icon to view tables of standard vald and braild forms of arguments. Let prepresent."The morning Must woke up "and let a represent “This morning I got out of bed.” Seled the cared choice below and II in the answer ber with the symbolic form of the argument (Type the terms of your expression in the same order as they appear in the original expression) A. The argument is valid In symbolic form the argument is $\square $ B. The angunent is braid In symbolic form the argument is $\square $arrow_forwardWrite the prime factorization of 8. Use exponents when appropriate and order the factors from least to greatest (for example, 22.3.5). Submitarrow_forward
- No chatgpt pls will upvote Already got wrong chatgpt answer Plzarrow_forwardEvery chatgpt give wrong answer Plz no chatgptarrow_forward55 Logic and Set Theory: Continuum Hypothesis Task: Refer to Question 55 in the provided document. Link: https://drive.google.com/file/d/1wKSrun-GlxirS3IZ9qoHazb9tC440AZF/view?usp=sharing 5 6 Differential Geometry: Ricci Curvature Task: Refer to Question 56 in the provided document. Link: https://drive.google.com/file/d/1wKSrun-GlxirS31Z9qoHazb9tC440AZF/view?usp=sharingarrow_forward
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage