UNIVERSITY PHYSICS UCI PKG
11th Edition
ISBN: 9781323575208
Author: YOUNG
Publisher: PEARSON C
expand_more
expand_more
format_list_bulleted
Concept explainers
Textbook Question
Chapter 27, Problem 27.14E
The magnetic field
Figure E27.14
Expert Solution & Answer
Learn your wayIncludes step-by-step video
schedule04:43
Chapter 27 Solutions
UNIVERSITY PHYSICS UCI PKG
Ch. 27.1 - Suppose you cut off the part of the compass needle...Ch. 27.2 - Prob. 27.2TYUCh. 27.3 - Imagine moving along the axis of the...Ch. 27.4 - Prob. 27.4TYUCh. 27.5 - In Example 27.6 He+ ions with charge +e move at...Ch. 27.6 - The accompanying figure shows a top view of two...Ch. 27.7 - Figure 27.13c depicts the magnetic field lines due...Ch. 27.8 - Prob. 27.8TYUCh. 27.9 - A copper wire of square cross section is oriented...Ch. 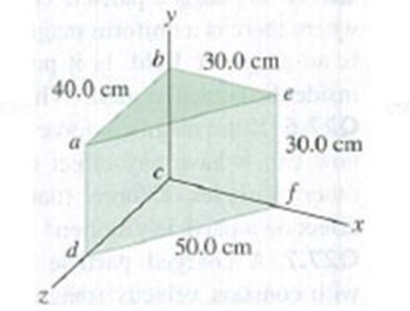27 - Can a charged particle move through a magnetic...
Ch. 27 - Prob. 27.2DQCh. 27 - Section 27.2 describes a procedure for finding the...Ch. 27 - The magnetic force on a moving charged particle is...Ch. 27 - A charged particle is fired into a cubical region...Ch. 27 - If the magnetic force does no work on a charged...Ch. 27 - A charged particle moves through a region of space...Ch. 27 - How might a loop of wire carrying a current be...Ch. 27 - How could the direction of a magnetic field be...Ch. 27 - A loose, floppy loop of wire is carrying current...Ch. 27 - Prob. 27.11DQCh. 27 - Each of the lettered points at the corners of the...Ch. 27 - A student claims that if lightning strikes a metal...Ch. 27 - Prob. 27.14DQCh. 27 - The magnetic force acting on a charged particle...Ch. 27 - When the polarity of the voltage applied to a dc...Ch. 27 - Prob. 27.17DQCh. 27 - Prob. 27.18DQCh. 27 - A particle with a charge of 1.24 108C is moving...Ch. 27 - A particle of mass 0.195 g carries a charge of...Ch. 27 - In a 1.25-T magnetic field directed vertically...Ch. 27 - A particle with mass 1.81 103 kg and a charge of...Ch. 27 - An electron experiences a magnetic force of...Ch. 27 - An electron moves at 1.40 106m/s through a region...Ch. 27 - CP A particle with charge 7.80 C is moving with...Ch. 27 - CP A particle with charge 5.60 nC is moving in a...Ch. 27 - A group of particles is traveling in a magnetic...Ch. 27 - A flat, square surface with side length 3.40 cm is...Ch. 27 - A circular area with a radius of 6.50 cm lies in...Ch. 27 - A horizontal rectangular surface has dimensions...Ch. 27 - An open plastic soda bottle with an opening...Ch. 27 - The magnetic field B in a certain region is 0.128...Ch. 27 - An election at point A in Fig. E27.15 has a speed...Ch. 27 - Repeat Exercise 27.15 for the case in which the...Ch. 27 - CP A 150-g ball containing 4.00 108 excess...Ch. 27 - An alpha particle (a He nucleus, containing two...Ch. 27 - In an experiment with cosmic rays, a vertical beam...Ch. 27 - BIO Cyclotrons are widely used in nuclear medicine...Ch. 27 - Prob. 27.21ECh. 27 - In a cyclotron, the orbital radius of protons with...Ch. 27 - An electron in the beam of a cathode-ray tube is...Ch. 27 - A beam of protons traveling at 1.20 km/s enters a...Ch. 27 - A proton (q = 1.60 1019 C, m = 1.67 1027 kg)...Ch. 27 - A singly charged ion of 7Li (an isotope of...Ch. 27 - Crossed E and B Fields. A particle with initial...Ch. 27 - (a) What is the speed of a beam of electrons when...Ch. 27 - A 150-V battery is connected across two parallel...Ch. 27 - A singly ionized (one electron removed) 40K atom...Ch. 27 - Singly ionized (one electron removed) atoms are...Ch. 27 - In the Bainbridge mass spectrometer (see Fig....Ch. 27 - Prob. 27.33ECh. 27 - A straight, 2.5-m wire carries a typical household...Ch. 27 - A long wire carrying 4.50 A of current makes two...Ch. 27 - An electromagnet produces a magnetic field of...Ch. 27 - A thin, 50.0-cm-long metal bar with mass 750 g...Ch. 27 - A straight, vertical wire carries a current of...Ch. 27 - Prob. 27.39ECh. 27 - The plane of a 5.0 cm X 8.0 cm rectangular loop of...Ch. 27 - The 20.0 cm 35.0 cm rectangular circuit shown in...Ch. 27 - A rectangular coil of wire, 22.0 cm by 35.0 cm and...Ch. 27 - CP A uniform rectangular coil of total mass 212 g...Ch. 27 - Both circular coils A and B (Fig. E27.44) have...Ch. 27 - Prob. 27.45ECh. 27 - Prob. 27.46ECh. 27 - Prob. 27.47ECh. 27 - A dc motor with its rotor and field coils...Ch. 27 - Figure E27.49 shows a portion of a silver ribbon...Ch. 27 - Prob. 27.50ECh. 27 - When a particle of charge q 0 moves with a...Ch. 27 - A particle with charge 7.26 108C is moving in a...Ch. 27 - Prob. 27.53PCh. 27 - Prob. 27.54PCh. 27 - Prob. 27.55PCh. 27 - The magnetic poles of a small cyclotron produce a...Ch. 27 - A particle with negative charge q and mass m =...Ch. 27 - A particle of charge q 0 is moving at speed in...Ch. 27 - Suppose the electric field between the plates in...Ch. 27 - Mass Spectrograph. A mass spectrograph is used to...Ch. 27 - A straight piece of conducting wire with mass M...Ch. 27 - CP A 2.60-N metal bar, 0.850 m long and having a...Ch. 27 - BIO Determining Diet. One method for determining...Ch. 27 - CP A plastic circular loop has radius R, and a...Ch. 27 - Prob. 27.65PCh. 27 - A wire 25.0 cm long lies along the z-axis and...Ch. 27 - A long wire carrying 6.50 A of current makes two...Ch. 27 - The rectangular loop shown in Fig. P27.68 is...Ch. 27 - Prob. 27.69PCh. 27 - Prob. 27.70PCh. 27 - The loop of wire shown in Fig. P27.71 forms a...Ch. 27 - CP A uniform bar has mass 0.0120 kg and is 30.0 cm...Ch. 27 - CALC A Voice Coil. It was shown in Section 27.7...Ch. 27 - Prob. 27.74PCh. 27 - CALC Force on a Current Loop in a Nonuniform...Ch. 27 - Quark Model of the Neutron. The neutron is a...Ch. 27 - A circular loop of wire with area A lies in the...Ch. 27 - DATA You are using a type of mass spectrometer to...Ch. 27 - Prob. 27.79PCh. 27 - DATA You are a technician testing the operation of...Ch. 27 - A particle with charge 2.15 C and mass 3.20 1011...Ch. 27 - Prob. 27.82CPCh. 27 - If a proton is exposed to an external magnetic...Ch. 27 - BIO MAGNETIC FIELDS AND MRI. Magnetic resonance...Ch. 27 - The large magnetic fields used in MRI can produce...
Additional Science Textbook Solutions
Find more solutions based on key concepts
How does a person standing on the ground explain why you, sitting on the left side of a slippery back car seat,...
College Physics
Discuss a few ways of reaching the stars (other than nuclear rockets) that are, at least in principle, within o...
Life in the Universe (4th Edition)
Explain all answers clearly, with complete sentences and proper essay structure if needed. An asterisk (*) desi...
The Cosmic Perspective Fundamentals (2nd Edition)
25. A capacitor is connected to an AC generator. As the generator’s frequency is increased, the current in the ...
College Physics: A Strategic Approach (4th Edition)
One end of an iron rod 40 cm long and 3.0 cm in diameter is in ice water, the other in boiling water (Fig. 16.1...
Essential University Physics: Volume 1 (3rd Edition)
Answer Problems 3 through 5 by choosing one of the eight labeled acceleration vectors or selecting option I: = ...
Physics for Scientists and Engineers: A Strategic Approach with Modern Physics (4th Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.Similar questions
- A long, straight wire of radius R caries a current I that is distributed uniformly over the cross-section of the wire. At what distance from the axis of the wire is the magnitude of the magnetic field a maximum?arrow_forwardA strip of copper is placed in a uniform magnetic field of magnitude 2.5 T. The Hall electric field is measured to be 1.5103V/m (a) What is the drift speed of the conduction electrons? (b) Assuming that n =8.01028 elections per cubic meter and that the cross-sectional area of the strip is 5.0106m2 , calculate the current in the ship, (c) What is the Hall coefficient 1/nq?arrow_forwardAcircularcoiofwireofradius5.Ocmhas2Otums and carries a current of 2.0 A. The coil lies in a magnetic field of magnitude 0.50 T that is directed parallel to the plane of the coil. (a) What is the magnetic dipole moment of the coil? (b) What is the torque on the coil?arrow_forward
- A uniform magnetic field B=5.44104iT passes through a closed surface with a slanted top as shown in Figure P31.59. a. Given the dimensions and orientation of the closed surface shown, what is the magnetic flux through the slanted top of the surface? b. What is the net magnetic flux through the entire closed surface?arrow_forwardA circular loop of radius R carries a current I. At what distance along the axis of the loop is the magnetic field one- half its value at the center of the loop?arrow_forwardA toroid has a major radius R and a minor radius r and is tightly wound with N turns of wire on a hollow cardboard torus. Figure P31.6 shows half of this toroid, allowing us to see its cross section. If R r, the magnetic field in the region enclosed by the wire is essentially the same as the magnetic field of a solenoid that has been bent into a large circle of radius R. Modeling the field as the uniform field of a long solenoid, show that the inductance of such a toroid is approximately L=120N2r2R Figure P31.6arrow_forward
- When the current through a circular loop is 6.0 A, the magnetic field at its center is 2.0104 T. What is the radius of the loop?arrow_forwardA long, solid, cylindrical conductor of radius 3.0 cm carries a current of 50 A distributed uniformly over its cross-section. Plot the magnetic field as a function of the radial distance r from the center of the conductor.arrow_forwardTwo infinitely long current-carrying wires run parallel in the xy plane and are each a distance d = 11.0 cm from the y axis (Fig. P30.83). The current in both wires is I = 5.00 A in the negative y direction. a. Draw a sketch of the magnetic field pattern in the xz plane due to the two wires. What is the magnitude of the magnetic field due to the two wires b. at the origin and c. as a function of z along the z axis, at x = y = 0? FIGURE P30.83arrow_forward
- Two long coaxial copper tubes, each of length L, are connected to a battery of voltage V. The inner tube has inner radius o and outer radius b, and the outer tube has inner radius c and outer radius d. The tubes are then disconnected from the battery and rotated in the same direction at angular speed of radians per second about their common axis. Find the magnetic field (a) at a point inside the space enclosed by the inner tube r d. (Hint: Hunk of copper tubes as a capacitor and find the charge density based on the voltage applied, Q=VC, C=20LIn(c/b) .)arrow_forwardA long, straight, cylindrical conductor contains a cylindrical cavity whose axis is displaced by n from the axis of the conductor, as shown in the accompanying figure. The current density in the conductor is given by J=J0k, where J0 is a constant and k is along the axis of the conductor. Calculate the magnetic field at an arbitrary point P in the cavity by superimposing the field of a solid cylindrical conductor with radius R1and current density Jonto the field of a solid cylindrical conductor with radius R2and current density J . Then use the fact that the appropriate azimuthal unit vectors can be expressed as 1=kr1and 2=kr2 to show that everywhere inside the cavity the magnetic field is given by the constant B=120J0ka , where a=r1r2 and r1=r1r1 is the position of P relative to the center of the conductor and r2=r2r2 is the position of P relative to the center of the cavity.arrow_forwardAssume the region to the right of a certain plane contains a uniform magnetic field of magnitude 1.00 mT and the field is zero in the region to the left of the plane as shown in Figure P22.71. An electron, originally traveling perpendicular to the boundary plane, passes into the region of the field. (a) Determine the time interval required for the electron to leave the field-filled region, noting that the electrons path is a semicircle. (b) Assuming the maximum depth of penetration into the field is 2.00 cm, find the kinetic energy of the electron.arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...
Physics
ISBN:9781133939146
Author:Katz, Debora M.
Publisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...
Physics
ISBN:9781337553292
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
Principles of Physics: A Calculus-Based Text
Physics
ISBN:9781133104261
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
Physics for Scientists and Engineers
Physics
ISBN:9781337553278
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
Physics for Scientists and Engineers, Technology ...
Physics
ISBN:9781305116399
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning