Chapter 2.5, Problem 2.115P

For the rectangular plate of Probs. 2.109 and 2.110, determine the tension in each of the three cables knowing that the weight of the plate is 792 N.

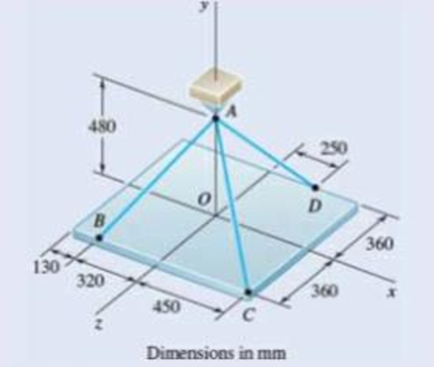
Fig. P2.109 and P2.110

To determine

The tension in three cables shown in figure $\text{P2}\text{.109}$ , knowing that weight of the plate is $792\text{N}$.

Answer to Problem 2.115P

The tension in cable $AB$ is $\underset{\_}{510\text{N}}$ , the tension in the cable $AC$ is $\underset{\_}{56.2\text{N}}$ and the tension in the cable $AD$ is $\underset{\_}{536\text{N}}$.

Explanation of Solution

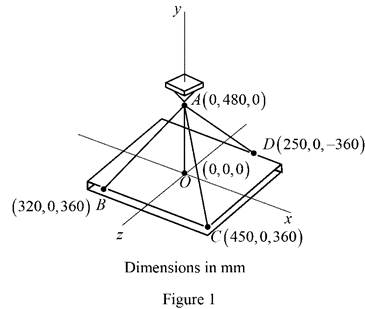
The sketch of plate supported by three cables is shown in figure 1.

Free body diagram at $\text{A}$ is shown in figure 2.

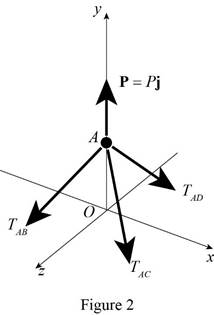
Here, $T_{AB}$ is the magnitude of tension in cable $AB$, $T_{AC}$ is the magnitude of tension in cable $AC$, $T_{AD}$ is the magnitude of tension in the cable $AD$ , $p$ is the magnitude of force $P$ exerted by the support on point $A$ which is equal to the weight of the rectangular plate.

The weight of the plate is $792\text{N}$.

Let $T_{AB}$, $T_{AC}$,$T_{AD}$ and $P$ are the tension vector in cable $AB$, $AC$, $AD$ and force exerted at $A$ in the upward direction due to the weight of the plate.

Let $i$ , $j$ and $k$ are the unit vectors along the of $x,y\text{and}z$ direction.

Write the equation of vector distance $AB$.

$\overrightarrow{AB}=\left(x_2-x_1\right)i+(y_2-y_1)j+(z_2-z_1)k$ (I)

Here, $\overrightarrow{AB}$ is the vector distance of the cable $AB$, the variables $x_{1,}{y}_1{\text{and z}}_1$ are the coordinates of point $A$ and $x_2,y_2{\text{and z}}_2$ are the coordinates of point $B$.

Write the vector distance of the cable $AC$.

$\overrightarrow{AC}=\left(x_3-x_1\right)i+(y_3-y_1)j+(z_3-z_1)k$ (II)

Here, $\overrightarrow{AC}$ is the vector distance of the cable $AC$, the variables $x_{1,}{y}_1{\text{and z}}_1$ are the coordinates of point $A$ and $x_3,y_3{\text{and z}}_3$ are the coordinates of point $C$.

Write the vector distance of the cable $AD$.

$\overrightarrow{AD}=\left(x_4-x_1\right)i+(y_4-y_1)j+(z_4-z_1)k$ (III)

Here, $\overrightarrow{AD}$ is the vector distance of the cable $AD$, the variables $x_{1,}{y}_1{\text{and z}}_1$ are the coordinates of point $A$ and $x_4,y_4{\text{and z}}_4$ are the coordinates of point $D$.

Write the equation of tension in the cable $AB$.

$T_{AB}={\lambda }_{AB}{T}_{AB}$ (IV)

Here, $T_{AB}$ is the tension in the cable $AB$ , $T_{AB}$ is the magnitude of the tension in the cable $AB$ and ${\lambda }_{AB}$ is the unit vector in the direction of $AB$.

Write the equation of ${\lambda }_{AB}$.

${\lambda }_{AB}=\frac{\overrightarrow{AB}}{AB}$ (V)

Write the equation of tension in the cable $AC$.

$T_{AC}={\lambda }_{AC}{T}_{AC}$ (VI)

Here, $T_{AC}$ is the tension in the cable $AC$ , $T_{AC}$ is the magnitude of the tension in the cable $AC$ and ${\lambda }_{AC}$ is the unit vector in the direction of $AC$.

Write the equation of ${\lambda }_{AC}$.

${\lambda }_{AC}=\frac{\overrightarrow{AC}}{AC}$ (VII)

Write the equation of tension in the cable $AD$.

$T_{AD}={\lambda }_{AD}{T}_{AD}$ (VIII)

Here, $T_{AD}$ is the tension in the cable $AD$ , $T_{AD}$ is the magnitude of the tension in the cable $AD$ and ${\lambda }_{AD}$ is the unit vector in the direction of $AD$.

Write the equation of ${\lambda }_{AD}$.

${\lambda }_{AD}=\frac{\overrightarrow{AD}}{AD}$ (IX)

Write the equation of force exerting at point $A$ along $y$ direction.

$P=Pj$ (X)

Here, $P$ is the force exerted at $A$ in the upward direction due to the weight of the plate.

Write the equilibrium condition for the forces at $A$.

${\displaystyle \sum F}=0$

Here, F is the force

The above equation implies that at equilibrium, total force acting on the cable at $A$ is zero.

Refer figure 2 and write the equation of equilibrium of forces at A.

$T_{AB}+T_{AC}+T_{AD}+P=0$

Conclusion:

Substitute $0\text{mm}$ for $x_1$ , $480\text{mm}$ for $y_1$ , $0\text{mm}$ for $z_1$ , $320\text{mm}$ for $x_2$ , $0\text{mm}$ for $y_2$ and $360\text{mm}$ for $z_2$ in equation (I) to get $\overrightarrow{AB}$.

$\overrightarrow{AB}=-\left(320\text{mm}\right)i-(480\text{mm})j+(360\text{mm})k$

Calculate the magnitude of $\overrightarrow{AB}$.

$\begin{array}{c}AB=\sqrt{{\left(-320\text{mm}\right)}^2+{(-480\text{mm})}^2+{(360\text{mm})}^2}\\ =\text{680}\text{mm}\end{array}$

Substitute $0\text{mm}$ for $x_1$ , $480\text{mm}$ for $y_1$ , $0\text{mm}$ for $z_1$ , $450\text{mm}$ for $x_3$ , $0\text{mm}$ for $y_3$ and $360\text{mm}$ for $z_3$ in equation (II) to get $\overrightarrow{AC}$.

$\overrightarrow{AC}=\left(450\text{mm}\right)i-(480\text{mm})j+(360\text{mm})k$

Calculate the magnitude of $\overrightarrow{AC}$.

$\begin{array}{c}AC=\sqrt{{\left(450\text{mm}\right)}^2+{(-480\text{mm})}^2+{(360\text{mm})}^2}\\ =750\text{mm}\end{array}$

Substitute $0\text{mm}$ for $x_1$ , $480\text{mm}$ for $y_1$ , $0\text{mm}$ for $z_1$ , $250\text{mm}$ for $x_4$ , $0\text{mm}$ for $y_4$ and $-360\text{mm}$ for $z_4$ in equation (III) to get $\overrightarrow{AD}$.

$\overrightarrow{AD}=\left(250\text{mm}\right)i-(480\text{mm})j-(360\text{mm})k$

Calculate the magnitude of $\overrightarrow{AD}$.

$\begin{array}{c}AD=\sqrt{{\left(250\text{mm}\right)}^2-{(480\text{mm})}^2-{(360\text{mm})}^2}\\ =650\text{mm}\end{array}$

Substitute $\overrightarrow{AB}=-\left(320\text{mm}\right)i-(480\text{mm})j+(360\text{mm})k$ for $\overrightarrow{AB}$ and $680\text{mm}$ for $AB$ in equation (V) to get ${\lambda }_{AB}$.

$\begin{array}{c}{\lambda }_{AB}=\frac{-\left(320\text{mm}\right)i-(480\text{mm})j+(360\text{mm})k}{680\text{mm}}\\ =-\frac{8}{17}i-\frac{12}{17}j+\frac{19}{17}k\end{array}$

Substitute $-\frac{8}{17}i-\frac{12}{17}j+\frac{19}{17}k$ for ${\lambda }_{AB}$ in equation (IV) to get $T_{AB}$.

$T_{AB}=\left(-\frac{8}{17}i-\frac{12}{17}j+\frac{19}{17}k\right)T_{AB}$

Substitute $\left(250\text{mm}\right)i-(480\text{mm})j-(360\text{mm})k$ for $\overrightarrow{AC}$ and $7.40\text{mm}$ for $AC$ in equation
(VII) to get ${\lambda }_{AC}$.

$\begin{array}{c}{\lambda }_{AC}=\frac{\left(250\text{mm}\right)i-(480\text{mm})j-(360\text{mm})k}{750\text{mm}}\\ =0.6i-0.64j+0.48k\end{array}$

Substitute $0.6i-0.64j+0.48k$ for ${\lambda }_{AC}$ in equation (VI) to get $T_{AC}$.

$T_{AC}=\left(0.6i-0.64j+0.48k\right)T_{AC}$

Substitute $\left(250\text{mm}\right)i-(480\text{mm})j-(360\text{mm})k$ for $\overrightarrow{AD}$ and $\text{650}\text{mm}$ for $AD$ in equation (IX) to get ${\lambda }_{AD}$.

$\begin{array}{c}{\lambda }_{AD}=\frac{\left(250\text{mm}\right)i-(480\text{mm})j-(360\text{mm})k}{\text{650}\text{mm}}\\ =\frac{5}{13}i-\frac{9.6}{13}j-\frac{7.2}{13}k\end{array}$

Substitute $\frac{5}{13}i-\frac{9.6}{13}j-\frac{7.2}{13}k$ for ${\lambda }_{AD}$ in equation (VIII) to get $T_{AD}$.

$T_{AD}=\left(\frac{5}{13}i-\frac{9.6}{13}j-\frac{7.2}{13}k\right)T_{AD}$

Substitute $\left(-\frac{8}{17}i-\frac{12}{17}j+\frac{19}{17}k\right)T_{AB}$ for $T_{AB}$ , $\left(0.6i-0.64j+0.48k\right)T_{AC}$ for $T_{AC}$ , $\left(\frac{5}{13}i-\frac{9.6}{13}j-\frac{7.2}{13}k\right)T_{AD}$, $792j$ for $P$ in the in equation (XI) to get force $P$ exerted at point $A$.

$\begin{array}{l}\left(-\frac{8}{17}i-\frac{12}{17}j+\frac{9}{17}k\right)T_{AB}+\left(0.6i-0.64j+0.48k\right)T_{AC}+\left(\frac{5}{13}i-\frac{9.6}{13}j-\frac{7.2}{13}k\right)T_{AD}+792j=0\\ \left(-\frac{8}{17}{T}_{AB}+0.6T_{AC}+\frac{5}{13}{T}_{AD}\right)i+\left(-\frac{12}{17}{T}_{AB}-0.64T_{AC}+-\frac{9.6}{13}{T}_{AD}+792\right)j+\left(+\frac{9}{17}{T}_{AB}+0.48T_{AC}-\frac{7.2}{13}{T}_{AD}\right)k=0\end{array}$

Since total force is zero. Equate force along each direction as zero.

$-\frac{8}{17}{T}_{AB}+0.6T_{AC}+\frac{5}{13}{T}_{AD}=0$ (XII)

$-\frac{12}{17}{T}_{AB}-0.64T_{AC}+-\frac{9.6}{13}{T}_{AD}+792=0$ (XIII)

$+\frac{9}{17}{T}_{AB}+0.48T_{AC}-\frac{7.2}{13}{T}_{AD}\text{=}\text{0}$ (XIV)

Multiply equation (XII) with 12 and equation (XIV) with $-9$ and add to get new equation.

$\begin{array}{l}-\frac{96}{17}{T}_{AB}+7.2T_{AC}+\frac{60}{13}{T}_{AD}+\frac{96}{17}{T}_{AB}+5.12T_{AC}+\frac{76.8}{13}{T}_{AD}-6336=0\\ \end{array}$

$12.32T_{AC}+\frac{136.8}{13}{T}_{AD}-6336=0$ (XV)

Multiply equation (XII) with $9$ and equation (XIV) with $8$ and add to get new equation.

$\begin{array}{c}-\frac{72}{17}{T}_{AB}+5.4T_{AC}+\frac{45}{13}{T}_{AD}+\frac{72}{17}{T}_{AB}+3.84T_{AC}-\frac{57.6}{13}{T}_{AD}\text{=}\text{0}\\ 5.4T_{AC}+\frac{45}{13}{T}_{AD}+3.84T_{AC}-\frac{57.6}{13}{T}_{AD}\text{=}\text{0}\end{array}$
$\text{9}\text{.24}{T}_{AC}\frac{-12.6}{13}{T}_{AD}=0$ (XVI)

Multiply equation (XV) with $12.6$ and (XVI) with $136.8$ to get $T_{AC}$.

$155.232T_{AC}+\frac{1723.68}{13}{T}_{AD}-79833.6\text{N}+\text{1264}\text{.032}{T}_{AC}-\frac{1723.68}{13}{T}_{AD}=0$

$\begin{array}{c}\left(155.232+\text{1264}\text{.032}\right)T_{AC}=79833.6\text{N}\\ {\text{T}}_{AC}=56.25\text{N}\end{array}$

Substitute $56.2\text{N}$ for $T_{AC}$ in equation (XVI) to get $T_{AD}$.

$\begin{array}{c}\text{9}\text{.24}\left(56.25\text{N}\right)\frac{-12.6}{13}{T}_{AD}=0\\ T_{AD}=536.25\text{N}\end{array}$

Substitute $56.25\text{N}$ for $T_{AC}$ and $536.25\text{N}$ for $T_{AD}$ in equation (XII) to get $T_{AB}$.

$-\frac{8}{17}{T}_{AB}+0.6\left(56.25\text{N}\right)+\frac{5}{13}\left(536.25\text{N}\right)=0$

$T_{AB}=\text{510}\text{N}$

Therefore, the tension in cable $AB$ is $\underset{\_}{510\text{N}}$ , the tension in the cable $AC$ is $\underset{\_}{56.2\text{N}}$ and the tension in the cable $AD$ is $\underset{\_}{536\text{N}}$.