Applied Physics (11th Edition)
11th Edition
ISBN: 9780134159386
Author: Dale Ewen, Neill Schurter, Erik Gundersen
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 2.3, Problem 5P
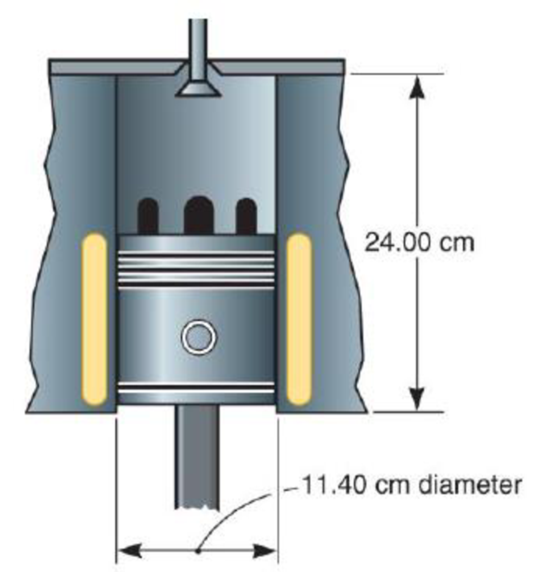
Find the cross-sectional area of the cylinder.
Figure 2.6
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
Sketch the harmonic.
For number 11 please sketch the harmonic on graphing paper.
#
E
94
20
13.
Time
a) What is the frequency of the above wave?
b) What is the period?
c) Highlight the second cycle
d) Sketch the sine wave of the second harmonic of this wave
%
7
&
5
6
7
8
* ∞
Y
U
9
0
0
P
150
Chapter 2 Solutions
Applied Physics (11th Edition)
Ch. 2.1 - =stforSCh. 2.1 - a=tforVCh. 2.1 - w = mg for mCh. 2.1 - F = ma for aCh. 2.1 - E = IR for RCh. 2.1 - V = lwh for wCh. 2.1 - Ep = mgh for gCh. 2.1 - Ep = mgh for hCh. 2.1 - 2 = 2gh for hCh. 2.1 - XL = 2 f L for f
Ch. 2.1 - P=WtforWCh. 2.1 - p=FAforFCh. 2.1 - P=WtforiCh. 2.1 - p=FAforACh. 2.1 - Ek=12m2formCh. 2.1 - Ek=12m2Ch. 2.1 - W = Fs for SCh. 2.1 - f = i + at for aCh. 2.1 - V = E Ir for lCh. 2.1 - 2 = 1 + at for tCh. 2.1 - R=2PforPCh. 2.1 - R=kLd2forLCh. 2.1 - Prob. 23PCh. 2.1 - XC=12fCforfCh. 2.1 - R=LAforLCh. 2.1 - RT = R1 + R2 + R3 + R4 for R3Ch. 2.1 - Q1 = P(Q2 Q1) for Q2Ch. 2.1 - ISIP=NPNSforIPCh. 2.1 - VPVS=NPNSforNSCh. 2.1 - Prob. 31PCh. 2.1 - Prob. 32PCh. 2.1 - Prob. 33PCh. 2.1 - Ft=m(V2V1)forV1Ch. 2.1 - Q=I2RtJforRCh. 2.1 - x=xi+it+12at2forX1Ch. 2.1 - A = r2 for r, Where r is a radiusCh. 2.1 - V = r2h for r, Where r is a radiusCh. 2.1 - R=kLd2 for d, where d is a diameterCh. 2.1 - V=13r2h for r, where r is a radiusCh. 2.1 - Solve each formula for the quantity given. 41....Ch. 2.1 - Solve each formula for the quantity given. 42....Ch. 2.2 - For each formula, (a) solve for the indicated...Ch. 2.2 - For each formula, (a) solve for the indicated...Ch. 2.2 - For each formula, (a) solve for the indicated...Ch. 2.2 - For each formula, (a) solve for the indicated...Ch. 2.2 - For each formula, (a) solve for the indicated...Ch. 2.2 - For each formula, (a) solve for the indicated...Ch. 2.2 - For each formula, (a) solve for the indicated...Ch. 2.2 - For each formula, (a) solve for the indicated...Ch. 2.2 - For each formula, (a) solve for the indicated...Ch. 2.2 - For each formula, (a) solve for the indicated...Ch. 2.2 - For each formula, (a) solve for the indicated...Ch. 2.2 - For each formula, (a) solve for the indicated...Ch. 2.2 - For each formula, (a) solve for the indicated...Ch. 2.2 - For each formula, (a) solve for the indicated...Ch. 2.2 - For each formula, (a) solve for the indicated...Ch. 2.2 - For each formula, (a) solve for the indicated...Ch. 2.2 - For each formula, (a) solve for the indicated...Ch. 2.2 - For each formula, (a) solve for the indicated...Ch. 2.2 - For each formula, (a) solve for the indicated...Ch. 2.2 - For each formula, (a) solve for the indicated...Ch. 2.3 - Find the volume of the box in Fig. 2.3. Figure 2.3Ch. 2.3 - Find the volume of a cylinder whose height is 7.50...Ch. 2.3 - Find the volume of a cone whose height is 9.30 cm...Ch. 2.3 - Find the volume of the cylinder. Figure 2.6Ch. 2.3 - Find the cross-sectional area of the cylinder....Ch. 2.3 - Find the lateral surface area of the cylinder....Ch. 2.3 - Find the total volume of the building shown in...Ch. 2.3 - Find the cross-sectional area of the concrete...Ch. 2.3 - Find the volume of a rectangular storage facility...Ch. 2.3 - Find the cross-sectional area of a piston head...Ch. 2.3 - Find the area of a right triangle that has legs of...Ch. 2.3 - Find the length of the hypotenuse of the right...Ch. 2.3 - Find the cross-sectional area of a pipe with outer...Ch. 2.3 - Find the volume of a spherical water tank with...Ch. 2.3 - The area of a rectangular parking lot is 900m2. If...Ch. 2.3 - The volume of a rectangular crate is 192 ft3. If...Ch. 2.3 - Find the volume of a brake cylinder whose diameter...Ch. 2.3 - Find the volume of a tractor engine cylinder whose...Ch. 2.3 - A cylindrical silo has a circumference of 29.5 m....Ch. 2.3 - If the silo in Problem 19 has a capacity of...Ch. 2.3 - A wheel 30.0 cm in diameter moving along level...Ch. 2.3 - The side of the silo in Problems 19 and 20 needs...Ch. 2.3 - You are asked to design a cylindrical water tank...Ch. 2.3 - If the height of the water tank in Problem 23 were...Ch. 2.3 - A ceiling is 12.0 ft by 15.0 ft. How many...Ch. 2.3 - Find the cross-sectional area of the dovetail...Ch. 2.3 - Find tile volume of the storage bin shown in Fig....Ch. 2.3 - The maximum cross-sectional area of a spherical...Ch. 2.3 - How many cubic yards of concrete are needed to...Ch. 2.3 - What length of sidewalk 4.00 in. thick and 4.00 ft...Ch. 2.3 - Find the volume of each figure.Ch. 2.3 - Inside diameter: 20.0 cm Outside diameter: 50.0 cmCh. 2 - A formula is a. the amount of each value needed....Ch. 2 - Subscripts are a. the same as exponents. b. used...Ch. 2 - A working equation a. is derived from the basic...Ch. 2 - Cite two examples in industry in which formulas...Ch. 2 - How are subscripts used in measurement?Ch. 2 - Why is reading the problem carefully the most...Ch. 2 - How can making a sketch help in problem solving?Ch. 2 - What do we call the relationship between data that...Ch. 2 - How is a working equation different from a basic...Ch. 2 - How can analysis of the units in a problem assist...Ch. 2 - How can making an estimate of your answer assist...Ch. 2 - Solve F = ma for (a) m and (b) a.Ch. 2 - Solve =2ghforh.Ch. 2 - Solve s=12(f+i)tforf.Ch. 2 - Prob. 4RPCh. 2 - Given P = a + b + c, with P = 36 ft, a = 12 ft,...Ch. 2 - Given A=(a+b2)h, with A=210m2, b = 16.0 m, and h =...Ch. 2 - Given A = r2, if A. = 15.0 m2, find r.Ch. 2 - Given A=12bh, if b = 12.2 cm and h = 20.0 cm, what...Ch. 2 - A cone has a volume of 314 cm3 and radius of 5.00...Ch. 2 - A right triangle has a side of 41.2 mm and a side...Ch. 2 - Given a cylinder with a radius of 7 .20 cm and a...Ch. 2 - A rectangle has a perimeter of 40.0 cm. One side...Ch. 2 - The formula for the volume of a cylinder is V =...Ch. 2 - The formula for the area of a triangle is A=12bh....Ch. 2 - Find the volume of the lead sleeve with the cored...Ch. 2 - A rectangular plot of land measure 40.0 m by...Ch. 2 - You run a landscaping business and know that you...Ch. 2 - A room that measures 10.0 ft wide, 32.0 ft long,...Ch. 2 - Instead of using a solid iron beam, structural...Ch. 2 - A shipping specialist at a craft store needs to...Ch. 2 - A crane needs to lift a spool of fine steel cable...
Additional Science Textbook Solutions
Find more solutions based on key concepts
1.14 Classify each of the following as a pure substance or a mixture. If a mixture, indicate whether it is homo...
Chemistry: The Central Science (14th Edition)
WHAT IF? Most prairies experience regular fires, typically every few years. If these disturbances were relativ...
Campbell Biology (11th Edition)
What were the major microbiological interests of Martinus Beijerinck and Sergei Winogradsky? It can be said tha...
Brock Biology of Microorganisms (15th Edition)
Which type of cartilage is most plentiful in the adult body?
Anatomy & Physiology (6th Edition)
5.2 In a diploid species of plant, the genes for plant height and fruit shape are syntenic and separated by m....
Genetic Analysis: An Integrated Approach (3rd Edition)
2. Define equilibrium population. Outline the conditions that must be met for a population to stay in genetic e...
Biology: Life on Earth (11th Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.Similar questions
- Show work using graphing paperarrow_forwardCan someone help me answer this physics 2 questions. Thank you.arrow_forwardFour capacitors are connected as shown in the figure below. (Let C = 12.0 μF.) a C 3.00 με Hh. 6.00 με 20.0 με HE (a) Find the equivalent capacitance between points a and b. 5.92 HF (b) Calculate the charge on each capacitor, taking AV ab = 16.0 V. 20.0 uF capacitor 94.7 6.00 uF capacitor 67.6 32.14 3.00 µF capacitor capacitor C ☑ με με The 3 µF and 12.0 uF capacitors are in series and that combination is in parallel with the 6 μF capacitor. What quantity is the same for capacitors in parallel? μC 32.14 ☑ You are correct that the charge on this capacitor will be the same as the charge on the 3 μF capacitor. μCarrow_forward
- In the pivot assignment, we observed waves moving on a string stretched by hanging weights. We noticed that certain frequencies produced standing waves. One such situation is shown below: 0 ст Direct Measurement ©2015 Peter Bohacek I. 20 0 cm 10 20 30 40 50 60 70 80 90 100 Which Harmonic is this? Do NOT include units! What is the wavelength of this wave in cm with only no decimal places? If the speed of this wave is 2500 cm/s, what is the frequency of this harmonic (in Hz, with NO decimal places)?arrow_forwardFour capacitors are connected as shown in the figure below. (Let C = 12.0 µF.) A circuit consists of four capacitors. It begins at point a before the wire splits in two directions. On the upper split, there is a capacitor C followed by a 3.00 µF capacitor. On the lower split, there is a 6.00 µF capacitor. The two splits reconnect and are followed by a 20.0 µF capacitor, which is then followed by point b. (a) Find the equivalent capacitance between points a and b. µF(b) Calculate the charge on each capacitor, taking ΔVab = 16.0 V. 20.0 µF capacitor µC 6.00 µF capacitor µC 3.00 µF capacitor µC capacitor C µCarrow_forwardTwo conductors having net charges of +14.0 µC and -14.0 µC have a potential difference of 14.0 V between them. (a) Determine the capacitance of the system. F (b) What is the potential difference between the two conductors if the charges on each are increased to +196.0 µC and -196.0 µC? Varrow_forward
- Please see the attached image and answer the set of questions with proof.arrow_forwardHow, Please type the whole transcript correctly using comma and periods as needed. I have uploaded the picture of a video on YouTube. Thanks,arrow_forwardA spectra is a graph that has amplitude on the Y-axis and frequency on the X-axis. A harmonic spectra simply draws a vertical line at each frequency that a harmonic would be produced. The height of the line indicates the amplitude at which that harmonic would be produced. If the Fo of a sound is 125 Hz, please sketch a spectra (amplitude on the Y axis, frequency on the X axis) of the harmonic series up to the 4th harmonic. Include actual values on Y and X axis.arrow_forward
- Sketch a sign wave depicting 3 seconds of wave activity for a 5 Hz tone.arrow_forwardSketch a sine wave depicting 3 seconds of wave activity for a 5 Hz tone.arrow_forwardThe drawing shows two long, straight wires that are suspended from the ceiling. The mass per unit length of each wire is 0.050 kg/m. Each of the four strings suspending the wires has a length of 1.2 m. When the wires carry identical currents in opposite directions, the angle between the strings holding the two wires is 20°. (a) Draw the free-body diagram showing the forces that act on the right wire with respect to the x axis. Account for each of the strings separately. (b) What is the current in each wire? 1.2 m 20° I -20° 1.2 marrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
An Introduction to Physical SciencePhysicsISBN:9781305079137Author:James Shipman, Jerry D. Wilson, Charles A. Higgins, Omar TorresPublisher:Cengage Learning
University Physics Volume 1PhysicsISBN:9781938168277Author:William Moebs, Samuel J. Ling, Jeff SannyPublisher:OpenStax - Rice University
Glencoe Physics: Principles and Problems, Student...PhysicsISBN:9780078807213Author:Paul W. ZitzewitzPublisher:Glencoe/McGraw-Hill
College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
College PhysicsPhysicsISBN:9781285737027Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
An Introduction to Physical Science
Physics
ISBN:9781305079137
Author:James Shipman, Jerry D. Wilson, Charles A. Higgins, Omar Torres
Publisher:Cengage Learning
University Physics Volume 1
Physics
ISBN:9781938168277
Author:William Moebs, Samuel J. Ling, Jeff Sanny
Publisher:OpenStax - Rice University
Glencoe Physics: Principles and Problems, Student...
Physics
ISBN:9780078807213
Author:Paul W. Zitzewitz
Publisher:Glencoe/McGraw-Hill
College Physics
Physics
ISBN:9781305952300
Author:Raymond A. Serway, Chris Vuille
Publisher:Cengage Learning
Principles of Physics: A Calculus-Based Text
Physics
ISBN:9781133104261
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
College Physics
Physics
ISBN:9781285737027
Author:Raymond A. Serway, Chris Vuille
Publisher:Cengage Learning
Components of a Vector (Part 1) | Unit Vectors | Don't Memorise; Author: Don't Memorise;https://www.youtube.com/watch?v=fwMUELxZ0Pw;License: Standard YouTube License, CC-BY
02 - Learn Unit Conversions, Metric System & Scientific Notation in Chemistry & Physics; Author: Math and Science;https://www.youtube.com/watch?v=W_SMypXo7tc;License: Standard Youtube License