
Concept explainers
(a)
The total electric potential at a large distance from the quadrupole.
(a)
Answer to Problem 34P
The total electric potential at a large distance from the quadrupole is
Explanation of Solution
Formula used:
The expression for the electric potential due to quadrupole is given as,
Here,
Calculation:
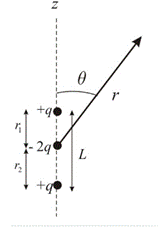
Figure (1)
The electric potential at a large distance from dipole is calculated as,
The electric potential due to quadrupole is calculated as,
Substitute the values,
Conclusion:
Therefore, the total electric potential at a large distance from the quadrupole is
(b)
The electric field for
(b)
Answer to Problem 34P
The electric field for
Explanation of Solution
Formula used:
The expression for the electric potential due to quadrupole on the
Calculation:
On
The electric field on the positive
Conclusion:
Therefore, the electric field for
(c)
The result of part (b) will be obtained by the addition of three point charges.
(c)
Answer to Problem 34P
The result of part (b) will be obtained by the addition of three point charges and the magnitude of the electric field is
Explanation of Solution
Formula used:
The expression for the net electric field on the positive
Calculation:
The net electric field on the positive
By using binomial theorem,
Substitute the binomial equation in equation (1)
Conclusion:
Therefore, the result of part (b) will be obtained by the addition of three point charges and the magnitude of the electric field is
Want to see more full solutions like this?
Chapter 23 Solutions
Physics for Scientists and Engineers
- Pls help asaparrow_forward3. If the force of gravity stopped acting on the planets in our solar system, what would happen? a) They would spiral slowly towards the sun. b) They would continue in straight lines tangent to their orbits. c) They would continue to orbit the sun. d) They would fly straight away from the sun. e) They would spiral slowly away from the sun. 4. 1 The free-body diagram of a wagon being pulled along a horizontal surface is best represented by A F N B C 0 Ꭰ FN E a) A b) B c) C app app The app 10 app d) e) ס ח D E 10 apparrow_forwardPls help ASAParrow_forward
- Pls help asaparrow_forwardPls help asaparrow_forwardThe acceleration of an object sliding along a frictionless ramp is inclined at an angle 0 is 9. a) g tano b) g cose c) g sino 10. d) g e) zero A 1.5 kg cart is pulled with a force of 7.3 N at an angle of 40° above the horizontal. If a kinetic friction force of 3.2 N acts against the motion, the cart's acceleration along the horizontal surface will be a) 5.0 m/s² b) 1.6 m/s² c) 2.4 m/s² 11. d) 1.0 m/s² e) 2.7 m/s² What is the net force acting on an object with a mass of 10 kg moving at a constant velocity of 10 m/s [North]? a) 100 N [North] b) 100 N [South] 10 N [North} d) 10 N [South] e) None of these.arrow_forward
- Modified True/False - indicate whether the sentence or statement is true or false. If the statement is false, correct the statement to make it true. 12. An object in uniform circular motion has a constant velocity while experiencing centripetal acceleration. 13. An object travelling in uniform circular motion experiences an outward centrifugal force that tends to pull the object out of the circular path. 14. An object with less inertia can resist changes in motion more than an object with more inertia. 15. For an object sliding on a horizontal surface with a horizontal applied force, the frictional force will always increase as the applied force increases.arrow_forwardPls help asaparrow_forwardAnswer the given question showing step by step by and all necessary working out.arrow_forward
 Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning College PhysicsPhysicsISBN:9781285737027Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
College PhysicsPhysicsISBN:9781285737027Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning





