Mathematics with Applications, Books a la Carte, and MyLab Math with Pearson eText - Title-Specific Access Card Package (12th Edition)
12th Edition
ISBN: 9780134862651
Author: Lial
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 2.2, Problem 77E
Olympic 5000-Meters The accompanying graph shows the winning time (in minutes) at the Olympic Games from 1952 to 2016 for the men's 5000-meterrun, together with a linear approximation of these data. (Data from: The WorldAlmanac and Book of Facts: 2017.)
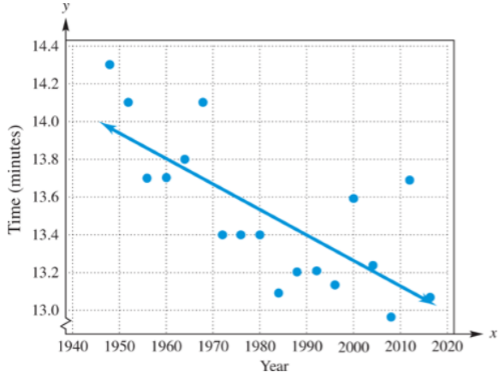
The equation for the linear approximation is
Luxury Handbags MichaelKors now dominates the handbag market in North America. In 2011, the company had 5% of the market;in 2015, it had 23%. (Data from: The Wall Street Journal.)
a. Write a linear equation expressing the percentage of the luxury handbag market y that Michael Kors had x years after 2000.
b. What was the percentage of the market that Michael Kors had in 2013?
c. If the trend continues, what will be the first full year in which Michael Kors had 30% of the market?
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
No chatgpt pls will upvote Already got wrong chatgpt answer
data management Q7
data management Q10
Chapter 2 Solutions
Mathematics with Applications, Books a la Carte, and MyLab Math with Pearson eText - Title-Specific Access Card Package (12th Edition)
Ch. 2.1 - Checkpoint 1
Locate and (−5,0) on a coordinate...Ch. 2.1 - Checkpoint 2
Which of the following are solutions...Ch. 2.1 - Checkpoint 3
Graph
Ch. 2.1 - Checkpoint 4
Find the x- and y-intercepts of the...Ch. 2.1 - Checkpoint 5
From Figure 2.7 determine when the...Ch. 2.1 - Checkpoint 6
In Example 6, find the profit from...Ch. 2.1 - Checkpoint 7
Use a graphing calculator to graph ...Ch. 2.1 - Checkpoint 8
Use a graphical root finder to...Ch. 2.1 - State the quadrant in which each point lies.
1.
Ch. 2.1 - State the quadrant in which each point lies.
2.
Ch. 2.1 - Determine whether the given ordered pair is a...Ch. 2.1 - Determine whether the given ordered pair is a...Ch. 2.1 - Determine whether the given ordered pair is a...Ch. 2.1 - Determine whether the given ordered pair is a...Ch. 2.1 - Prob. 7ECh. 2.1 - Prob. 8ECh. 2.1 - Prob. 9ECh. 2.1 - Prob. 10ECh. 2.1 - Prob. 11ECh. 2.1 - Prob. 12ECh. 2.1 - List the x-intercepts and y-intercepts of each...Ch. 2.1 - List the x-intercepts and y-intercepts of each...Ch. 2.1 - List the x-intercepts and y-intercepts of each...Ch. 2.1 - List the x-intercepts and y-intercepts of each...Ch. 2.1 - Find the x-intercepts and y-intercepts of the...Ch. 2.1 - Find the x-intercepts and y-intercepts of the...Ch. 2.1 - Find the x-intercepts and y-intercepts of the...Ch. 2.1 - Find the x-intercepts and y-intercepts of the...Ch. 2.1 - Find the x-intercepts and y-intercepts of the...Ch. 2.1 - Find the x-intercepts and y-intercepts of the...Ch. 2.1 - Find the x-intercepts and y-intercepts of the...Ch. 2.1 - Find the x-intercepts and y-intercepts of the...Ch. 2.1 - Find the x-intercepts and y-intercepts of the...Ch. 2.1 - Find the x-intercepts and y-intercepts of the...Ch. 2.1 - Sketch the graph of the equation. (See Examples...Ch. 2.1 - Prob. 28ECh. 2.1 - Sketch the graph of the equation. (See Examples...Ch. 2.1 - Prob. 30ECh. 2.1 - Sketch the graph of the equation. (See Examples...Ch. 2.1 - Sketch the graph of the equation. (See Examples...Ch. 2.1 - Sketch the graph of the equation. (See Examples...Ch. 2.1 - Sketch the graph of the equation. (See Examples...Ch. 2.1 - Prob. 35ECh. 2.1 - Prob. 36ECh. 2.1 - Sketch the graph of the information in the...Ch. 2.1 - Sketch the graph of the information in the...Ch. 2.1 - Sketch the graph of the information in the...Ch. 2.1 - Sketch the graph of the information in the...Ch. 2.1 - Prob. 41ECh. 2.1 - Prob. 42ECh. 2.1 - Prob. 43ECh. 2.1 - Prob. 44ECh. 2.1 - Business Use the revenue and cost graphs for the...Ch. 2.1 - Prob. 46ECh. 2.1 - Business Use the revenue and cost graphs for the...Ch. 2.1 - Prob. 48ECh. 2.1 - Business The graph below gives the annual...Ch. 2.1 - Business The graph below gives the annual...Ch. 2.1 - Prob. 51ECh. 2.1 - Prob. 52ECh. 2.1 - Netflix and Apple Stock Prices The graph below...Ch. 2.1 - Prob. 54ECh. 2.1 - Prob. 55ECh. 2.1 - Prob. 56ECh. 2.1 - Prob. 57ECh. 2.1 - Prob. 58ECh. 2.1 - Prob. 59ECh. 2.1 - Netflix and Apple stock prices The graph below...Ch. 2.1 - Netflix and Apple stock prices The graph below...Ch. 2.1 - Prob. 62ECh. 2.1 - Use a graphing calculator to find the graph of the...Ch. 2.1 - Use a graphing calculator to find the graph of the...Ch. 2.1 - Use a graphing calculator to find the graph of the...Ch. 2.1 - Use a graphing calculator to find the graph of the...Ch. 2.1 - Prob. 67ECh. 2.1 - Prob. 68ECh. 2.1 - Prob. 69ECh. 2.1 - Prob. 70ECh. 2.1 - Prob. 71ECh. 2.1 - Prob. 72ECh. 2.1 - Use a graphing calculator to approximate all real...Ch. 2.1 - Prob. 74ECh. 2.1 - Prob. 75ECh. 2.1 - Use a graphing calculator to approximate all real...Ch. 2.2 - Checkpoint 1
Find the slope of the line through...Ch. 2.2 - Checkpoint 2
Find an equation for the line...Ch. 2.2 - Prob. 3CPCh. 2.2 - Checkpoint 4
List the slopes of the following...Ch. 2.2 - Checkpoint 5
Graph the given lines and label the...Ch. 2.2 - Prob. 6CPCh. 2.2 - Checkpoint 7
Find both the point–slope and the...Ch. 2.2 - Prob. 8CPCh. 2.2 -
Checkpoint 9
The online viewership in India was...Ch. 2.2 - Find the slope of the given line, if it is...Ch. 2.2 - Find the slope of the given line, if it is...Ch. 2.2 - Prob. 3ECh. 2.2 - Find the slope of the given line, if it is...Ch. 2.2 - Prob. 5ECh. 2.2 - Find the slope of the given line, if it is...Ch. 2.2 - Find the slope of the given line, if it is...Ch. 2.2 - Prob. 8ECh. 2.2 - Find an equation of the line with the given...Ch. 2.2 - Prob. 10ECh. 2.2 - Prob. 11ECh. 2.2 - Prob. 12ECh. 2.2 - Find an equation of the line with the given...Ch. 2.2 - Prob. 14ECh. 2.2 - Prob. 15ECh. 2.2 - Find the slope m and the y-intercept b of the line...Ch. 2.2 - Prob. 17ECh. 2.2 - Prob. 18ECh. 2.2 - Find the slope m and the y-intercept b of the line...Ch. 2.2 - Find the slope m and the y-intercept b of the line...Ch. 2.2 - Prob. 21ECh. 2.2 - Prob. 22ECh. 2.2 - Prob. 23ECh. 2.2 - Prob. 24ECh. 2.2 - 25. For which of the line segments in the figure...Ch. 2.2 - 26. Match each equation with the line that most...Ch. 2.2 - Prob. 27ECh. 2.2 - Prob. 28ECh. 2.2 - Prob. 29ECh. 2.2 - Prob. 30ECh. 2.2 - Sketch the graph of the given equation and label...Ch. 2.2 - Prob. 32ECh. 2.2 - Prob. 33ECh. 2.2 - Determine whether each pair of lines is parallel,...Ch. 2.2 - Prob. 35ECh. 2.2 - Prob. 36ECh. 2.2 - Prob. 37ECh. 2.2 - Determine whether each pair of lines is parallel,...Ch. 2.2 - Prob. 39ECh. 2.2 - Prob. 40ECh. 2.2 - Find an equation of the line with slope m that...Ch. 2.2 - Prob. 42ECh. 2.2 - Prob. 43ECh. 2.2 - Prob. 44ECh. 2.2 - Prob. 45ECh. 2.2 - Prob. 46ECh. 2.2 - Prob. 47ECh. 2.2 - Prob. 48ECh. 2.2 - Find an equation of the line that passes through...Ch. 2.2 - Prob. 50ECh. 2.2 - Find an equation of the line that passes through...Ch. 2.2 - Find an equation of the line that passes through...Ch. 2.2 - Find an equation of the line satisfying the given...Ch. 2.2 - Prob. 54ECh. 2.2 - Find an equation of the line satisfying the given...Ch. 2.2 - Prob. 56ECh. 2.2 - Find an equation of the line satisfying the given...Ch. 2.2 - Prob. 58ECh. 2.2 - Prob. 59ECh. 2.2 - Prob. 60ECh. 2.2 - Find an equation of the line satisfying the given...Ch. 2.2 - Prob. 62ECh. 2.2 - Prob. 63ECh. 2.2 - Prob. 64ECh. 2.2 - Prob. 65ECh. 2.2 -
66. Ral Corp. has an incentive compensation plan...Ch. 2.2 - Prob. 67ECh. 2.2 - Prob. 68ECh. 2.2 -
Light Beer Sales Data from the Wall Street...Ch. 2.2 - Light Beer Sales Data from the Wall Street Journal...Ch. 2.2 - Global Malaria Cases Data from the Wall Street...Ch. 2.2 - Global Malaria Cases Data from the Wall Street...Ch. 2.2 - Prob. 73ECh. 2.2 - Prob. 74ECh. 2.2 - Olympic 5000-Meters The accompanying graph shows...Ch. 2.2 - Olympic 5000-Meters The accompanying graph shows...Ch. 2.2 - Olympic 5000-Meters The accompanying graph shows...Ch. 2.2 - Olympic 5000-Meters The accompanying graph shows...Ch. 2.2 - Olympic 5000-Meters The accompanying graph shows...Ch. 2.2 -
Olympic 5000-Meters The accompanying graph shows...Ch. 2.3 - Checkpoint 1
Use the points (5, 917) and (9, 1038)...Ch. 2.3 - Checkpoint 2
Another model for the data in...Ch. 2.3 - Checkpoint 3 Use the least-squares regression line...Ch. 2.3 - Checkpoint 4
Using only the data from 2005, 2007,...Ch. 2.3 - Checkpoint 5
Use only the data of 2010 and later...Ch. 2.3 - 1. Physical Science The following table shows...Ch. 2.3 - Physical Science Use the linear equation derived...Ch. 2.3 - Physical Science Use the liner equation derived in...Ch. 2.3 - Physical Science Use the linear equation derived...Ch. 2.3 - In each of the next set of problems, assume that...Ch. 2.3 - In each of the next set of problems, assume that...Ch. 2.3 - In each of the next set of problems, assume that...Ch. 2.3 - In each of the next set of problems, assume that...Ch. 2.3 -
In each of the next set of problems, assume that...Ch. 2.3 - Prob. 10ECh. 2.3 - In each of the next two problems, two linear...Ch. 2.3 -
In each of the next two problems, two linear...Ch. 2.3 - In each of the following problems, determine...Ch. 2.3 -
In each of the following problems, determine...Ch. 2.3 -
In Exercises 15-18 find the required linear...Ch. 2.3 - In Exercises 15-18 find the required linear model...Ch. 2.3 - In Exercises 15-18 find the required linear model...Ch. 2.3 - Prob. 18ECh. 2.3 - Street and Highway Construction The total amount...Ch. 2.3 - Cable Subscribers The number of basic cable...Ch. 2.3 - Prob. 21ECh. 2.3 - 22. Life Expectancy The following table shown...Ch. 2.4 - Checkpoint 1
(a) First multiply both sides of −6 <...Ch. 2.4 - Checkpoint 2
Solve these inequalities. Graph each...Ch. 2.4 - Prob. 3CPCh. 2.4 - Prob. 4CPCh. 2.4 - Prob. 5CPCh. 2.4 - Prob. 6CPCh. 2.4 - Prob. 7CPCh. 2.4 - Prob. 8CPCh. 2.4 - Prob. 9CPCh. 2.4 - Prob. 1ECh. 2.4 - 2. The three-part inequality means “p is less...Ch. 2.4 - Solve each inequality and graph each solution....Ch. 2.4 - Solve each inequality and graph each solution....Ch. 2.4 - Prob. 5ECh. 2.4 - Prob. 6ECh. 2.4 - Prob. 7ECh. 2.4 - Prob. 8ECh. 2.4 - Prob. 9ECh. 2.4 - Prob. 10ECh. 2.4 - Prob. 11ECh. 2.4 - Solve each inequality and graph each solution....Ch. 2.4 - Solve each inequality and graph each solution....Ch. 2.4 - Solve each inequality and graph each solution....Ch. 2.4 - Solve each inequality and graph each solution....Ch. 2.4 - Solve each inequality and graph each solution....Ch. 2.4 - Solve each inequality and graph each solution....Ch. 2.4 - Prob. 18ECh. 2.4 - Prob. 19ECh. 2.4 - Prob. 20ECh. 2.4 - Prob. 21ECh. 2.4 - Solve each inequality and graph each solution....Ch. 2.4 - Solve each inequality and graph each solution....Ch. 2.4 - Solve each inequality and graph each solution....Ch. 2.4 - Solve each inequality and graph each solution....Ch. 2.4 - Prob. 26ECh. 2.4 - In the following exercises, write a linear...Ch. 2.4 - In the following exercises, write a linear...Ch. 2.4 - In the following exercises, write a linear...Ch. 2.4 - In the following exercises, write a linear...Ch. 2.4 - Break-Even Point In Exercises 31-36, find all...Ch. 2.4 - Break-Even Point In Exercises 31-36, find all...Ch. 2.4 - Business In Exercises 31–36, find all values of x...Ch. 2.4 - Prob. 34ECh. 2.4 - Business In Exercises 31–36, find all values of x...Ch. 2.4 - Prob. 36ECh. 2.4 - Solve each inequality. Graph each solution. (See...Ch. 2.4 - Prob. 38ECh. 2.4 - Prob. 39ECh. 2.4 - Prob. 40ECh. 2.4 - Prob. 41ECh. 2.4 - Prob. 42ECh. 2.4 - Prob. 43ECh. 2.4 - Prob. 44ECh. 2.4 - Prob. 45ECh. 2.4 - Prob. 46ECh. 2.4 - Prob. 47ECh. 2.4 - Prob. 48ECh. 2.4 - Prob. 49ECh. 2.4 - Prob. 50ECh. 2.4 - Prob. 51ECh. 2.4 - Prob. 52ECh. 2.4 - Prob. 53ECh. 2.4 - Social Science A Gallup poll in January 2017 found...Ch. 2.4 - Prob. 55ECh. 2.4 - Prob. 56ECh. 2.5 - Checkpoint 1
Solve each inequality. Graph the...Ch. 2.5 - Prob. 2CPCh. 2.5 - Prob. 3CPCh. 2.5 - Prob. 4CPCh. 2.5 - Prob. 5CPCh. 2.5 - Prob. 6CPCh. 2.5 - Prob. 7CPCh. 2.5 - Prob. 1ECh. 2.5 - Prob. 2ECh. 2.5 - Solve each of these quadratic inequalities. Graph...Ch. 2.5 - Solve each of these quadratic inequalities. Graph...Ch. 2.5 - Prob. 5ECh. 2.5 - Prob. 6ECh. 2.5 - Prob. 7ECh. 2.5 - Prob. 8ECh. 2.5 - Solve each of these quadratic inequalities. Graph...Ch. 2.5 - Prob. 10ECh. 2.5 - Solve each of these quadratic inequalities. Graph...Ch. 2.5 - Prob. 12ECh. 2.5 - Solve these inequalities. (See Example 4.) 13.
Ch. 2.5 - Solve these inequalities. (See Example 4.) 14.
Ch. 2.5 - Prob. 15ECh. 2.5 - Prob. 16ECh. 2.5 - Solve these inequalities. (See Example 4.)
17.
Ch. 2.5 - Prob. 18ECh. 2.5 - Prob. 19ECh. 2.5 - Prob. 20ECh. 2.5 - 21. A student solved the inequality by taking...Ch. 2.5 - Prob. 22ECh. 2.5 - Use a graphing calculator to solve these...Ch. 2.5 - Use a graphing calculator to solve these...Ch. 2.5 - Prob. 25ECh. 2.5 - Prob. 26ECh. 2.5 - Prob. 27ECh. 2.5 - Prob. 28ECh. 2.5 - Solve these rational inequalities. (See Examples 7...Ch. 2.5 - Prob. 30ECh. 2.5 - Prob. 31ECh. 2.5 - Prob. 32ECh. 2.5 - Prob. 33ECh. 2.5 - Prob. 34ECh. 2.5 - Prob. 35ECh. 2.5 - Solve these rational inequalities. (See Examples 7...Ch. 2.5 - Solve these rational inequalities. (See Examples 7...Ch. 2.5 - Prob. 38ECh. 2.5 - Prob. 39ECh. 2.5 - Prob. 40ECh. 2.5 - 41. Business An analyst has found that her...Ch. 2.5 - Prob. 42ECh. 2.5 - Prob. 43ECh. 2.5 - Prob. 44ECh. 2 - Which of the ordered pairs (−2, 3), (0, −5), (2,...Ch. 2 - Prob. 2RECh. 2 - Sketch the graph of each equation. 3.
Ch. 2 - Prob. 4RECh. 2 - Sketch the graph of each equation. 5.
Ch. 2 - Prob. 6RECh. 2 - Prob. 7RECh. 2 - Prob. 8RECh. 2 - Prob. 9RECh. 2 - Prob. 10RECh. 2 - Prob. 11RECh. 2 - Prob. 12RECh. 2 - In Exercises 12–21, find the slope of the line...Ch. 2 - Prob. 14RECh. 2 - Prob. 15RECh. 2 - Prob. 16RECh. 2 - In Exercises 12–21, find the slope of the line...Ch. 2 - Prob. 18RECh. 2 - In Exercises 12–21, find the slope of the line...Ch. 2 - In Exercises 12–21, find the slope of the line...Ch. 2 - In Exercises 12–21, find the slope of the line...Ch. 2 - Prob. 22RECh. 2 - 23. Graph the line through (−4, 1) with m = 3.
Ch. 2 - 24. What information is needed to determine the...Ch. 2 - Find an equation for each of the following...Ch. 2 - Prob. 26RECh. 2 - Find an equation for each of the following...Ch. 2 - Find an equation for each of the following...Ch. 2 - Prob. 29RECh. 2 - Prob. 30RECh. 2 - Find an equation for each of the following...Ch. 2 - 32. Here is a sample SAT question: Which of the...Ch. 2 - Student Loans In 2010, the total owed on federal...Ch. 2 - Prob. 34RECh. 2 - Median Income The following table gives the median...Ch. 2 - Prob. 36RECh. 2 - Two-Year School Tuition The following table shows...Ch. 2 - 38. Four-Year School Tuition The following table...Ch. 2 - Solve each inequality. 39.
Ch. 2 - Solve each inequality. 40.
Ch. 2 - Solve each inequality. 41.
Ch. 2 - Solve each inequality. 42.
Ch. 2 - Solve each inequality. 43.
Ch. 2 - Solve each inequality. 44.
Ch. 2 - Solve each inequality. 45.
Ch. 2 - Solve each inequality. 46.
Ch. 2 - Solve each inequality. 47.
Ch. 2 - Solve each inequality. 48.
Ch. 2 - Solve each inequality. 49.
Ch. 2 - Solve each inequality. 50.
Ch. 2 - Prob. 51RECh. 2 - Prob. 52RECh. 2 - Prob. 53RECh. 2 - 54. Business One car rental firm charges $125 for...Ch. 2 - Solve each inequality. 55.
Ch. 2 - Solve each inequality. 56.
Ch. 2 - Solve each inequality. 57
Ch. 2 - Solve each inequality. 58.
Ch. 2 - Solve each inequality. 59.
Ch. 2 - Solve each inequality. 60.
Ch. 2 - Solve each inequality.
61.
Ch. 2 - Solve each inequality.
62.
Ch. 2 - Solve each inequality.
63.
Ch. 2 - Solve each inequality.
64.
Ch. 2 - Solve each inequality.
65.
Ch. 2 - Solve each inequality.
66.
Ch. 2 - Business The following table gives the number of...Ch. 2 - Business The following table gives the number of...Ch. 2 - Prob. 5CECh. 2 - Prob. 6CECh. 2 - Prob. 7CECh. 2 - Prob. 8CECh. 2 - Prob. 9CECh. 2 - Prob. 1EPCh. 2 - Prob. 2EPCh. 2 - Prob. 3EPCh. 2 - Prob. 4EPCh. 2 - Prob. 5EP
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, subject and related others by exploring similar questions and additional content below.Similar questions
- The final answer is 8/π(sinx) + 8/3π(sin 3x)+ 8/5π(sin5x)....arrow_forwardKeity x२ 1. (i) Identify which of the following subsets of R2 are open and which are not. (a) A = (2,4) x (1, 2), (b) B = (2,4) x {1,2}, (c) C = (2,4) x R. Provide a sketch and a brief explanation to each of your answers. [6 Marks] (ii) Give an example of a bounded set in R2 which is not open. [2 Marks] (iii) Give an example of an open set in R2 which is not bounded. [2 Marksarrow_forward2. (i) Which of the following statements are true? Construct coun- terexamples for those that are false. (a) sequence. Every bounded sequence (x(n)) nEN C RN has a convergent sub- (b) (c) (d) Every sequence (x(n)) nEN C RN has a convergent subsequence. Every convergent sequence (x(n)) nEN C RN is bounded. Every bounded sequence (x(n)) EN CRN converges. nЄN (e) If a sequence (xn)nEN C RN has a convergent subsequence, then (xn)nEN is convergent. [10 Marks] (ii) Give an example of a sequence (x(n))nEN CR2 which is located on the parabola x2 = x², contains infinitely many different points and converges to the limit x = (2,4). [5 Marks]arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Big Ideas Math A Bridge To Success Algebra 1: Stu...AlgebraISBN:9781680331141Author:HOUGHTON MIFFLIN HARCOURTPublisher:Houghton Mifflin Harcourt
College AlgebraAlgebraISBN:9781305115545Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Glencoe Algebra 1, Student Edition, 9780079039897...
Algebra
ISBN:9780079039897
Author:Carter
Publisher:McGraw Hill
Big Ideas Math A Bridge To Success Algebra 1: Stu...
Algebra
ISBN:9781680331141
Author:HOUGHTON MIFFLIN HARCOURT
Publisher:Houghton Mifflin Harcourt
College Algebra
Algebra
ISBN:9781305115545
Author:James Stewart, Lothar Redlin, Saleem Watson
Publisher:Cengage Learning
Holt Mcdougal Larson Pre-algebra: Student Edition...
Algebra
ISBN:9780547587776
Author:HOLT MCDOUGAL
Publisher:HOLT MCDOUGAL
What is a Linear Equation in One Variable?; Author: Don't Memorise;https://www.youtube.com/watch?v=lDOYdBgtnjY;License: Standard YouTube License, CC-BY
Linear Equation | Solving Linear Equations | What is Linear Equation in one variable ?; Author: Najam Academy;https://www.youtube.com/watch?v=tHm3X_Ta_iE;License: Standard YouTube License, CC-BY