Bundle: Physics For Scientists And Engineers With Modern Physics, 10th + Webassign Printed Access Card For Serway/jewett's Physics For Scientists And Engineers, 10th, Multi-term
10th Edition
ISBN: 9781337888516
Author: Raymond A. Serway, John W. Jewett
Publisher: Cengage Learning
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 21.7, Problem 21.5QQ
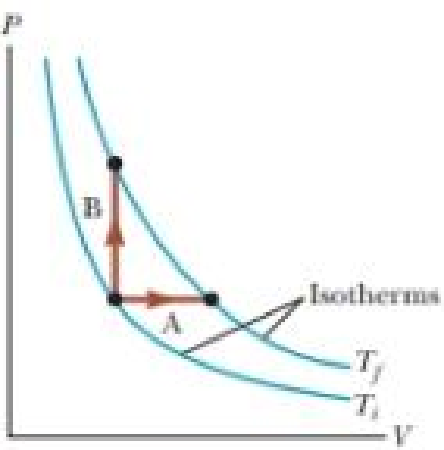
An ideal gas is taken from an initial temperature Ti to a higher final temperature Tf along two different reversible paths as shown in Figure 21.15. Path A is at constant pressure, and path B is at constant volume. What is the relation between the entropy changes of the gas for these paths? (a) ΔSA > ΔSB (b) ΔSA = ΔSB (c) ΔSA < ΔSB
Figure 21.15 (Quick Quiz 21.5) An ideal gas is taken from temperature Ti to Tf via two different paths.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
The shear leg derrick is used to haul the 200-kg net of fish onto the dock as shown in. Assume the force in each leg acts along
its axis.
5.6 m.
4 m-
B
Part A
Determine the compressive force along leg AB.
Express your answer to three significant figures and include the appropriate units.
FAB =
Value
Submit
Request Answer
Part B
Units
?
Determine the compressive force along leg CB.
Express your answer to three significant figures and include the appropriate units.
FCB=
Value
Submit
Request Answer
Part C
?
Units
Determine the tension in the winch cable DB.
Express your answer with the appropriate units.
2m
Part A
(Figure 1) shows a bucket suspended from a cable by means of a small
pulley at C.
If the bucket and its contents have a mass of 10 kg, determine the location of the pulley for equilibrium. The cable is 6 m long.
Express your answer to three significant figures and include the appropriate units.
Figure
4 m
B
НА
x =
Value
Submit
Request Answer
Provide Feedback
<
1 of 1
T
1 m
Units
?
The particle in is in equilibrium and F4 = 165 lb.
Part A
Determine the magnitude of F1.
Express your answer in pounds to three significant figures.
ΑΣΦ
tvec
F₁ =
Submit
Request Answer
Part B
Determine the magnitude of F2.
Express your answer in pounds to three significant figures.
ΑΣΦ
It vec
F2 =
Submit
Request Answer
Part C
Determine the magnitude of F3.
Express your answer in pounds to three significant figures.
?
?
lb
lb
F₂
225 lb
135°
45°
30°
-60°-
Chapter 21 Solutions
Bundle: Physics For Scientists And Engineers With Modern Physics, 10th + Webassign Printed Access Card For Serway/jewett's Physics For Scientists And Engineers, 10th, Multi-term
Ch. 21.1 - The energy input to an engine is 4.00 times...Ch. 21.2 - The energy entering an electric heater by...Ch. 21.4 - Three engines operate between reservoirs separated...Ch. 21.6 - (a) Suppose you select four cards at random from a...Ch. 21.7 - An ideal gas is taken from an initial temperature...Ch. 21.7 - True or False: The entropy change in an adiabatic...Ch. 21 - A particular heat engine has a mechanical power...Ch. 21 - The work done by an engine equals one-fourth the...Ch. 21 - Suppose a heat engine is connected to two energy...Ch. 21 - During each cycle, a refrigerator ejects 625 kJ of...
Ch. 21 - A freezer has a coefficient of performance of...Ch. 21 - Prob. 6PCh. 21 - One of the most efficient heat engines ever built...Ch. 21 - Prob. 8PCh. 21 - If a 35.0% -efficient Carnot heat engine (Fig....Ch. 21 - Prob. 10PCh. 21 - Prob. 11PCh. 21 - A power plant operates at a 32.0% efficiency...Ch. 21 - You are working on a summer job at a company that...Ch. 21 - Prob. 14PCh. 21 - Prob. 15PCh. 21 - Suppose you build a two-engine device with the...Ch. 21 - A heat pump used for heating shown in Figure...Ch. 21 - Prob. 18PCh. 21 - An idealized diesel engine operates in a cycle...Ch. 21 - Prob. 20PCh. 21 - Prob. 21PCh. 21 - A Styrofoam cup holding 125 g of hot water at 100C...Ch. 21 - A 1 500-kg car is moving at 20.0 m/s. The driver...Ch. 21 - A 2.00-L container has a center partition that...Ch. 21 - Calculate the change in entropy of 250 g of water...Ch. 21 - What change in entropy occurs when a 27.9-g ice...Ch. 21 - Prob. 27PCh. 21 - Prob. 28PCh. 21 - Prob. 29PCh. 21 - Prob. 30APCh. 21 - Prob. 31APCh. 21 - In 1993, the U.S. government instituted a...Ch. 21 - In 1816, Robert Stirling, a Scottish clergyman,...Ch. 21 - Prob. 34APCh. 21 - Prob. 35APCh. 21 - Prob. 36APCh. 21 - A 1.00-mol sample of an ideal monatomic gas is...Ch. 21 - Prob. 38APCh. 21 - A heat engine operates between two reservoirs at...Ch. 21 - You are working as an assistant to a physics...Ch. 21 - Prob. 41APCh. 21 - You are working as an expert witness for an...Ch. 21 - Prob. 43APCh. 21 - Prob. 44APCh. 21 - A sample of an ideal gas expands isothermally,...Ch. 21 - Prob. 46APCh. 21 - The compression ratio of an Otto cycle as shown in...
Additional Science Textbook Solutions
Find more solutions based on key concepts
1. Rub your hands together vigorously. What happens? Discuss the energy transfers and transformations that take...
College Physics: A Strategic Approach (3rd Edition)
Gregor Mendel never saw a gene, yet he concluded that some inherited factors were responsible for the patterns ...
Campbell Essential Biology (7th Edition)
To test your knowledge, discuss the following topics with a study partner or in writing ideally from memory. Th...
HUMAN ANATOMY
Choose the best answer to each of the following. Explain your reasoning. If Earth were twice as far as it actua...
Cosmic Perspective Fundamentals
Label each statement about the polynucleotide ATGGCG as true or false. The polynucleotide has six nucleotides. ...
General, Organic, and Biological Chemistry - 4th edition
Why do scientists think that all forms of life on earth have a common origin?
Genetics: From Genes to Genomes
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.Similar questions
- The 10-lb weight is supported by the cord AC and roller and by the spring that has a stiffness of k = 10 lb/in. and an unstretched length of 12 in. as shown in. Part A Determine the distance d to maintain equilibrium. Express your answer in inches to three significant figures. 節 ΕΠΙ ΑΣΦ d = *k J vec 5 t 0 ? d C A in. 12 in. Barrow_forwardThe members of a truss are connected to the gusset plate as shown in . The forces are concurrent at point O. Take = 90° and T₁ = 7.5 kN. Part A Determine the magnitude of F for equilibrium. Express your answer to three significant figures and include the appropriate units. F = Value Submit Request Answer Part B 0 ? Units Determine the magnitude of T2 for equilibrium. Express your answer to three significant figures and include the appropriate units. ? T₂ = Value Units T₁ Carrow_forwardpls help on botharrow_forward
- pls helparrow_forwardpls helparrow_forward6. 6. There are 1000 turns on the primary side of a transformer and 200 turns on thesecondary side. If 440 V are supplied to the primary winding, what is the voltageinduced in the secondary winding? Is this a step-up or step-down transformer? 7. 80 V are supplied to the primary winding of a transformer that has 50 turns. If thesecondary side has 50,000 turns, what is the voltage induced on the secondary side?Is this a step-up or step-down transformer? 8. There are 50 turns on the primary side of a transformer and 500 turns on thesecondary side. The current through the primary winding is 6 A. What is the turnsratio of this transformer? What is the current, in milliamps, through the secondarywinding?9. The current through the primary winding on a transformer is 5 A. There are 1000turns on the primary winding and 20 turns on the secondary winding. What is theturns ratio of this transformer? What is the current, in amps, through the secondarywinding?arrow_forward
- No chatgpt plsarrow_forwardWhat is the current, in amps, across a conductor that has a resistance of10 Ω and a voltage of 20 V? 2. A conductor draws a current of 100 A and a resistance of 5 Ω. What is thevoltageacross the conductor? 3. What is the resistance, in ohm’s, of a conductor that has a voltage of 80 kVand acurrent of 200 mA? 4. An x-ray imaging system that draws a current of 90 A is supplied with 220V. What is the power consumed? 5. An x-ray is produced using 800 mA and 100 kV. What is the powerconsumed in kilowatts?arrow_forwardՍՈՈՒ XVirginia Western Community Coll x P Course Home X + astering.pearson.com/?courseld=13289599#/ Figure y (mm) x=0x = 0.0900 m All ✓ Correct For either the time for one full cycle is 0.040 s; this is the period. Part C - ON You are told that the two points x = 0 and x = 0.0900 m are within one wavelength of each other. If the wave is moving in the +x-direction, determine the wavelength. Express your answer to two significant figures and include the appropriate units. 0 t(s) λ = Value m 0.01 0.03 0.05 0.07 Copyright © 2025 Pearson Education Inc. All rights reserved. 日 F3 F4 F5 1775 % F6 F7 B F8 Submit Previous Answers Request Answer ? × Incorrect; Try Again; 3 attempts remaining | Terms of Use | Privacy Policy | Permissions | Contact Us | Cookie Settings 28°F Clear 4 9:23 PM 1/20/2025 F9 prt sc F10 home F11 end F12 insert delete 6 7 29 & * ( 8 9 0 t = back Οarrow_forward
- Part C Find the height yi from which the rock was launched. Express your answer in meters to three significant figures. Learning Goal: To practice Problem-Solving Strategy 4.1 for projectile motion problems. A rock thrown with speed 12.0 m/s and launch angle 30.0 ∘ (above the horizontal) travels a horizontal distance of d = 19.0 m before hitting the ground. From what height was the rock thrown? Use the value g = 9.800 m/s2 for the free-fall acceleration. PROBLEM-SOLVING STRATEGY 4.1 Projectile motion problems MODEL: Is it reasonable to ignore air resistance? If so, use the projectile motion model. VISUALIZE: Establish a coordinate system with the x-axis horizontal and the y-axis vertical. Define symbols and identify what the problem is trying to find. For a launch at angle θ, the initial velocity components are vix=v0cosθ and viy=v0sinθ. SOLVE: The acceleration is known: ax=0 and ay=−g. Thus, the problem becomes one of…arrow_forwardPhys 25arrow_forwardPhys 22arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
College PhysicsPhysicsISBN:9781938168000Author:Paul Peter Urone, Roger HinrichsPublisher:OpenStax College
Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...
Physics
ISBN:9781337553292
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
Principles of Physics: A Calculus-Based Text
Physics
ISBN:9781133104261
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
College Physics
Physics
ISBN:9781938168000
Author:Paul Peter Urone, Roger Hinrichs
Publisher:OpenStax College
Physics for Scientists and Engineers, Technology ...
Physics
ISBN:9781305116399
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
Physics for Scientists and Engineers
Physics
ISBN:9781337553278
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
The Second Law of Thermodynamics: Heat Flow, Entropy, and Microstates; Author: Professor Dave Explains;https://www.youtube.com/watch?v=MrwW4w2nAMc;License: Standard YouTube License, CC-BY