
Concept explainers
(a)
To explain:The reason to restrict the interval
(a)
Explanation of Solution
Given information:The function is
For the right hand limit of given function check the points close to
The restrict interval to
(b)
To show:The area of
(b)
Explanation of Solution
Given information:The figure is given below:
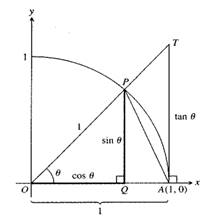
Proof:
The formula for the area of triangle is given by,
In
In
The formula for the area of a sector with radius r is given by,
The radius of the sector is 1 with central angle
Hence, it is proved that area of
(c)
To show:The inequality
(c)
Explanation of Solution
Given information:The figure is given below:
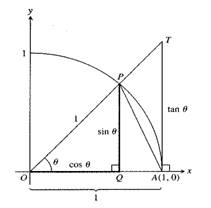
Proof:
From part (b), it is proved that that area of
To prove the given inequality, compare the areas of
Also, the area of sector OAP contains under the region of
Now, compare inequality (1) and (2).
Hence, theinequality
(d)
To show:The inequality
(d)
Explanation of Solution
Proof:
From part (c), for the interval
Multiply the inequality by 2.
Divide the inequality by
Hence, theinequality proved in part (c) can be written as
(e)
To show:The inequality
(e)
Explanation of Solution
Proof:
From part (d), for the interval
Use the inverse property of inequality and take the inverse of each term to rewrite the inequality.
Hence, theinequality proved in part (d) can be written as
(f)
To show:The result
(f)
Explanation of Solution
Proof:
Sandwich theorem: If a function
From part (e), the condition for Sandwich theorem the inequality can be written as:
The limit of the function
The limit of the function 1 at
Now, by Sandwich theorem
Hence, theresult
(g)
To show:The function
(g)
Explanation of Solution
Given information: The function is
Proof:
Even function: If
Substitute
Hence, it is proved that
(g)
To show:The result
(g)
Explanation of Solution
Given information: The function is
Proof:
It is known that if a function is even function, then its graph is symmetric about the y -axis.
In part (f), it is already proved that the right-hand limit of the function at 0 is 1. From part (g), it is proved that that
The left hand limit is:
Hence, the result
(h)
To show:The result
(h)
Explanation of Solution
Given information: The function is
Proof:
If the left hand limit and right hand limit of a function is equal, then the limit at that point is the same as RHL or LHL.
In above parts it is already proved that at
Hence, the result
Chapter 2 Solutions
Calculus: Graphical, Numerical, Algebraic: Solutions Manual
Additional Math Textbook Solutions
Calculus for Business, Economics, Life Sciences, and Social Sciences (14th Edition)
Thinking Mathematically (6th Edition)
Elementary Statistics
Elementary Statistics: Picturing the World (7th Edition)
Algebra and Trigonometry (6th Edition)
Calculus: Early Transcendentals (2nd Edition)
- uestion 10 of 12 A Your answer is incorrect. L 0/1 E This problem concerns hybrid cars such as the Toyota Prius that are powered by a gas-engine, electric-motor combination, but can also function in Electric-Vehicle (EV) only mode. The figure below shows the velocity, v, of a 2010 Prius Plug-in Hybrid Prototype operating in normal hybrid mode and EV-only mode, respectively, while accelerating from a stoplight. 1 80 (mph) Normal hybrid- 40 EV-only t (sec) 5 15 25 Assume two identical cars, one running in normal hybrid mode and one running in EV-only mode, accelerate together in a straight path from a stoplight. Approximately how far apart are the cars after 15 seconds? Round your answer to the nearest integer. The cars are 1 feet apart after 15 seconds. Q Search M 34 mlp CHarrow_forwardFind the volume of the region under the surface z = xy² and above the area bounded by x = y² and x-2y= 8. Round your answer to four decimal places.arrow_forwardУ Suppose that f(x, y) = · at which {(x, y) | 0≤ x ≤ 2,-x≤ y ≤√x}. 1+x D Q Then the double integral of f(x, y) over D is || | f(x, y)dxdy = | Round your answer to four decimal places.arrow_forward
- D The region D above can be describe in two ways. 1. If we visualize the region having "top" and "bottom" boundaries, express each as functions of and provide the interval of x-values that covers the entire region. "top" boundary 92(x) = | "bottom" boundary 91(x) = interval of values that covers the region = 2. If we visualize the region having "right" and "left" boundaries, express each as functions of y and provide the interval of y-values that covers the entire region. "right" boundary f2(y) = | "left" boundary fi(y) =| interval of y values that covers the region =arrow_forwardFind the volume of the region under the surface z = corners (0,0,0), (2,0,0) and (0,5, 0). Round your answer to one decimal place. 5x5 and above the triangle in the xy-plane witharrow_forwardGiven y = 4x and y = x² +3, describe the region for Type I and Type II. Type I 8. y + 2 -24 -1 1 2 2.5 X Type II N 1.5- x 1- 0.5 -0.5 -1 1 m y -2> 3 10arrow_forward
- Given D = {(x, y) | O≤x≤2, ½ ≤y≤1 } and f(x, y) = xy then evaluate f(x, y)d using the Type II technique. 1.2 1.0 0.8 y 0.6 0.4 0.2 0- -0.2 0 0.5 1 1.5 2 X X This plot is an example of the function over region D. The region identified in your problem will be slightly different. y upper integration limit Integral Valuearrow_forwardThis way the ratio test was done in this conflicts what I learned which makes it difficult for me to follow. I was taught with the limit as n approaches infinity for (an+1)/(an) = L I need to find the interval of convergence for the series tan-1(x2). (The question has a table of Maclaurin series which I followed as well) https://www.bartleby.com/solution-answer/chapter-92-problem-7e-advanced-placement-calculus-graphical-numerical-algebraic-sixth-edition-high-school-binding-copyright-2020-6th-edition/9781418300203/2c1feea0-c562-4cd3-82af-bef147eadaf9arrow_forwardSuppose that f(x, y) = y√√r³ +1 on the domain D = {(x, y) | 0 ≤y≤x≤ 1}. D Then the double integral of f(x, y) over D is [ ], f(x, y)dzdy =[ Round your answer to four decimal places.arrow_forward
- Consider the function f(x) = 2x² - 8x + 3 over the interval 0 ≤ x ≤ 9. Complete the following steps to find the global (absolute) extrema on the interval. Answer exactly. Separate multiple answers with a comma. a. Find the derivative of f (x) = 2x² - 8x+3 f'(x) b. Find any critical point(s) c within the intervl 0 < x < 9. (Enter as reduced fraction as needed) c. Evaluate the function at the critical point(s). (Enter as reduced fraction as needed. Enter DNE if none of the critical points are inside the interval) f(c) d. Evaluate the function at the endpoints of the interval 0 ≤ x ≤ 9. f(0) f(9) e. Based on the above results, find the global extrema on the interval and where they occur. The global maximum value is at a The global minimum value is at xarrow_forwardDetermine the values and locations of the global (absolute) and local extrema on the graph given. Assume the domain is a closed interval and the graph represents the entirety of the function. 3 y -6-5-4-3 2 1 -1 -2 -3 Separate multiple answers with a comma. Global maximum: y Global minimum: y Local maxima: y Local minima: y x 6 at a at a at x= at x=arrow_forwardA ball is thrown into the air and its height (in meters) is given by h (t) in seconds. -4.92 + 30t+1, where t is a. After how long does the ball reach its maximum height? Round to 2 decimal places. seconds b. What is the maximum height of the ball? Round to 2 decimal places. metersarrow_forward
 Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning
Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON
Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON
Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
 Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning
Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning





