Physics for Scientists and Engineers with Modern Physics, Technology Update
9th Edition
ISBN: 9781305401969
Author: SERWAY, Raymond A.; Jewett, John W.
Publisher: Cengage Learning
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 2, Problem 84CP
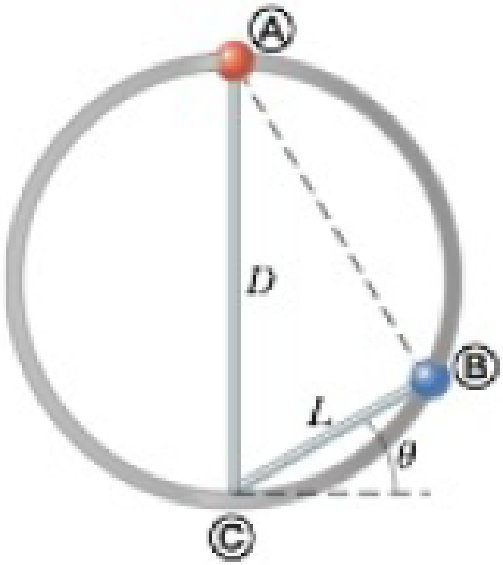
Two thin rods are fastened to the inside of a circular ring as shown in Figure P2.42. One rod of length D is vertical, and the other of length L makes an angle θ with the horizontal. The two rods and the ring lie in a vertical plane. Two small beads are free to slide without friction along the rods. (a) If the two beads are released from rest simultaneously from the positions shown, use your intuition and guess which bead reaches the bottom first. (b) Find an expression for the time interval required for the red head to fall from point Ⓐ to point Ⓒ in terms of g and D. (c) Find an expression for the time interval required for the blue bead to slide from point Ⓑ to point Ⓒ in terms of g, L, and θ. (d) Show that the two time intervals found in parts (b) and (c) are equal. Hint: What is the angle between the chords of the circle Ⓐ Ⓑ and Ⓑ Ⓒ? (e) Do these results surprise you? Was your intuitive guess in part (a) correct? This problem was inspired by an article by Thomas B. Greenslade, Jr., “Galileo’s Paradox,” Phys. Teach. 46, 294 (May 2008).
Figure P2.42
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
Over the holiday break you have an internship with an ice skating show. An ice skater will start from rest and slide down an ice-covered ramp. At the bottom of the ramp, the skater will glide around an ice-covered loop which is the inside of a vertical circle before emerging out onto the skating rink floor. For a spectacular effect, the circular loop will have a diameter of 30 feet. Your task is to determine the minimum height from the rink floor to the top of the ramp for the skater to make it around the loop. When barely making it around, the skater briefly loses contact with the ice at the top of the loop.
I'm not sure how to work this problem. The problem gives an answer of Itot=Icm+Md^2=18.1 kg*m^2 for part A. The problem also gives an answer of a=6.41 rad/s^2 for part B but does not show how to work the problems to get to these answers.
A pendulum has a length l (the rope is massless). The mass of the object suspended from the pendulum is m. With rope horizontal θ = 90o When it makes an angle of degrees, we first leave the object at no speed. Any friction can be neglected. Gravitational acceleration g. Give your answers in terms of l, m and g.
When = 0o, what is the tension in the rope?
Chapter 2 Solutions
Physics for Scientists and Engineers with Modern Physics, Technology Update
Ch. 2.1 - Under which of the following conditions is the...Ch. 2.2 - Are officers in the highway patrol more interested...Ch. 2.4 - Make a velocitytime graph for the car in Figure...Ch. 2.4 - If a car is traveling eastward and slowing down,...Ch. 2.5 - Which one of the following statements is true? (a)...Ch. 2.6 - In Figure 2.12, match each vxt graph on the top...Ch. 2.7 - Consider the following choices: (a) increases, (b)...Ch. 2 - Prob. 1OQCh. 2 - A racing car starts from rest at t = 0 and reaches...Ch. 2 - Prob. 3OQ
Ch. 2 - When applying the equations of kinematics for an...Ch. 2 - Prob. 5OQCh. 2 - Prob. 6OQCh. 2 - When the pilot reverses the propeller in a boat...Ch. 2 - Prob. 8OQCh. 2 - A skateboarder starts from rest and moves down a...Ch. 2 - Prob. 10OQCh. 2 - Prob. 11OQCh. 2 - A pebble is dropped from rest from the top of a...Ch. 2 - A student at the top of a building of height h...Ch. 2 - You drop a ball from a window located on an upper...Ch. 2 - A pebble is released from rest at a certain height...Ch. 2 - A ball is thrown straight up in the air. For which...Ch. 2 - Prob. 17OQCh. 2 - Each of the strobe photographs (a), (b), and (c)...Ch. 2 - If the average velocity of an object is zero in...Ch. 2 - Prob. 2CQCh. 2 - If a car is traveling eastward, can its...Ch. 2 - Prob. 4CQCh. 2 - Prob. 5CQCh. 2 - You throw a ball vertically upward so that it...Ch. 2 - (a) Can the equations of kinematics (Eqs....Ch. 2 - (a) Can the velocity of an object at an instant of...Ch. 2 - Two cars are moving in the same direction in...Ch. 2 - Position, Velocity, and Speed The position versus...Ch. 2 - The speed of a nerve impulse in the human body is...Ch. 2 - A person walks first at a constant speed of 5.00...Ch. 2 - A particle moves according to the equation x =...Ch. 2 - The position of a pinewood derby car was observed...Ch. 2 - Prob. 6PCh. 2 - A positiontime graph for a particle moving along...Ch. 2 - An athlete leaves one end of a pool of length L at...Ch. 2 - Find the instantaneous velocity of the particle...Ch. 2 - Prob. 10PCh. 2 - Prob. 11PCh. 2 - A car travels along a straight line at a constant...Ch. 2 - A person takes a trip, driving with a constant...Ch. 2 - Prob. 14PCh. 2 - Prob. 15PCh. 2 - A child rolls a marble on a bent track that is 100...Ch. 2 - Figure P2.9 shows a graph of vx versus t for the...Ch. 2 - (a) Use the data in Problem 3 to construct a...Ch. 2 - A particle starts from rest and accelerates as...Ch. 2 - An object moves along the x axis according to the...Ch. 2 - Prob. 21PCh. 2 - Draw motion diagrams for (a) an object moving to...Ch. 2 - Each of the strobe photographs (a), (b), and (c)...Ch. 2 - Prob. 24PCh. 2 - An electron in a cathode-ray tube accelerates...Ch. 2 - Prob. 26PCh. 2 - A parcel of air moving in a straight tube with a...Ch. 2 - A truck covers 40.0 m in 8.50 s while smoothly...Ch. 2 - An object moving with uniform acceleration has a...Ch. 2 - In Example 2.7, we investigated a jet landing on...Ch. 2 - Prob. 31PCh. 2 - Solve Example 2.8 by a graphical method. On the...Ch. 2 - Prob. 33PCh. 2 - Why is the following situation impossible?...Ch. 2 - Prob. 35PCh. 2 - Prob. 36PCh. 2 - Prob. 37PCh. 2 - Prob. 38PCh. 2 - A glider of length moves through a stationary...Ch. 2 - A glider of length 12.4 cm moves on an air track...Ch. 2 - Prob. 41PCh. 2 - At t = 0, one toy car is set rolling on a straight...Ch. 2 - Prob. 43PCh. 2 - Prob. 44PCh. 2 - Prob. 45PCh. 2 - An attacker at the base of a castle wall 3.65 m...Ch. 2 - Prob. 47PCh. 2 - Prob. 48PCh. 2 - Prob. 49PCh. 2 - The height of a helicopter above the ground is...Ch. 2 - Prob. 51PCh. 2 - Prob. 52PCh. 2 - Prob. 53PCh. 2 - At time t = 0, a student throws a set of keys...Ch. 2 - Prob. 55PCh. 2 - Prob. 56PCh. 2 - Prob. 57PCh. 2 - A student drives a moped along a straight road as...Ch. 2 - The speed of a bullet as it travels down the...Ch. 2 - Prob. 60APCh. 2 - The froghopper Philaenus spumarius is supposedly...Ch. 2 - Prob. 62APCh. 2 - Prob. 63APCh. 2 - In Figure 2.11b, the area under the velocitytime...Ch. 2 - Prob. 65APCh. 2 - A woman is reported to have fallen 144 ft from the...Ch. 2 - An elevator moves downward in a tall building at a...Ch. 2 - Prob. 68APCh. 2 - Prob. 69APCh. 2 - Prob. 70APCh. 2 - At t = 0, one athlete in a race running on a long,...Ch. 2 - Prob. 72APCh. 2 - Prob. 73APCh. 2 - Prob. 74APCh. 2 - Two objects, A and B, are connected by hinges to a...Ch. 2 - Prob. 76APCh. 2 - Prob. 77APCh. 2 - Prob. 78APCh. 2 - Prob. 79APCh. 2 - Prob. 80APCh. 2 - Prob. 81CPCh. 2 - Prob. 82CPCh. 2 - In a womens 100-m race, accelerating uniformly,...Ch. 2 - Two thin rods are fastened to the inside of a...Ch. 2 - Prob. 85CP
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.Similar questions
- A ball is held at rest at position A in the figure (Figure 1) by two light strings. The horizontal string is cut and the ball starts swinging as a pendulum. Point B is the farthest to the right the ball goes as it swings back and forth. What is the ratio of the tension in the supporting string in position B to its value at A before the horizontal string was cut?arrow_forwardA board 2.5 m long leans against a vertical wall. It starts to slide down the wall. The instant the bottom end is 2 m from the base of the wall, the top end is moving down the wall at the rate of 2 m/s. At that moment, how fast is the bottom sliding along the horizontal ground. 1.5 m/s 2 m/s 0.5 m/s 2.5 m/sarrow_forwardA Ferris wheel has a radius of 30 m and its centre is 32 m above the ground. It rotates once every 48 s. Ethan gets on the Feris wheel at the lowest point and then the wheel starts to rotate. The amount of time it takes Ethan to reach 38 m above the ground for the first time, to the nearest tenth of a second, is S. Your answerarrow_forward
- A physics lab instructor is working on a new demonstration. She attaches two identical copper balls with mass m = 0.180 g to threads of length L as shown in the figure. There are two strings in the figure. The top of each string is connected to the ceiling, and both strings are connected at the same point. The bottom of each string is connected to a spherical mass labeled m. Both strings have length L and hang at an angle of ? to the vertical, with the two strings on opposite sides of the vertical. Both balls have the same charge of 7.60 nC, and are in static equilibrium when ? = 4.75°. What is L (in m)? Assume the threads are massless. m (b) What If? The charge on both balls is increased until each thread makes an angle of ? = 9.50° with the vertical. If both balls have the same electric charge, what is the charge (in nC) on each ball in this case?arrow_forwardAt an amusement park there is a ride in which cylindrically shaped chambers spin around a central axis. People sit in seats facing the axis, their backs against the outer wall. At one instant the outer wall moves at a speed of 5 m/s, and a 50-kg person feels a 125-N force pressing against his back. What is the radius of a chamber in km?arrow_forwardI am currently really struggling in my physics class and really need help. I've reached out to both my teacher and school and neither are helping me. My teacher is really hard for me to understand so when learning the material I am left confused. I really do not know how to solve this problem or any problem like this at all. I need as much help as I can get.arrow_forward
- A 5.0 g coin is placed 15 cm from the center of a turntable. The coin has static and kinetic coefficients of friction with the turntable surface of μs = 0.70 and μk = 0.50. The turntable very slowly speeds up. What is the frequency of the rotation of the turntable expressed in revolutions per minute when the coin slides off? Express your answer in revolutions per minute.arrow_forwardAfter landing on an exoplanet, an astronaut constructs a simple pendulum of length 49 cm. The pendulum completes 89 full swing cycles in a time of 101 s. What is the magnitude of the gravitational acceleration in m/s2 on the exoplanet? Give your answer to 2 decimal places.arrow_forwardA skate park is formed by two semicircles of radius R1 = 8.3 m and R2 = 1.0 m and a flat central part, as shown in the following figure. The flat part has a length L = 3.6 m. The semicircles are tangent to the horizontal in B and C and to the vertical in A and D. There is no friction in the curved parts and in the flat part the coefficient of kinetic friction is μC = 0.2. A block with mass m = 3.2 kg is dropped from A. Suppose the acceleration due to gravity is 9.8 m / s2. What is the magnitude of the velocity of the block as it passes through point B the second time? Choose one: a. 45,4 m/sb. None of the other alternatives.c. 22,7 m/sd. 11,6 m/se. 12,0 m/sf. 5,7 m/sg. 7,6 m/sh. 129,0 m/sarrow_forward
- THE STRING ATTACHED TO THE KITE WEIGHS 0.4 OZ/FT. IF THE TENSION IN THE STRING IS 2.8 LB AT O AND 3.2 LB AT B, DETERMINE THE LENGTH S OF THE STRING AND THE HEIGHT H OF THE KITE.arrow_forwardA clown is juggling four balls simultaneously. Students use a video tape to determine that it takes the clown 0.9 s to cycle each ball through his hands (including catching, transferring, and throwing) and to be ready to catch the next ball. What is the minimum vertical speed the clown must throw each ball upward with?arrow_forwardA 64 kg skier encounters a dip in the snow's surface that has a circular cross section with a radius of curvature of 12 m. Figure 1 of 1 \r = 12 m A 1.75 marrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based Text
Physics
ISBN:9781133104261
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
Physics for Scientists and Engineers
Physics
ISBN:9781337553278
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...
Physics
ISBN:9781337553292
Author:Raymond A. Serway, John W. Jewett
Publisher:Cengage Learning
Newton's Second Law of Motion: F = ma; Author: Professor Dave explains;https://www.youtube.com/watch?v=xzA6IBWUEDE;License: Standard YouTube License, CC-BY