
In regions far from the entrance, fluid flow through a circular pipe is one dimensional, and the velocity profile for laminar flow is given by
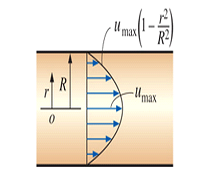
FIGURE P2-83
Want to see the full answer?
Check out a sample textbook solution
Chapter 2 Solutions
EBK FLUID MECHANICS: FUNDAMENTALS AND A
- Water flows between two parallel plates. The bottom plate is fixed and the top plate moves with the water. The velocity profile between the plates is linear with the highest velocity near the top plate. The pressure is Flow direction Velocity profile Ohighest upstream Ohighest downstream Oconstant along the flowarrow_forwardA plate moving 10 cm/s to the right is 0.5 cm above a stagnant wall. The fluid between the plate and the wall has a viscosity of 1.8 cP. Determine the shear rate.arrow_forwardA fluid of density ? and viscosity ? flows through a section of horizontal converging–diverging duct. The duct cross-sectional areas Ainlet, Athroat, and Aoutlet are known at the inlet, throat (minimum area), and outlet, respectively. Average pressure Poutlet is measured at the outlet, and average velocity Vinlet is measured at the inlet. (a) Neglecting any irreversibility such as friction, generate expressions for the average velocity and average pressure at the inlet and the throat in terms of the given variables. (b) In a real flow (with irreversibility), do you expect the actual pressure at the inlet to be higher or lower than the prediction? Explain.arrow_forward
- What do you mean by divergence of the velocity ? Write it's physical meaning.arrow_forwardpls replyyy Air is flowing through a square conduit whose section changes from 100 mm (section 1) to 200 (section 2). At section 1, v1=6 m/s and the density at section 1 is 1 kg/m3. If the velocity of flow is 1.8 m/s at section 2, find the density of gas at section 2arrow_forward7. Two parallel plates separated by 15.5 cm move with a relative velocity of 7 m/s. How much force per unit area is required to maintain the velocity difference if the fluid separating the plates is (a) water with µ = 1 cP (b) SAE 30 motor oil with µ = 200 cP and (c) honey with µ = 10,000 cP?arrow_forward
- Fluid mechanicsarrow_forward(1) Given the working form of the Bernoulli equation as dW - F dm Where 5 is the friction heating per unit mass dQ F = Au dm Given also that friction heating in laminar flow of Newtonian fluids in circular pipes is given as -AP F = = -gAz = Q Ax u 128 Ax is change in the x-direction. A typical capillary viscometer has a large-diameter reservoir and a long, small diameter, vertical tube. The sample is placed in the reservoir and the flow rate due to gravity is measured. The tube is 0.1 m long and has a 1 mm ID. The height of the fluid in the reservoir above the inlet to the tube is 0.02 m. The fluid being tested has a density of 1050 kg / m. The flow rate is 10* m³ / s. What is the viscosity of the fluid? Typical capillary viscometerarrow_forwardA liquid of density 1150 kg/m3 flows steadily through a pipe of varying diameter and height. At Location 1 along the pipe, the flow speed is 9.47 m/s and the pipe diameter d1 is 11.7 cm. At Location 2, the pipe diameter d2 is 17.7 cm. At Location 1, the pipe is Ay=8.19 m higher than it is at Location 2. Ignoring viscosity, calculate the difference APbetween the fluid pressure at Location 2 and the fluid pressure at Location 1.arrow_forward
- 4. In the regions far from the entrance, fluid flow through a circular pipe is one-dimensional, and the velocity profile for laminar flow is given by u(r) = Umax (1- r/R), where R is the radius of the pipe, r is the radial distance from the center of the pipe, and umax is the maximum flow velocity, which occurs at the centerline. Obtain (a) a relation for the drag force applied by the fluid on a section of the pipe of length L and (b) the value of the drag force of water flow at 20°C with R = 0.08m, L=15m and umax = 3m/s, and µ=0.0010 kg/m s. u(r) = Umax(1 – 72IR?) Umaxarrow_forwardDuring a trip to the beach (Patm = 101 kPa), a car runs out of gasoline (density is 750 kg/m^3), and it becomes necessary to siphon gas out of another nearby car to a tank of a volume of 4 litres using a hose whose cross-sectional area is 0.2 cm^2. The shown setting is used where L1=0.7 m and L2=2.2 m. Consider point 1 to be at the free surface of gasoline in the tank so that P_1= 1 atm, V_1 ~= 0 since the tank is large relative to the tube diameter. Consider the gravitational acceleration to be 9.81 m/s^2. Using Bernoulli equation, the pressure at point_3 in kPa is: Select one: O a. 72.55 Z3 Ob. 122.34 Gasoline OC. 79.66 siphoning tube L2 Gas tank L1 22 Gas can/arrow_forward2. A cylindrical shaft of radius 4 cm is rotating at a speed of 30 rad/s in a cylindrical bearing . Shaft and bearing are concentric . The lubricant of viscosity 0,4 Pa s and SG= 0,85 completely fills the clearance between shaft and bearing surface. Take clearance as 0,5 cm and length of shaft as 10 cm . The lubricant flow is laminar steady and uniform .Frictional force on shaft surface is ?Lütfen birini seçin: a. 4,47 N b. 2,41 N c. 5,53 N d. 9,42 N e. 1,23 Narrow_forward
 Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press
Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON
Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education
Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY
Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY
Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY





