
Concept explainers
(a)
The average acceleration of given particle.
(a)
Answer to Problem 63P
The average acceleration in interval AB is
Explanation of Solution
Given:
The variation of velocity of particle with time is plotted.
Formula used:
Write the expression for average acceleration.
Here,
Calculation:
Substitute
Substitute
Substitute
Conclusion:
Thus, the average acceleration in interval AB is
(b)
The positionof particle after given time interval.
(b)
Answer to Problem 63P
The particle is located at distance
Explanation of Solution
Formula used:
The displacement of the particle from the velocity-time graph is given by the area under the curve of the plot for the particular time interval.
The area under the graph for the interval
Write the expression for the area under the curve for the first interval.
Here,
The area under the graph for the interval
Write the expression for the area under the curve for the second interval.
Here,
The area under the graph for the interval
Write the expression for the area under the curve for the third interval.
Here,
The area under the graph for the interval
Write the expression for the area under the curve for the fourth interval.
Here,
Write the expression for displacement.
Here,
Calculation:
Substitute
Substitute
Substitute
Substitute
Substitute
Conclusion:
Thus the particle is located at distance
(c)
The plot of displacement of particle as a function of time
(c)
Answer to Problem 63P
The plot of displacement time graph is shown ion figure 1.
Explanation of Solution
The time interval BC is with constant velocity so in displacement time graph interval of BC will be straight line
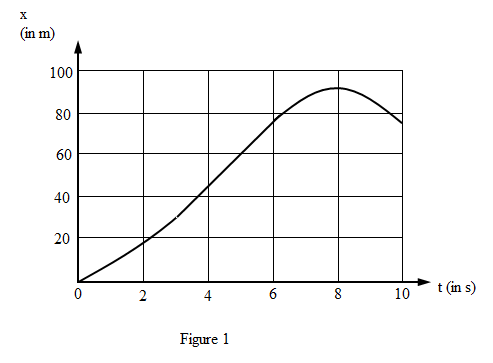
Conclusion:
Thus, the plot of displacement time graph is shown ion figure 1.
Want to see more full solutions like this?
Chapter 2 Solutions
Physics for Scientists and Engineers
- An automobile tire is shown in the figure below. The tire is made of rubber with a uniform density of 1.10 × 103 kg/m³. The tire can be modeled as consisting of two flat sidewalls and a tread region. Each of the sidewalls has an inner radius of 16.5 cm and an outer radius of 30.5 cm as shown, and a uniform thickness of 0.600 cm. The tread region can be approximated as having a uniform thickness of 2.50 cm (that is, its inner radius is 30.5 cm and outer radius is 33.0 cm as shown) and a width of 19.2 cm. What is the moment of inertia (in kg. m²) of the tire about an axis perpendicular to the page through its center? 2.18 x Sidewall 33.0 cm 30.5 cm 16.5 cm Treadarrow_forwardA person on horseback is on a drawbridge which is at an angle = 20.0° above the horizontal, as shown in the figure. The center of mass of the person-horse system is d = 1.35 m from the end of the bridge. The bridge is l = 7.00 m long and has a mass of 2,300 kg. A cable is attached to the bridge 5.00 m from the frictionless hinge and to a point on the wall h = 12.0 m above the bridge. The mass of person plus horse is 1,100 kg. Assume the bridge is uniform. Suddenly (and most unfortunately for the horse and rider), the ledge where the bridge usually rests breaks off, and at the same moment the cable snaps and the bridge swings down until it hits the wall. ÚI MAJI A TLA MAJA AUTA (a) Find the angular acceleration (magnitude, in rad/s²) of the bridge once it starts to move. 2.22 Use the rotational analogue of Newton's second law. The drawbridge can be modeled as a rod, with rotation axis about one end. rad/s² (b) How long (in s) does the horse and rider stay in contact with the bridge…arrow_forwardTwo long, parallel wires carry currents of I₁ = 2.70 A and I2 = 4.85 A in the directions indicated in the figure below, where d = 22.0 cm. (Take the positive x direction to be to the right.) 12 (a) Find the magnitude and direction of the magnetic field at a point midway between the wires. magnitude direction 3.91 270 μπ ⚫ counterclockwise from the +x axis (b) Find the magnitude and direction of the magnetic field at point P, located d = 22.0 cm above the wire carrying the 4.85-A current. magnitude direction Your response differs significantly from the correct answer. Rework your solution from the beginning and check each step carefully. μT The response you submitted has the wrong sign.° counterclockwise from the +x axisarrow_forward
- O Macmillan Learning The mass of a particular eagle is twice that of a hunted pigeon. Suppose the pigeon is flying north at Vi2 = 16.1 m/s when the eagle swoops down, grabs the pigeon, and flies off. At the instant right before the attack, the eagle is flying toward the pigeon at an angle 0 = 64.3° below the horizontal and a speed of Vi,1 = 37.9 m/s. What is the speed of of the eagle immediately after it catches its prey? What is the magnitude & of the angle, measured from horizontal, at which the eagle is flying immediately after the strike? Uf = II x10 TOOLS Vi.1 Vi,2 m/sarrow_forwardWhat is the equivalent resistance if you connect a 1.7 Ohm, a 9.3 Ohm, and a 22 Ohm resistor in series? (Give your answer as the number of Ohms.)arrow_forwardThree wires meet at a junction. One wire carries a current of 5.2 Amps into the junction, and a second wire carries a current of 3.7 Amps out of the junction. What is the current in the third wire? Give your answer as the number of Amps, and give a positive number if the current in that wire flows out of the junction, or a negative number if the current in that wire flows into the junction.arrow_forward
- What is the equivalent resistance if you connect a 4.5 Ohm, a 6.8 Ohm, and a 15 Ohm resistor in parallel? (Give your answer as the number of Ohms.)arrow_forwardSuppose a heart defibrillator passes 10.5 Amps of current through a patient's torso for 5.0 x 10-3 seconds in order to restore a regular heartbeat. The voltage across the defibrillator is 9800 volts for the entire time that current is flowing. If 7.25 kg of body tissue is involved, with a specific heat of 3500 J/(kg°C), then what is the resulting temperature increase of the person's torso? (Give your answer as the number of degrees C.)arrow_forwardThe figure below is a cross-sectional view of a coaxial cable. The center conductor is surrounded by a rubber layer, an outer conductor, and another rubber layer. In a particular application, the current in the inner conductor is I₁ = 1.04 A out of the page and the current in the outer conductor is I2 = 2.90 A into the page. Assuming the distance d = 1.00 mm, answer the following. 4 12 (a) Determine the magnitude and direction of the magnetic field at point a. magnitude 208 direction upward (b) Determine the magnitude and direction of the magnetic field at point b. magnitude direction 238 You can approach this problem by finding the field produced by current I₁ and the field produced by I2 and then adding them vectorially. μT downwardarrow_forward
- Shoto, from My Hero Academia, has a power (or a “quirk”) that allows him to make large amounts of ice from nothing. Let us say that due to a fire a 361 kg steel beam is heated to 943.˚C and Shoto creates 390. kg of ice at 0.00˚C around it to cool it down. What is the final temperature of the system after the ice melts and it reaches thermal equilibrium? The specific heat of steel is 502 J/kg˚C. The specific heat of water is 4186 J/kg˚C. The latent heat of fusion for ice is 3.33⋅10^5 J/kg.arrow_forwardA 25.0 cm long organ pipe is filled with air and is open at one end and closed at the other. The speed of sound in air at 0°C is 331 m/s. What is the frequency of the fourth mode of vibration? Multiple Choice О 1,550 Hz О 1,750 Hz О 2,320 Hz О 2,720 Hz О 3,170 Hzarrow_forward23.4 g of coffee beans at room temperature (18.6 °C) is mixed into 316 g of water at 96.8 °C in an effort to make coffee. The entire system is poured in a 363 g ceramic mug. Assume the mug is initally also at room temperature (18.6 °C). What is the final temperature of the mixture? The specific heat of ground coffee beans is 1670 J/kg˚C, the specific heat of water is 4186 J/kg˚C, and the specific heat of the mug is 850. J/kg˚C.arrow_forward
 Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning University Physics Volume 1PhysicsISBN:9781938168277Author:William Moebs, Samuel J. Ling, Jeff SannyPublisher:OpenStax - Rice University
University Physics Volume 1PhysicsISBN:9781938168277Author:William Moebs, Samuel J. Ling, Jeff SannyPublisher:OpenStax - Rice University Glencoe Physics: Principles and Problems, Student...PhysicsISBN:9780078807213Author:Paul W. ZitzewitzPublisher:Glencoe/McGraw-Hill
Glencoe Physics: Principles and Problems, Student...PhysicsISBN:9780078807213Author:Paul W. ZitzewitzPublisher:Glencoe/McGraw-Hill College PhysicsPhysicsISBN:9781938168000Author:Paul Peter Urone, Roger HinrichsPublisher:OpenStax College
College PhysicsPhysicsISBN:9781938168000Author:Paul Peter Urone, Roger HinrichsPublisher:OpenStax College Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning





