
Review. A house roof is a perfectly flat plane that makes an angle θ with the horizontal. When its temperature changes, between Ti before dawn each day and Tk in the middle of each afternoon, the roof expands and contracts uniformly with a coefficient of thermal expansion α1. Resting on the roof is a flat, rectangular metal plate with expansion coefficient α2, greater than α1. The length of the plate is L, measured along the slope of the roof. The component of the plate’s weight perpendicular to the roof is supported by a normal force uniformly distributed over the area of the plate. The coefficient of kinetic friction between the plate and the roof is μk. The plate is always at the same temperature as the roof, so we assume its temperature is continuously changing. Because of the difference in expansion coefficients, each bit of the plate is moving relative to the roof below it, except for points along a certain horizontal line running across the plate called the stationary line. If the temperature is rising, parts of the plate below the stationary line are moving down relative to the roof and feel a force of kinetic friction acting up the roof. Elements of area above the stationary line are sliding up the roof, and on them kinetic friction acts downward parallel to the roof. The stationary line occupies no area, so we assume no force of static friction acts on the plate while the temperature is changing. The plate as a whole is very nearly in equilibrium, so the net
below the top edge of the plate. (b) Analyze the forces that act on the plate when the temperature is falling and prove that the stationary line is at that same distance above the bottom edge of the plate. (c) Show that the plate steps down the roof like an inchworm, moving each day by the distance
(d) Evaluate the distance an aluminum plate moves each day if its length is 1.20 m, the temperature cycles between 4.00°C and 36.0°C, and if the roof has slope 18.5°, coefficient of linear expansion 1.50 × 10−5 (°C) −1, and coefficient of friction 0.420 with the plate. (e) What If? What if the expansion coefficient of the plate is less than that of the roof? Will the plate creep up the roof?
(a)
To show: The stationary line is at distance of
Answer to Problem 44CP
Explanation of Solution
Given info: The angle made by the roof with the horizontal plane is
Consider the figure given below.
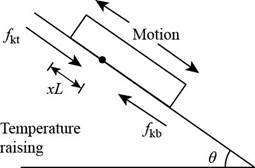
Figure 1
Consider that
The normal force on the lower part of the plane is,
Here,
The force due to gravity is,
The equation for the kinematic friction force is,
The equation for the downward force is,
The force equation for the plate is,
Further, solve for
The distance of the stationary line below the top edge is,
Conclusion:
Therefore, the distance at which stationary line lie is
(b)
To show: The stationary line is at that same distance above the bottom edge of the plate.
Answer to Problem 44CP
Explanation of Solution
Given info: The angle made by the roof with the horizontal plane is
Consider the figure given below.
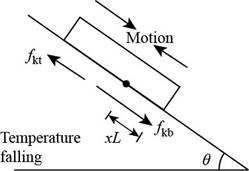
Figure 2
With the temperature falling, the plate contracts faster than the roof. The upper part slides down and feels an upward frictional force,
Then the force equation remains same as in part (a) and the stationary line is above the bottom edge by,
Conclusion:
Therefore, it is proved that the stationary line is at that same distance above the bottom edge of the plate.
(c)
To show: The plate steps down the roof like an inchworm moving each day by the distance
Answer to Problem 44CP
Explanation of Solution
Given info: The angle made by the roof with the horizontal plane is
Consider the figure given below.
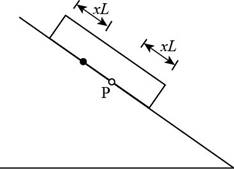
Figure 3
Consider the plate at dawn, as the temperature starts to rise. As in part (a), a line at distance
In the above figure, the point
The change in the length of the plate is,
The change in the length of the roof is,
The point on the roof originally under point
When the temperature drops, point
The displacement for a day is,
Substitute
Conclusion:
Therefore, the distance by which the plate steps down the roof like an inchworm moving each day is
(d)
Answer to Problem 44CP
Explanation of Solution
Given info: The angle made by the roof with the horizontal plane is
The length of the plate is
The coefficient of linear expansion for aluminum is
The formula for the displacement for a day is,
Substitute
Conclusion:
Therefore, the distance an aluminum plate moves each day is
(e)
To explain: The effect on the plate if the expansion coefficient of the plate is less than the expansion coefficient of the roof.
Answer to Problem 44CP
Explanation of Solution
Given info: The angle made by the roof with the horizontal plane is
If
The figure I, applies to the temperature falling and figure II applies to temperature rising. A point on the plate
The plate creeps down the roof each day by an amount given by,
Conclusion:
Therefore, the plate creeps down the roof each day by an amount given by
Want to see more full solutions like this?
Chapter 18 Solutions
PHYSICS:F/SCI.+ENGRS-W/WEBASSIGN
- A thrown brick hits a window, but doesn't break it. Instead it reverses direction and ends down on the ground below the window. Since the brick didn't break the glass, we know: О The force of the brick on the glass > the force of the glass on the brick. О The force of the brick on the glass the force of the glass on the brick. = О The force of the brick on the glass < the force of the glass on the brick. О The brick didn't slow down as it broke the glass.arrow_forwardAlexandra (wearing rubber boots for traction) is attempting to drag her 32.6-kg Golden Retriever across the smooth ice by applying a horizontal force. What force must she apply to move the dog with a constant speed of 0.950 m/s? ☐ 31.0 lb. ☐ 319 kg. ○ Zero. 32.6 kg.arrow_forwardThe figure shows a graph of the acceleration of an object as a function of the net force acting on it. The mass of this object, in grams, is closest to 11 a(m/s²) 8.0+ 6.0- 4.0- 2.0- 0+ F(N) 0.00 0.50 1.00 ☐ 130 ○ 8000 ☐ 89arrow_forward
- Values that are within standard deviations represent measurements that are considered to be near the true value. Review the data from the lab and determine whether your data is within standard deviations. Report, using numerical values, whether your data for each angle is within standard deviations. An acceptable margin of error typically falls between 4% and 8% at the 95% confidence level. Review your data for each angle to determine whether the margin of error is within an acceptable range. Report with numerical values, whether your data for each angle is within an acceptable margin of error. Can you help explain what my data means in terms of the standard deviation and the ME? Thanks!arrow_forwardA sinusoidal wave is propagating along a stretched string that lies along the x-axis. The displacement of the string as a function of time is graphed in (Figure 1) for particles at x = 0 and at x = 0.0900 m. You are told that the two points x = 0 and x = 0.0900 m are within one wavelength of each other. If the wave is moving in the +x-direction, determine the wavelength. If instead the wave is moving in the -x-direction, determine the wavelength. Please show all stepsarrow_forwardYou are designing a two-string instrument with metal strings 35.0 cm long, as shown in (Figure 1). Both strings are under the same tension. String S1 has a mass of 8.30 g and produces the note middle C (frequency 262 Hz ) in its fundamental mode. What should be the tension in the string? What should be the mass of string S2 so that it will produce A-sharp (frequency 466 Hz ) as its fundamental? To extend the range of your instrument, you include a fret located just under the strings but not normally touching them. How far from the upper end should you put this fret so that when you press S1 tightly against it, this string will produce C-sharp (frequency 277 Hz ) in its fundamental? That is, what is x in the figure? If you press S2 against the fret, what frequency of sound will it produce in its fundamental?arrow_forward
- Please solve and answer the problem correctly please. Thank you!!arrow_forwardPlease help explain this. The experiment without the sandpaper had a 5% experimental error, with sandpaper it is 9.4%. Would the explaination be similar to the experiment without sandpaper? Thanks!arrow_forwardA sinusoidal wave with wavelength 0.400 m travels along a string. The maximum transverse speed of a point on the string is 3.00 m/s and the maximum transverse acceleration is 8.10×104m/s2. What is the propagation speed v of the wave? What is the amplitude A of the wave?arrow_forward
 Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning College PhysicsPhysicsISBN:9781285737027Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
College PhysicsPhysicsISBN:9781285737027Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning





