
Concept explainers
Consider the
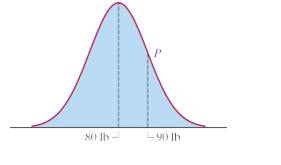
Figure 17-12
a. Find the mean
b. Find the median M of the distribution.
c. Find the standard deviation
(a)
To find:
The mean
Answer to Problem 1E
Solution:
The mean
Explanation of Solution
Given:
The normal distribution curve is,
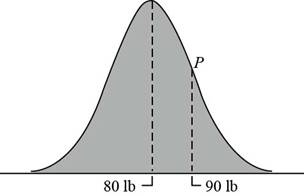
With P as a point of inflection of the curve.
Definition:
The point of intersection of the horizontal (data) axis and the line of symmetry of the normal curve, the center of the distribution. The center corresponds to both the median and mean of the distribution.
Calculation:
From the given figure, it is seen that the point of intersection of the horizontal axis and the line of symmetry is at
This implies that the mean
Conclusion:
The mean
(b)
To find:
The median M of the distribution.
Answer to Problem 1E
Solution:
The median M of the distribution is
Explanation of Solution
Given:
The normal distribution curve is,
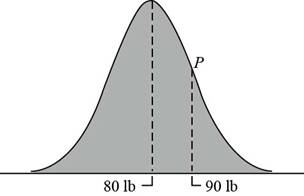
With P as a point of inflection of the curve.
Definition:
The point of intersection of the horizontal (data) axis and the line of symmetry of the normal curve, the center of the distribution. The center corresponds to both the median and mean of the distribution.
Calculation:
From the given figure, it is seen that the point of intersection of the horizontal axis and the line of symmetry is at
This implies that the median M of the distribution.is
Conclusion:
The median M of the distribution is
(c)
To find:
The standard deviation
Answer to Problem 1E
Solution:
The standard deviation
Explanation of Solution
Given:
The normal distribution curve is,
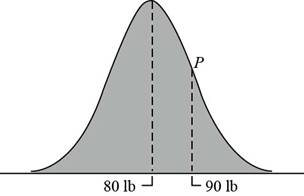
With P as a point of inflection of the curve.
Definition:
The standard deviation of the normal distribution is the horizontal distance between the axis of symmetry of the curve and one of the two points of inflection.
Calculation:
From the given figure, it is seen that the point of intersection of the horizontal axis and the line of symmetry is at
The point of inflection of the curve is
Therefore the distance between the point of inflection and the line of symmetry is,
This implies that the standard deviation
Conclusion:
The standard deviation
Want to see more full solutions like this?
Chapter 17 Solutions
Excursions In Modern Mathematics, 9th Edition
- question 3 part a and barrow_forwarddo question 2arrow_forward21. ANALYSIS OF LAST DIGITS Heights of statistics students were obtained by the author as part of an experiment conducted for class. The last digits of those heights are listed below. Construct a frequency distribution with 10 classes. Based on the distribution, do the heights appear to be reported or actually measured? Does there appear to be a gap in the frequencies and, if so, how might that gap be explained? What do you know about the accuracy of the results? 3 4 555 0 0 0 0 0 0 0 0 0 1 1 23 3 5 5 5 5 5 5 5 5 5 5 5 5 6 6 8 8 8 9arrow_forward
- A side view of a recycling bin lid is diagramed below where two panels come together at a right angle. 45 in 24 in Width? — Given this information, how wide is the recycling bin in inches?arrow_forwardf'(x)arrow_forwardIf you are using chatgpt leave it I will downvote .arrow_forwardTemperature measurements are based on the transfer of heat between the sensor of a measuring device (such as an ordinary thermometer or the gasket of a thermocouple) and the medium whose temperature is to be measured. Once the sensor or thermometer is brought into contact with the medium, the sensor quickly receives (or loses, if warmer) heat and reaches thermal equilibrium with the medium. At that point the medium and the sensor are at the same temperature. The time required for thermal equilibrium to be established can vary from a fraction of a second to several minutes. Due to its small size and high conductivity it can be assumed that the sensor is at a uniform temperature at all times, and Newton's cooling law is applicable. Thermocouples are commonly used to measure the temperature of gas streams. The characteristics of the thermocouple junction and the gas stream are such that λ = hA/mc 0.02s-1. Initially, the thermocouple junction is at a temperature Ti and the gas stream at…arrow_forwardA body of mass m at the top of a 100 m high tower is thrown vertically upward with an initial velocity of 10 m/s. Assume that the air resistance FD acting on the body is proportional to the velocity V, so that FD=kV. Taking g = 9.75 m/s2 and k/m = 5 s, determine: a) what height the body will reach at the top of the tower, b) how long it will take the body to touch the ground, and c) the velocity of the body when it touches the ground.arrow_forwardA chemical reaction involving the interaction of two substances A and B to form a new compound X is called a second order reaction. In such cases it is observed that the rate of reaction (or the rate at which the new compound is formed) is proportional to the product of the remaining amounts of the two original substances. If a molecule of A and a molecule of B combine to form a molecule of X (i.e., the reaction equation is A + B ⮕ X), then the differential equation describing this specific reaction can be expressed as: dx/dt = k(a-x)(b-x) where k is a positive constant, a and b are the initial concentrations of the reactants A and B, respectively, and x(t) is the concentration of the new compound at any time t. Assuming that no amount of compound X is present at the start, obtain a relationship for x(t). What happens when t ⮕∞?arrow_forwardarrow_back_iosSEE MORE QUESTIONSarrow_forward_ios
 Big Ideas Math A Bridge To Success Algebra 1: Stu...AlgebraISBN:9781680331141Author:HOUGHTON MIFFLIN HARCOURTPublisher:Houghton Mifflin Harcourt
Big Ideas Math A Bridge To Success Algebra 1: Stu...AlgebraISBN:9781680331141Author:HOUGHTON MIFFLIN HARCOURTPublisher:Houghton Mifflin Harcourt Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill

