Mathematics for Elementary Teachers with Activities, Books a la carte edition (5th Edition)
5th Edition
ISBN: 9780134423319
Author: Sybilla Beckmann
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 15.4, Problem 3P
The three histograms in Figure 15.37 show the hypotheticalperformance of students in three different school districts on thesame test. A score below 40 on the test is considered failing. Ascore of 80 or above is considered excellent.
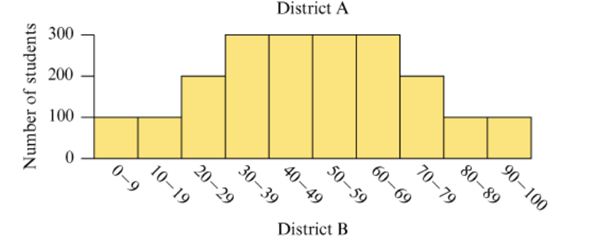
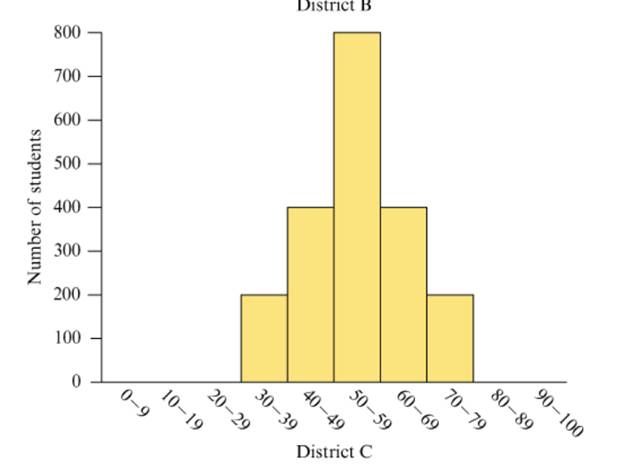
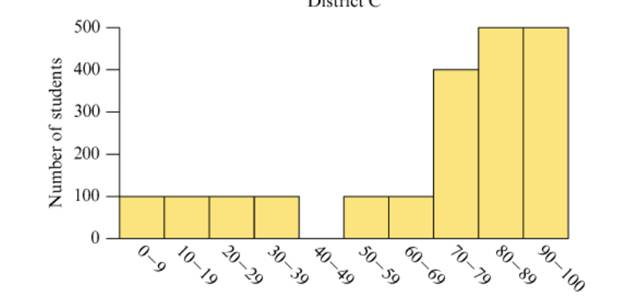
Figure 15.37 Performance of three hypothetical schooldistricts on a test.
a. Estimate the mean score on the test for each school district by viewing the mean as a balance point, as discussed in Section 15.3.
b. Discuss what information you can glean from the histograms that wouldn’t be apparent just from knowing the mean or median scores on the test.
c. Discuss how each school district could argue that it did better than at least one other school district.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
Given the following set
X = {2, 4, 6, 8} and Y = {1, 2, 3},
explicitly give (e.g., write down the sets with numerical entries) of the outputs of the
following requested set operations:
(a) [2 points] XUY (Union)
(b) [2 points] XY (Intersection)
(c) [3 points] X\Y (Difference)
(d) [3 points] XAY (Symmetric Difference)
4.2 Product and Quotient Rules
1.
9(x)=125+1
y14+2
Use the product and/or quotient rule to find the derivative of each function.
a. g(x)=
b. y (2x-3)(x-1)
c. y==
3x-4
√x
4.2 Product and Quotient Rules
1. Use the product and/or quotient rule to find the derivative of each function.
2.5
a. g(x)=+1
y14+2
√x-1)
b. y=(2x-3)(x-:
Chapter 15 Solutions
Mathematics for Elementary Teachers with Activities, Books a la carte edition (5th Edition)
Ch. 15.1 - Give two examples of a statistical question and...Ch. 15.1 - A class has a collection of 100 bottle caps and...Ch. 15.1 - Neil, a third-grader, asked 10 of his classmates...Ch. 15.1 - An announcer of a TV program invited viewers to...Ch. 15.1 - There is a bowl containing 300 plastic tiles. Some...Ch. 15.1 - There is a bowl containing 80 green tiles and an...Ch. 15.1 - At a factory that produces doorknobs, 1500...Ch. 15.1 - At a factory that produces switches, 3000 switches...Ch. 15.1 - At a light bulb factory, 1728 light bulbs are...Ch. 15.1 - Carter has a large collection of marbles. He knows...
Ch. 15.1 - The following problem is an example of the...Ch. 15.1 - A group studying violence wants to determine the...Ch. 15.2 - Three third-grade classes are having a contest to...Ch. 15.2 - Find 3 coins of any type (as long as all 3 have a...Ch. 15.2 - Table 15.3 shows women’s 400-meter freestyle...Ch. 15.2 - Using an Internet browser, go to census.gov, the...Ch. 15.2 - Using an Internet browser, go to the webpage of...Ch. 15.2 - Using an Internet browser, go to one of the...Ch. 15.2 - Using an Internet browser, go to one of the...Ch. 15.2 - a. Describe in detail an activity suitable for use...Ch. 15.3 - In your own words, explain why the following two...Ch. 15.3 - Explain why the mean of a list of numbers must...Ch. 15.3 - Explain why the mean of two numbers is exactly...Ch. 15.3 - Shante caught 17 ladybugs every day for 4 days....Ch. 15.3 - John’s average annual income over a 4-year period...Ch. 15.3 - Tracy’s times swimming 200 yards were as follows:...Ch. 15.3 - Explain how you can quickly calculate the mean of...Ch. 15.3 - Explain how you can quickly calculate the mean of...Ch. 15.3 - average on her first 3 math tests was 80. Her...Ch. 15.3 - A teacher gives a 10-point test to a class of 10...Ch. 15.3 - In your own words, describe how to view the mean...Ch. 15.3 - Discuss Jessica’s reasoning about calculating the...Ch. 15.3 - ]The dot plot in Figure 15.21 represents the...Ch. 15.3 - For each of the following situations, decide...Ch. 15.3 - A teacher gives a 10-point test to a class of 9...Ch. 15.3 - A teacher gives a 10-point test to a class of 9...Ch. 15.3 - In Ritzy County, the average annual household...Ch. 15.3 - In county A, the average score on the grade 5 Iowa...Ch. 15.3 - a. The histogram at the top of Figure 15.22 shows...Ch. 15.3 - * Ms. Smith needs to figure her students’ homework...Ch. 15.3 - The average speed of a moving object during a...Ch. 15.4 - What is the difference between scoring in the 90th...Ch. 15.4 - What is the purpose of reporting a student’s...Ch. 15.4 - The three histograms in Figure 15.37 show the...Ch. 15.4 - At a math center in a class, there is a bag filled...Ch. 15.4 - Refer to Figure 15.26 on page 706. a. Refer to the...Ch. 15.4 - Refer to Figure 15.26 on page 706. a. Refer to the...Ch. 15.4 - Refer to Figure 15.26 on page 706. a. Write at...Ch. 15.4 - Use the NAEP long-term trend data about...Ch. 15.4 - Determine the median and interquartile range for...Ch. 15.4 - a. Make a box plot for the data in Figure 15.38....Ch. 15.4 - Determine the medians and interquartile ranges for...Ch. 15.4 - a. Make box plots for the dot plots in Figure...Ch. 15.4 - A fifth-grade class is wondering ¡f girls’ names...Ch. 15.4 - Compute the mean and the MAD for each of the dot...Ch. 15.4 - On a state-wide test graded on a 10-point scale,...Ch. 15.4 - A 400-point test is given to a group of students....
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, subject and related others by exploring similar questions and additional content below.Similar questions
- For what values of k will the equation (k + 1)x² + 6kx + 2k² - x = 0 have: a) one root equal zero b) one root the reciprocal of the other c) roots numerically equal but of opposite signarrow_forward3. The total profit (in dollars) from selling x watches is P(x)=0.52x²-0.0002x². Find and interpret the following. a) P(100) b) P'(100)arrow_forward3. Find the slope and the equation of the tangent line to the graph of the given function at the given value of x. -4 f(x)=x-x³;x=2arrow_forward
- 2. Find the equation of the tangent line to the graph of the given function at the given point. f(x)=(x+3)(2x²-6) at (1,-16)arrow_forward6. Researchers who have been studying the alarming rate at which the level of the Dead Sea has been dropping have shown that the density d (x) (in g per cm³) of the Dead Sea brine during evaporation can be estimated by the function d(x)=1.66 0.90x+0.47x², where x is the fraction of the remaining brine, 0≤x≤1. a) Estimate the density of the brine when 60% of the brine remains. b) Find and interpret the instantaneous rate of change of the density when 60% of the brine remains.arrow_forward5. If g'(5) 10 and h'(5)=-4, find f'(5) for f(x)=4g(x)-2h(x)+3.arrow_forward
- 2. Find each derivative. Write answers with positive exponents. a) Dx 9x -3 [97] b) f'(3) if f(x) = x²-5x² 8arrow_forwardT3.2: Prove that if the Graceful Tree Conjecture (every tree has a graceful labeling) is true and T' is a tree with m edges, then K2, decomposes into 2m - 1 copies of T. Hint - Delete a leaf to get 7" and apply the decomposition of K2(m-1)+1 = K2m-1 into T'. Then explain how the decomposition allows the pendant edge to be added to a new vertex to obtain a decomposition of K2m into copies of T.arrow_forwardUse the matrix tree theorem to determine the number of spanning trees of the graphs Kr∨sK1.These are the graphs formed by by adding all edges between a complete graph on r vertices and atrivial graph (no edges) on s vertices.arrow_forward
- The maximum capacity spanning tree problem is as follows for a given graph G = (V, E) withcapacities c(uv) on the edges. The capacity of a tree T is defined as the minimum capacity of anedge in T. The maximum capacity spanning tree problem is to determine the maximum capacity ofa spanning tree.(i) Describe how to modify the input graph to find a maximum weight spanning tree making use ofa minimum weight spanning tree algorithm.(ii) Show that a maximum (weight) spanning tree is also a maximum capacity spanning tree.(iii) Is the converse of part (ii) true? That is, is it true that a maximum capacity spanning tree is alsoa maximum spanning tree? Either give counterexamples (of all sizes) or a proof.(iv) Prove the following max-min result. The maximum capacity of a spanning tree is equal to theminimum bottleneck value of a cut. For a subset U ⊆ V , the cut [U, V − U] is the set of edgesbetween U and V − U. The bottleneck value of a cut [U, V − U] is the largest capacity among theedges of…arrow_forward1) Find The inverse The domain of m(x) = tion and of the function The inverse function 3- √x-aarrow_forwardProve that the following version of a greedy algorithm produces a minimum spanning tree in aweighted graph. Start with a vertex v as the initial tree and at each stage add an edge with minimumweight having exactly one end in the current tree. Stop when all vertices have been addedarrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Big Ideas Math A Bridge To Success Algebra 1: Stu...AlgebraISBN:9781680331141Author:HOUGHTON MIFFLIN HARCOURTPublisher:Houghton Mifflin Harcourt
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Glencoe Algebra 1, Student Edition, 9780079039897...
Algebra
ISBN:9780079039897
Author:Carter
Publisher:McGraw Hill
Big Ideas Math A Bridge To Success Algebra 1: Stu...
Algebra
ISBN:9781680331141
Author:HOUGHTON MIFFLIN HARCOURT
Publisher:Houghton Mifflin Harcourt
Holt Mcdougal Larson Pre-algebra: Student Edition...
Algebra
ISBN:9780547587776
Author:HOLT MCDOUGAL
Publisher:HOLT MCDOUGAL
The Shape of Data: Distributions: Crash Course Statistics #7; Author: CrashCourse;https://www.youtube.com/watch?v=bPFNxD3Yg6U;License: Standard YouTube License, CC-BY
Shape, Center, and Spread - Module 20.2 (Part 1); Author: Mrmathblog;https://www.youtube.com/watch?v=COaid7O_Gag;License: Standard YouTube License, CC-BY
Shape, Center and Spread; Author: Emily Murdock;https://www.youtube.com/watch?v=_YyW0DSCzpM;License: Standard Youtube License